Accounting and Finance Assignment: EMI, Annuity, and NPV Analysis

This document provides a comprehensive solution to a Master of Accounting statistics assignment. The assignment covers core financial concepts, including the calculation of EMI (Equated Monthly Installment) using the provided formula and real-world scenarios with different loan amounts, interest ra...Read More

MASTER OF ACCOUNTING-STATS
STUDENT ID:
[Pick the date]

Question 1
The requisite formula for EMI computation is shown below.
For the given scenario, P = $ 1,000,000, R = 6% p.a. or 0.5% per month, N= 30 years or 360
months
Hence, EMI = (1000000*0.005*1.005360)/(1.005360-1) = $5,995.51
Question 2
a) Effective annual interest rate = (1 + (12/200))2 -1 = 12.36%
b) Effective annual interest rate = (1 + (12/400))4 -1 = 12.55%
c) Effective annual interest rate = (1 + (12/36500))365 -1 = 12.75%
d) Effective annual interest rate = (e0.12) -1 = 12.77%
Question 3
The relevant formula for determining future value of annuity is indicated below.
a) Here P= $1,000, r= 12% p.a., n=3
Hence, FV of annuity at the end of three years = 1000*(1.123-1)/0.12 = $3,374.4
b) Here P= $1,000, r= 12% p.a., n=5

Hence, FV of annuity at the end of five years = 1000*(1.125-1)/0.12 = $6,382.85
c) It is imperative to note that for 5th and 6th year, there is no annuity payment. As a result, the
future value obtained at the end of year 5 would be compounded till the end of year 7.
Hence, FV of annuity at the end of seven years = $6,382.85*1.122 = $7,969.01
Question 4
The present value of annuity can be estimated using the following formula.
The lottery essentially consists of two streams as indicated below.
Stream 1- $ 40,000 per annum from Year 1 to Year 10
Stream 2 - $ 60,000 per annum from Year 11 to Year 20
Rate of interest (r) = 10% p.a.
Present value of stream 1 = 40000*(1-1.1-10)/0.1 = $245,782.7
Present value of stream 2 = [60000*(1-1.1-10)/0.1]/(1.110) = $142,139.8
Hence, value of proceeds of lottery in present terms = $245,782.7 + $142,139.8 = $387,922.50
Question 5
The requisite formula for EMI computation is shown below.

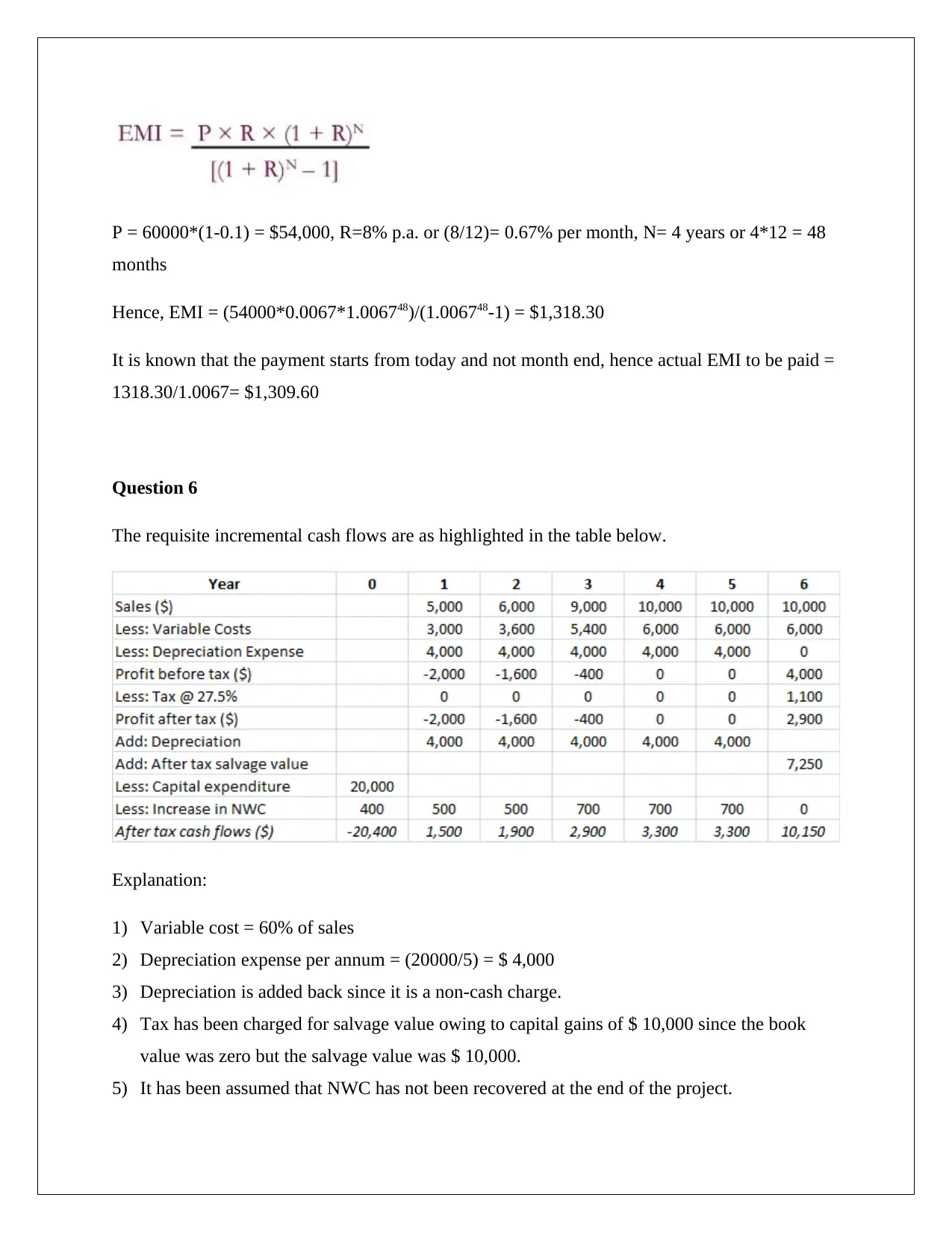
P = 60000*(1-0.1) = $54,000, R=8% p.a. or (8/12)= 0.67% per month, N= 4 years or 4*12 = 48
months
Hence, EMI = (54000*0.0067*1.006748)/(1.006748-1) = $1,318.30
It is known that the payment starts from today and not month end, hence actual EMI to be paid =
1318.30/1.0067= $1,309.60
Question 6
The requisite incremental cash flows are as highlighted in the table below.
Explanation:
1) Variable cost = 60% of sales
2) Depreciation expense per annum = (20000/5) = $ 4,000
3) Depreciation is added back since it is a non-cash charge.
4) Tax has been charged for salvage value owing to capital gains of $ 10,000 since the book
value was zero but the salvage value was $ 10,000.
5) It has been assumed that NWC has not been recovered at the end of the project.

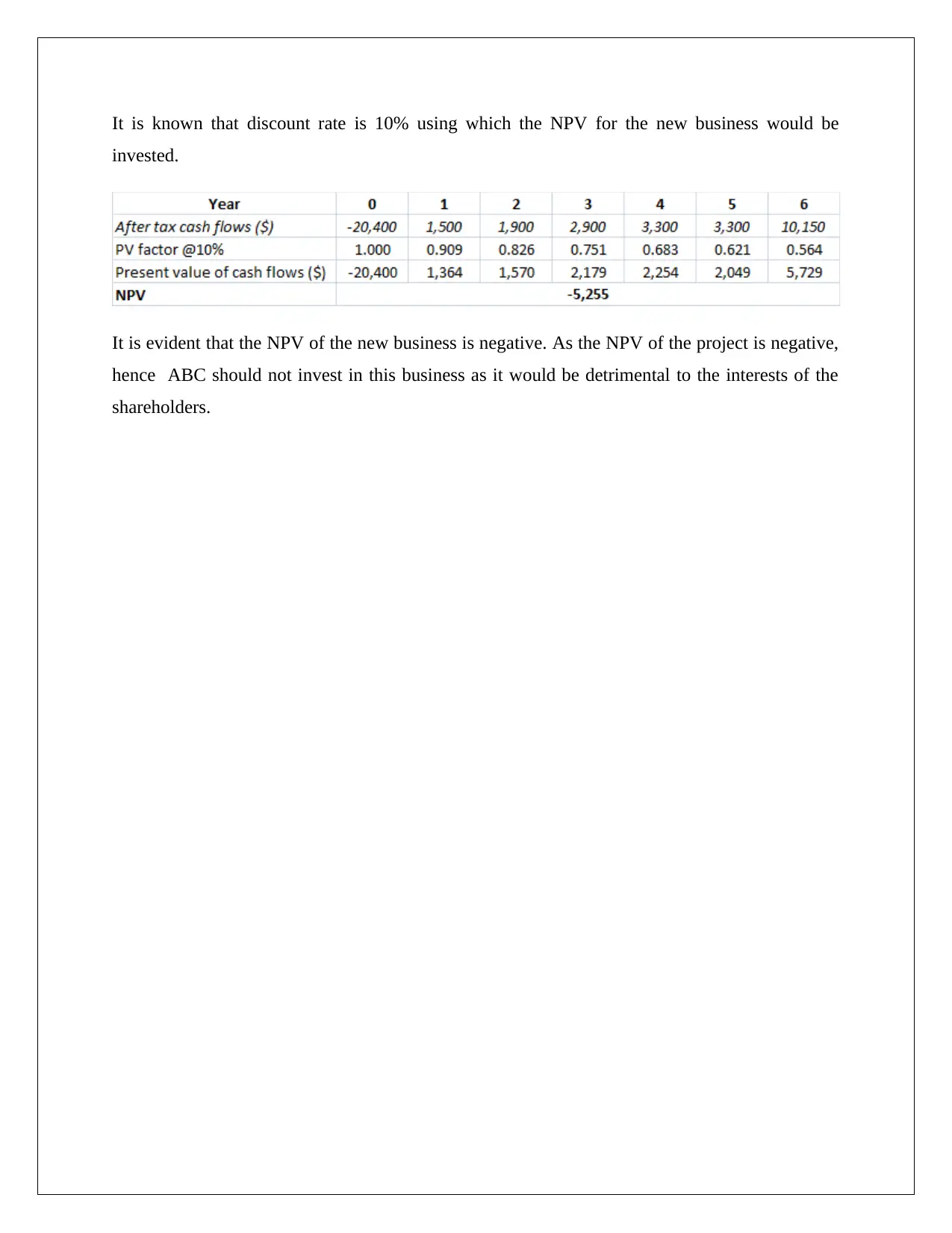
It is known that discount rate is 10% using which the NPV for the new business would be
invested.
It is evident that the NPV of the new business is negative. As the NPV of the project is negative,
hence ABC should not invest in this business as it would be detrimental to the interests of the
shareholders.