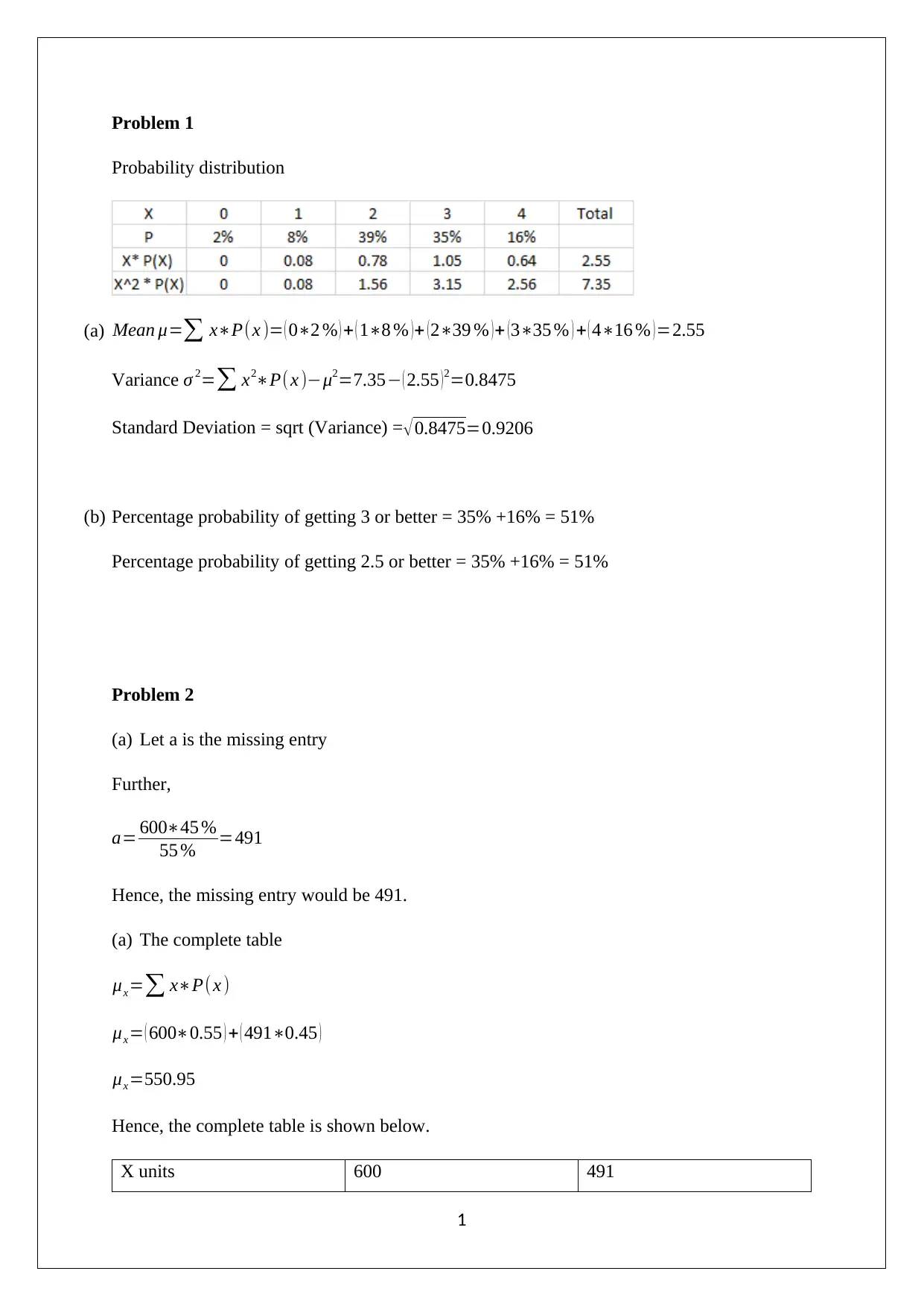
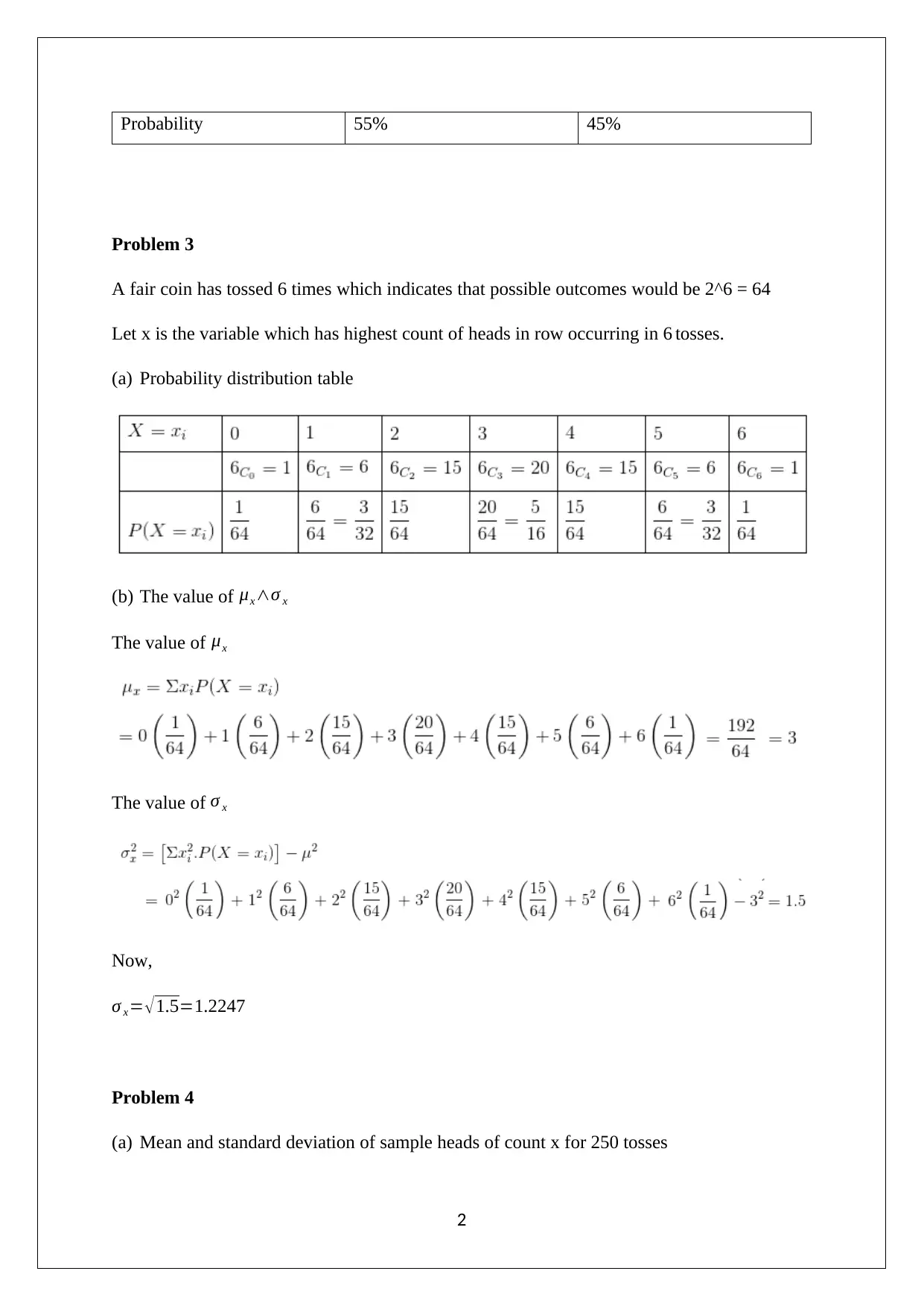
Math 243 Group Project - Probability Distribution, Mean, Standard Deviation, Binomial Distribution, Sample Proportion

This Math 243 group project covers topics such as probability distribution, mean, standard deviation, binomial distribution, and sample proportion. It includes tables and calculations for each problem.

MATH 243 GROUP PROJECT
[DATE]

Problem 1
Probability distribution
(a) Mean μ=∑ x∗P(x )= ( 0∗2 % ) + ( 1∗8 % )+ (2∗39 % )+ (3∗35 % ) + ( 4∗16 % )=2.55
Variance σ 2=∑ x2∗P( x )−μ2=7.35− ( 2.55 )2=0.8475
Standard Deviation = sqrt (Variance) =√ 0.8475=0.9206
(b) Percentage probability of getting 3 or better = 35% +16% = 51%
Percentage probability of getting 2.5 or better = 35% +16% = 51%
Problem 2
(a) Let a is the missing entry
Further,
a= 600∗45 %
55 % =491
Hence, the missing entry would be 491.
(a) The complete table
μx=∑ x∗P( x )
μx= ( 600∗0.55 ) + ( 491∗0.45 )
μx=550.95
Hence, the complete table is shown below.
X units 600 491
1

Probability 55% 45%
Problem 3
A fair coin has tossed 6 times which indicates that possible outcomes would be 2^6 = 64
Let x is the variable which has highest count of heads in row occurring in 6 tosses.
(a) Probability distribution table
(b) The value of μx∧σ x
The value of μx
The value of σ x
Now,
σ x= √ 1.5=1.2247
Problem 4
(a) Mean and standard deviation of sample heads of count x for 250 tosses
2

Binomial distribution
N = 250
P = ½ = 0.5
Mean = N*P = 0.5 *250 = 125
Standard deviation = sqrt (P*(1-P)*N) = sqrt(0.5*0.5*250) = 7.9
(b) Difference d between the head counts X and expected head counts
Expected head counts = E(H) = ½ *250 = 125
Hence, difference = 140 – 125 = 15
(c) % probability that X would fall at least d ways from expected cunts in positive direction
Positive direction = P(X>= 140)
¿ 1−P ( X <140 )=1−P (Z− ( 140−125 )
7.9 )=1−P ( Z< 1.89 )
From standard normal table P ( Z <1.89 )=0.97
P=1−0.97=0.03
(d) % probability that X would fall at least d ways from expected cunts in either direction
P=P ( X< ( 125−15 ) ) + P ( X >140 )=P (Z< 110−125
7.9 )+0.0 3
P=0.03+0.03=0.06
3

Problem 5
(a) The mean and standard deviation of the sample proportion p of heads
Mean == 0.5
Standard deviation = sqrt (0.5 * 0.5 /250) = 0.0316
(b) The Difference between head proportion and expected head counts
The proportion of head from test = 140/250 = 0.56
Expected head count = 125/250 = 0.5
Difference = 0.56 – 0.5 = 0.06
(c) % probability that head proportion would fall at least d away from expected in
positive direction
P ( 0.5−d< p< 0.5+d )=P ( p−0.5
0.0316 > d
0.0316 )=0.0 2∨2 %
(d) % probability that head proportion would fall at least d away from expected in either
direction
P ( 0.5−d< p< 0.5+d ) =P ( −d
0.0316 < p−0.5
0.0316 > d
0.0316 )=2∗0.025=0.05∨5 %
4

5