Math Task: Calculus Applications in Cost and Channel Optimization
VerifiedAdded on 2023/06/12
|9
|988
|163
Homework Assignment
AI Summary
This assignment explores the application of calculus in solving practical optimization problems. It consists of two main questions: The first question focuses on minimizing the cost of laying a cable both underwater and on land, using derivatives to find the optimal distance for the cable to transition fro...

Math Task 1
Math Task
Student’s Name
Course
Professor’s Name
University
City (State)
Date
Math Task
Student’s Name
Course
Professor’s Name
University
City (State)
Date
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Math Task 2
Math Task
Question 1
Part a
To calculate the cost of laying the cable, we have to calculate the distances AE and AI.
AE=(42−x )
Then from Pythagoras Theorem, AI2=252+ x2=625+ x2
The length of the cable under water ¿ AI= √ 625+ x2 km
Hence, the cost of the cable under water ¿ $ 3600 √ 625+x2
The length of the cable on land¿ AE= ( 42−x ) km
Hence, the cost of the cable on land ¿ $ 2700 ( 42−x )
Total cost of cable=Cost of cable under water +Cost of cable on land
C (x)=$ ( 3600 √625+ x2 +2700 ( 42−x ) )
Part b
To get the minimum cost of laying the cable, dC ( x )
dx =0
dC ( x )
dx = d
dx $ ( 3600 √ 625+ x2 +2700 ( 42−x ) )
¿ 3600 d
dx ( √625+ x2 ) +2700 d
dx ( 42−x )
Let 625+ x2=u so that d u
dx =2 x
Math Task
Question 1
Part a
To calculate the cost of laying the cable, we have to calculate the distances AE and AI.
AE=(42−x )
Then from Pythagoras Theorem, AI2=252+ x2=625+ x2
The length of the cable under water ¿ AI= √ 625+ x2 km
Hence, the cost of the cable under water ¿ $ 3600 √ 625+x2
The length of the cable on land¿ AE= ( 42−x ) km
Hence, the cost of the cable on land ¿ $ 2700 ( 42−x )
Total cost of cable=Cost of cable under water +Cost of cable on land
C (x)=$ ( 3600 √625+ x2 +2700 ( 42−x ) )
Part b
To get the minimum cost of laying the cable, dC ( x )
dx =0
dC ( x )
dx = d
dx $ ( 3600 √ 625+ x2 +2700 ( 42−x ) )
¿ 3600 d
dx ( √625+ x2 ) +2700 d
dx ( 42−x )
Let 625+ x2=u so that d u
dx =2 x

Math Task 3
√625+ x2= √u=u
1
2
dC ( x )
du =1
2 u
−1
2 = 1
2 √625+ x2
3600 dC ( x )
dx =3600 dC ( x )
du × du
dx =2 x 3600
2 √625+x2 = 3600 x
√ 625+ x2
2700 d
dx ( 42−x ) =2700 (−1 )=−2700
dC ( x )
dx = 3600 x
√ 625+ x2 −2700=0
3600 x
√625+ x2 =2700
(3600 x)2=27002(625+ x2)
625+ x2= ( 3600 ) 2
27002 x2= 16
9 x2
16
9 x2−x2=625
7
9 x2=625
x= √625 × 9
7 = √ 5625
7 = 75
√ 7 =28.3473 m
The cost of laying the cable is minimum when x=28.3473 m
Minimum cost¿ C (28.3473)=$ ( 3600 √625+28.34732+ 2700 ( 42−28.3473 ) )
Minimum cable cost=$ 172,929.4045
√625+ x2= √u=u
1
2
dC ( x )
du =1
2 u
−1
2 = 1
2 √625+ x2
3600 dC ( x )
dx =3600 dC ( x )
du × du
dx =2 x 3600
2 √625+x2 = 3600 x
√ 625+ x2
2700 d
dx ( 42−x ) =2700 (−1 )=−2700
dC ( x )
dx = 3600 x
√ 625+ x2 −2700=0
3600 x
√625+ x2 =2700
(3600 x)2=27002(625+ x2)
625+ x2= ( 3600 ) 2
27002 x2= 16
9 x2
16
9 x2−x2=625
7
9 x2=625
x= √625 × 9
7 = √ 5625
7 = 75
√ 7 =28.3473 m
The cost of laying the cable is minimum when x=28.3473 m
Minimum cost¿ C (28.3473)=$ ( 3600 √625+28.34732+ 2700 ( 42−28.3473 ) )
Minimum cable cost=$ 172,929.4045
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
Math Task 4
Question 2
Part a
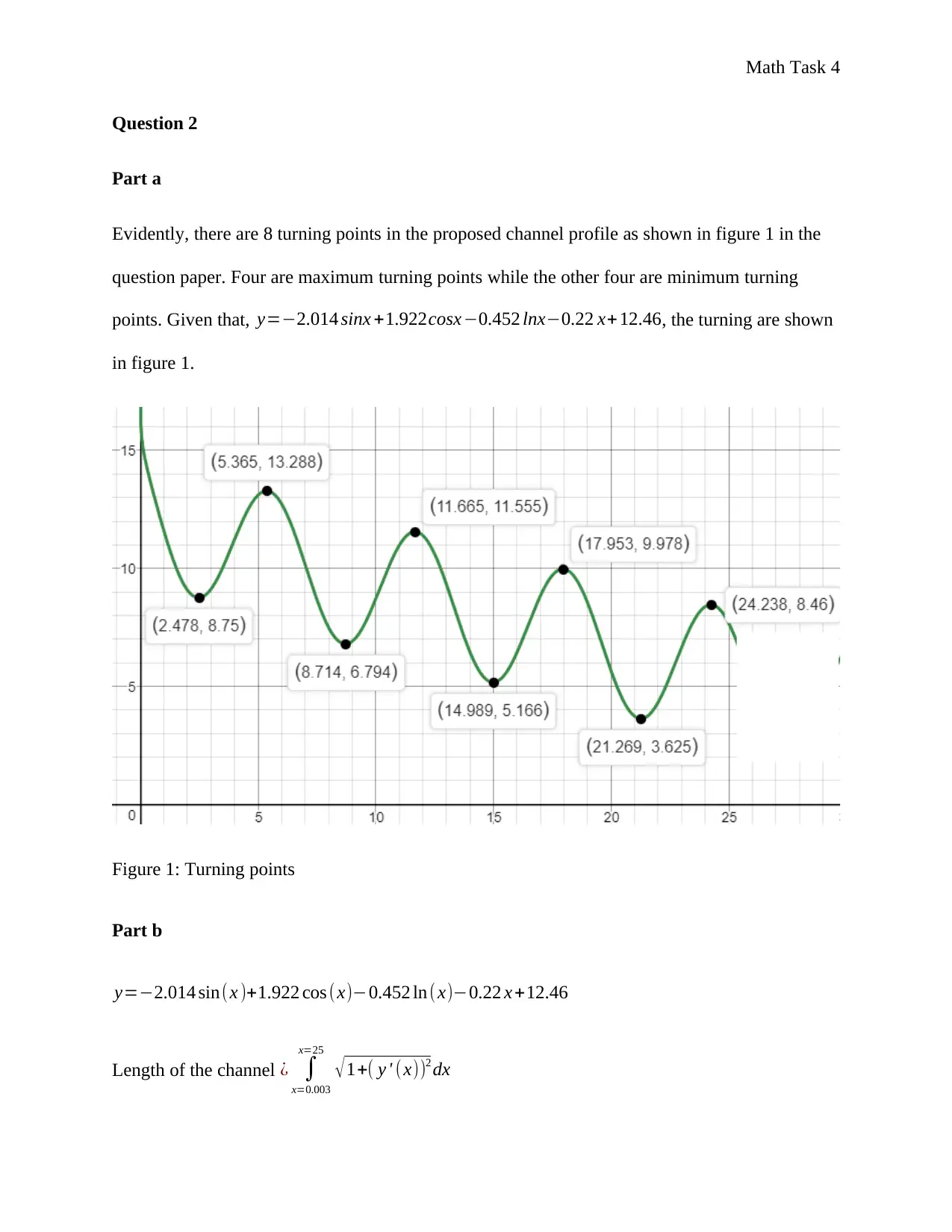
Evidently, there are 8 turning points in the proposed channel profile as shown in figure 1 in the
question paper. Four are maximum turning points while the other four are minimum turning
points. Given that, y=−2.014 sinx +1.922cosx−0.452 lnx−0.22 x+ 12.46, the turning are shown
in figure 1.
Figure 1: Turning points
Part b
y=−2.014 sin(x )+1.922 cos (x)−0.452 ln( x)−0.22 x +12.46
Length of the channel ¿ ∫
x=0.003
x=25
√ 1+( y ' (x))2 dx
Question 2
Part a
Evidently, there are 8 turning points in the proposed channel profile as shown in figure 1 in the
question paper. Four are maximum turning points while the other four are minimum turning
points. Given that, y=−2.014 sinx +1.922cosx−0.452 lnx−0.22 x+ 12.46, the turning are shown
in figure 1.
Figure 1: Turning points
Part b
y=−2.014 sin(x )+1.922 cos (x)−0.452 ln( x)−0.22 x +12.46
Length of the channel ¿ ∫
x=0.003
x=25
√ 1+( y ' (x))2 dx
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Math Task 5
y' ( x ) = dy
dx = d
dx ( −2.014 sin ( x ) +1.922 cos ( x ) −0.452 ln ( x ) −0.22 x+12.46 )
¿−2.014 cos ( x )−1.922 sin(x )− 0.452
x −0.22
¿ y' ( x ) ∨¿2.014 cos ( x ) + 1.922sin ( x ) + 0.452
x +0.22
Channel length= ∫
x=0.003
x=25
√1+(2.014 cos ( x ) +1.922 sin ( x ) + 0.452
x +0.22)
2
dx
Solving the equation above we obtain, Channel length=55.41631km
Part c
Maximum channel width ¿ 6 m
Minimum cross section area ¿ 8 m2
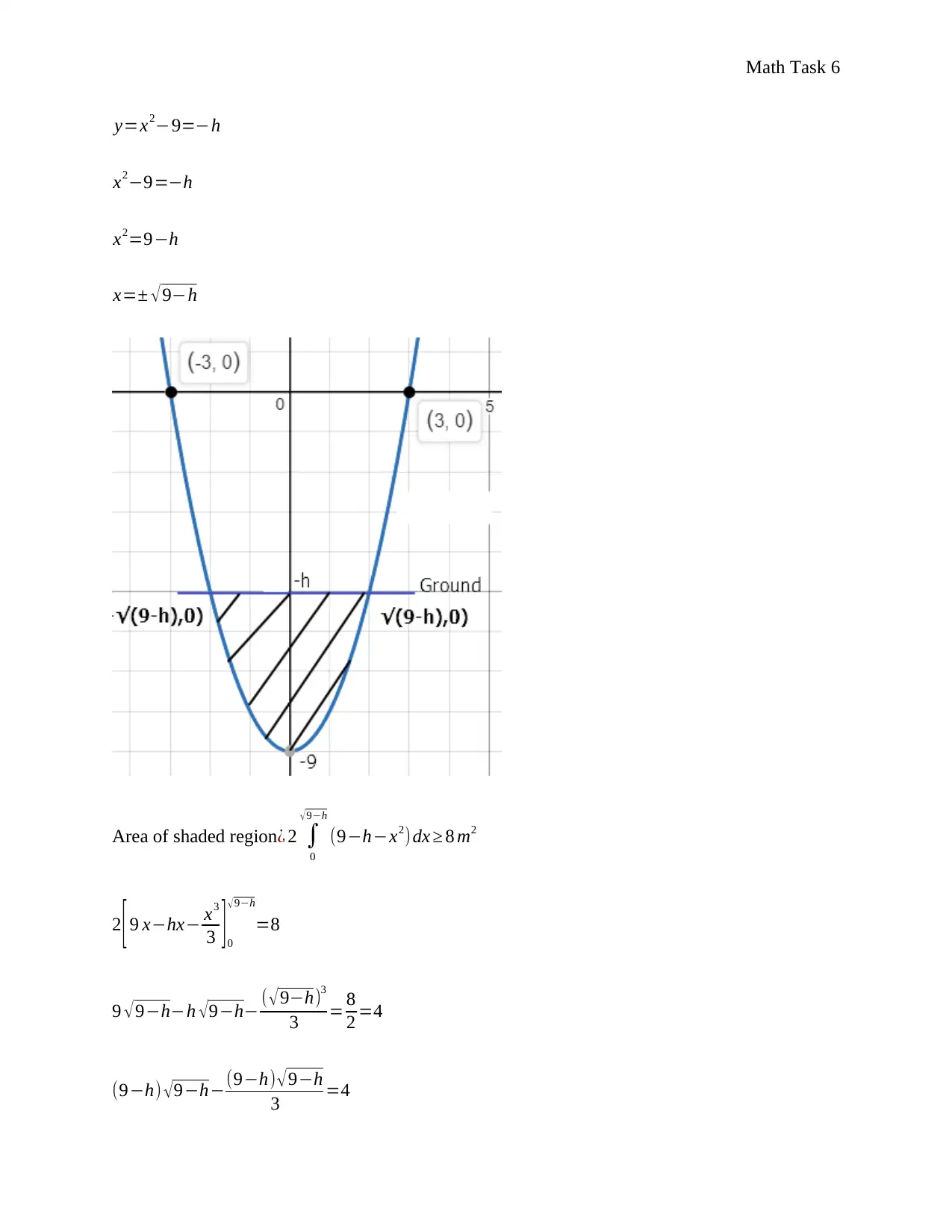
Depth of channel=h
The x-intercepts are x=−3∧, x=3
Which implies that x +3=0∧, x−3=0
Then, ( x +3 ) ( x−3 )=0= y
Expanding the equation, we get,
y=x2−9
The equation of the shaded region is −h− ( x2−9 ) =9−h−x2
The curve y=x2−9 and the line y=−h meet at:
y' ( x ) = dy
dx = d
dx ( −2.014 sin ( x ) +1.922 cos ( x ) −0.452 ln ( x ) −0.22 x+12.46 )
¿−2.014 cos ( x )−1.922 sin(x )− 0.452
x −0.22
¿ y' ( x ) ∨¿2.014 cos ( x ) + 1.922sin ( x ) + 0.452
x +0.22
Channel length= ∫
x=0.003
x=25
√1+(2.014 cos ( x ) +1.922 sin ( x ) + 0.452
x +0.22)
2
dx
Solving the equation above we obtain, Channel length=55.41631km
Part c
Maximum channel width ¿ 6 m
Minimum cross section area ¿ 8 m2
Depth of channel=h
The x-intercepts are x=−3∧, x=3
Which implies that x +3=0∧, x−3=0
Then, ( x +3 ) ( x−3 )=0= y
Expanding the equation, we get,
y=x2−9
The equation of the shaded region is −h− ( x2−9 ) =9−h−x2
The curve y=x2−9 and the line y=−h meet at:
Math Task 6
y=x2−9=−h
x2−9=−h
x2=9−h
x=± √ 9−h
Area of shaded region ¿ 2 ∫
0
√ 9−h
(9−h−x2)dx ≥ 8 m2
2 [9 x−hx− x3
3 ]0
√9−h
=8
9 √ 9−h−h √ 9−h− ( √ 9−h)3
3 = 8
2 =4
(9−h) √ 9−h−(9−h) √ 9−h
3 =4
y=x2−9=−h
x2−9=−h
x2=9−h
x=± √ 9−h
Area of shaded region ¿ 2 ∫
0
√ 9−h
(9−h−x2)dx ≥ 8 m2
2 [9 x−hx− x3
3 ]0
√9−h
=8
9 √ 9−h−h √ 9−h− ( √ 9−h)3
3 = 8
2 =4
(9−h) √ 9−h−(9−h) √ 9−h
3 =4
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide

Math Task 7
(9−h) √9−h−(9−h) √9−h
3 =4
2
3 ( 9−h ) √ 9−h=4
( 9−h ) √9−h=4 × 3
2 =6
√ ( 9−h)((9−h)2 )=6
√( 9−h)3=(9−h)
3
2 =6
9−h=(6)
2
3
h=9− ( 6 )
2
3 =5.698 m
The depth of the channel below the ground level is ( 9−5.698 ) m=3.302 m
y=x2 +h−9=x2 +5.698−9=x2−3.302
The channel profile equation is y=x2−3.302
Part d
Channel height¿ 9 m
Safe flow capacity¿ 9 m× 0.75=6.75 m
(9−h) √9−h−(9−h) √9−h
3 =4
2
3 ( 9−h ) √ 9−h=4
( 9−h ) √9−h=4 × 3
2 =6
√ ( 9−h)((9−h)2 )=6
√( 9−h)3=(9−h)
3
2 =6
9−h=(6)
2
3
h=9− ( 6 )
2
3 =5.698 m
The depth of the channel below the ground level is ( 9−5.698 ) m=3.302 m
y=x2 +h−9=x2 +5.698−9=x2−3.302
The channel profile equation is y=x2−3.302
Part d
Channel height¿ 9 m
Safe flow capacity¿ 9 m× 0.75=6.75 m
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
Math Task 8
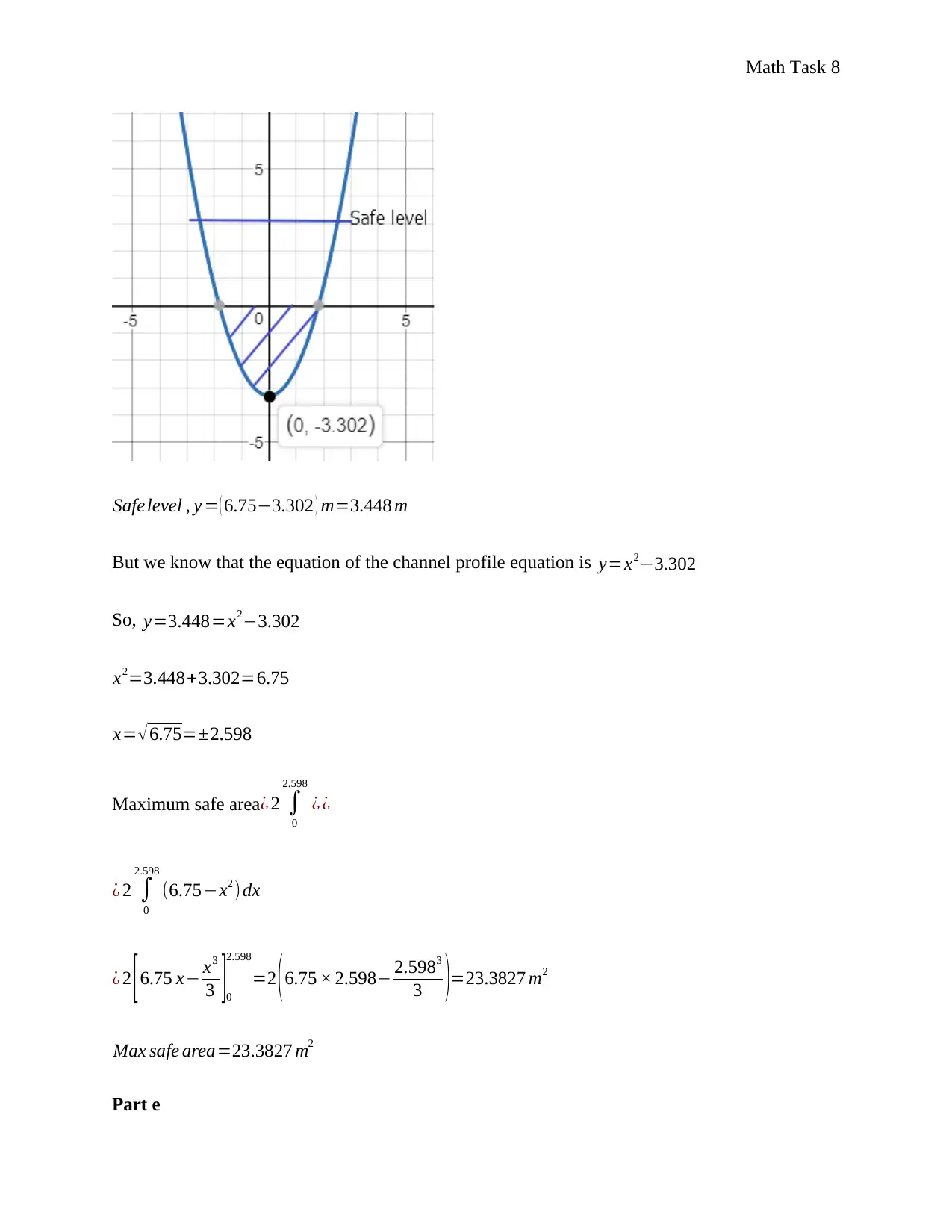
Safelevel , y = ( 6.75−3.302 ) m=3.448 m
But we know that the equation of the channel profile equation is y=x2−3.302
So, y=3.448=x2−3.302
x2=3.448+3.302=6.75
x= √6.75=±2.598
Maximum safe area ¿ 2 ∫
0
2.598
¿ ¿
¿ 2 ∫
0
2.598
(6.75−x2 ) dx
¿ 2 [ 6.75 x− x3
3 ]0
2.598
=2 ( 6.75 × 2.598− 2.5983
3 )=23.3827 m2
Max safe area=23.3827 m2
Part e
Safelevel , y = ( 6.75−3.302 ) m=3.448 m
But we know that the equation of the channel profile equation is y=x2−3.302
So, y=3.448=x2−3.302
x2=3.448+3.302=6.75
x= √6.75=±2.598
Maximum safe area ¿ 2 ∫
0
2.598
¿ ¿
¿ 2 ∫
0
2.598
(6.75−x2 ) dx
¿ 2 [ 6.75 x− x3
3 ]0
2.598
=2 ( 6.75 × 2.598− 2.5983
3 )=23.3827 m2
Max safe area=23.3827 m2
Part e

Math Task 9
Total area where the lining material will be used is the area of the channel up to ground level.
That is, ( 23.3827−8 ) m2=15.3827 m2
Cost of lining material Total area×Cost per unit area
Cost =15.3827 m2 × $ 30/m2
Cost =$ 461.481
Total area where the lining material will be used is the area of the channel up to ground level.
That is, ( 23.3827−8 ) m2=15.3827 m2
Cost of lining material Total area×Cost per unit area
Cost =15.3827 m2 × $ 30/m2
Cost =$ 461.481
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
1 out of 9
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.

