Mathematics Assignment - Desklib
VerifiedAdded on 2022/10/04
|12
|761
|335
AI Summary
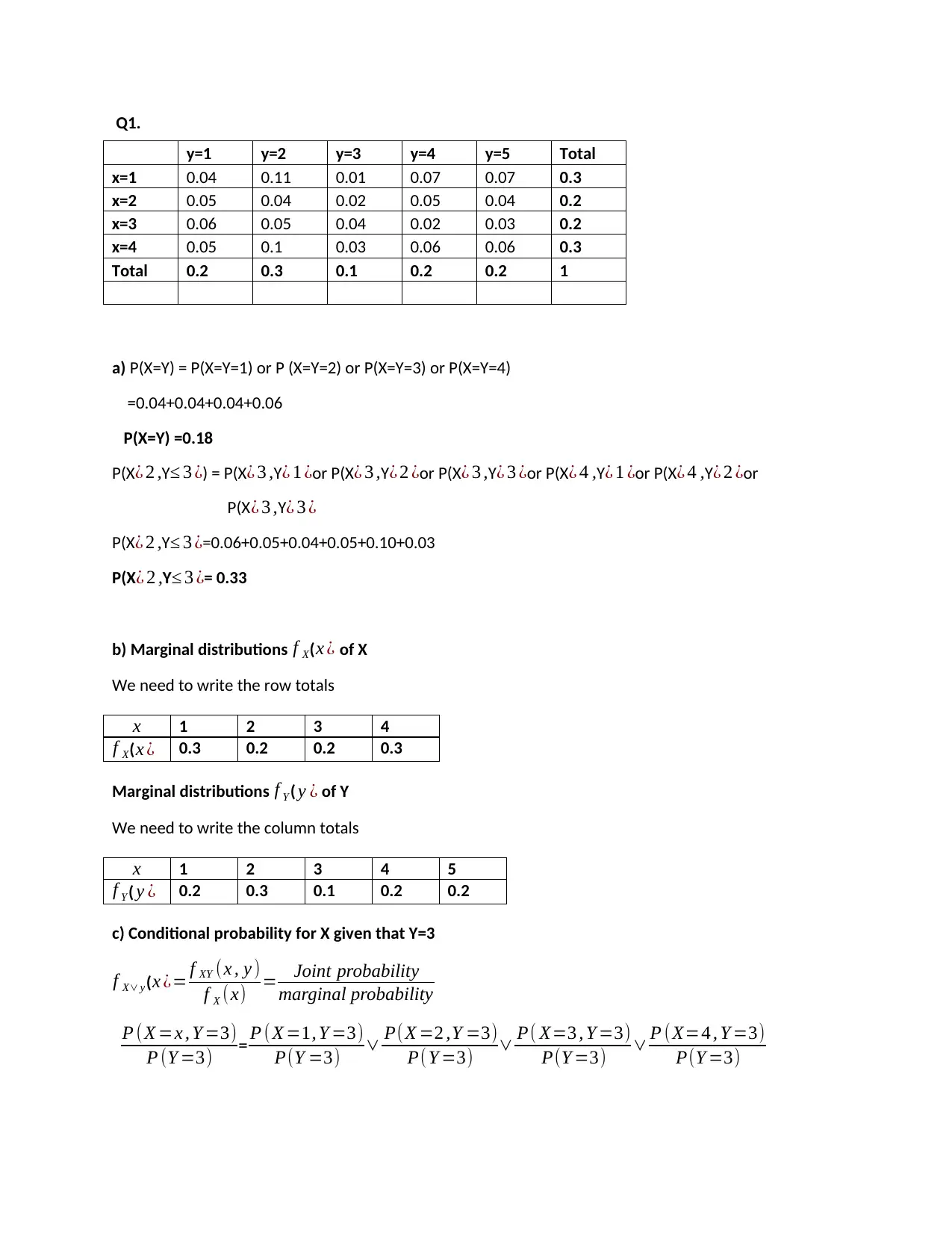
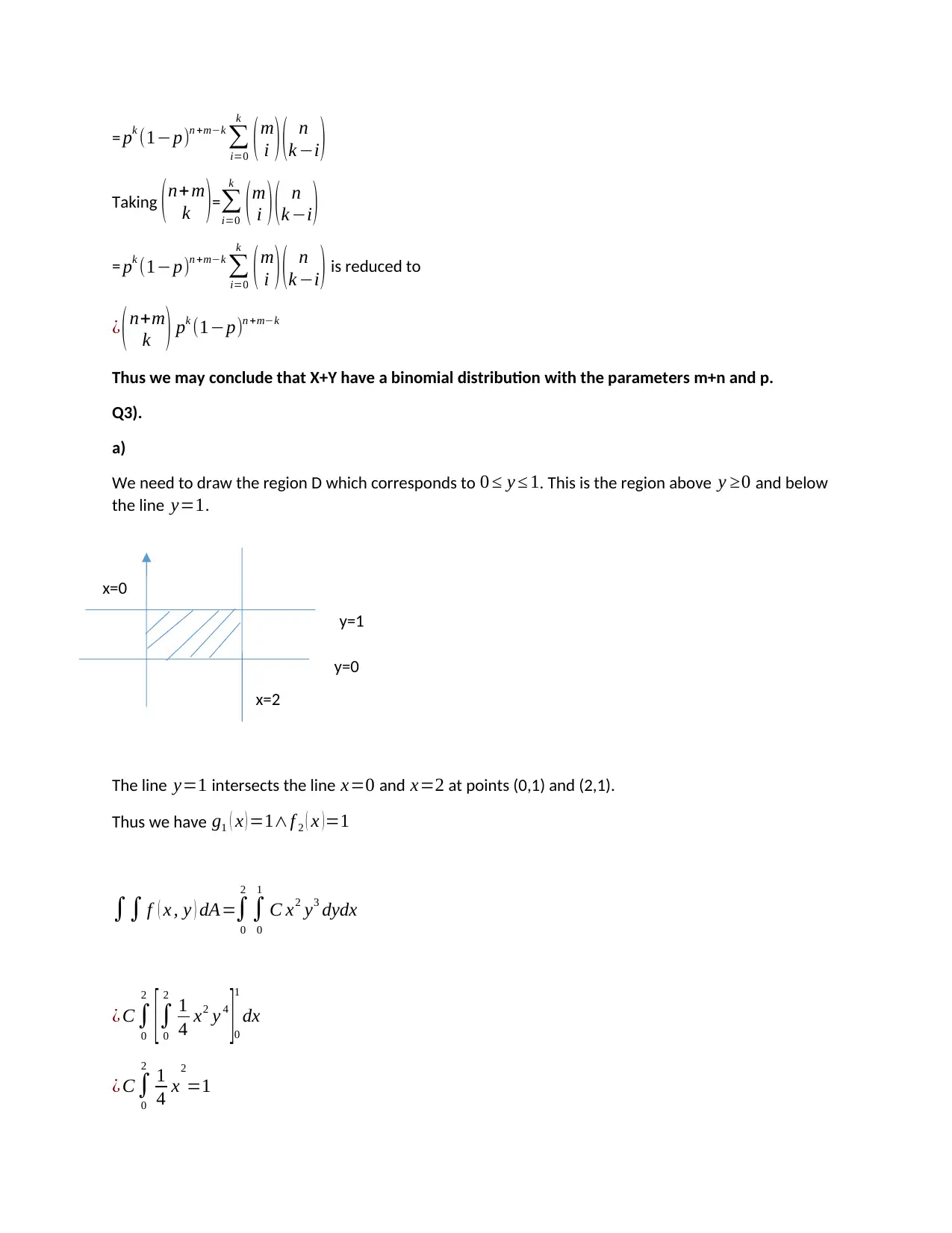
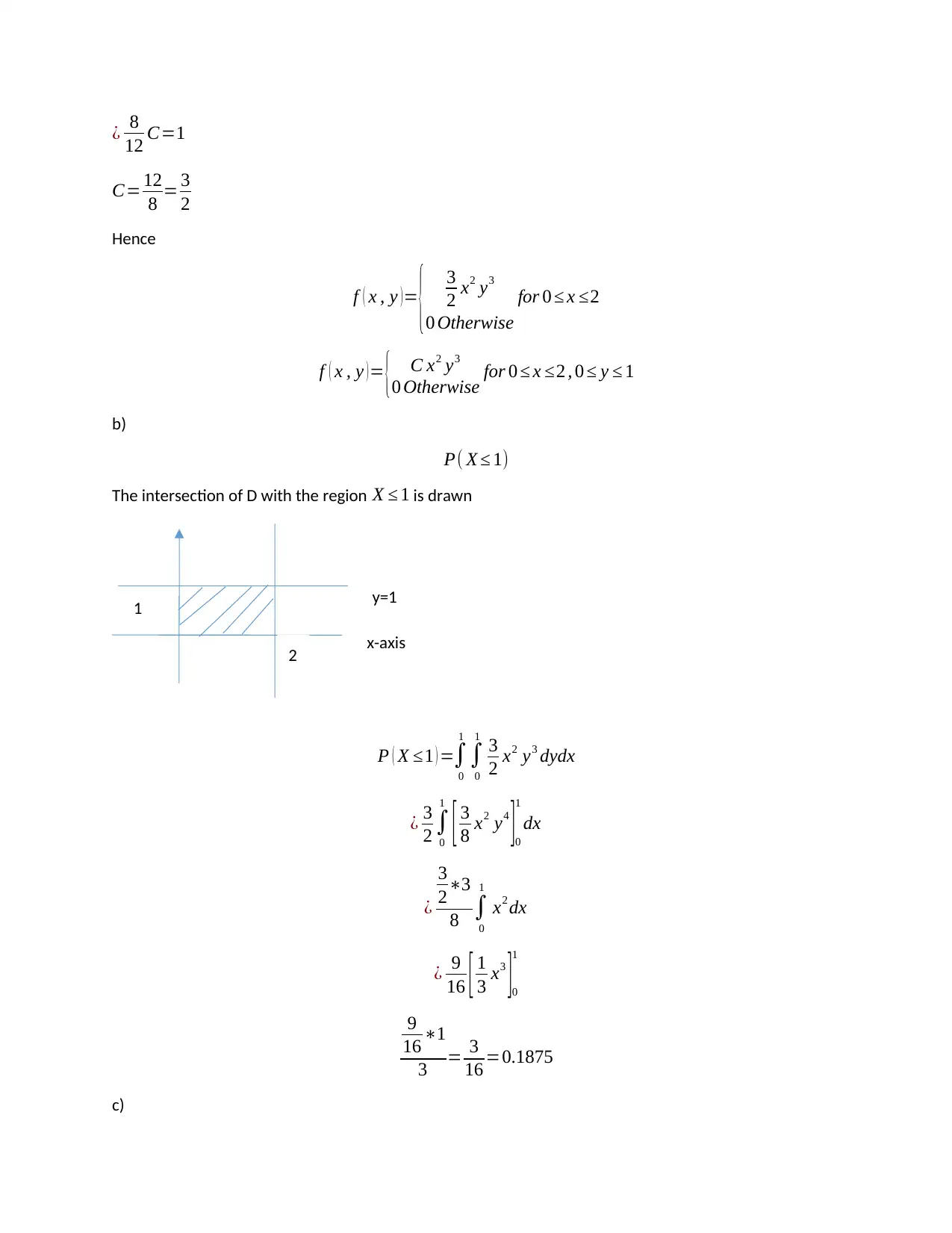
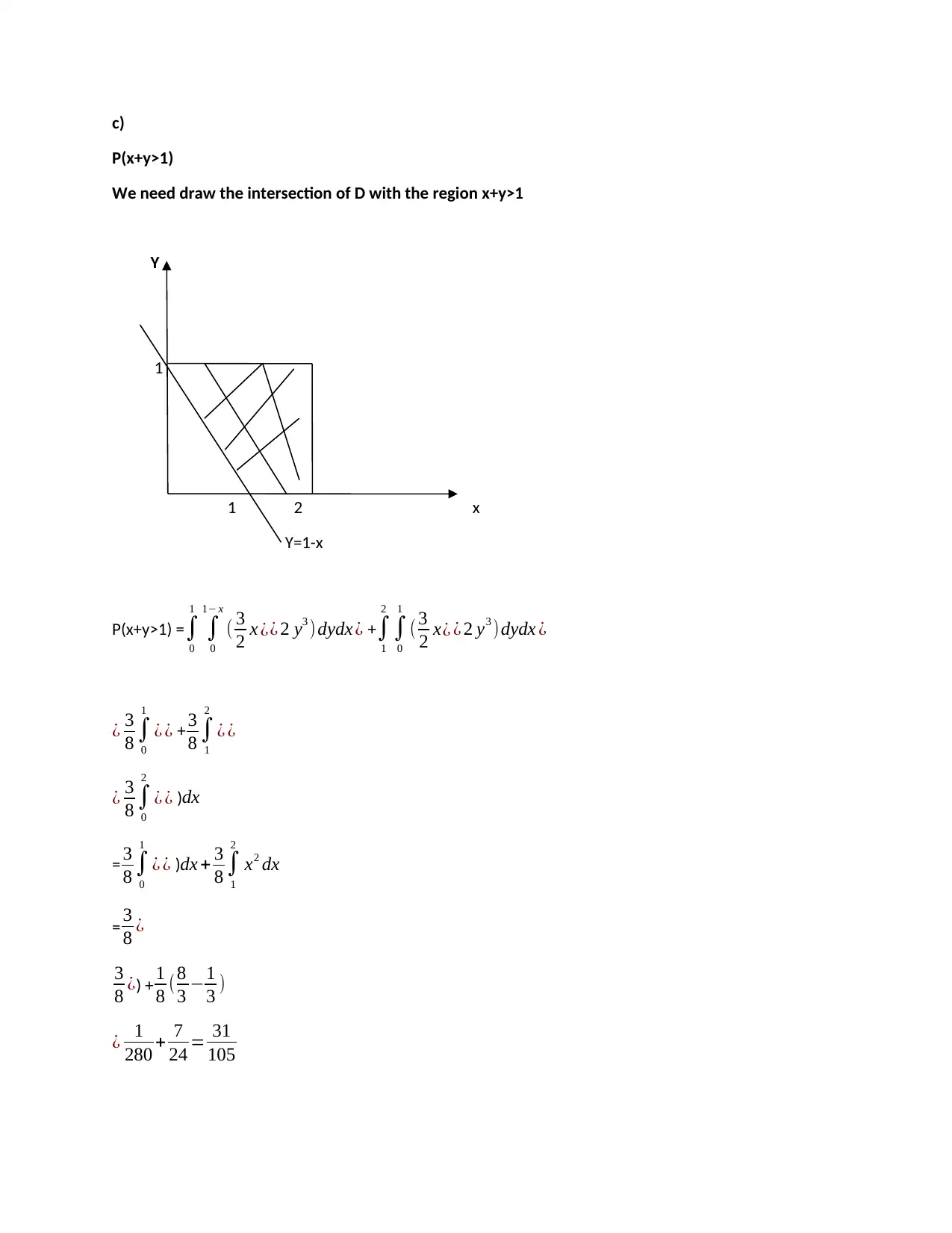
This Mathematics Assignment covers topics like conditional probability, independence, binomial distribution, joint and marginal pdf of continuous random variables. It also includes solved examples and graphs. The document type is an assignment and the subject is Mathematics. The course code, course name, and college/university are not mentioned.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.
1 out of 12


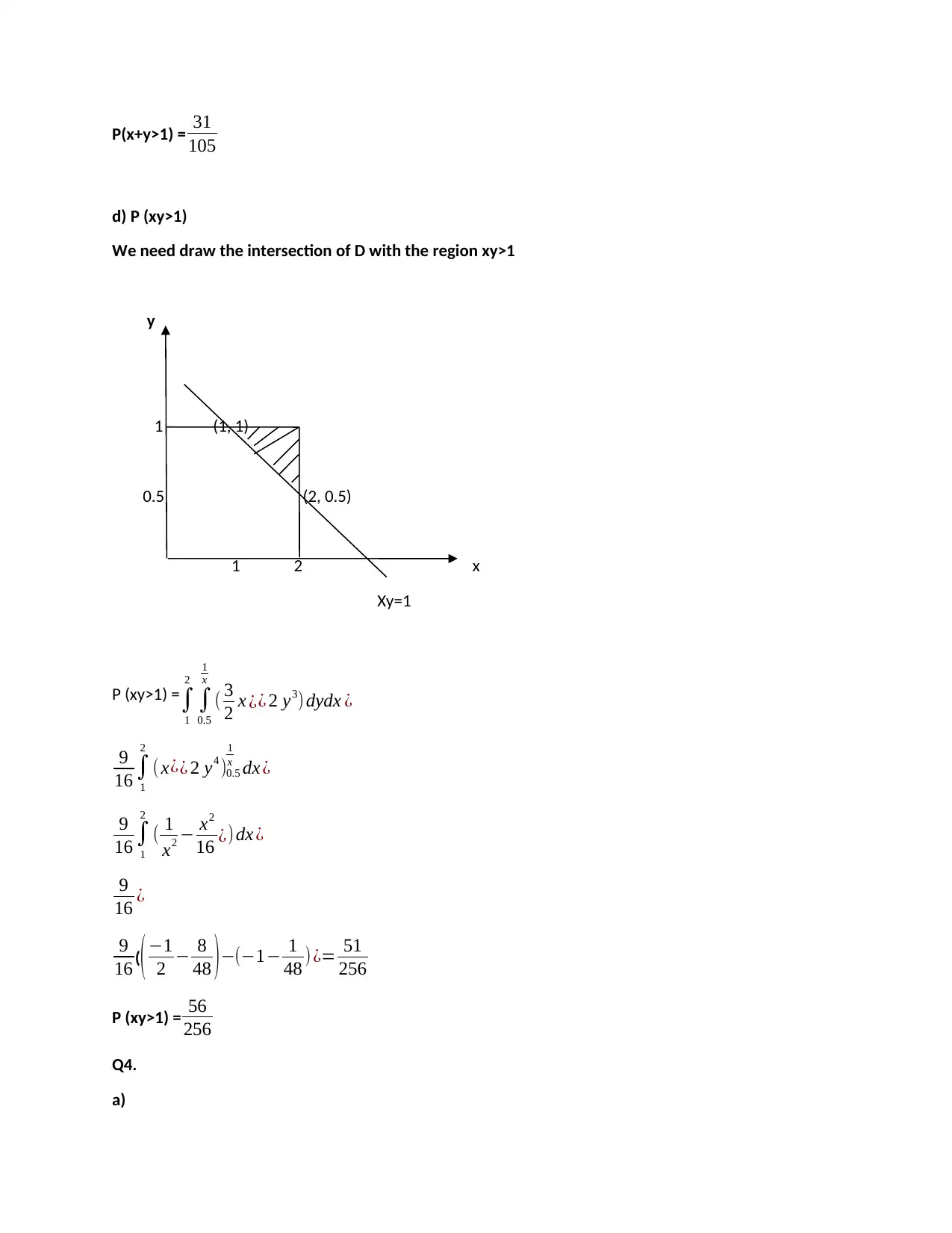











![[object Object]](/_next/static/media/star-bottom.7253800d.svg)