Mathematics Assignment Solution - Foundations of Mathematics, 2019
VerifiedAdded on 2022/11/14
|9
|746
|119
Homework Assignment
AI Summary
This document provides a comprehensive solution to a mathematics assignment. The assignment covers various topics, including finding unique solutions to equations, working with parametric and Cartesian equations, and determining normal vectors. It explores the concept of vector equations and the intersection of lines in coordinate planes. The solution also involves analyzing linear equations, identifying parallel vectors, and performing error analysis. Detailed step-by-step explanations are provided for each problem, making it a valuable resource for students studying foundations of mathematics. This assignment offers a complete understanding of the topics covered.

Mathematics Assignment
Student Name:
Instructor Name:
Course Number:
17th July 2019
Student Name:
Instructor Name:
Course Number:
17th July 2019
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

1. Show that e−8 x+5 cos ( 20 x ) =0 has a unique solution from x=0 ¿ x= π
20
An equation is said to have a unique solution if it has only one solution at a particular point.
At x=0 ,
e−8 x+5 cos (20 x )=e−8(0)+5 cos ¿ ¿
¿ e0 +5 cos 0=1+5=6
At x= π
20 ,
e−8 ( π
20 )
+5 cos ( 20 ) π
20 =e−0.4 π +5 cos ( π ) =0.2846-5= -0.4.7154
Therefore the above equation has a unique s0lution within the given closed interval.
2 a ¿ π 1 : (x1
x2
x3
)=
( 3
1
−1 )+ t
( 1
2
−2 )+ p ( 3
1
−3 )
Parametric equations are
x1=3+t +3 p------------------------------i
x2=1+2 t+ p………………………………..ii
x3=−1−2t−3 p -------------------------iii
From i and ii we have;
p=0.4 x1−0.2 x2−1 And t = x2 −x1
3
Substituting p and t in iii
x3=−1−2t−3 p
x3=−1−2( x2−x1
3 )−3 ¿ )
x3=−1−2
3 x2− 2
3 x1−1.2 x1−0.6 x2−3
x3=−28
15 x1− 19
15 x2−4
−28
15 x1− 19
15 x2−x3−4=0
20
An equation is said to have a unique solution if it has only one solution at a particular point.
At x=0 ,
e−8 x+5 cos (20 x )=e−8(0)+5 cos ¿ ¿
¿ e0 +5 cos 0=1+5=6
At x= π
20 ,
e−8 ( π
20 )
+5 cos ( 20 ) π
20 =e−0.4 π +5 cos ( π ) =0.2846-5= -0.4.7154
Therefore the above equation has a unique s0lution within the given closed interval.
2 a ¿ π 1 : (x1
x2
x3
)=
( 3
1
−1 )+ t
( 1
2
−2 )+ p ( 3
1
−3 )
Parametric equations are
x1=3+t +3 p------------------------------i
x2=1+2 t+ p………………………………..ii
x3=−1−2t−3 p -------------------------iii
From i and ii we have;
p=0.4 x1−0.2 x2−1 And t = x2 −x1
3
Substituting p and t in iii
x3=−1−2t−3 p
x3=−1−2( x2−x1
3 )−3 ¿ )
x3=−1−2
3 x2− 2
3 x1−1.2 x1−0.6 x2−3
x3=−28
15 x1− 19
15 x2−4
−28
15 x1− 19
15 x2−x3−4=0

Normal vector to π1 is (−28
15 ,−19
15 ,−1 ¿
n1=¿ ¿(−28
15 ,−19
15 ,−1 ¿
π2 : (x1
x2
x3
)=
( 3
−3
3 )+ μ1
( 1
−2
2 )+ μ2
( 3
−3
1 )
Parametric equations are
x1=3+ μ1 +3 μ2------------------------------i
x2=−3−2 μ1−3 μ2………………………………..ii
x3=3+2 μ1+ μ2----------------------iii
From i and ii we have;
μ1=−x1−x2 and μ2= 2 x1 + x2+3
3
Substituting μ1and μ2in iii
x3=3+2 μ1+ μ2
x3=3+2 ( −x1−x2 ) +( 2 x1 + x2 +3
3 )
−4
3 x1− 5
3 x2−x3+ 4=0
−4
3 x1− 5
3 x2−x3+ 4=0
Normal vector to π2 is (−4
3 ,−5
3 ,−1 ¿
n2=¿ ¿ (−4
3 ,−5
3 ,−1 ¿
b) The Cartesian equation for π1can be found by eliminating the parameters s and t from the
parametric equations.
π1 : ( x1
x2
x3
)=
( 3
1
−1 )+ t
( 1
2
−2 )+ p ( 3
1
−3 )
Parametric equations are
15 ,−19
15 ,−1 ¿
n1=¿ ¿(−28
15 ,−19
15 ,−1 ¿
π2 : (x1
x2
x3
)=
( 3
−3
3 )+ μ1
( 1
−2
2 )+ μ2
( 3
−3
1 )
Parametric equations are
x1=3+ μ1 +3 μ2------------------------------i
x2=−3−2 μ1−3 μ2………………………………..ii
x3=3+2 μ1+ μ2----------------------iii
From i and ii we have;
μ1=−x1−x2 and μ2= 2 x1 + x2+3
3
Substituting μ1and μ2in iii
x3=3+2 μ1+ μ2
x3=3+2 ( −x1−x2 ) +( 2 x1 + x2 +3
3 )
−4
3 x1− 5
3 x2−x3+ 4=0
−4
3 x1− 5
3 x2−x3+ 4=0
Normal vector to π2 is (−4
3 ,−5
3 ,−1 ¿
n2=¿ ¿ (−4
3 ,−5
3 ,−1 ¿
b) The Cartesian equation for π1can be found by eliminating the parameters s and t from the
parametric equations.
π1 : ( x1
x2
x3
)=
( 3
1
−1 )+ t
( 1
2
−2 )+ p ( 3
1
−3 )
Parametric equations are
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
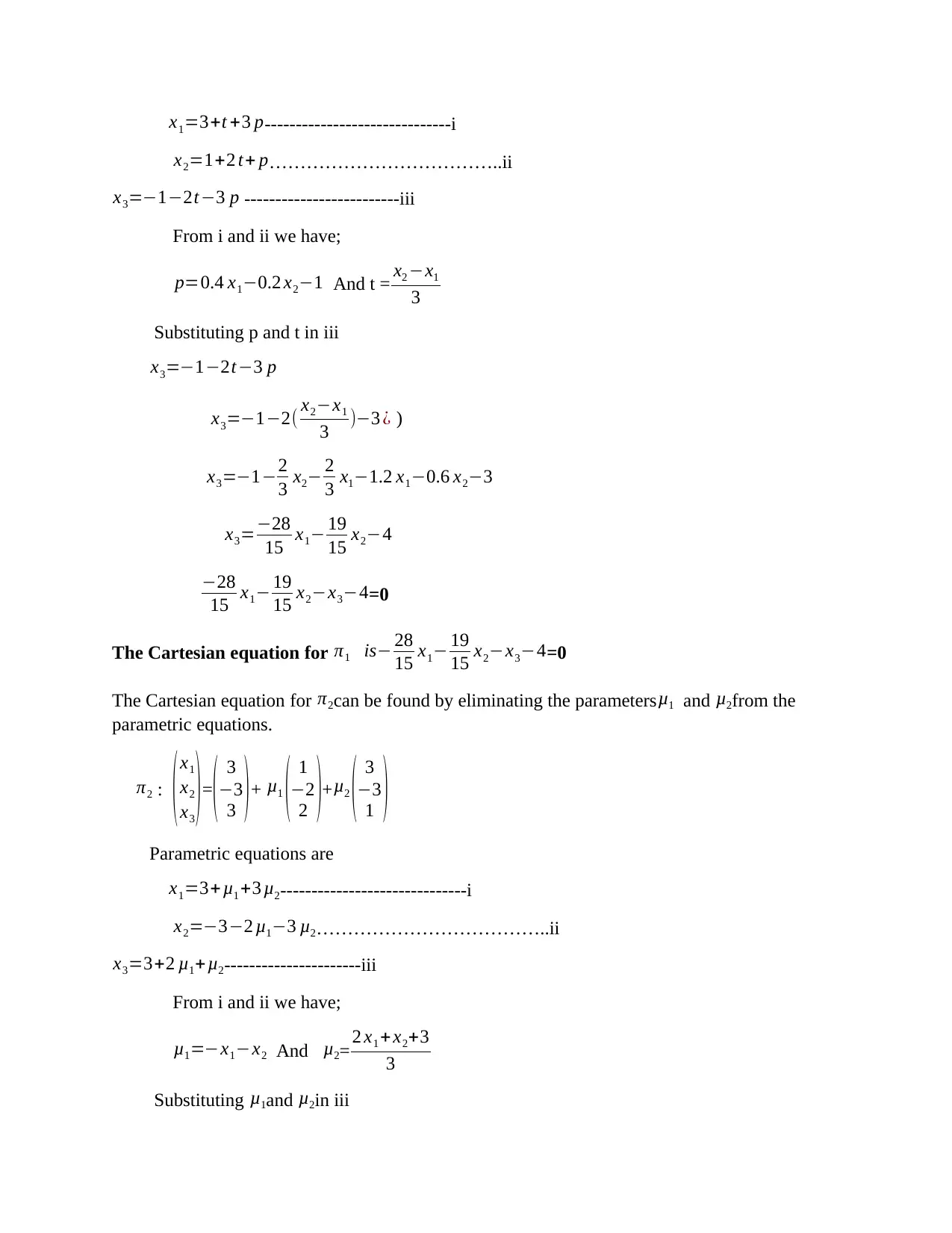
x1=3+t +3 p------------------------------i
x2=1+2 t+ p………………………………..ii
x3=−1−2t−3 p -------------------------iii
From i and ii we have;
p=0.4 x1−0.2 x2−1 And t = x2 −x1
3
Substituting p and t in iii
x3=−1−2t−3 p
x3=−1−2( x2−x1
3 )−3 ¿ )
x3=−1−2
3 x2− 2
3 x1−1.2 x1−0.6 x2−3
x3=−28
15 x1− 19
15 x2−4
−28
15 x1− 19
15 x2−x3−4=0
The Cartesian equation for π1 is− 28
15 x1− 19
15 x2−x3−4=0
The Cartesian equation for π2can be found by eliminating the parametersμ1 and μ2from the
parametric equations.
π2 : ( x1
x2
x3
)=
( 3
−3
3 )+ μ1
( 1
−2
2 )+ μ2
( 3
−3
1 )
Parametric equations are
x1=3+ μ1 +3 μ2------------------------------i
x2=−3−2 μ1−3 μ2………………………………..ii
x3=3+2 μ1+ μ2----------------------iii
From i and ii we have;
μ1=−x1−x2 And μ2= 2 x1 + x2+3
3
Substituting μ1and μ2in iii
x2=1+2 t+ p………………………………..ii
x3=−1−2t−3 p -------------------------iii
From i and ii we have;
p=0.4 x1−0.2 x2−1 And t = x2 −x1
3
Substituting p and t in iii
x3=−1−2t−3 p
x3=−1−2( x2−x1
3 )−3 ¿ )
x3=−1−2
3 x2− 2
3 x1−1.2 x1−0.6 x2−3
x3=−28
15 x1− 19
15 x2−4
−28
15 x1− 19
15 x2−x3−4=0
The Cartesian equation for π1 is− 28
15 x1− 19
15 x2−x3−4=0
The Cartesian equation for π2can be found by eliminating the parametersμ1 and μ2from the
parametric equations.
π2 : ( x1
x2
x3
)=
( 3
−3
3 )+ μ1
( 1
−2
2 )+ μ2
( 3
−3
1 )
Parametric equations are
x1=3+ μ1 +3 μ2------------------------------i
x2=−3−2 μ1−3 μ2………………………………..ii
x3=3+2 μ1+ μ2----------------------iii
From i and ii we have;
μ1=−x1−x2 And μ2= 2 x1 + x2+3
3
Substituting μ1and μ2in iii
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

x3=3+2 μ1+ μ2
x3=3+2 ( −x1−x2 ) +( 2 x1 + x2 +3
3 )
−4
3 x1− 5
3 x2−x3+ 4=0
−4
3 x1− 5
3 x2−x3+ 4=0
The Cartesian equation for π2 is −4
3 x1− 5
3 x2−x3+4=0
c) Let x1=w
π1 : ( w
x2
x3 )=
( 3
1
−1 )+ t
( 1
2
−2 )+ p ( 3
1
−3 )
Let x1=w
Parametric equations are
w=3+t +3 p------------------------------i
x2=1+2 t+ p………………………………..ii
x3=−1−2t−3 p -------------------------iii
From i and ii we have;
p=0.4 w−0.2 x2 −1 And t = x2 −w
3
Substituting p and t in iii
x3=−1−2t−3 p
x3=−1−2( x2−w
3 )−3 ¿ )
x3=−1−2
3 x2− 2
3 w−1.2 w−0.6 x2−3
x3=−28
15 w− 19
15 x2−4
−28
15 w− 19
15 x2−x3−4=0
x3=3+2 ( −x1−x2 ) +( 2 x1 + x2 +3
3 )
−4
3 x1− 5
3 x2−x3+ 4=0
−4
3 x1− 5
3 x2−x3+ 4=0
The Cartesian equation for π2 is −4
3 x1− 5
3 x2−x3+4=0
c) Let x1=w
π1 : ( w
x2
x3 )=
( 3
1
−1 )+ t
( 1
2
−2 )+ p ( 3
1
−3 )
Let x1=w
Parametric equations are
w=3+t +3 p------------------------------i
x2=1+2 t+ p………………………………..ii
x3=−1−2t−3 p -------------------------iii
From i and ii we have;
p=0.4 w−0.2 x2 −1 And t = x2 −w
3
Substituting p and t in iii
x3=−1−2t−3 p
x3=−1−2( x2−w
3 )−3 ¿ )
x3=−1−2
3 x2− 2
3 w−1.2 w−0.6 x2−3
x3=−28
15 w− 19
15 x2−4
−28
15 w− 19
15 x2−x3−4=0
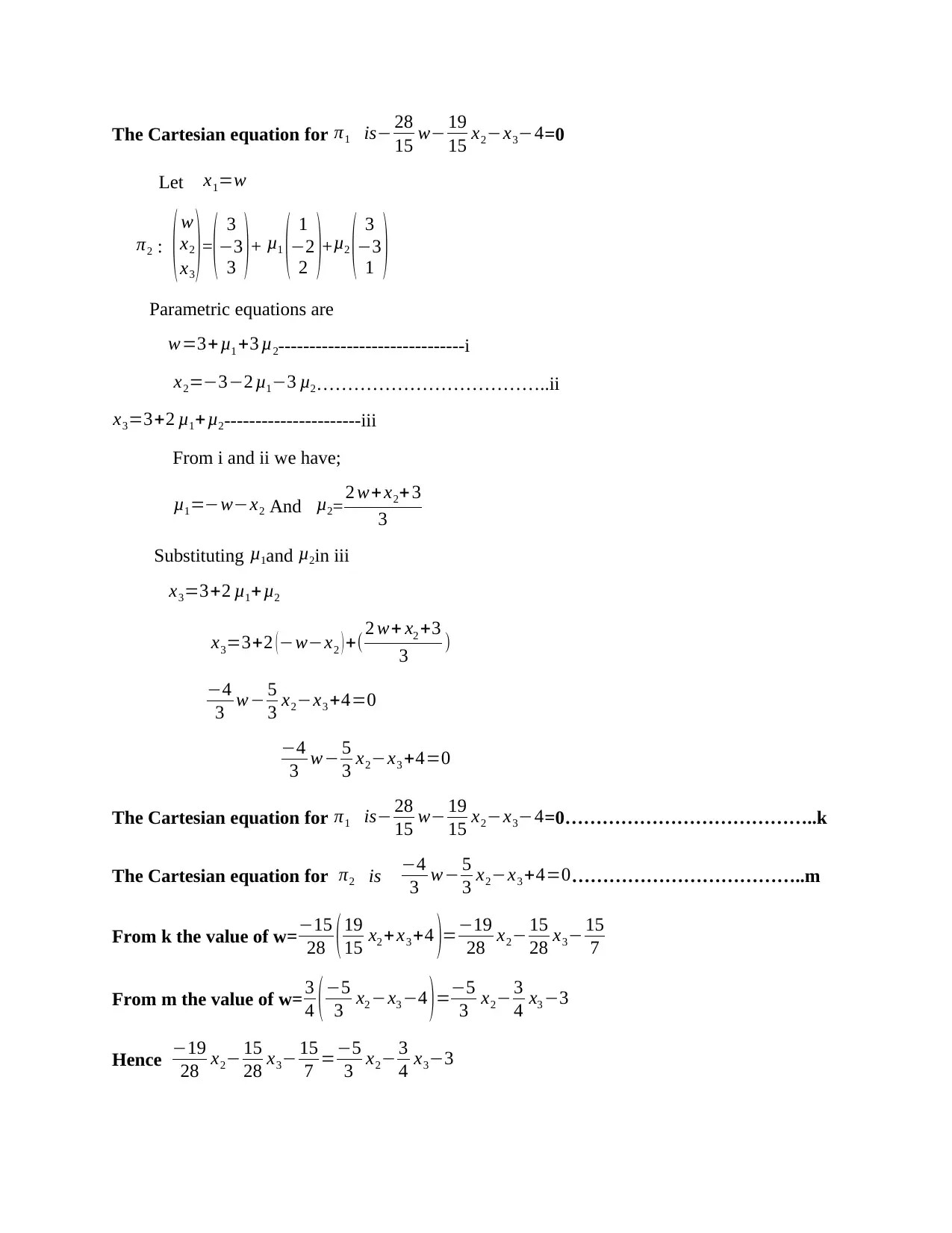
The Cartesian equation for π1 is− 28
15 w− 19
15 x2−x3−4=0
Let x1=w
π2 : ( w
x2
x3 )=
( 3
−3
3 )+ μ1
( 1
−2
2 )+ μ2
( 3
−3
1 )
Parametric equations are
w=3+ μ1 +3 μ2------------------------------i
x2=−3−2 μ1−3 μ2………………………………..ii
x3=3+2 μ1+ μ2----------------------iii
From i and ii we have;
μ1=−w−x2 And μ2= 2 w+ x2+ 3
3
Substituting μ1and μ2in iii
x3=3+2 μ1+ μ2
x3=3+2 (−w−x2 ) +( 2 w+ x2 +3
3 )
−4
3 w− 5
3 x2−x3 +4=0
−4
3 w− 5
3 x2−x3 +4=0
The Cartesian equation for π1 is− 28
15 w− 19
15 x2−x3−4=0…………………………………..k
The Cartesian equation for π2 is −4
3 w− 5
3 x2−x3 +4=0………………………………..m
From k the value of w=−15
28 (19
15 x2 +x3 +4 )=−19
28 x2− 15
28 x3− 15
7
From m the value of w= 3
4 ( −5
3 x2 −x3 −4 ) =−5
3 x2− 3
4 x3 −3
Hence −19
28 x2− 15
28 x3− 15
7 =−5
3 x2− 3
4 x3−3
15 w− 19
15 x2−x3−4=0
Let x1=w
π2 : ( w
x2
x3 )=
( 3
−3
3 )+ μ1
( 1
−2
2 )+ μ2
( 3
−3
1 )
Parametric equations are
w=3+ μ1 +3 μ2------------------------------i
x2=−3−2 μ1−3 μ2………………………………..ii
x3=3+2 μ1+ μ2----------------------iii
From i and ii we have;
μ1=−w−x2 And μ2= 2 w+ x2+ 3
3
Substituting μ1and μ2in iii
x3=3+2 μ1+ μ2
x3=3+2 (−w−x2 ) +( 2 w+ x2 +3
3 )
−4
3 w− 5
3 x2−x3 +4=0
−4
3 w− 5
3 x2−x3 +4=0
The Cartesian equation for π1 is− 28
15 w− 19
15 x2−x3−4=0…………………………………..k
The Cartesian equation for π2 is −4
3 w− 5
3 x2−x3 +4=0………………………………..m
From k the value of w=−15
28 (19
15 x2 +x3 +4 )=−19
28 x2− 15
28 x3− 15
7
From m the value of w= 3
4 ( −5
3 x2 −x3 −4 ) =−5
3 x2− 3
4 x3 −3
Hence −19
28 x2− 15
28 x3− 15
7 =−5
3 x2− 3
4 x3−3
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
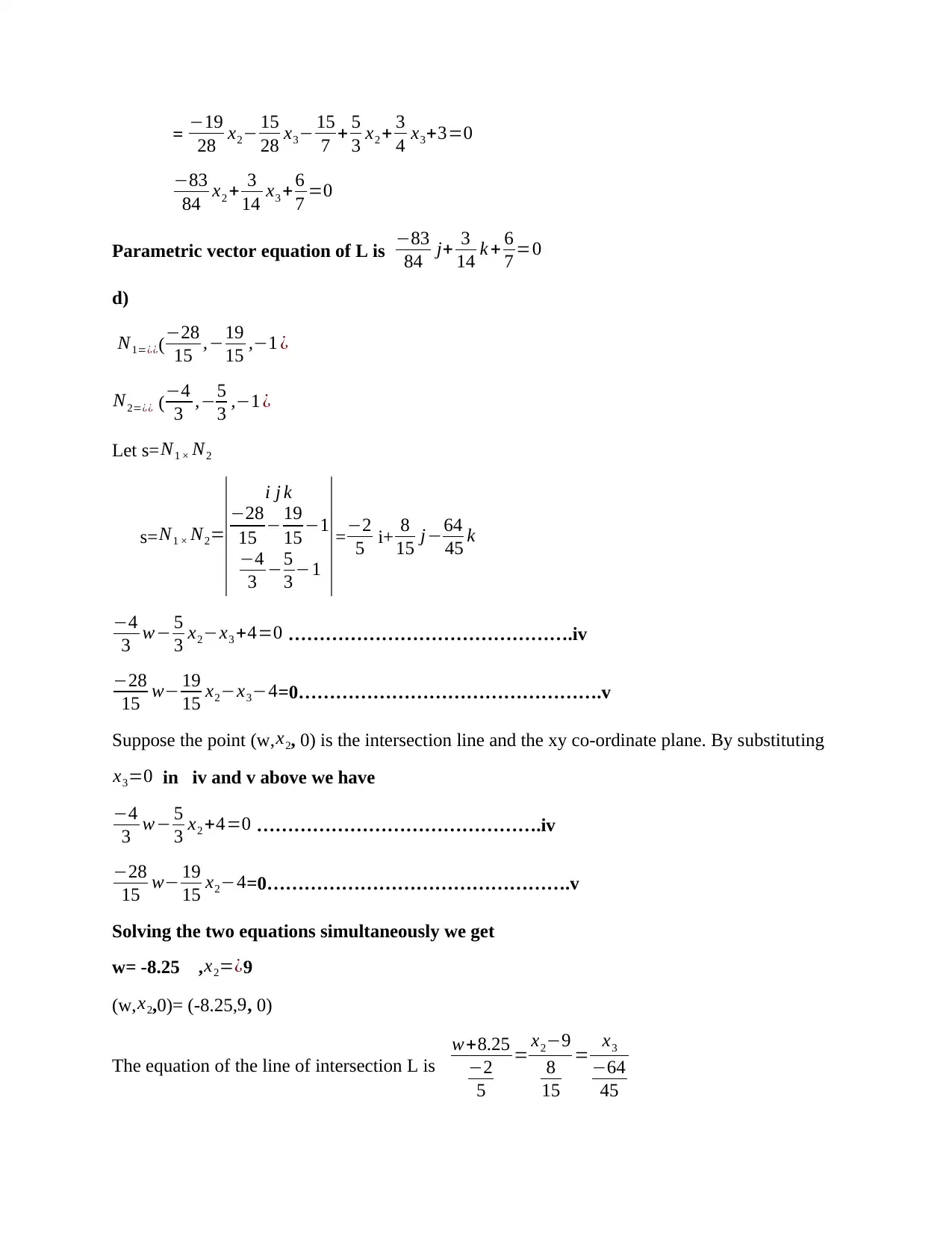
= −19
28 x2− 15
28 x3− 15
7 + 5
3 x2 + 3
4 x3+3=0
−83
84 x2 + 3
14 x3 + 6
7 =0
Parametric vector equation of L is −83
84 j+ 3
14 k + 6
7 =0
d)
N1=¿¿(−28
15 ,−19
15 ,−1 ¿
N2=¿¿ (−4
3 ,−5
3 ,−1 ¿
Let s=N1 × N2
s= N1 × N2=
| i j k
−28
15 − 19
15 −1
−4
3 −5
3 −1 |=−2
5 i+ 8
15 j−64
45 k
−4
3 w− 5
3 x2−x3 +4=0 ……………………………………….iv
−28
15 w− 19
15 x2−x3−4=0………………………………………….v
Suppose the point (w, x2, 0) is the intersection line and the xy co-ordinate plane. By substituting
x3=0 in iv and v above we have
−4
3 w− 5
3 x2 +4=0 ……………………………………….iv
−28
15 w− 19
15 x2−4=0………………………………………….v
Solving the two equations simultaneously we get
w= -8.25 , x2=¿9
(w,x2,0)= (-8.25,9, 0)
The equation of the line of intersection L is
w+8.25
−2
5
= x2−9
8
15
= x3
−64
45
28 x2− 15
28 x3− 15
7 + 5
3 x2 + 3
4 x3+3=0
−83
84 x2 + 3
14 x3 + 6
7 =0
Parametric vector equation of L is −83
84 j+ 3
14 k + 6
7 =0
d)
N1=¿¿(−28
15 ,−19
15 ,−1 ¿
N2=¿¿ (−4
3 ,−5
3 ,−1 ¿
Let s=N1 × N2
s= N1 × N2=
| i j k
−28
15 − 19
15 −1
−4
3 −5
3 −1 |=−2
5 i+ 8
15 j−64
45 k
−4
3 w− 5
3 x2−x3 +4=0 ……………………………………….iv
−28
15 w− 19
15 x2−x3−4=0………………………………………….v
Suppose the point (w, x2, 0) is the intersection line and the xy co-ordinate plane. By substituting
x3=0 in iv and v above we have
−4
3 w− 5
3 x2 +4=0 ……………………………………….iv
−28
15 w− 19
15 x2−4=0………………………………………….v
Solving the two equations simultaneously we get
w= -8.25 , x2=¿9
(w,x2,0)= (-8.25,9, 0)
The equation of the line of intersection L is
w+8.25
−2
5
= x2−9
8
15
= x3
−64
45
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
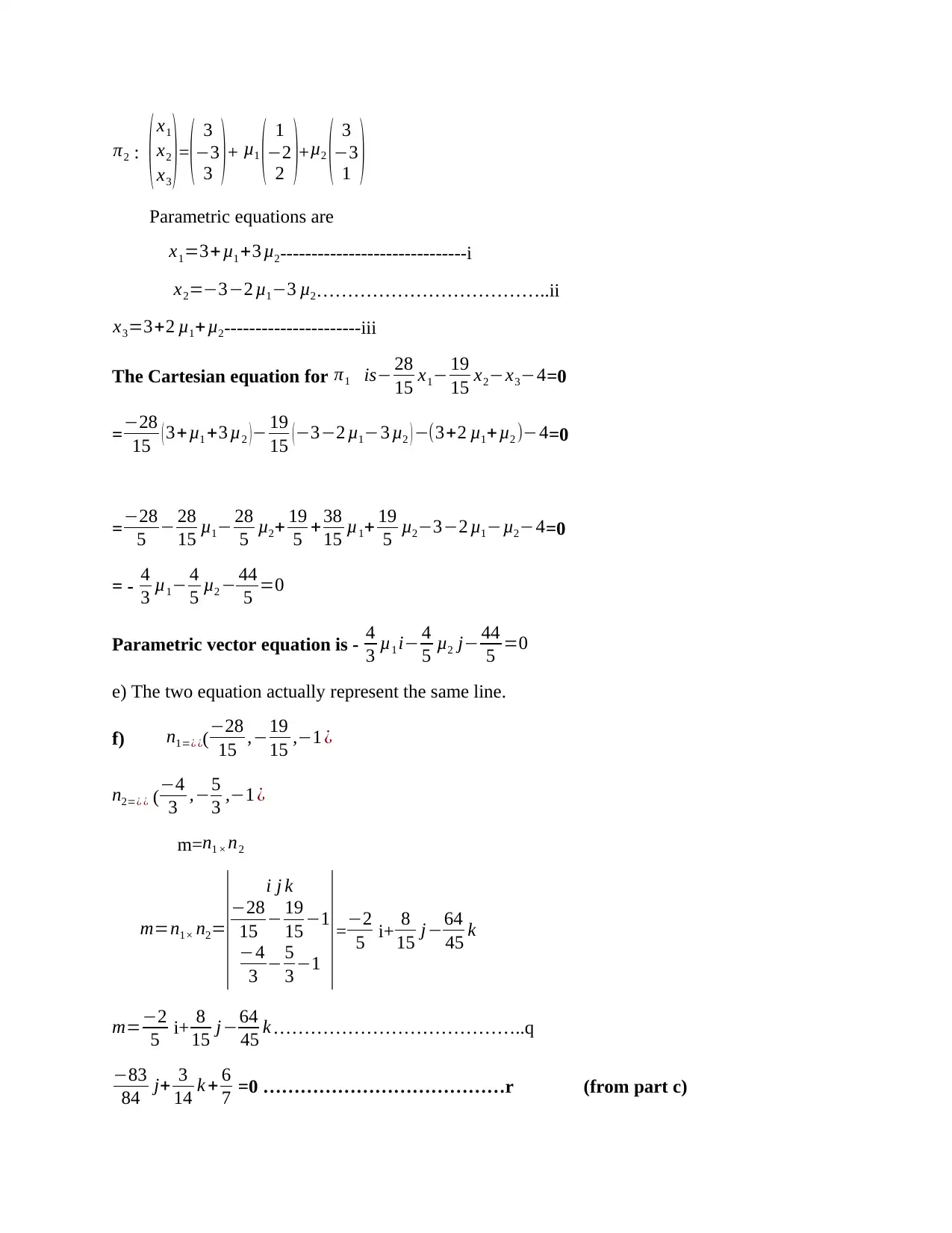
π2 : (x1
x2
x3
)=
( 3
−3
3 )+ μ1
( 1
−2
2 )+ μ2
( 3
−3
1 )
Parametric equations are
x1=3+ μ1 +3 μ2------------------------------i
x2=−3−2 μ1−3 μ2………………………………..ii
x3=3+2 μ1+ μ2----------------------iii
The Cartesian equation for π1 is− 28
15 x1− 19
15 x2−x3−4=0
= −28
15 ( 3+μ1 +3 μ2 )− 19
15 ( −3−2 μ1−3 μ2 ) −(3+2 μ1+μ2 )−4=0
=−28
5 − 28
15 μ1− 28
5 μ2+ 19
5 + 38
15 μ1+ 19
5 μ2−3−2 μ1−μ2−4=0
= - 4
3 μ1− 4
5 μ2 − 44
5 =0
Parametric vector equation is - 4
3 μ1 i− 4
5 μ2 j− 44
5 =0
e) The two equation actually represent the same line.
f) n1=¿ ¿(−28
15 ,−19
15 ,−1 ¿
n2=¿ ¿ (−4
3 ,−5
3 ,−1 ¿
m=n1 × n2
m=n1× n2=
| i j k
−28
15 − 19
15 −1
−4
3 − 5
3 −1 |=−2
5 i+ 8
15 j−64
45 k
m=−2
5 i+ 8
15 j−64
45 k…………………………………..q
−83
84 j+ 3
14 k + 6
7 =0 …………………………………r (from part c)
x2
x3
)=
( 3
−3
3 )+ μ1
( 1
−2
2 )+ μ2
( 3
−3
1 )
Parametric equations are
x1=3+ μ1 +3 μ2------------------------------i
x2=−3−2 μ1−3 μ2………………………………..ii
x3=3+2 μ1+ μ2----------------------iii
The Cartesian equation for π1 is− 28
15 x1− 19
15 x2−x3−4=0
= −28
15 ( 3+μ1 +3 μ2 )− 19
15 ( −3−2 μ1−3 μ2 ) −(3+2 μ1+μ2 )−4=0
=−28
5 − 28
15 μ1− 28
5 μ2+ 19
5 + 38
15 μ1+ 19
5 μ2−3−2 μ1−μ2−4=0
= - 4
3 μ1− 4
5 μ2 − 44
5 =0
Parametric vector equation is - 4
3 μ1 i− 4
5 μ2 j− 44
5 =0
e) The two equation actually represent the same line.
f) n1=¿ ¿(−28
15 ,−19
15 ,−1 ¿
n2=¿ ¿ (−4
3 ,−5
3 ,−1 ¿
m=n1 × n2
m=n1× n2=
| i j k
−28
15 − 19
15 −1
−4
3 − 5
3 −1 |=−2
5 i+ 8
15 j−64
45 k
m=−2
5 i+ 8
15 j−64
45 k…………………………………..q
−83
84 j+ 3
14 k + 6
7 =0 …………………………………r (from part c)

- 4
3 μ1 i− 4
5 μ2 j− 44
5 =0 ……………………………..s (from part d)
Comparing q and r i.e.
−2
5 i+ 8
15 j−64
45 k=g( −83
84 j+ 3
14 k + 6
7 ¿where g is a scalar.
It is clearly evident that q isn’t a scalar multiple of r hence the two vectors aren’t parallel.
Comparing q and s i.e.
−2
5 i+ 8
15 j−64
45 k=h( 4
3 μ1 i− 4
5 μ2 j− 44
5 )where h is a scalar.
It is clearly evident that q isn’t a scalar multiple of s hence the two vectors aren’t parallel.
g) For vectors to be parallel then one must be a scalar multiple of the other.
3. Let f= ( 16.022 ¿ ¿
3
4 , f 1=¿
16.022=16+X
X=0.022 Where X is the error
Absolute error=(0.022)
3
4 =0.057124
3 μ1 i− 4
5 μ2 j− 44
5 =0 ……………………………..s (from part d)
Comparing q and r i.e.
−2
5 i+ 8
15 j−64
45 k=g( −83
84 j+ 3
14 k + 6
7 ¿where g is a scalar.
It is clearly evident that q isn’t a scalar multiple of r hence the two vectors aren’t parallel.
Comparing q and s i.e.
−2
5 i+ 8
15 j−64
45 k=h( 4
3 μ1 i− 4
5 μ2 j− 44
5 )where h is a scalar.
It is clearly evident that q isn’t a scalar multiple of s hence the two vectors aren’t parallel.
g) For vectors to be parallel then one must be a scalar multiple of the other.
3. Let f= ( 16.022 ¿ ¿
3
4 , f 1=¿
16.022=16+X
X=0.022 Where X is the error
Absolute error=(0.022)
3
4 =0.057124
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
1 out of 9