Mathematics for Economics Mathematics for Economics

Different the following function F (x) = -4+3x-1 F(x) = -4+3(2x)-1=2 F(x) =-4+3(2x)-1=2(-4+3x-1) =-16+6x-1=-8+3x-1 =-16+6x-6x-1+2 =-+1 =-( X= The value of x is or - F(2x)=f(x) =-4+3(2x)-1=-4+3

Mathematics for Economics

Table of Contents
Part A...............................................................................................................................................3
Question 1....................................................................................................................................3
Question 2....................................................................................................................................3
Question 3....................................................................................................................................3
Question 4....................................................................................................................................3
Part -B..............................................................................................................................................3
Question 5....................................................................................................................................3
Question 6....................................................................................................................................3
Question 7....................................................................................................................................3
Question 8....................................................................................................................................3
2

Part A
Question 1
(a). Different the following function F (x) = -4x2+3x-1
F(x) = -4 x2+3x-1
F(2x)=2 F(x)
=-4 (2 x)2+3(2x)-1=2(-4 x2+3x-1)
=-16x2+6x-1=-8x2+3x-1
=-16 x2+8 x2+6x-6x-1+2
=-8 x2+1
=-( 8 x2−1 ¿
8 x2−1=0
8 x2=1
x2= 1
8
X= ± √ 1
8
The value of x is √ 1
8 or -
√ 1
8
F(2x)=f(x)
=-4 (2 x)2+3(2x)-1=-4 x2+3x-1
=-16x2+3x-1=4x2+3x-1
=-16 x2+4 x2+3x-3x-1+1
=- 12 x2+4x2=0
=-4x(3x-1)=0
-4x=0 3x-4=0
3

X=0 3x=4 x=4/3
The find the final value of the different function is x=0 and x=1
(b) g(x)=x2 ln x
The function g(x)= x2 ln x is the from f(x)=g(x).h(x) which makes it suitable for appliance of
equation is
F’(x)=g’(x)h(x)+g(x)h’(x)
Following value of each function,
G(x)=x2
H(x)= ln x
G’(x)= 2 x2
2
h’(x)= 1
x
When we substitute each of these into equation is
F’(x)= 2 x2
2 ln x + x2 ln x
= x2 ln x+ x2. 1
x
= x2 ln x + x
=x3 ln x
(c) h(x)= 3ex √ x3 +1
d
dx (h(x)=3ex √x3+1
The different function equation that can follows that,
d
dx (h(x))= dh(u)
du . du
dx ' =u=x
d
du (h(u)=h’(u)
4

d
dx (h’(x)) = d
dx (3ex √x3+1)
= d
dx (1+3ex √x3)
=3( d
dx (x
3
2).ex+x
3
2 . d
dx (ex)¿+0
=3( 3
2 x
3
2-1 .ex +e x . x
3
2 ¿
=3(x
3
2 e x+ 3 √x ex
3 )
=3x
3
2 e x+ 9 √ x ex
3
= 3 √ x (2 x+3)ex
2
2. For function f(x) in 1a, write down the difference ratio ∆ f
∆ x (equivalently indicated with ∆ f
h )
and show that the limit for the increment going to zero is equal to the derivative function.
Formally, show that lim
h→ 0
∆ f
h ( x )=f ' (x ) briefly discuss the meaning of the difference ratio.
3. In a carefully drawn and labelled two-dimensional Euclidean space, draw function f(x) and its
derivative, clearly indicating the coordinates of the maximisers, minimisers, maxima, minima,
and the intersections with both axes, and the intersections between the two functions. Discuss the
relationship between the graphs of f(x) and the graph of f’(x).
Let us start by thinking about the multidimensional functions we can graph:
5

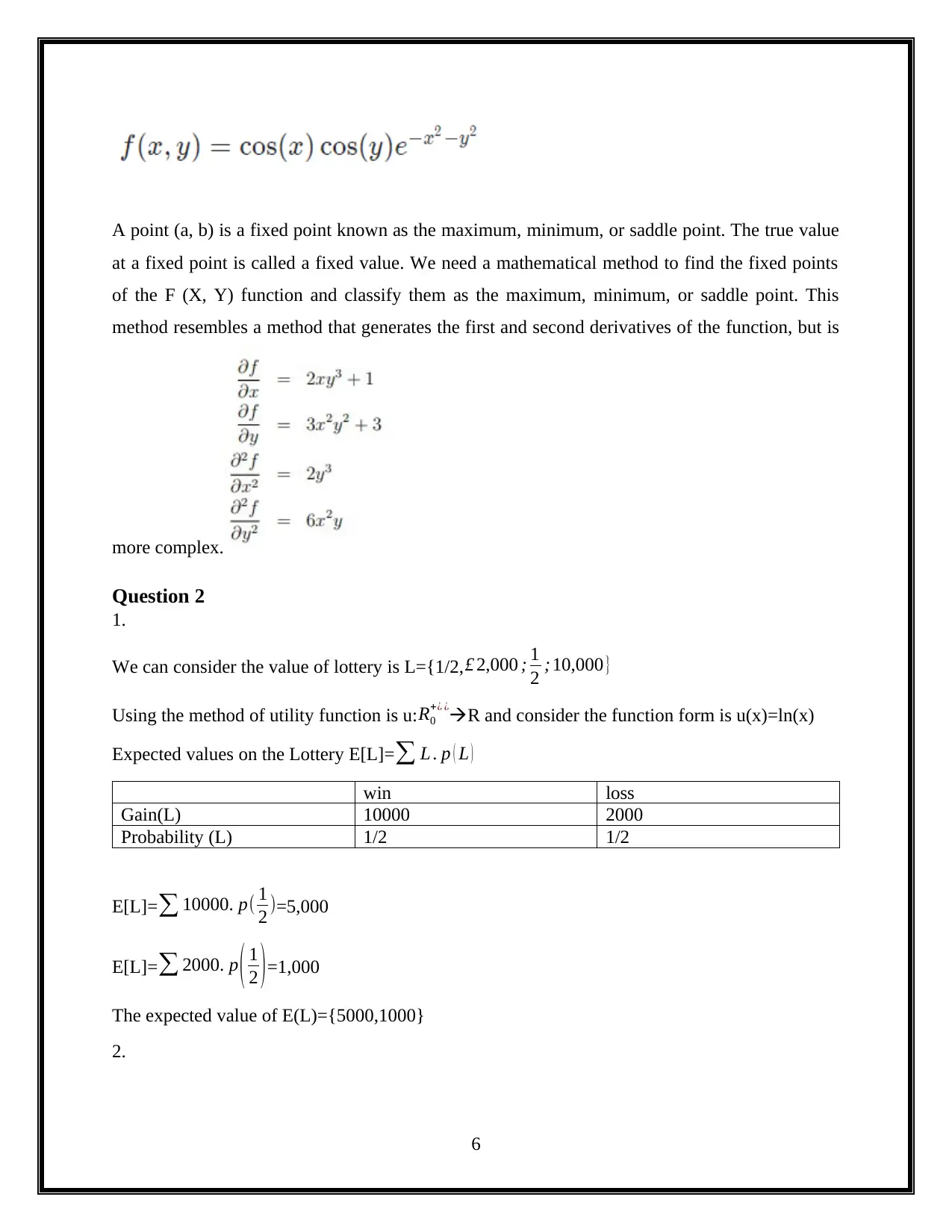
A point (a, b) is a fixed point known as the maximum, minimum, or saddle point. The true value
at a fixed point is called a fixed value. We need a mathematical method to find the fixed points
of the F (X, Y) function and classify them as the maximum, minimum, or saddle point. This
method resembles a method that generates the first and second derivatives of the function, but is
more complex.
Question 2
1.
We can consider the value of lottery is L={1/2,£ 2,000 ; 1
2 ;10,000}
Using the method of utility function is u: R0
+¿ ¿R and consider the function form is u(x)=ln(x)
Expected values on the Lottery E[L]=∑ L . p ( L )
win loss
Gain(L) 10000 2000
Probability (L) 1/2 1/2
E[L]=∑ 10000. p( 1
2 )=5,000
E[L]=∑ 2000. p ( 1
2 )=1,000
The expected value of E(L)={5000,1000}
2.
6

∫
a
b
¿ ¿)dx
∫
a
b
xdx+∫
a
b
1
x dx
=[ x
2 ]a
b
+[ln ( x )]a
b
3.
∫
a
b
¿ ¿)dx
∫
a
b
xdx+∫
a
b
1
x dx
=[ x
2 ]a
b
+[ln ( x )]a
b
=12%=0.12
Question 3
F(x) =1/5 (x3+1/x+2)
=1/5∫ x3 dx+∫ 1
x dx+2∫ dx
∫ 1
5 (x3+ 1
x + 2)dx
∫ x3 dx= x4
4
∫ 1
x dx=ln(x)
2∫ dx=2 x
1/5∫ x3 dx+∫ 1
x dx+2∫ dx= x4
4 + ln(x)+2 x+c
2
∫ 1
x dx=ln(x)=15,000
7

2∫ dx=2 x
1/5∫ x3 dx+∫ 1
x dx+2∫ dx= x4
4 + ln(x)+2 x+c
3.
=1/5∫ x3 dx+∫ 1
x dx+2∫ dx=12%
∫ 1
5 (x3+ 1
x + 2)dx
∫ x3 dx= x4
4
∫ 1
x dx=ln(x)
2∫ dx=2 x
1/5∫ x3 dx+∫ 1
x dx+2∫ dx= x4
4 + ln(x)+2 x+c
Question 4
Consider the following function
a)
f(x1,x2)=3x1
2-2 x1+x2
2-x1
3
=3 x1
2- x1
3+ x2
2−¿2x1
=x1
2(3- x1 ¿+x ¿-2)
3- x1=0 , x2-2=0
To find the two variable on the value is (-3,2)
b)
g(x1,x2)= 3 x1
2+2−10 x1−10 x2-2 x1 x2
= x1 ¿)-10( x1−x2)+ 2 x1 x2
3 x1+2 x2=0 x1−x2=0
8

To find the two variable on the value is (-3,-2)
=-3(3x-3+2x-2)-10(-3+2)+2(-3-2)
=41.
c)
h(x1,x2)=(x1
2−1 ¿( x 2+1)
Let as consider the two different equation is,
x1
2−1=0
x 2+1=0
x1
2=1
H(x1) =0.5
H(x2)=1
Two variable is (0.5,1)
e)
l(x1,x2)=ex 1 ex 2
l(x1)=ex 1
=∫ ex1dx= x ex 1
x 1
=∫ ex2dx= x ex 2
x 2
= x ex 1
x 1 . x ex 2
x 2
=x2( xex 1).x1 ( x ex2)
= x 2(xex 1) . x 1( x ex 2)
x 1−x 2
e)
l(x1,x2)= 2 x 1−x 2
x 1+2 x 2
l(x1)=∫2 x 1−x 2dx
=∫2 x 1 dx−¿∫ x 2¿dx
9

=2 x 2
2 - x 3
3
= 3 x 2+2 x 3
6 .2= 3 x 2+2 x 3
3
l(x1)=∫ x 1+ 2 x 2dx
=∫ x 1 dx+¿∫2 x 2 ¿dx
= x 2
2 -2. x 3
3
= 3 x 2+2 x 3
6 .2= 3 x 2+2 x 3
3
= 3 x 2+2 x 3
3 x 2+2 x 3 =1
1. Stationary points is f(x1, x2), g(x1, x2) and h(x1, x2)
x= x 1
2 - x
2 -2x
dx
dy =x1-x-2
x1-x-2=0
(x-2)(x-1)=0
(2,1)
X= 2
2- 1
2-2.1
=0.19
G(x1,x2) and h(x1, x2) of the standard points is (1,0.19)
2.
Consider a function y=f(x1,x1…xn) where the x0 are all independent, so each can very the
without affecting with other variables, suppose that only x1 changes then we will have the
difference quotient of the increase and decrease of the gradient function is
∆ y
∆ x 1 = f (x 1+∆ x 1 , x 2 , … … .. xn)
∆ x 1 + f ( x 1−∆ x 1 , x 2, … … .. xn)
∆ x 1
10

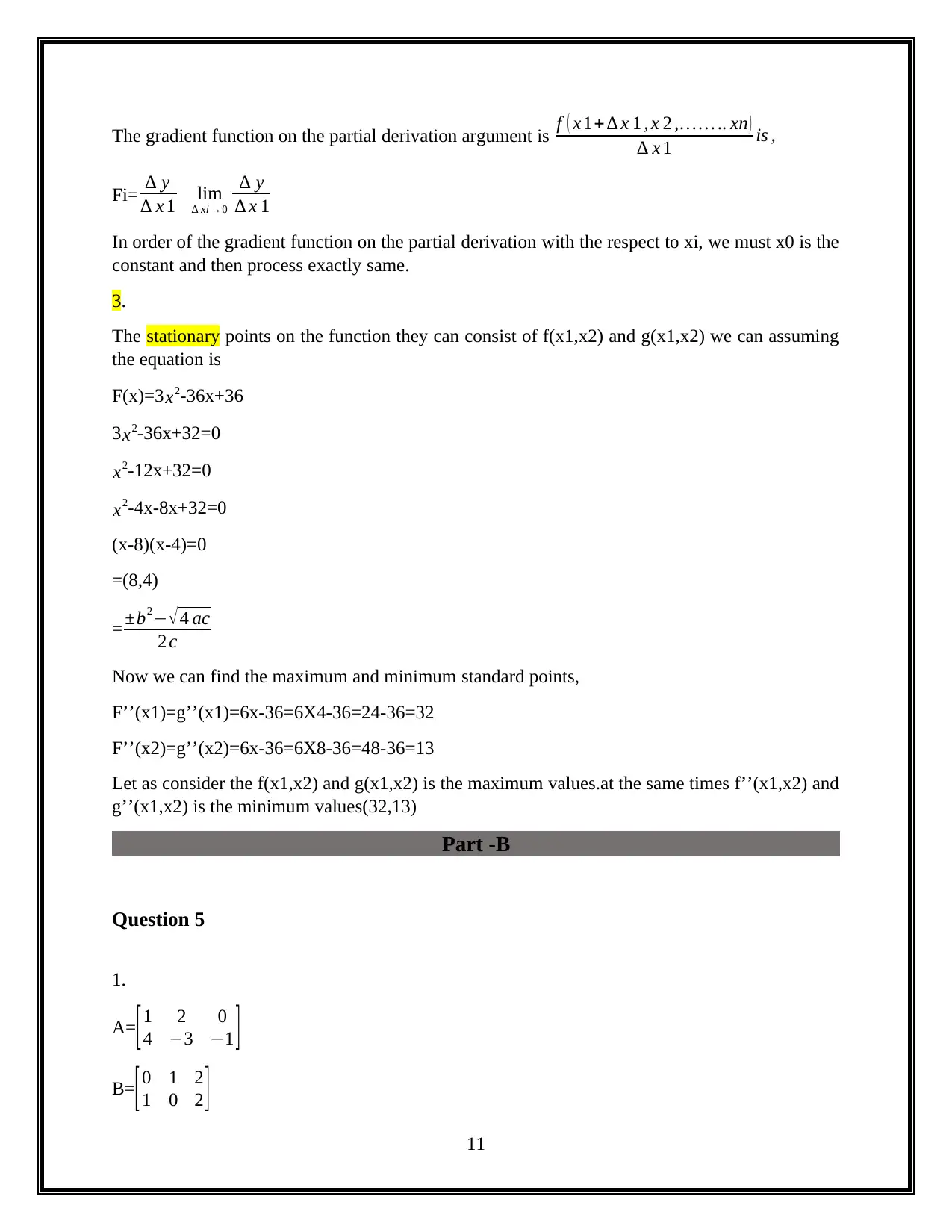
The gradient function on the partial derivation argument is f ( x 1+∆ x 1 , x 2 ,… … .. xn )
∆ x 1 is ,
Fi= ∆ y
∆ x 1 lim
∆ xi →0
∆ y
∆ x 1
In order of the gradient function on the partial derivation with the respect to xi, we must x0 is the
constant and then process exactly same.
3.
The stationary points on the function they can consist of f(x1,x2) and g(x1,x2) we can assuming
the equation is
F(x)=3 x2-36x+36
3x2-36x+32=0
x2-12x+32=0
x2-4x-8x+32=0
(x-8)(x-4)=0
=(8,4)
= ±b2− √ 4 ac
2 c
Now we can find the maximum and minimum standard points,
F’’(x1)=g’’(x1)=6x-36=6X4-36=24-36=32
F’’(x2)=g’’(x2)=6x-36=6X8-36=48-36=13
Let as consider the f(x1,x2) and g(x1,x2) is the maximum values.at the same times f’’(x1,x2) and
g’’(x1,x2) is the minimum values(32,13)
Part -B
Question 5
1.
A= [1 2 0
4 −3 −1 ]
B= [ 0 1 2
1 0 2 ]
11

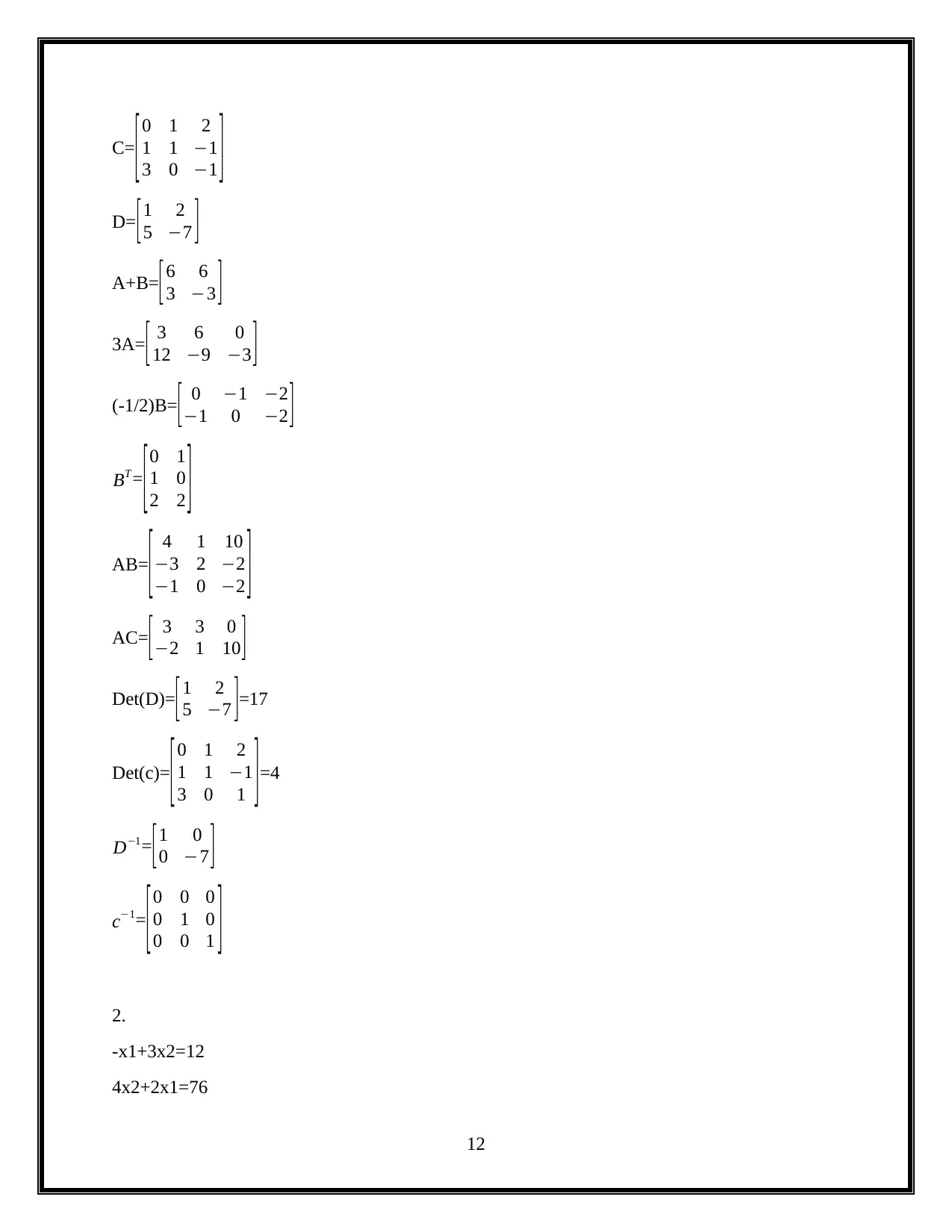
C= [0 1 2
1 1 −1
3 0 −1 ]
D= [1 2
5 −7 ]
A+B=[ 6 6
3 −3 ]
3A= [ 3 6 0
12 −9 −3 ]
(-1/2)B=[ 0 −1 −2
−1 0 −2 ]
BT =
[0 1
1 0
2 2 ]
AB= [ 4 1 10
−3 2 −2
−1 0 −2 ]
AC= [ 3 3 0
−2 1 10 ]
Det(D)= [ 1 2
5 −7 ]=17
Det(c)=
[0 1 2
1 1 −1
3 0 1 ]=4
D−1=[ 1 0
0 −7 ]
c−1=
[0 0 0
0 1 0
0 0 1 ]
2.
-x1+3x2=12
4x2+2x1=76
12

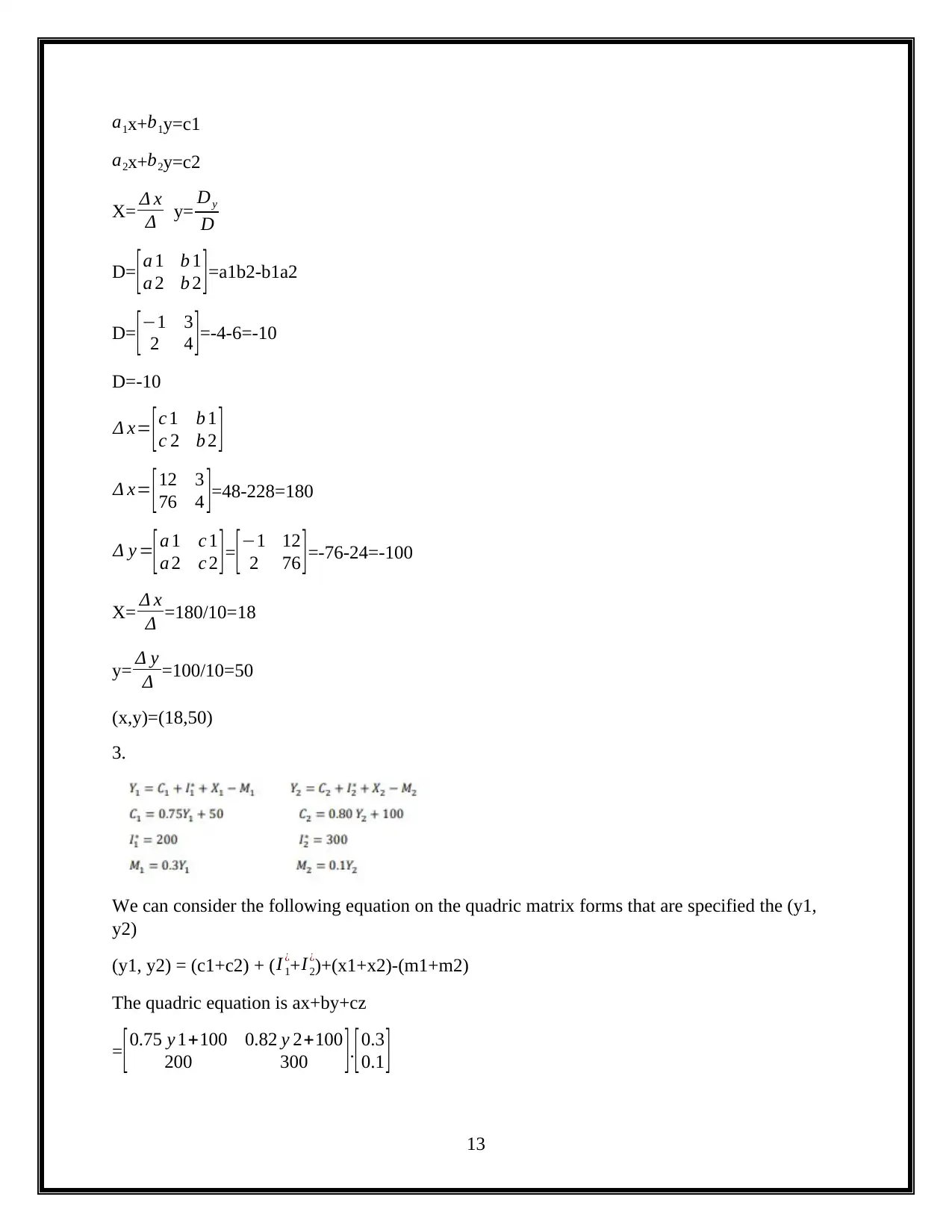
a1x+b1y=c1
a2x+b2y=c2
X= Δ x
Δ y= Dy
D
D= [a 1 b 1
a 2 b 2 ]=a1b2-b1a2
D= [ −1 3
2 4 ]=-4-6=-10
D=-10
Δ x= [c 1 b 1
c 2 b 2 ]
Δ x= [ 12 3
76 4 ]=48-228=180
Δ y =
[a 1 c 1
a 2 c 2 ]=[−1 12
2 76 ]=-76-24=-100
X= Δ x
Δ =180/10=18
y= Δ y
Δ =100/10=50
(x,y)=(18,50)
3.
We can consider the following equation on the quadric matrix forms that are specified the (y1,
y2)
(y1, y2) = (c1+c2) + (I 1
¿+ I 2
¿)+(x1+x2)-(m1+m2)
The quadric equation is ax+by+cz
=[0.75 y 1+100 0.82 y 2+100
200 300 ].[0.3
0.1 ]
13

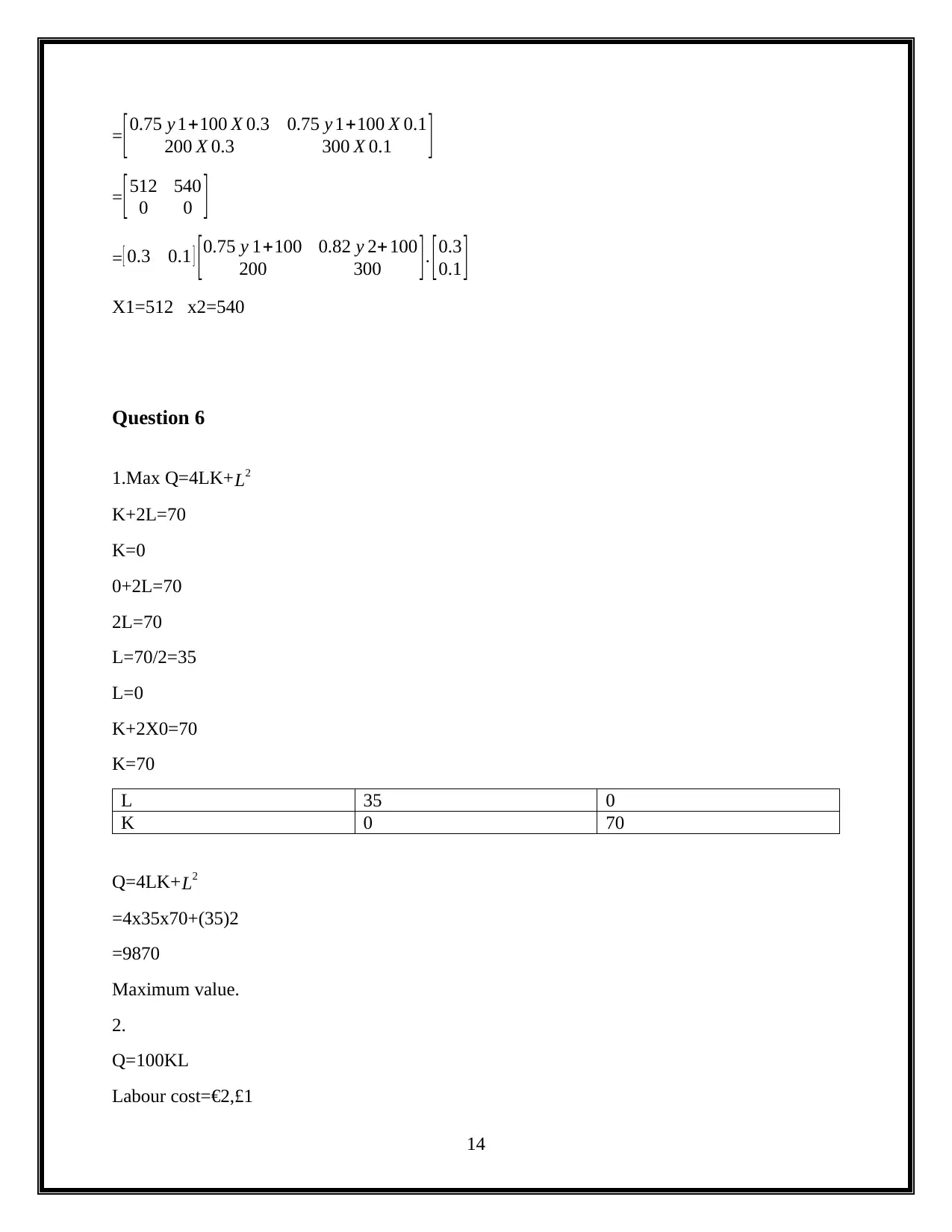
=[0.75 y 1+100 X 0.3 0.75 y 1+100 X 0.1
200 X 0.3 300 X 0.1 ]
=[ 512 540
0 0 ]
=[ 0.3 0.1 ] [0.75 y 1+100 0.82 y 2+ 100
200 300 ]. [0.3
0.1 ]
X1=512 x2=540
Question 6
1.Max Q=4LK+ L2
K+2L=70
K=0
0+2L=70
2L=70
L=70/2=35
L=0
K+2X0=70
K=70
L 35 0
K 0 70
Q=4LK+L2
=4x35x70+(35)2
=9870
Maximum value.
2.
Q=100KL
Labour cost=€2,£1
14

Contract unit=5000 units
F(q)=2x1+x2+100=5000
F’(q)= 2x1+2x2+300=5000
2x1+2x2=5000-300
2x1+2x2=4700
2(x1+x2)=4700
X1+x2=4700/2
=2650
iii).
Q=k α Lβ
C= pk
k + pL
L
f x(x,y)= (x,y)
f y(x,y)= λλ gx gy (x,y)
G(x,y)=0
Δ x= λ Δ g
Fk(k,L)= λ gk (x,y)
FL(k,L)= λ gL(x,y)
Fk(k,L)= pk
k + pL
L
= pk
k
pK + pL
L
pl
= pL ( pk
k ) +pK ( pL
L)
pK + pL
Question 7
Maximize -2x+2y
Constraints 1.5x+2y ≤ 6
x≥ 0∧ y ≥ 0
x=0
1.5x+2y ≤ 6
15

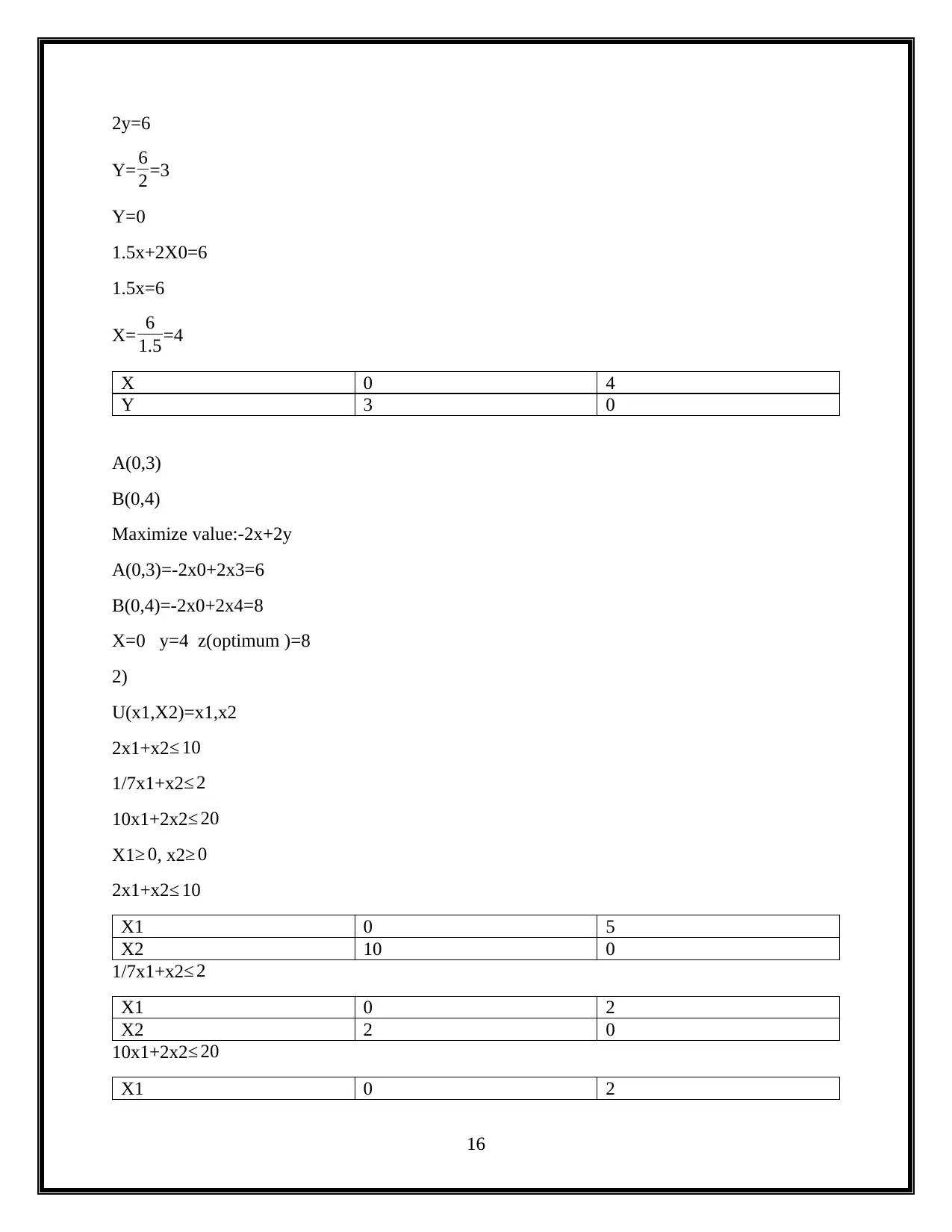
2y=6
Y= 6
2 =3
Y=0
1.5x+2X0=6
1.5x=6
X= 6
1.5=4
X 0 4
Y 3 0
A(0,3)
B(0,4)
Maximize value:-2x+2y
A(0,3)=-2x0+2x3=6
B(0,4)=-2x0+2x4=8
X=0 y=4 z(optimum )=8
2)
U(x1,X2)=x1,x2
2x1+x2≤ 10
1/7x1+x2≤ 2
10x1+2x2 ≤ 20
X1≥ 0, x2≥ 0
2x1+x2≤ 10
X1 0 5
X2 10 0
1/7x1+x2≤ 2
X1 0 2
X2 2 0
10x1+2x2 ≤ 20
X1 0 2
16

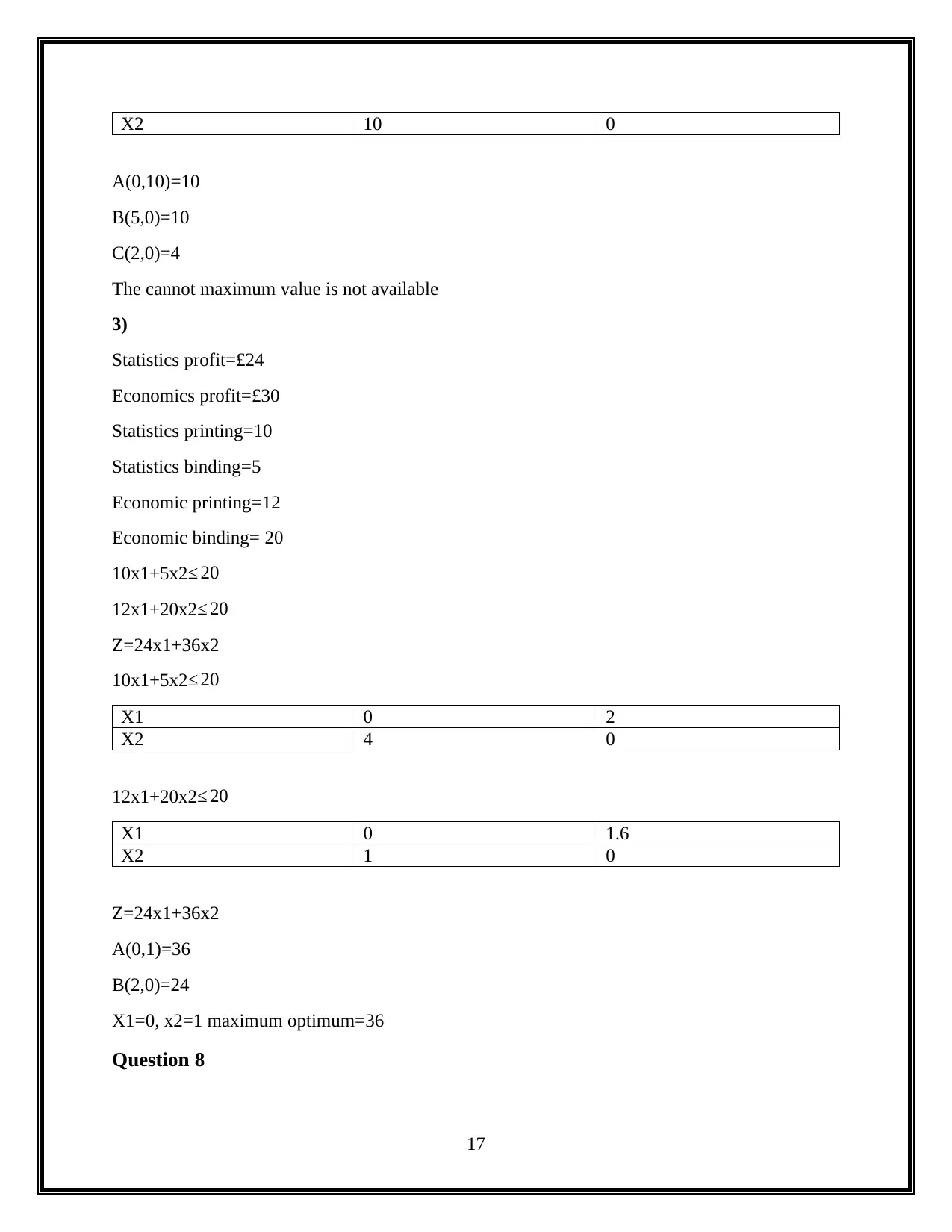
X2 10 0
A(0,10)=10
B(5,0)=10
C(2,0)=4
The cannot maximum value is not available
3)
Statistics profit=£24
Economics profit=£30
Statistics printing=10
Statistics binding=5
Economic printing=12
Economic binding= 20
10x1+5x2 ≤ 20
12x1+20x2≤ 20
Z=24x1+36x2
10x1+5x2 ≤ 20
X1 0 2
X2 4 0
12x1+20x2≤ 20
X1 0 1.6
X2 1 0
Z=24x1+36x2
A(0,1)=36
B(2,0)=24
X1=0, x2=1 maximum optimum=36
Question 8
17

Find indefinite integral of
F(x)=(x+1)
∫ ( x +1 ) dx= x2
2 +c
∫ ( x +1 ) dx=∫ f ( x ) dx ±∫ g ( x ) dx
F(x)dx= ∫ xdx +∫1 dx
∫ ( x +1 ) dx= x2
2 +x+c
We can add the indefinite constants is,
∫ ( x +1 ) dx= x2
2 +x+c
F(x)=x2+x
=∫( x2+x )dx
∫ ( x2 + x ¿ ) dx=∫ x2 dx+∫ xdx
= x3
3 + x2
2
= x2 (2 x +3)
6
We can add the constant values function
= x2 (2 x +3)
6 +c
F(x) =1/5 ( x3+1/x+2)
=1/5∫ x3 dx+∫ 1
x dx+2∫ dx
∫ 1
5 (x3+ 1
x + 2)dx
∫ x3 dx= x4
4
∫ 1
x dx=ln(x)
18

2∫ dx=2 x
1/5∫ x3 dx+∫ 1
x dx+2∫ dx= x4
4 + ln(x)+2 x+c
Let as consider the constant final value is,
x4
4 + ln(x)+ 2 x+c
Find the definite integral of
∫
0
2
¿ ¿+1) dx
We can apply the equation is
F(x)±g(x)dx=∫ f ( x ) dx ±∫ g ( x ) dx
∫
0
2
¿ ¿+∫
0
2
1 dx
[ x3
3 ]0
2
+[ x]0
2
[ 23
3 - 0
3 ]+[2-0]= 14
3
ii)
∫
a
b
¿ ¿)dx
∫
a
b
xdx+∫
a
b
1
x dx
=[ x
2 ]a
b
+[ln ( x )]a
b
=[ b2
2 - a2
2 ]+[ln ( b ) −ln (a)¿
∫
a
b
¿ ¿)dx=[ b2
2 - a2
2 ]+[ln ( b )−ln (a)¿
iii.
19

∫
−1
1
( 7 x+2 ) dx
∫
−1
1
7 xdx+∫
−1
1
2 dx
7[ x2
2 ]−1
1
+[ x]−1
1
=7(0)-2(2)=4
2)
Marginal cost function
Mc=2Q+6
Total cost=212
Q=6
To find total cost functions is q=8
Mc=2Q+6
Mc=F(q)=2q+6
Tc(q)= ∫ ( 2 q+ 6 ) dq
=∫2 qdq+ 6∫ dq
= 2q2
2 +6q+F
F is the constant value of integration Q=6
= 2 x (6)2
2 +6x6+212
=284
F is the constant value of integration Q=8
2 x (8)2
2 +6x8+212=324
3)
20

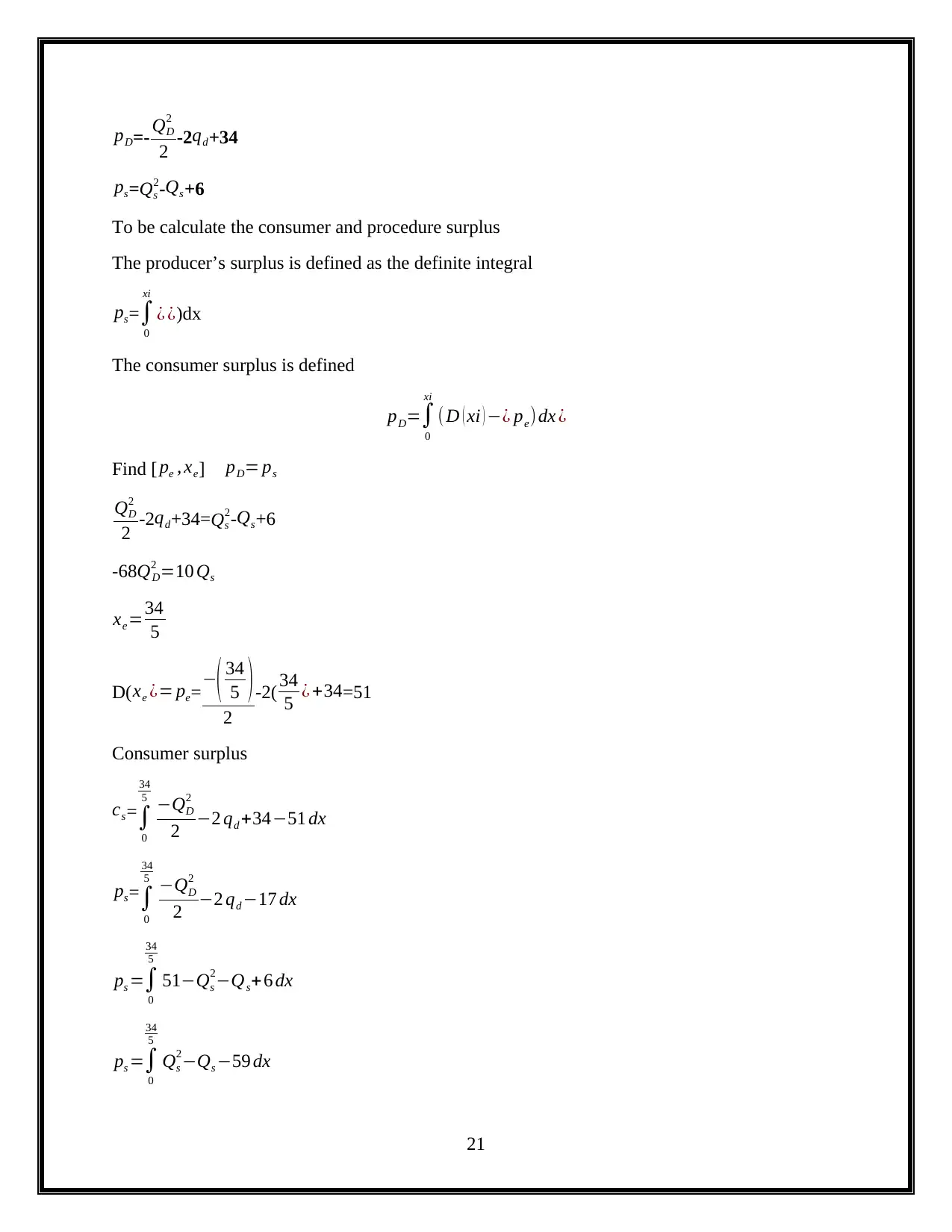
pD=- QD
2
2 -2qd+34
ps=Qs
2-Qs+6
To be calculate the consumer and procedure surplus
The producer’s surplus is defined as the definite integral
ps=∫
0
xi
¿ ¿)dx
The consumer surplus is defined
pD=∫
0
xi
(D ( xi ) −¿ pe)dx ¿
Find [ pe , xe] pD= ps
QD
2
2 -2qd+34= Qs
2-Qs+6
-68QD
2 =10 Qs
xe=34
5
D( xe ¿= pe=−( 34
5 )
2
-2( 34
5 ¿ +34=51
Consumer surplus
cs=∫
0
34
5 −QD
2
2 −2 qd +34−51 dx
ps=∫
0
34
5 −QD
2
2 −2 qd −17 dx
ps =∫
0
34
5
51−Qs
2−Qs+ 6 dx
ps =∫
0
34
5
Qs
2−Qs −59 dx
21

22

23

24