MATHEMATICS IN EARLY CHILDHOOD EDUCATION.
VerifiedAdded on 2023/04/21
|18
|3558
|91
AI Summary
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

Running head: MATHEMATICS IN EARLY CHILDHOOD EDUCATION
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Name of the Student
Name of the University
Author Note
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Name of the Student
Name of the University
Author Note
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

2
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Abstract
The main objective of imparting education during the early stages is to make the child familiar
with the basic concepts and ideas about the subject. It is therefore very important to create ways
to develop the lesson plans in a manner that creates impact in the child’s mind and provides the
student with a clear picture of the topic being taught. Mathematics being a critical subject
requires adequate skills that are to be developed at an early age through several practices that
allow the child to think and analyse the basic concepts of mathematics that we experience every
day. This paper will look into the theories of learning mathematics during early age and develop
lesson plans that can be implemented to impart mathematical learning at an early age. The paper
will finally conclude with a reflective assessment of the implementation of the study plan and
note the outcomes derived out of the implementation of the lesson plan.
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Abstract
The main objective of imparting education during the early stages is to make the child familiar
with the basic concepts and ideas about the subject. It is therefore very important to create ways
to develop the lesson plans in a manner that creates impact in the child’s mind and provides the
student with a clear picture of the topic being taught. Mathematics being a critical subject
requires adequate skills that are to be developed at an early age through several practices that
allow the child to think and analyse the basic concepts of mathematics that we experience every
day. This paper will look into the theories of learning mathematics during early age and develop
lesson plans that can be implemented to impart mathematical learning at an early age. The paper
will finally conclude with a reflective assessment of the implementation of the study plan and
note the outcomes derived out of the implementation of the lesson plan.

3
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Table of Contents
Introduction:....................................................................................................................................5
Theories in the learning of Mathematics.........................................................................................6
Piaget’s Theory of Child Development.......................................................................................6
Sensori-motor stage.................................................................................................................7
Pre-Operational stage...............................................................................................................7
Concrete Operational stage......................................................................................................8
Formal Operational Stage........................................................................................................8
Jerome Burner’s Theory of Three Modes of Representation......................................................9
Enactive mode.........................................................................................................................9
Iconic mode...........................................................................................................................10
Symbolic mode......................................................................................................................10
Lesson Plan for Teaching Mathematics to preschoolers...............................................................10
LESSON PLAN FOR THE AGE GROUP 4-5 YEARS...........................................................11
Learning outcomes:...............................................................................................................11
Lesson plan for 6 Years.................................................................................................................13
Simple addition..............................................................................................................................13
Understanding the concept of greater and smaller........................................................................14
Implementation and assessment of the Lesson Plan......................................................................14
Conclusion.....................................................................................................................................14
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Table of Contents
Introduction:....................................................................................................................................5
Theories in the learning of Mathematics.........................................................................................6
Piaget’s Theory of Child Development.......................................................................................6
Sensori-motor stage.................................................................................................................7
Pre-Operational stage...............................................................................................................7
Concrete Operational stage......................................................................................................8
Formal Operational Stage........................................................................................................8
Jerome Burner’s Theory of Three Modes of Representation......................................................9
Enactive mode.........................................................................................................................9
Iconic mode...........................................................................................................................10
Symbolic mode......................................................................................................................10
Lesson Plan for Teaching Mathematics to preschoolers...............................................................10
LESSON PLAN FOR THE AGE GROUP 4-5 YEARS...........................................................11
Learning outcomes:...............................................................................................................11
Lesson plan for 6 Years.................................................................................................................13
Simple addition..............................................................................................................................13
Understanding the concept of greater and smaller........................................................................14
Implementation and assessment of the Lesson Plan......................................................................14
Conclusion.....................................................................................................................................14

4
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Bibliography..................................................................................................................................16
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Bibliography..................................................................................................................................16
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

5
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Introduction:
The teaching of mathematics in early childhood is an important aspect and should be
appropriately addressed in order to ensure that the children develop skills that help them to
understand and think the concepts of mathematics in a constructive way. While developing
lesson plan for the children in their initial schooling year it should be kept in mind that the
instructional strategies that would be given to the children to cater their thinking and analytical
skills and help them to foster the growth of the mathematical knowledge. The developed nations
of the world such as United States and Australia have implemented good practices an
introspection into their educational framework will allow us to understand the teaching of critical
subjects such as mathematics. With respect to the educational framework of the country, it seems
that there is a necessity to restructure and revise the curriculum of the kindergarten schools and
though there is freedom to design the curriculum of the kindergarten schools it is important to
note that the curriculum satisfies the need of the children. Teaching mathematics to preschool
children might seem like an easy task, but it should be noted that the skills required to impart
such education require adequate knowledge of pedagogy, skill development techniques, and
effective learning and development skills along with the amalgamation of concepts in a way that
is lucid, attractive and easy to understand. The paper will look into the two different theories of
imparting education to children in their early stages and review these theories in way that can be
implemented to prepare lesson plans for the children. The following sections will go through the
theories of Piaget who propounded the ‘Theory of Child Development’ and Jerome Bruner’s
theory of ‘Three Modes of Representation’. Based on the understanding of these, a sample lesson
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Introduction:
The teaching of mathematics in early childhood is an important aspect and should be
appropriately addressed in order to ensure that the children develop skills that help them to
understand and think the concepts of mathematics in a constructive way. While developing
lesson plan for the children in their initial schooling year it should be kept in mind that the
instructional strategies that would be given to the children to cater their thinking and analytical
skills and help them to foster the growth of the mathematical knowledge. The developed nations
of the world such as United States and Australia have implemented good practices an
introspection into their educational framework will allow us to understand the teaching of critical
subjects such as mathematics. With respect to the educational framework of the country, it seems
that there is a necessity to restructure and revise the curriculum of the kindergarten schools and
though there is freedom to design the curriculum of the kindergarten schools it is important to
note that the curriculum satisfies the need of the children. Teaching mathematics to preschool
children might seem like an easy task, but it should be noted that the skills required to impart
such education require adequate knowledge of pedagogy, skill development techniques, and
effective learning and development skills along with the amalgamation of concepts in a way that
is lucid, attractive and easy to understand. The paper will look into the two different theories of
imparting education to children in their early stages and review these theories in way that can be
implemented to prepare lesson plans for the children. The following sections will go through the
theories of Piaget who propounded the ‘Theory of Child Development’ and Jerome Bruner’s
theory of ‘Three Modes of Representation’. Based on the understanding of these, a sample lesson

6
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
plan will be devised for two different age groups one belonging from 4-5 years and the other for
children of 6 years of age.
Theories in the learning of Mathematics
Among various theories that have been developed regarding the education in early child
hood, the theories of Piaget and Jerome Bruner gives considerable insight into the concept of
early childhood learning. These theories contribute greatly in the development of cognitive and
analytical skills of children. The following sections will discuss these theories in detail to
provide an insight into the theories and the way these theories can be implemented into learning
mathematics for children of early age.
Piaget’s Theory of Child Development
The theory of Child Development was brought forward by Jean Piaget, a child
psychologist who has contributed greatly through his research in cognitive development of
children. Based on the constructivism, Piaget emphasized the learning should be constructed
from personal experiences and the continuous linking of new learning with previously learned
concepts should be done to promote effective learning skills. Piaget has continuously stressed in
the various learning abilities deriving out of practical exercises and had identified that there are
different stages of learning in a children (Ghazi & Ullah, 2016). Based on this he devised the
famous Four Stage Theory of Development where he claims that there are various stages of
cognitive development which develop with a growing child starting from birth. On the basis of
this theory he divided the mental and cognitive development of children through four stages as
described below.
Sensori-motor stage
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
plan will be devised for two different age groups one belonging from 4-5 years and the other for
children of 6 years of age.
Theories in the learning of Mathematics
Among various theories that have been developed regarding the education in early child
hood, the theories of Piaget and Jerome Bruner gives considerable insight into the concept of
early childhood learning. These theories contribute greatly in the development of cognitive and
analytical skills of children. The following sections will discuss these theories in detail to
provide an insight into the theories and the way these theories can be implemented into learning
mathematics for children of early age.
Piaget’s Theory of Child Development
The theory of Child Development was brought forward by Jean Piaget, a child
psychologist who has contributed greatly through his research in cognitive development of
children. Based on the constructivism, Piaget emphasized the learning should be constructed
from personal experiences and the continuous linking of new learning with previously learned
concepts should be done to promote effective learning skills. Piaget has continuously stressed in
the various learning abilities deriving out of practical exercises and had identified that there are
different stages of learning in a children (Ghazi & Ullah, 2016). Based on this he devised the
famous Four Stage Theory of Development where he claims that there are various stages of
cognitive development which develop with a growing child starting from birth. On the basis of
this theory he divided the mental and cognitive development of children through four stages as
described below.
Sensori-motor stage

7
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Pre- operational stage
Concrete Operational stage
Formal Operational stage
In these stages of learning, the children develop certain skills which should be nurtured in a
certain way to develop learning skills in children. By identifying the various cognitive skills that
develop during these periods, the learning plan should be developed accordingly to foster
learning capacities within children.
Sensori-motor stage
This stage is the first stage right after birth till the child is two years of age. During this stage the
child develops the concept of object permanence which makes him identify and search things
that are known to the child. At this stage the child depends on their basic senses to develop their
skills through concrete experiences. During this stage the children learn only through their
interaction with the environment round them. They are ego centric at this stage. Therefore any
development in stage will require imparting knowledge through personal experiences and that
may include play activities and identification of basic shapes, colour and size in terms of bigger
or smaller. In the sensorimotor stage as identified by Piaget the cognitive skills develop till the
child is introduced to language. He also remarks that during this stage the child can relate the
concept of numbers by identifying the number of objects that he or she perceives. As for
example a child can understand the number of cows, or cars or toys that he generally relates to
from his surroundings. The development of the concept of counting can be enabled at this stage
by asking the child to count his fingers or the number of toys or similar such things.
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Pre- operational stage
Concrete Operational stage
Formal Operational stage
In these stages of learning, the children develop certain skills which should be nurtured in a
certain way to develop learning skills in children. By identifying the various cognitive skills that
develop during these periods, the learning plan should be developed accordingly to foster
learning capacities within children.
Sensori-motor stage
This stage is the first stage right after birth till the child is two years of age. During this stage the
child develops the concept of object permanence which makes him identify and search things
that are known to the child. At this stage the child depends on their basic senses to develop their
skills through concrete experiences. During this stage the children learn only through their
interaction with the environment round them. They are ego centric at this stage. Therefore any
development in stage will require imparting knowledge through personal experiences and that
may include play activities and identification of basic shapes, colour and size in terms of bigger
or smaller. In the sensorimotor stage as identified by Piaget the cognitive skills develop till the
child is introduced to language. He also remarks that during this stage the child can relate the
concept of numbers by identifying the number of objects that he or she perceives. As for
example a child can understand the number of cows, or cars or toys that he generally relates to
from his surroundings. The development of the concept of counting can be enabled at this stage
by asking the child to count his fingers or the number of toys or similar such things.
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

8
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Pre-Operational stage
During the preoperational stage there is gradual development with the basic skill sets remaining
same as the earlier stage. Children at this stage develop skills from concrete experiences similar
to the sensorimotor stage. This stage sees the development of cognitive intelligence where the
children start learning language skills and therefore can express their learning from sources that
guide the development of the children. The children also learn the concept of order and
sequencing in this stage. Children can solve one step problems in this step like simple addition
and subtraction, but since they still depend on concrete experiences the learning process must
include objects through which they can relate to their learning. It is also important to provide
them examples from their daily activities that help them to understand the knowledge of numbers
and counting. The visual representation of objects affects the children greatly at this stage and
therefore they can help create knowledge through attractive representation of objects in this
stage.
Concrete Operational stage
The concrete operational stage is a advanced stage where cognitive intelligence grows with
development and children develop thinking abilities. The classification of objects based on
certain characteristics and qualities develop in this stage. The children learn understanding
various concepts from more than one dimension. The concrete operational stage generally
includes children of the age of 7 to 11. This stage also sees development in the children’s
understanding of concepts from different perspectives other than visual representation. They can
also develop growth of multiple concepts and understand the various solutions to a problem.
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Pre-Operational stage
During the preoperational stage there is gradual development with the basic skill sets remaining
same as the earlier stage. Children at this stage develop skills from concrete experiences similar
to the sensorimotor stage. This stage sees the development of cognitive intelligence where the
children start learning language skills and therefore can express their learning from sources that
guide the development of the children. The children also learn the concept of order and
sequencing in this stage. Children can solve one step problems in this step like simple addition
and subtraction, but since they still depend on concrete experiences the learning process must
include objects through which they can relate to their learning. It is also important to provide
them examples from their daily activities that help them to understand the knowledge of numbers
and counting. The visual representation of objects affects the children greatly at this stage and
therefore they can help create knowledge through attractive representation of objects in this
stage.
Concrete Operational stage
The concrete operational stage is a advanced stage where cognitive intelligence grows with
development and children develop thinking abilities. The classification of objects based on
certain characteristics and qualities develop in this stage. The children learn understanding
various concepts from more than one dimension. The concrete operational stage generally
includes children of the age of 7 to 11. This stage also sees development in the children’s
understanding of concepts from different perspectives other than visual representation. They can
also develop growth of multiple concepts and understand the various solutions to a problem.

9
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Formal Operational Stage
Children belonging from 12 to 16 years of age are categorised in this stage of formal operational
stage and experiences further development of cognitive intelligence. The growth of the stages
includes development in learning from concrete experiences, and therefore more advanced
critical thinking is generated in the child. The cognitive development till adulthood is carried on
within the time frame and the development is continued till the child becomes an adult. Abstract
thinking and reasoning capabilities develop in this stage and thus help in the development of the
cognitive thinking skills in the time frame. Therefore in this stage it is important to include
learning strategies that require analysis, synthesis and hypothesizing of concepts. Children at
this stage are ready to understand concepts of higher mathematical order and mathematical
thinking.
Jerome Burner’s Theory of Three Modes of Representation.
Similar to Piaget’s Theory of child development, Jerome Burner a constructivist put forward his
theory of the ‘Three modes of Representation’ where he states that the outcome of cognitive
development relates to the thinking capacity of a child. According to Bruner, learning outcome
does not only involve concept building or cognitive thinking capabilities but also include the
ability to reinvent or generate innovative thinking in the process. Bruner thus explaining these
developments devised his famous theory where he stated that there are three modes of
representation through which cognitive development takes place in children and that children
should be allowed to construct and develop their own knowledge based on their experience and
cognitive intelligence (Bruner, 2017). Bruner with relation to these three different modes also
states that the transition from one mode to another mode does not infer that the other modes will
not be put to use rather with substantial growing, more cognitive application of the modes can be
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Formal Operational Stage
Children belonging from 12 to 16 years of age are categorised in this stage of formal operational
stage and experiences further development of cognitive intelligence. The growth of the stages
includes development in learning from concrete experiences, and therefore more advanced
critical thinking is generated in the child. The cognitive development till adulthood is carried on
within the time frame and the development is continued till the child becomes an adult. Abstract
thinking and reasoning capabilities develop in this stage and thus help in the development of the
cognitive thinking skills in the time frame. Therefore in this stage it is important to include
learning strategies that require analysis, synthesis and hypothesizing of concepts. Children at
this stage are ready to understand concepts of higher mathematical order and mathematical
thinking.
Jerome Burner’s Theory of Three Modes of Representation.
Similar to Piaget’s Theory of child development, Jerome Burner a constructivist put forward his
theory of the ‘Three modes of Representation’ where he states that the outcome of cognitive
development relates to the thinking capacity of a child. According to Bruner, learning outcome
does not only involve concept building or cognitive thinking capabilities but also include the
ability to reinvent or generate innovative thinking in the process. Bruner thus explaining these
developments devised his famous theory where he stated that there are three modes of
representation through which cognitive development takes place in children and that children
should be allowed to construct and develop their own knowledge based on their experience and
cognitive intelligence (Bruner, 2017). Bruner with relation to these three different modes also
states that the transition from one mode to another mode does not infer that the other modes will
not be put to use rather with substantial growing, more cognitive application of the modes can be

10
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
implemented in developed stages. The Three modes of representation as put forward by Bruner
as stated as flows:
Enactive mode
The enactive mode sees development of skill is based from information that is stored in
the memory and is expressed by physical action. The basis of the enactive mode lies in the
learning from action or activity rather than other forms of perception. This stage generally
observes learning through interaction and activity. For example a child might learn beating a
drum by learning from the sound generated by the beating of the drum with sticks.
Iconic mode
The Iconic mode is generally developed with the visual memory of the brain and this
stage experiences the development in the form of the mental images formed out of the visual
perception of the brain. This mode ensures that children are taught in an attractive manner that
includes illustrations, pictorial representations and graphical expression of ideas and thoughts.
Symbolic mode
The symbolic mode signifies the stage of cognitive development which is accompanied
by the storing information in the form of symbols or language. The representation of cognitive
skills is expressed in the forms of symbols and the process of learning is also involves learning
from symbols and languages. This mode denotes high order cognitive skills and intuitive
expression of thoughts through expression in symbolic forms.
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
implemented in developed stages. The Three modes of representation as put forward by Bruner
as stated as flows:
Enactive mode
The enactive mode sees development of skill is based from information that is stored in
the memory and is expressed by physical action. The basis of the enactive mode lies in the
learning from action or activity rather than other forms of perception. This stage generally
observes learning through interaction and activity. For example a child might learn beating a
drum by learning from the sound generated by the beating of the drum with sticks.
Iconic mode
The Iconic mode is generally developed with the visual memory of the brain and this
stage experiences the development in the form of the mental images formed out of the visual
perception of the brain. This mode ensures that children are taught in an attractive manner that
includes illustrations, pictorial representations and graphical expression of ideas and thoughts.
Symbolic mode
The symbolic mode signifies the stage of cognitive development which is accompanied
by the storing information in the form of symbols or language. The representation of cognitive
skills is expressed in the forms of symbols and the process of learning is also involves learning
from symbols and languages. This mode denotes high order cognitive skills and intuitive
expression of thoughts through expression in symbolic forms.
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
11
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Lesson Plan for Teaching Mathematics to preschoolers
After gaining insights from the above discussed theories we can understand that the learning
skills of a child would require appropriate approach to cater to is levels of understanding.
Teaching mathematical skills to children should therefore include lesson guides that are devised
according to the level of understanding and skill sets (Kaiser, 2014). The lesson plan should also
include techniques that facilitate the development cognitive intelligence within the children
allowing them to cultivate their minds in a way that allows them to understand the concepts of
mathematics in their own perception (Artzt et al., 2015). The following section will include two
lesson plans each for two different age groups and will be implemented in a manner that would
help to develop these skills in a constructive way. The first lesson plan will be devised for the
age group ranging from 4-5 years while the second lesson plan will involve a lesson plan for the
age group of 6 years.
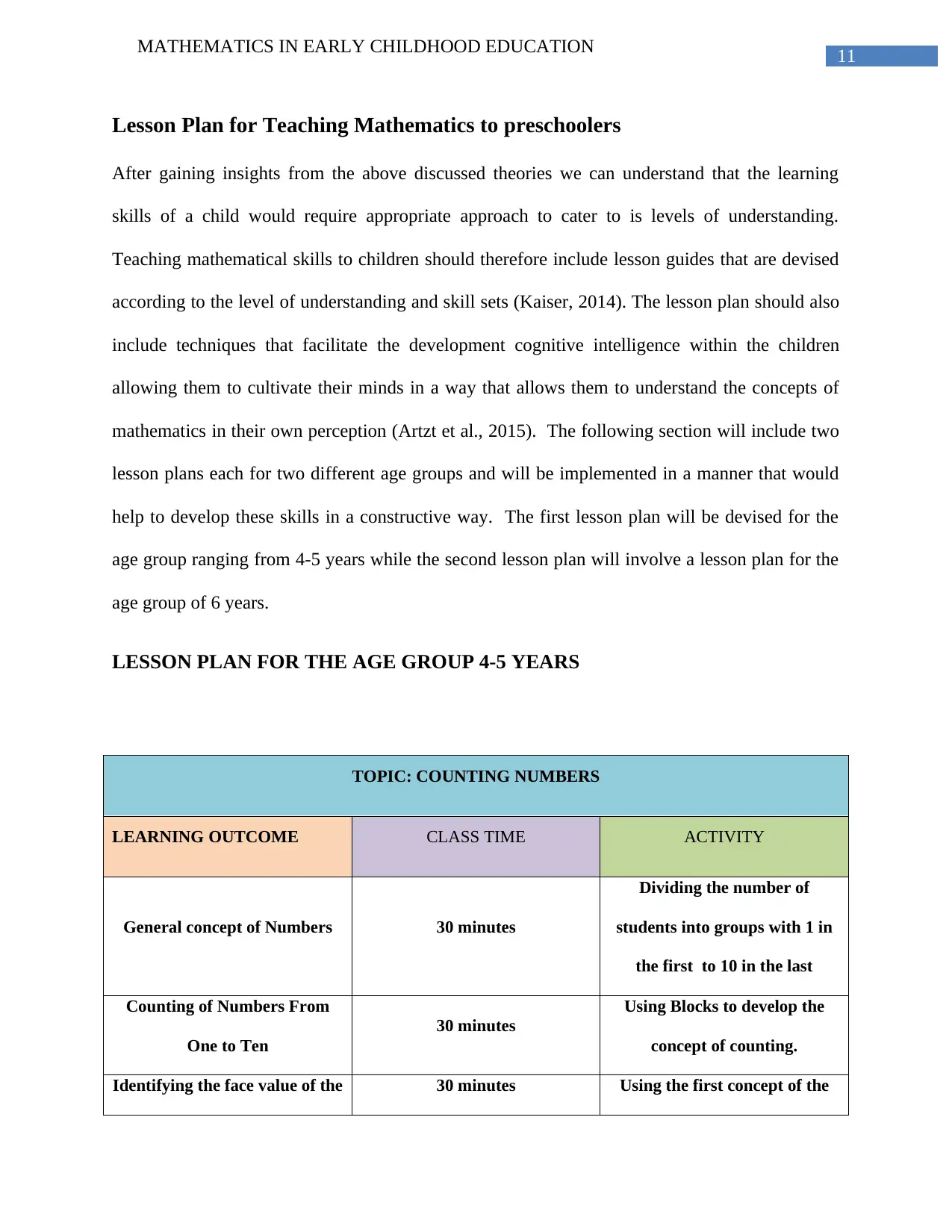
LESSON PLAN FOR THE AGE GROUP 4-5 YEARS
TOPIC: COUNTING NUMBERS
LEARNING OUTCOME CLASS TIME ACTIVITY
General concept of Numbers 30 minutes
Dividing the number of
students into groups with 1 in
the first to 10 in the last
Counting of Numbers From
One to Ten
30 minutes
Using Blocks to develop the
concept of counting.
Identifying the face value of the 30 minutes Using the first concept of the
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Lesson Plan for Teaching Mathematics to preschoolers
After gaining insights from the above discussed theories we can understand that the learning
skills of a child would require appropriate approach to cater to is levels of understanding.
Teaching mathematical skills to children should therefore include lesson guides that are devised
according to the level of understanding and skill sets (Kaiser, 2014). The lesson plan should also
include techniques that facilitate the development cognitive intelligence within the children
allowing them to cultivate their minds in a way that allows them to understand the concepts of
mathematics in their own perception (Artzt et al., 2015). The following section will include two
lesson plans each for two different age groups and will be implemented in a manner that would
help to develop these skills in a constructive way. The first lesson plan will be devised for the
age group ranging from 4-5 years while the second lesson plan will involve a lesson plan for the
age group of 6 years.
LESSON PLAN FOR THE AGE GROUP 4-5 YEARS
TOPIC: COUNTING NUMBERS
LEARNING OUTCOME CLASS TIME ACTIVITY
General concept of Numbers 30 minutes
Dividing the number of
students into groups with 1 in
the first to 10 in the last
Counting of Numbers From
One to Ten
30 minutes
Using Blocks to develop the
concept of counting.
Identifying the face value of the 30 minutes Using the first concept of the

12
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
numbers
groups, allowing the children
to identify the values of given
number of blocks or toys.
To attain the given outcomes, the outlined activities will be performed.
Learning outcomes:
General concept of Numbers: The class will be divided in to groups with numbers ranging
from 1 to 10. Since the class will not be that big, I have planned to make the group with the
available number of children and then amalgamate the groups as the numbers keep growing.
Once the groups are formed the children will be asked to count the number of children in each
group. This will help the children to remember the numbers by relating the groups they were part
of.
Counting of Numbers from One to Ten:
After this activity is complete the student will be given blocks arranged sequentially and asked to
write the numbers in the blocks. For example the block with only one cube will be written as
ONE and so. This will generate the skill of understanding the sequence of the numbers.
Identifying the face value of the numbers:
This activity will be performed with relation to the first activity that will be performed with the
children. This will allow them to understand the face value of the numbers. The children will be
given ping pong balls and asked to group them according to the value of the numbers in baskets
denoted with the numbers.
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
numbers
groups, allowing the children
to identify the values of given
number of blocks or toys.
To attain the given outcomes, the outlined activities will be performed.
Learning outcomes:
General concept of Numbers: The class will be divided in to groups with numbers ranging
from 1 to 10. Since the class will not be that big, I have planned to make the group with the
available number of children and then amalgamate the groups as the numbers keep growing.
Once the groups are formed the children will be asked to count the number of children in each
group. This will help the children to remember the numbers by relating the groups they were part
of.
Counting of Numbers from One to Ten:
After this activity is complete the student will be given blocks arranged sequentially and asked to
write the numbers in the blocks. For example the block with only one cube will be written as
ONE and so. This will generate the skill of understanding the sequence of the numbers.
Identifying the face value of the numbers:
This activity will be performed with relation to the first activity that will be performed with the
children. This will allow them to understand the face value of the numbers. The children will be
given ping pong balls and asked to group them according to the value of the numbers in baskets
denoted with the numbers.

13
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Through this activity the children will be acquainted with the concept of counting numbers by
activity and representation.
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Through this activity the children will be acquainted with the concept of counting numbers by
activity and representation.
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
14
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
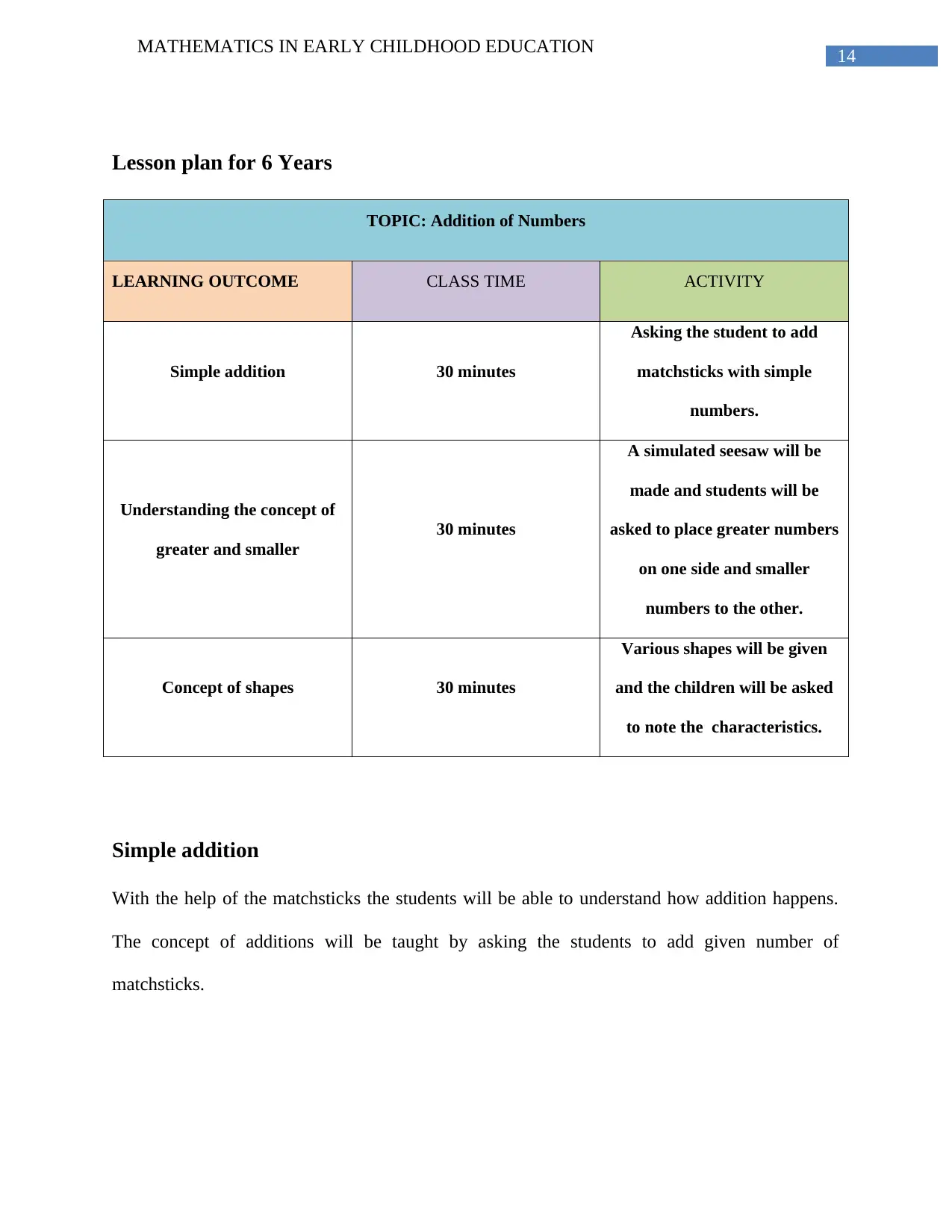
Lesson plan for 6 Years
TOPIC: Addition of Numbers
LEARNING OUTCOME CLASS TIME ACTIVITY
Simple addition 30 minutes
Asking the student to add
matchsticks with simple
numbers.
Understanding the concept of
greater and smaller
30 minutes
A simulated seesaw will be
made and students will be
asked to place greater numbers
on one side and smaller
numbers to the other.
Concept of shapes 30 minutes
Various shapes will be given
and the children will be asked
to note the characteristics.
Simple addition
With the help of the matchsticks the students will be able to understand how addition happens.
The concept of additions will be taught by asking the students to add given number of
matchsticks.
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Lesson plan for 6 Years
TOPIC: Addition of Numbers
LEARNING OUTCOME CLASS TIME ACTIVITY
Simple addition 30 minutes
Asking the student to add
matchsticks with simple
numbers.
Understanding the concept of
greater and smaller
30 minutes
A simulated seesaw will be
made and students will be
asked to place greater numbers
on one side and smaller
numbers to the other.
Concept of shapes 30 minutes
Various shapes will be given
and the children will be asked
to note the characteristics.
Simple addition
With the help of the matchsticks the students will be able to understand how addition happens.
The concept of additions will be taught by asking the students to add given number of
matchsticks.

15
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Understanding the concept of greater and smaller
The simulated seesaw will enable the students to understand the concept of greater and smaller
numbers. After the activity the students will be asked to write greater numbers on the lowered
side of the seesaw and the smaller numbers on the upper side of the seesaw.
Concept of shapes
The concept of shapes will be imparted by showing various shapes that are available in the
classroom and then ask them to identify the basic shapes with the, help of the previous
knowledge.
Implementation and assessment of the Lesson Plan
The implementation of the lesson plan was done in the local preschool and a positive response
was found among the children. With the children belonging to the age group of 4-5 years, they
enjoyed the activity immensely. The recollection at the end of the class proved that almost all the
children could understand the concept of the numbers and their Face values. Along with the
second lesson plan, the children took interest in learning the concept of addition and understood
the concept of simple addition. After performing the activity the children could add simple
numbers wit help of the fingers. Thus the implementation of the lesson plan was successful and
brought constructive results with the children.
Conclusion
Thus from the above discussion of theories regarding development of cognitive intelligence
among children it can be understood that children develop their cognitive and mental intelligence
through various stages and activities during the initial stages of growth. Inculcating these
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Understanding the concept of greater and smaller
The simulated seesaw will enable the students to understand the concept of greater and smaller
numbers. After the activity the students will be asked to write greater numbers on the lowered
side of the seesaw and the smaller numbers on the upper side of the seesaw.
Concept of shapes
The concept of shapes will be imparted by showing various shapes that are available in the
classroom and then ask them to identify the basic shapes with the, help of the previous
knowledge.
Implementation and assessment of the Lesson Plan
The implementation of the lesson plan was done in the local preschool and a positive response
was found among the children. With the children belonging to the age group of 4-5 years, they
enjoyed the activity immensely. The recollection at the end of the class proved that almost all the
children could understand the concept of the numbers and their Face values. Along with the
second lesson plan, the children took interest in learning the concept of addition and understood
the concept of simple addition. After performing the activity the children could add simple
numbers wit help of the fingers. Thus the implementation of the lesson plan was successful and
brought constructive results with the children.
Conclusion
Thus from the above discussion of theories regarding development of cognitive intelligence
among children it can be understood that children develop their cognitive and mental intelligence
through various stages and activities during the initial stages of growth. Inculcating these

16
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
techniques in the learning processes will enable children to develop constructive skills in
mathematics. Mathematics being a subject of analysis and logical thinking, it is evident that
children can develop mathematical skills from an early stage if proper techniques and
methodology is used which lays emphasis in the development of the child’s thinking and
reasoning capacities amalgamated with activities and illustrative representation of the
mathematical concepts . It is also understood that the thinking skills and high order critical
thinking depends on how well the child has been nourished with his or her inquisitiveness during
the process of their development. The implementation of mathematical skills by taking examples
from daily activities that the children perform can develop their cognitive skills accordingly.
Thus it can be concluded that children have their own mechanism to perceive and think and these
develop with their growth, thus with the proper devising of the learning techniques that sync with
the cognitive skills of the children will enable them to learn mathematics and other logical
disciplines with great interest and will also develop their knowledge based on these skills.
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
techniques in the learning processes will enable children to develop constructive skills in
mathematics. Mathematics being a subject of analysis and logical thinking, it is evident that
children can develop mathematical skills from an early stage if proper techniques and
methodology is used which lays emphasis in the development of the child’s thinking and
reasoning capacities amalgamated with activities and illustrative representation of the
mathematical concepts . It is also understood that the thinking skills and high order critical
thinking depends on how well the child has been nourished with his or her inquisitiveness during
the process of their development. The implementation of mathematical skills by taking examples
from daily activities that the children perform can develop their cognitive skills accordingly.
Thus it can be concluded that children have their own mechanism to perceive and think and these
develop with their growth, thus with the proper devising of the learning techniques that sync with
the cognitive skills of the children will enable them to learn mathematics and other logical
disciplines with great interest and will also develop their knowledge based on these skills.
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

17
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Bibliography
Artzt, A. F., Armour-Thomas, E., Curcio, F. R., & Gurl, T. J. (2015). Becoming a reflective
mathematics teacher: A guide for observations and self-assessment. Routledge.
Bruner, J. (2017). A study of thinking. Routledge.
Dunekacke, S., Jenßen, L., Eilerts, K., & Blömeke, S. (2016). Epistemological beliefs of
prospective preschool teachers and their relation to knowledge, perception, and planning
abilities in the field of mathematics: a process model. ZDM, 48(1-2), 125-137.
Ghazi, S. R., & Ullah, K. (2016). CONCRETE OPERATIONAL STAGE OF PIAGET’S
COGNITIVE DEVELOPMENT THEORY: AN IMPLICATION IN LEARNING
MATHEMATICS. Gomal University Journal of Research, 32(1).
Kaiser, G. (2014). Mathematical modelling and applications in education. In Encyclopedia of
mathematics education (pp. 396-404). Springer, Dordrecht.
Kelly, A. E., & Lesh, R. A. (2012). Handbook of research design in mathematics and science
education. Routledge.
Kermani, H., & Aldemir, J. (2015). Preparing children for success: integrating science, math, and
technology in early childhood classroom. Early Child Development and Care, 185(9),
1504-1527.
Ojose, B. (2008). Applying Piaget's theory of cognitive development to mathematics
instruction. The Mathematics Educator, 18(1).
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Bibliography
Artzt, A. F., Armour-Thomas, E., Curcio, F. R., & Gurl, T. J. (2015). Becoming a reflective
mathematics teacher: A guide for observations and self-assessment. Routledge.
Bruner, J. (2017). A study of thinking. Routledge.
Dunekacke, S., Jenßen, L., Eilerts, K., & Blömeke, S. (2016). Epistemological beliefs of
prospective preschool teachers and their relation to knowledge, perception, and planning
abilities in the field of mathematics: a process model. ZDM, 48(1-2), 125-137.
Ghazi, S. R., & Ullah, K. (2016). CONCRETE OPERATIONAL STAGE OF PIAGET’S
COGNITIVE DEVELOPMENT THEORY: AN IMPLICATION IN LEARNING
MATHEMATICS. Gomal University Journal of Research, 32(1).
Kaiser, G. (2014). Mathematical modelling and applications in education. In Encyclopedia of
mathematics education (pp. 396-404). Springer, Dordrecht.
Kelly, A. E., & Lesh, R. A. (2012). Handbook of research design in mathematics and science
education. Routledge.
Kermani, H., & Aldemir, J. (2015). Preparing children for success: integrating science, math, and
technology in early childhood classroom. Early Child Development and Care, 185(9),
1504-1527.
Ojose, B. (2008). Applying Piaget's theory of cognitive development to mathematics
instruction. The Mathematics Educator, 18(1).

18
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Tsamir, P., Tirosh, D., Levenson, E., Tabach, M., & Barkai, R. (2014). Developing preschool
teachers’ knowledge of students’ number conceptions. Journal of Mathematics Teacher
Education, 17(1), 61-83.
MATHEMATICS IN EARLY CHILDHOOD EDUCATION
Tsamir, P., Tirosh, D., Levenson, E., Tabach, M., & Barkai, R. (2014). Developing preschool
teachers’ knowledge of students’ number conceptions. Journal of Mathematics Teacher
Education, 17(1), 61-83.
1 out of 18
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.




