Maths Study Material and Solved Assignments
VerifiedAdded on 2023/01/17
|19
|2010
|36
AI Summary
This document provides study material and solved assignments for Maths. It covers topics like law of sine, amplitude and period of waveform, determinant of matrix, constructing histograms, and more.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

Maths
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
TASK 1
1.1 In ∆ABC, A = 530 , B = 610 and length of a = 12.6cm
So, b and c can be calculated by following way -
using law of sine -
a = b = c
Sin A Sin B Sin C
from, given ∆ -
A + B + C = 180
530 + 610 + C = 180
C = 660
therefore,
12.6 = b = c
Sin 530 Sin 610 Sin 660
12.6 = b = c
0.79 0.87 0.91
so, b = 13.87 cm
while, c = 14.51 cm
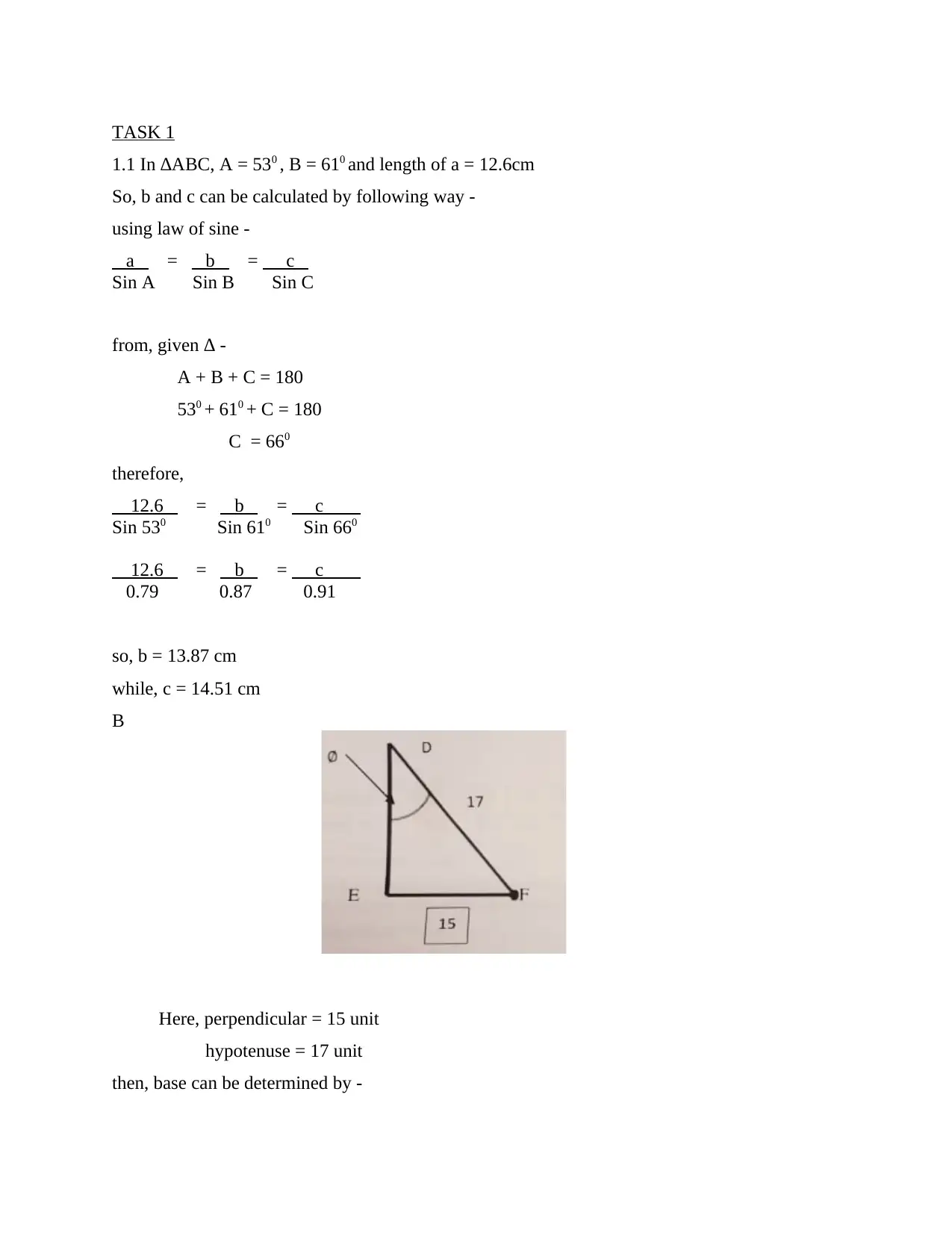
B
Here, perpendicular = 15 unit
hypotenuse = 17 unit
then, base can be determined by -
1.1 In ∆ABC, A = 530 , B = 610 and length of a = 12.6cm
So, b and c can be calculated by following way -
using law of sine -
a = b = c
Sin A Sin B Sin C
from, given ∆ -
A + B + C = 180
530 + 610 + C = 180
C = 660
therefore,
12.6 = b = c
Sin 530 Sin 610 Sin 660
12.6 = b = c
0.79 0.87 0.91
so, b = 13.87 cm
while, c = 14.51 cm
B
Here, perpendicular = 15 unit
hypotenuse = 17 unit
then, base can be determined by -

b2 = h2 – p2
= 172 – 152
= 289 – 225
= 64
or, b = √64 = 8 unit
Sin Ø = perpendicular / hypotenuse
Sin Ø = 15/17 unit
TASK 2
a) Amplitude and period of waveform
y = 4 cos(2θ + 450)
Amplitude of given equation is 4
and, period of above equation = 2 π / 2θ = π / θ
a) Amplitude and period of waveform
y = 6 sin (t - 300)
Amplitude of given equation is 6
and, period of above equation = 2 π / t
c) To Prove -
sin 2 x (sec x + cosec x) = 1 + tan x
cosx tanx
taking LHS side of given equation-
sin 2 x (sec x + cosec x)
cosx tanx
» sin 2 x (1/cosx + 1/sinx )
cox . sinx/cosx
» sin 2 x (sin x + cos x)/ sinx cosx
cox . sinx/cosx
» sinx + cos x
cox
» tanx + 1 = RHS Hence Proved
2
= 172 – 152
= 289 – 225
= 64
or, b = √64 = 8 unit
Sin Ø = perpendicular / hypotenuse
Sin Ø = 15/17 unit
TASK 2
a) Amplitude and period of waveform
y = 4 cos(2θ + 450)
Amplitude of given equation is 4
and, period of above equation = 2 π / 2θ = π / θ
a) Amplitude and period of waveform
y = 6 sin (t - 300)
Amplitude of given equation is 6
and, period of above equation = 2 π / t
c) To Prove -
sin 2 x (sec x + cosec x) = 1 + tan x
cosx tanx
taking LHS side of given equation-
sin 2 x (sec x + cosec x)
cosx tanx
» sin 2 x (1/cosx + 1/sinx )
cox . sinx/cosx
» sin 2 x (sin x + cos x)/ sinx cosx
cox . sinx/cosx
» sinx + cos x
cox
» tanx + 1 = RHS Hence Proved
2

Task 2
Given Indical equation -
x2 (2x-3)
(32) + (3) = 27
2x2 (2x-3) 3
(3) + (3) = 3
Using exponent rules,
2x2 + 2x – 3 3
3 = 3
3
Given Indical equation -
x2 (2x-3)
(32) + (3) = 27
2x2 (2x-3) 3
(3) + (3) = 3
Using exponent rules,
2x2 + 2x – 3 3
3 = 3
3
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

When base of equation is same, then power is also equal -
therefore,
2x2 + 2x – 3 = 3
or, 2x2 + 2x – 6 = 0
or, x2 + x – 3 = 0
Solving this quadratic equation -
x = -b ± √ (b2 – 4ac)
2a
x = -1 ± √ (12 – 4 x 1 x -3)
2 x 1
= -1 ±√13
3
therefore, x = (-1 + √13)/ 2 or, (-1 - √13)/ 2
4
therefore,
2x2 + 2x – 3 = 3
or, 2x2 + 2x – 6 = 0
or, x2 + x – 3 = 0
Solving this quadratic equation -
x = -b ± √ (b2 – 4ac)
2a
x = -1 ± √ (12 – 4 x 1 x -3)
2 x 1
= -1 ±√13
3
therefore, x = (-1 + √13)/ 2 or, (-1 - √13)/ 2
4
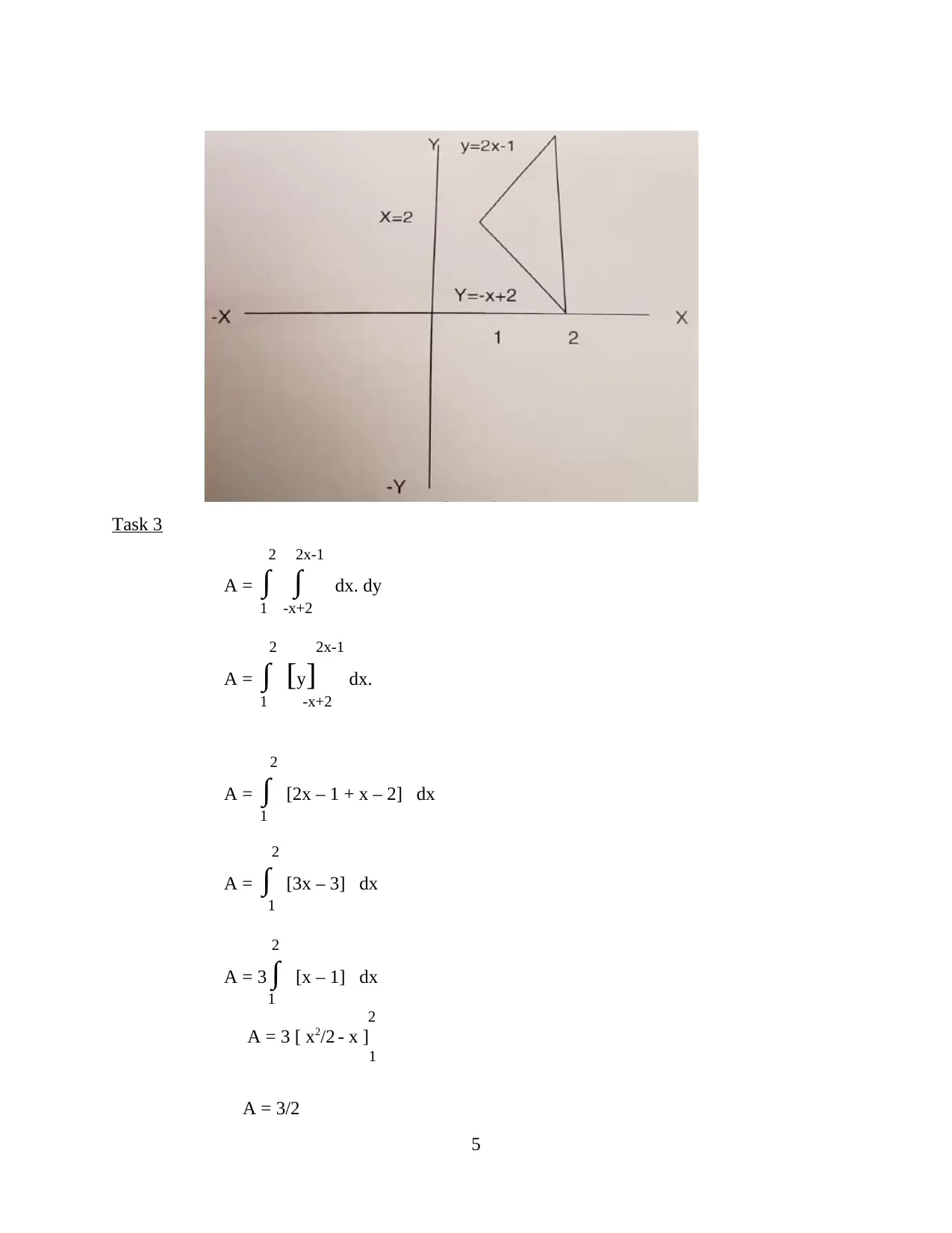
Task 3
2 2x-1
A = ∫ ∫ dx. dy
1 -x+2
2 2x-1
A = ∫ [y] dx.
1 -x+2
2
A = ∫ [2x – 1 + x – 2] dx
1
2
A = ∫ [3x – 3] dx
1
2
A = 3 ∫ [x – 1] dx
1
2
A = 3 [ x2/2 - x ]1
A = 3/2
5
2 2x-1
A = ∫ ∫ dx. dy
1 -x+2
2 2x-1
A = ∫ [y] dx.
1 -x+2
2
A = ∫ [2x – 1 + x – 2] dx
1
2
A = ∫ [3x – 3] dx
1
2
A = 3 ∫ [x – 1] dx
1
2
A = 3 [ x2/2 - x ]1
A = 3/2
5

2 2x-1
My = ∫ ∫ x. dy. dx
1 -x+2
2 2x-1
My = ∫ [x. y]. dx
1 -x+2
2
My = ∫ [x. (2x -1 + x – 2]. dx
1
2
My = 3. ∫ [x2 - x]. dx
1
or, 2
My = 3 [x3/3 – x2/2]1
= 5/6
6
My = ∫ ∫ x. dy. dx
1 -x+2
2 2x-1
My = ∫ [x. y]. dx
1 -x+2
2
My = ∫ [x. (2x -1 + x – 2]. dx
1
2
My = 3. ∫ [x2 - x]. dx
1
or, 2
My = 3 [x3/3 – x2/2]1
= 5/6
6
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

2 2x-1
Mx = ∫ ∫ y. dy. dx
1 -x+2
2 2x-1
Mx = ∫ [ y2/2]. dx
1 -x+2
2
Mx = ½ ∫ [ (2x-1)2 - (-x+2)2 ]. dx
1
2
Mx = 3/2 ∫ [x2 - 1]. dx
1
or, 2
Mx = 3/2 [x3/3 – x]1
= 2
7
Mx = ∫ ∫ y. dy. dx
1 -x+2
2 2x-1
Mx = ∫ [ y2/2]. dx
1 -x+2
2
Mx = ½ ∫ [ (2x-1)2 - (-x+2)2 ]. dx
1
2
Mx = 3/2 ∫ [x2 - 1]. dx
1
or, 2
Mx = 3/2 [x3/3 – x]1
= 2
7
Task 1
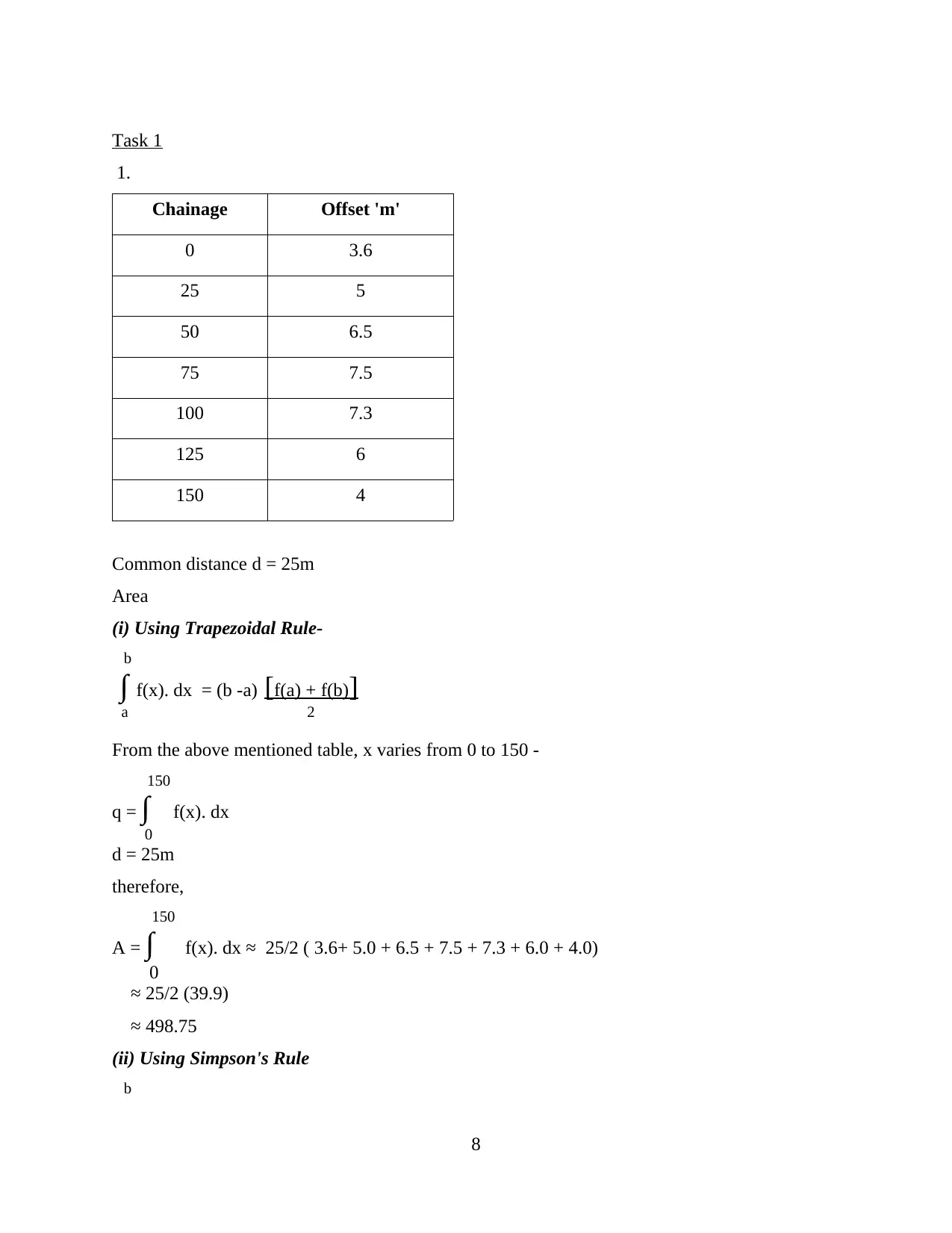
1.
Chainage Offset 'm'
0 3.6
25 5
50 6.5
75 7.5
100 7.3
125 6
150 4
Common distance d = 25m
Area
(i) Using Trapezoidal Rule-
b
∫ f(x). dx = (b -a) [f(a) + f(b)]
a 2
From the above mentioned table, x varies from 0 to 150 -
150
q = ∫ f(x). dx
0
d = 25m
therefore,
150
A = ∫ f(x). dx ≈ 25/2 ( 3.6+ 5.0 + 6.5 + 7.5 + 7.3 + 6.0 + 4.0)
0
≈ 25/2 (39.9)
≈ 498.75
(ii) Using Simpson's Rule
b
8
1.
Chainage Offset 'm'
0 3.6
25 5
50 6.5
75 7.5
100 7.3
125 6
150 4
Common distance d = 25m
Area
(i) Using Trapezoidal Rule-
b
∫ f(x). dx = (b -a) [f(a) + f(b)]
a 2
From the above mentioned table, x varies from 0 to 150 -
150
q = ∫ f(x). dx
0
d = 25m
therefore,
150
A = ∫ f(x). dx ≈ 25/2 ( 3.6+ 5.0 + 6.5 + 7.5 + 7.3 + 6.0 + 4.0)
0
≈ 25/2 (39.9)
≈ 498.75
(ii) Using Simpson's Rule
b
8

∫f(x). dx = (b -a) [(first observation + last observation) + 4 (sum of even observation) + 2 (sum of others)]
a 3
therefore,
150
A = ∫ f(x). dx
0
A ≈ (25/3) [ (3.6+4.0) + 2 (5.0+7.5+6.0) + 4 (6.5 + 7.3) ]
≈ (25/3) [ (7.6) + 2 (18.5) + 4 (13.8) ]
≈ (25/3) [ (3.6+4.0) + 2 (5.0+7.5+6.0) + 4 (6.5 + 7.3) ]
≈ 831.66
2.
(a) 2 log9 (√x) - log9 (6x – 1) = 0
using logarithmic rules i.e. log (a/b) = log a – log b
so,
2 log9 (√x / (6x- 1) = 0
√x / (6x- 1) = 0
√x = (6x- 1)
x = (6x – 1)2
or, x = 36 x2 – 12x + 1
or, 36 x2 – 13x + 1 = 0
or, (9x – 1) (4x + 1) = 0
or, x = 1/9 ; -1/4
3 Determinant of matrix
1 2 3
0 -4 1
0 3 -1
Solution Using determinant formula of 3 x 3 matrix as
A = a11 a12 a13
9
a 3
therefore,
150
A = ∫ f(x). dx
0
A ≈ (25/3) [ (3.6+4.0) + 2 (5.0+7.5+6.0) + 4 (6.5 + 7.3) ]
≈ (25/3) [ (7.6) + 2 (18.5) + 4 (13.8) ]
≈ (25/3) [ (3.6+4.0) + 2 (5.0+7.5+6.0) + 4 (6.5 + 7.3) ]
≈ 831.66
2.
(a) 2 log9 (√x) - log9 (6x – 1) = 0
using logarithmic rules i.e. log (a/b) = log a – log b
so,
2 log9 (√x / (6x- 1) = 0
√x / (6x- 1) = 0
√x = (6x- 1)
x = (6x – 1)2
or, x = 36 x2 – 12x + 1
or, 36 x2 – 13x + 1 = 0
or, (9x – 1) (4x + 1) = 0
or, x = 1/9 ; -1/4
3 Determinant of matrix
1 2 3
0 -4 1
0 3 -1
Solution Using determinant formula of 3 x 3 matrix as
A = a11 a12 a13
9
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

a21 a22 a23
a31 a31 a33
then, determinant of any 3x3 matrix can be calculated by using the given formula
A = a11 ( a22 x a33 – a23 x a31) – a12 ( a21 x a33 – a23 x a31) +a13 (a21 x a31 - a22 x a31)
Using this formula, to find determinant of given matrix is given by can be calculated as
1 2 3
A = 0 -4 1
0 3 -1
then, determinant of A = [ 1 ( -4 x -1 – 1 x3) - 2 ( 0 x -1 – 1 x 0 ) + 3 (0 x 3 – 1 x 0) ]
= [ 1 (4 – 3) – 2 ( 0 – 0) + 3 ( 0 – 0 )]
= 1
10
a31 a31 a33
then, determinant of any 3x3 matrix can be calculated by using the given formula
A = a11 ( a22 x a33 – a23 x a31) – a12 ( a21 x a33 – a23 x a31) +a13 (a21 x a31 - a22 x a31)
Using this formula, to find determinant of given matrix is given by can be calculated as
1 2 3
A = 0 -4 1
0 3 -1
then, determinant of A = [ 1 ( -4 x -1 – 1 x3) - 2 ( 0 x -1 – 1 x 0 ) + 3 (0 x 3 – 1 x 0) ]
= [ 1 (4 – 3) – 2 ( 0 – 0) + 3 ( 0 – 0 )]
= 1
10
Lo3
Task 1
Task 2
Scenario 1
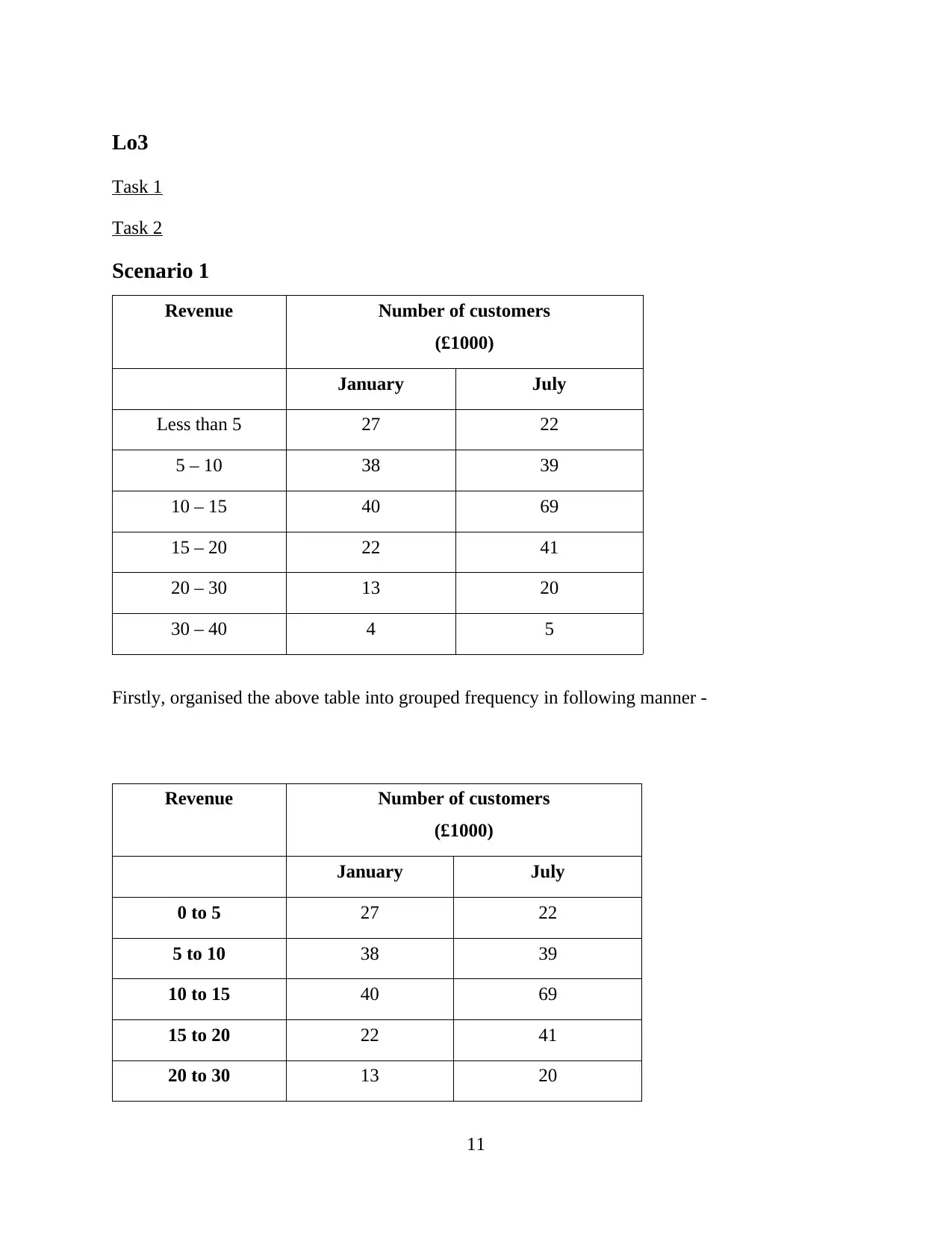
Revenue Number of customers
(£1000)
January July
Less than 5 27 22
5 – 10 38 39
10 – 15 40 69
15 – 20 22 41
20 – 30 13 20
30 – 40 4 5
Firstly, organised the above table into grouped frequency in following manner -
Revenue Number of customers
(£1000)
January July
0 to 5 27 22
5 to 10 38 39
10 to 15 40 69
15 to 20 22 41
20 to 30 13 20
11
Task 1
Task 2
Scenario 1
Revenue Number of customers
(£1000)
January July
Less than 5 27 22
5 – 10 38 39
10 – 15 40 69
15 – 20 22 41
20 – 30 13 20
30 – 40 4 5
Firstly, organised the above table into grouped frequency in following manner -
Revenue Number of customers
(£1000)
January July
0 to 5 27 22
5 to 10 38 39
10 to 15 40 69
15 to 20 22 41
20 to 30 13 20
11
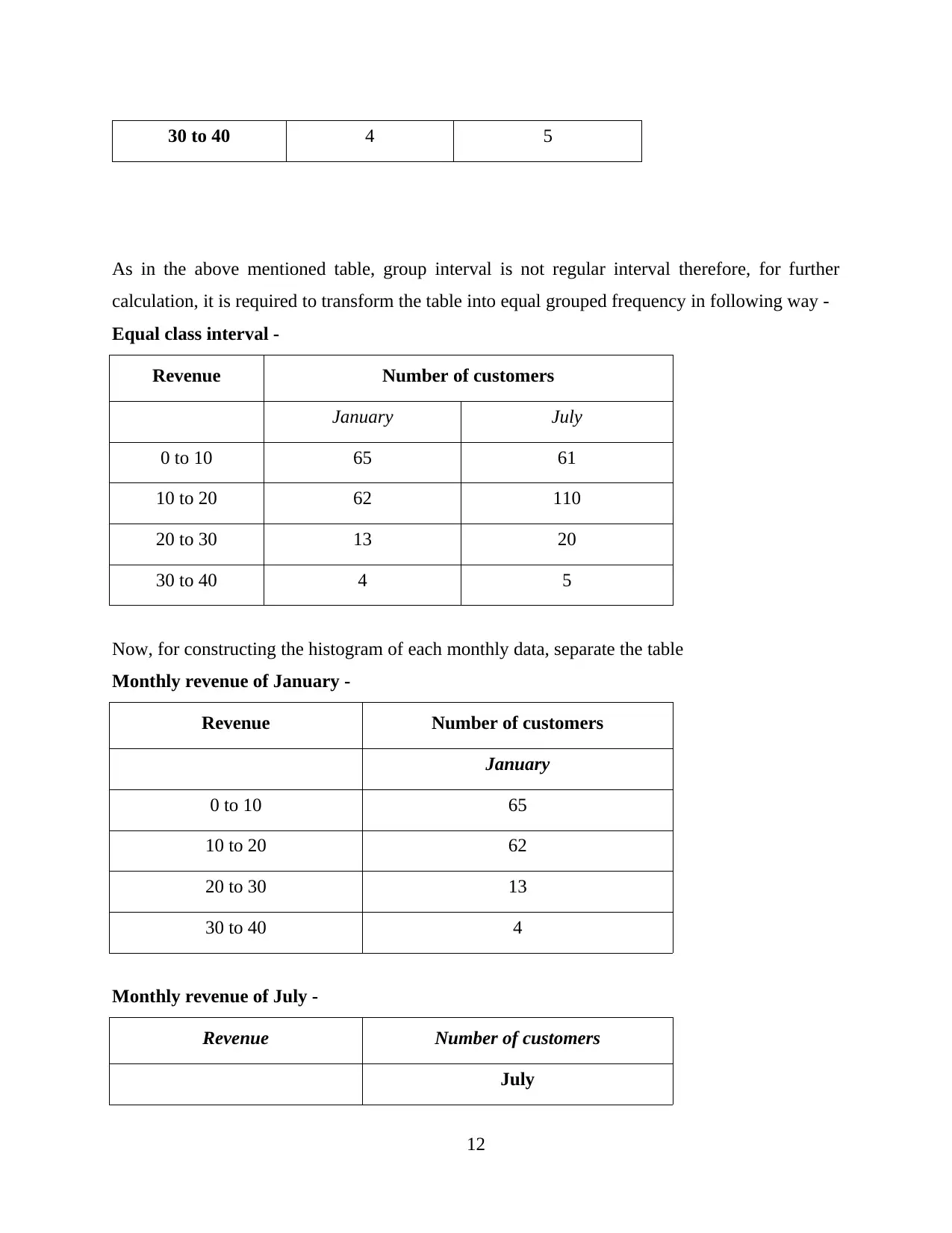
30 to 40 4 5
As in the above mentioned table, group interval is not regular interval therefore, for further
calculation, it is required to transform the table into equal grouped frequency in following way -
Equal class interval -
Revenue Number of customers
January July
0 to 10 65 61
10 to 20 62 110
20 to 30 13 20
30 to 40 4 5
Now, for constructing the histogram of each monthly data, separate the table
Monthly revenue of January -
Revenue Number of customers
January
0 to 10 65
10 to 20 62
20 to 30 13
30 to 40 4
Monthly revenue of July -
Revenue Number of customers
July
12
As in the above mentioned table, group interval is not regular interval therefore, for further
calculation, it is required to transform the table into equal grouped frequency in following way -
Equal class interval -
Revenue Number of customers
January July
0 to 10 65 61
10 to 20 62 110
20 to 30 13 20
30 to 40 4 5
Now, for constructing the histogram of each monthly data, separate the table
Monthly revenue of January -
Revenue Number of customers
January
0 to 10 65
10 to 20 62
20 to 30 13
30 to 40 4
Monthly revenue of July -
Revenue Number of customers
July
12
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
0 to 10 61
10 to 20 110
20 to 30 20
30 to 40 5
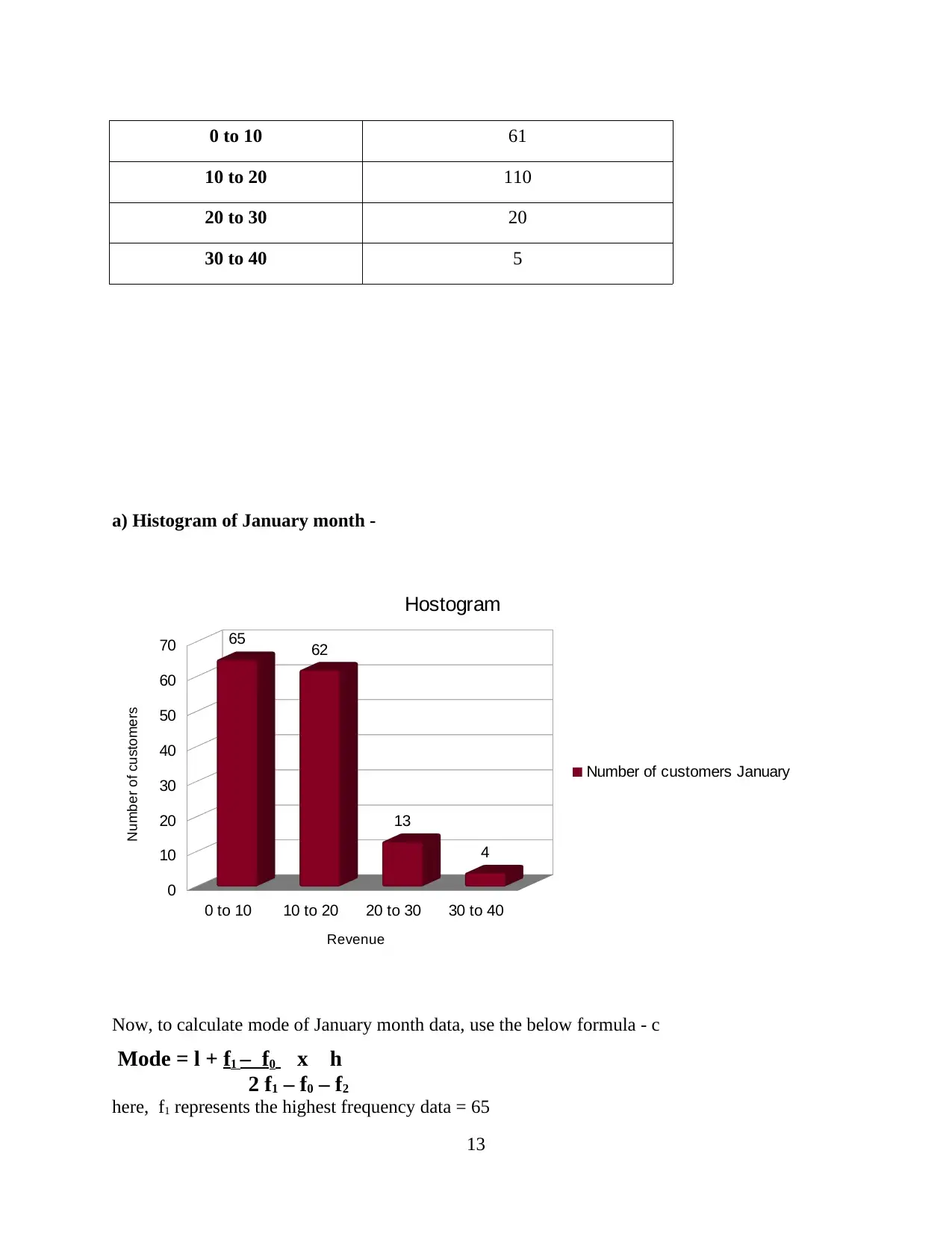
a) Histogram of January month -
Now, to calculate mode of January month data, use the below formula - c
Mode = l + f1 – f0 x h
2 f1 – f0 – f2
here, f1 represents the highest frequency data = 65
13
0 to 10 10 to 20 20 to 30 30 to 40
0
10
20
30
40
50
60
70 65 62
13
4
Hostogram
Number of customers January
Revenue
Number of customers
10 to 20 110
20 to 30 20
30 to 40 5
a) Histogram of January month -
Now, to calculate mode of January month data, use the below formula - c
Mode = l + f1 – f0 x h
2 f1 – f0 – f2
here, f1 represents the highest frequency data = 65
13
0 to 10 10 to 20 20 to 30 30 to 40
0
10
20
30
40
50
60
70 65 62
13
4
Hostogram
Number of customers January
Revenue
Number of customers
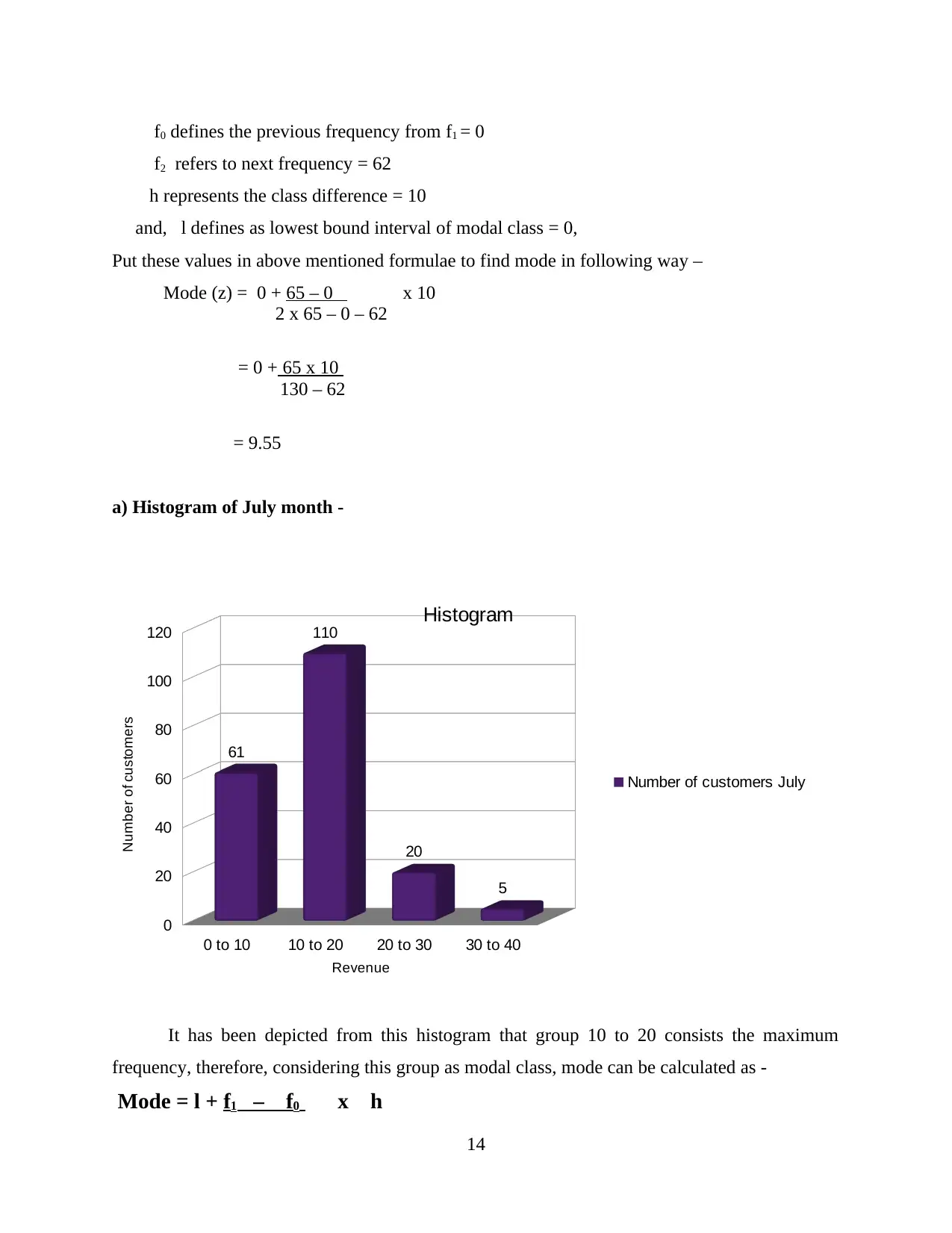
f0 defines the previous frequency from f1 = 0
f2 refers to next frequency = 62
h represents the class difference = 10
and, l defines as lowest bound interval of modal class = 0,
Put these values in above mentioned formulae to find mode in following way –
Mode (z) = 0 + 65 – 0 x 10
2 x 65 – 0 – 62
= 0 + 65 x 10
130 – 62
= 9.55
a) Histogram of July month -
It has been depicted from this histogram that group 10 to 20 consists the maximum
frequency, therefore, considering this group as modal class, mode can be calculated as -
Mode = l + f1 – f0 x h
14
0 to 10 10 to 20 20 to 30 30 to 40
0
20
40
60
80
100
120
61
110
20
5
Histogram
Number of customers July
Revenue
Number of customers
f2 refers to next frequency = 62
h represents the class difference = 10
and, l defines as lowest bound interval of modal class = 0,
Put these values in above mentioned formulae to find mode in following way –
Mode (z) = 0 + 65 – 0 x 10
2 x 65 – 0 – 62
= 0 + 65 x 10
130 – 62
= 9.55
a) Histogram of July month -
It has been depicted from this histogram that group 10 to 20 consists the maximum
frequency, therefore, considering this group as modal class, mode can be calculated as -
Mode = l + f1 – f0 x h
14
0 to 10 10 to 20 20 to 30 30 to 40
0
20
40
60
80
100
120
61
110
20
5
Histogram
Number of customers July
Revenue
Number of customers

2 f1 – f0 - f2
here, f1 = 110, f0 = 61, f2 = 20, h = 10 and l = 10,
then,
Mode (z) = 10 + 110 – 61 x 10
2x110 – 61 – 20
= 10 + 49 x 10
220 – 81
= 13.5
15
here, f1 = 110, f0 = 61, f2 = 20, h = 10 and l = 10,
then,
Mode (z) = 10 + 110 – 61 x 10
2x110 – 61 – 20
= 10 + 49 x 10
220 – 81
= 13.5
15
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
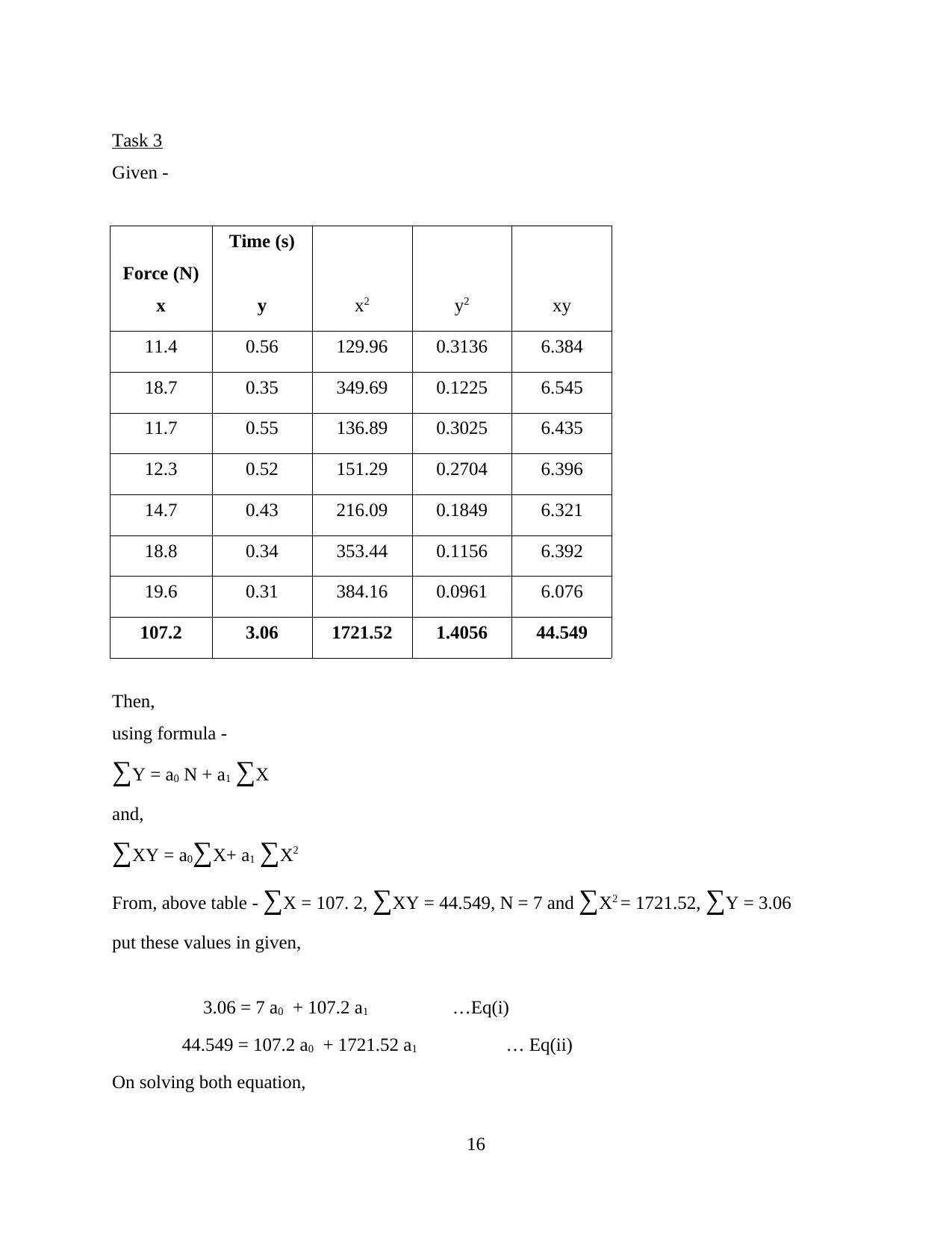
Task 3
Given -
Force (N)
x
Time (s)
y x2 y2 xy
11.4 0.56 129.96 0.3136 6.384
18.7 0.35 349.69 0.1225 6.545
11.7 0.55 136.89 0.3025 6.435
12.3 0.52 151.29 0.2704 6.396
14.7 0.43 216.09 0.1849 6.321
18.8 0.34 353.44 0.1156 6.392
19.6 0.31 384.16 0.0961 6.076
107.2 3.06 1721.52 1.4056 44.549
Then,
using formula -
∑Y = a0 N + a1 ∑X
and,
∑XY = a0∑X+ a1 ∑X2
From, above table - ∑X = 107. 2, ∑XY = 44.549, N = 7 and ∑X2 = 1721.52, ∑Y = 3.06
put these values in given,
3.06 = 7 a0 + 107.2 a1 …Eq(i)
44.549 = 107.2 a0 + 1721.52 a1 … Eq(ii)
On solving both equation,
16
Given -
Force (N)
x
Time (s)
y x2 y2 xy
11.4 0.56 129.96 0.3136 6.384
18.7 0.35 349.69 0.1225 6.545
11.7 0.55 136.89 0.3025 6.435
12.3 0.52 151.29 0.2704 6.396
14.7 0.43 216.09 0.1849 6.321
18.8 0.34 353.44 0.1156 6.392
19.6 0.31 384.16 0.0961 6.076
107.2 3.06 1721.52 1.4056 44.549
Then,
using formula -
∑Y = a0 N + a1 ∑X
and,
∑XY = a0∑X+ a1 ∑X2
From, above table - ∑X = 107. 2, ∑XY = 44.549, N = 7 and ∑X2 = 1721.52, ∑Y = 3.06
put these values in given,
3.06 = 7 a0 + 107.2 a1 …Eq(i)
44.549 = 107.2 a0 + 1721.52 a1 … Eq(ii)
On solving both equation,
16

a0 = 0.865 and a1 = - 0.028
then,
equation of regression line of time on force – Y = a0 + a1 X
so, Y = 0.865 – 0.028 X
Lo4
Task 1
Determine surface area of a line 350mm rotating around x axis at a distance of 440 mm using
pappus theorem
Task 2
M (x) = x3 - 3x2 - 4
Value of bending equation m, with the interval 0.5 from the range 1 to 4 can be determined by
Variable x Bending moment M
0.5 -4.63
1 -6
1.5 −2.85
2 -8
3.5 26.63
4 12
17
then,
equation of regression line of time on force – Y = a0 + a1 X
so, Y = 0.865 – 0.028 X
Lo4
Task 1
Determine surface area of a line 350mm rotating around x axis at a distance of 440 mm using
pappus theorem
Task 2
M (x) = x3 - 3x2 - 4
Value of bending equation m, with the interval 0.5 from the range 1 to 4 can be determined by
Variable x Bending moment M
0.5 -4.63
1 -6
1.5 −2.85
2 -8
3.5 26.63
4 12
17
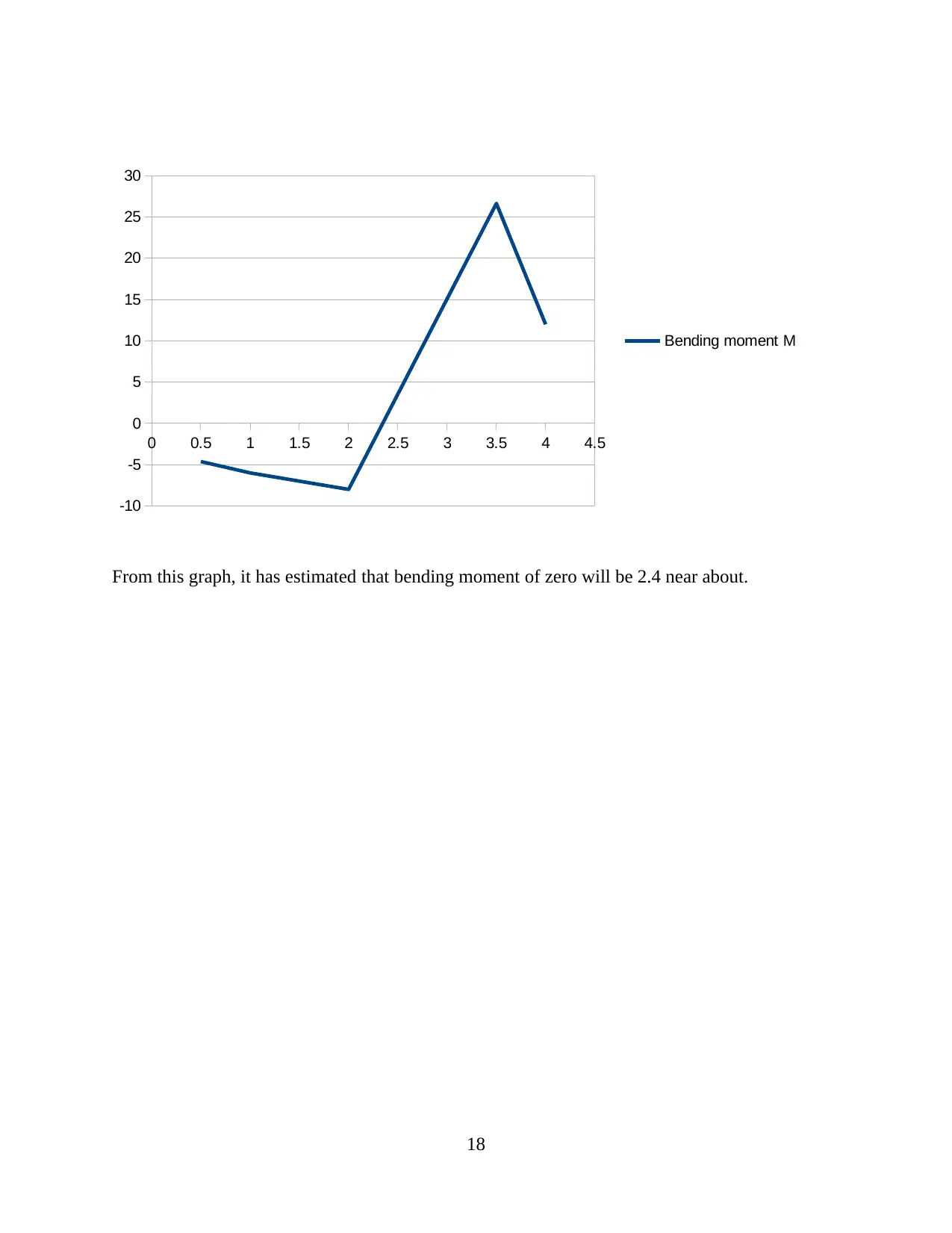
From this graph, it has estimated that bending moment of zero will be 2.4 near about.
18
0 0.5 1 1.5 2 2.5 3 3.5 4 4.5
-10
-5
0
5
10
15
20
25
30
Bending moment M
18
0 0.5 1 1.5 2 2.5 3 3.5 4 4.5
-10
-5
0
5
10
15
20
25
30
Bending moment M
1 out of 19
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.





