Maths Integration Homework: Calculus Problems and Solutions (2023)

This document provides a detailed solution to a maths integration assignment. The assignment covers a range of integration techniques including the trapezium rule, finding general expressions for integrals, evaluating definite integrals, and integration by parts and substitution. The solution includes step-by-step workings for each problem, including finding areas, volumes of revolution, and applying various integration methods. The assignment also includes problems involving algebraic manipulation and the use of factors to solve equations. The solutions are presented in a clear and concise manner, making it a valuable resource for students studying integration.

Maths integration

Contents
Contents...........................................................................................................................................2
MAIN BODY..................................................................................................................................1

MAIN BODY
1. The values of 𝑦, for a curve with equation 𝑦=𝑓(𝑥),have been tabulated below.
x 1 2.25 3.5 4.75 6
y 9 17 25 21 13
Use the trapezium rule with all the values from the above table to find an estimate
for the integral.
Solution: As per the formula which is as follows:
Area= 1/2 h[y0+2(y1+y2+_____+yn-1)+yn]
Where, h= b-a/n
Here we have, h= 6-1/4 = 5/4
Putting in the values we have,
=1/2 (5/4) [9+2(17+25+21)+13]
=5/8*148
=185/2
=92.5
2. Find a general expression for 𝑦 given that

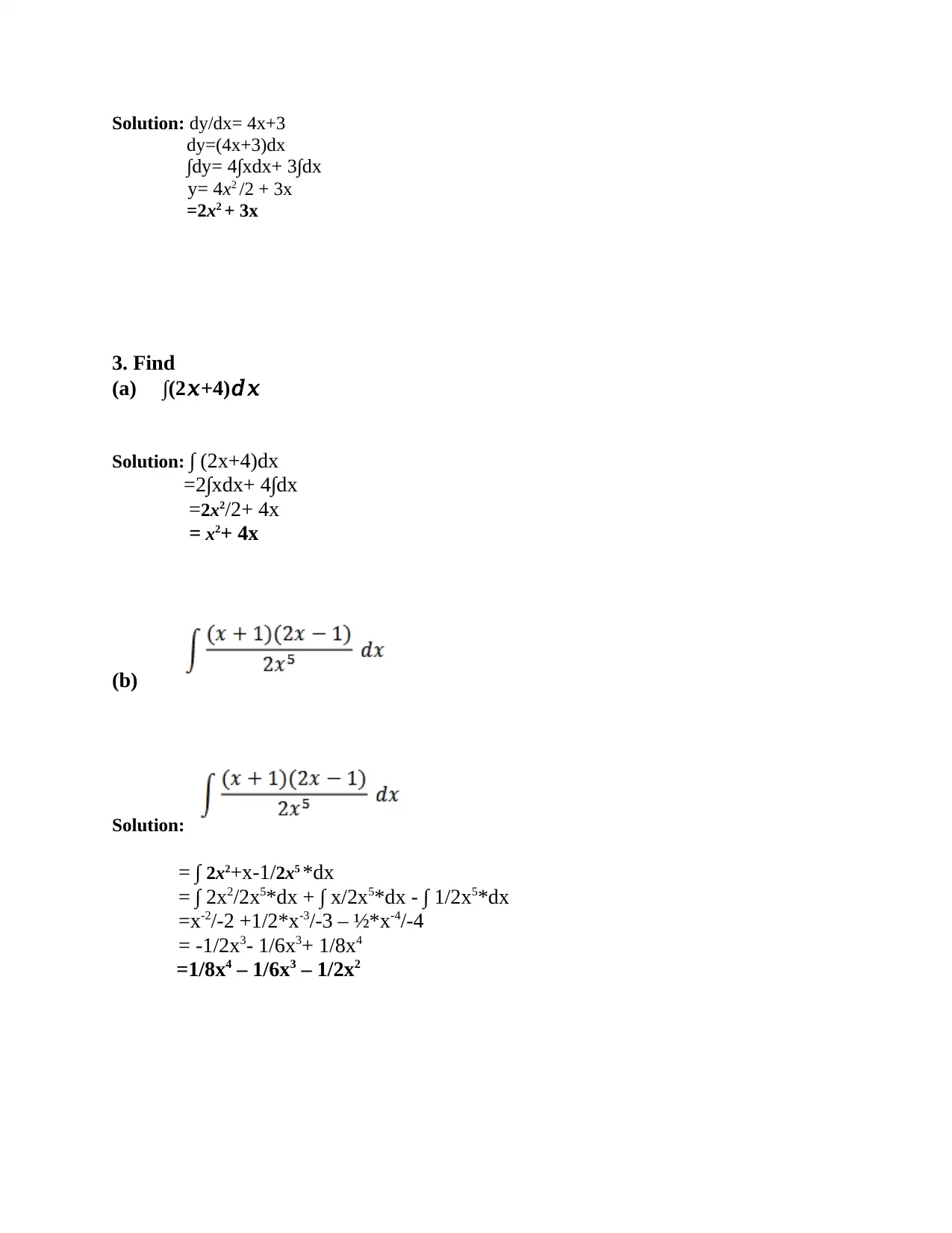
Solution: dy/dx= 4x+3
dy=(4x+3)dx
∫dy= 4∫xdx+ 3∫dx
y= 4x2 /2 + 3x
=2x2 + 3x
3. Find
(a) ∫(2𝑥+4)𝑑𝑥
Solution: ∫ (2x+4)dx
=2∫xdx+ 4∫dx
=2x2/2+ 4x
= x2+ 4x
(b)
Solution:
= ∫ 2x2+x-1/2x5 *dx
= ∫ 2x2/2x5*dx + ∫ x/2x5*dx - ∫ 1/2x5*dx
=x-2/-2 +1/2*x-3/-3 – ½*x-4/-4
= -1/2x3- 1/6x3+ 1/8x4
=1/8x4 – 1/6x3 – 1/2x2

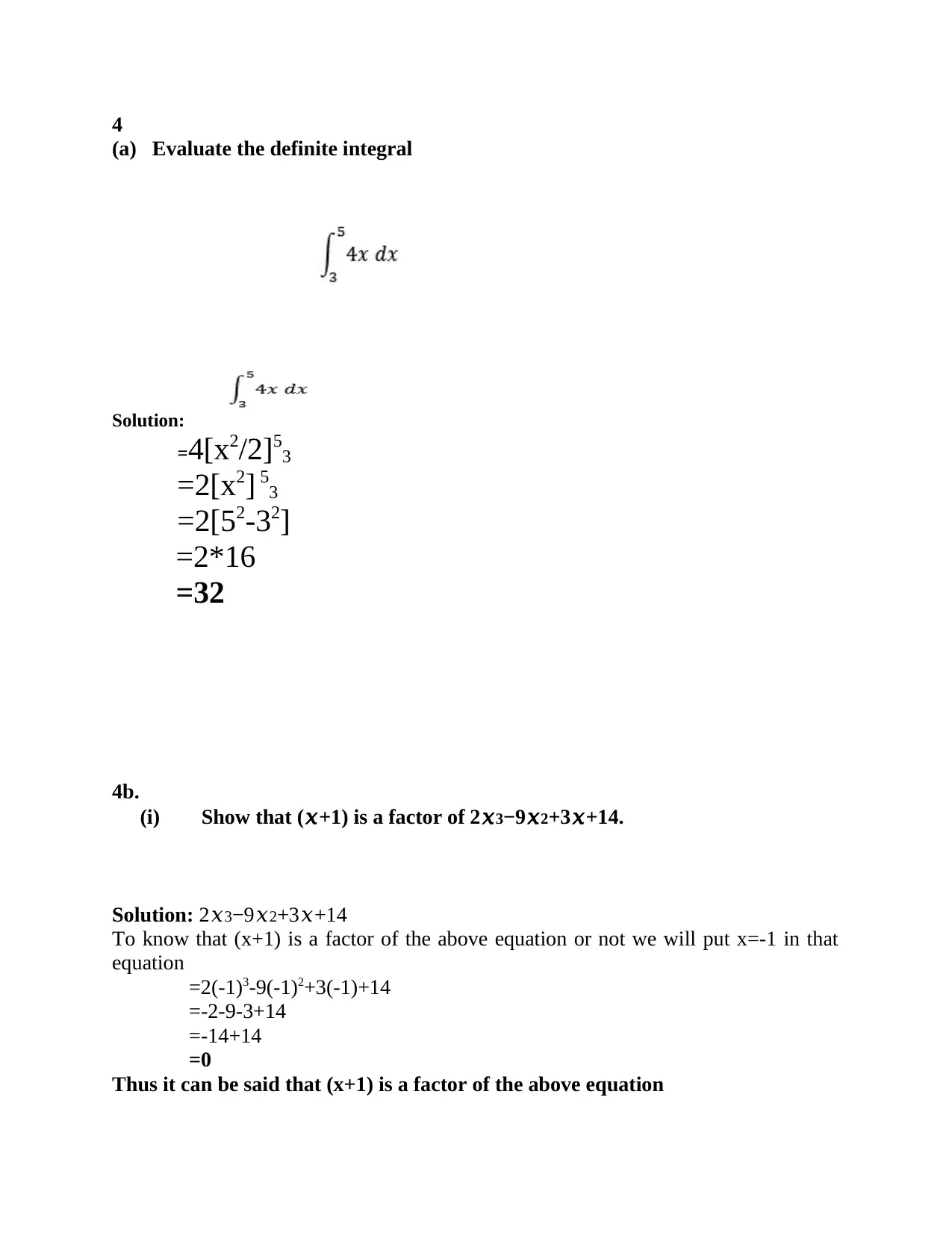
4
(a) Evaluate the definite integral
Solution:
=4[x2/2]53
=2[x2] 53
=2[52-32]
=2*16
=32
4b.
(i) Show that (𝑥+1) is a factor of 2𝑥3−9𝑥2+3𝑥+14.
Solution: 2𝑥3−9𝑥2+3𝑥+14
To know that (x+1) is a factor of the above equation or not we will put x=-1 in that
equation
=2(-1)3-9(-1)2+3(-1)+14
=-2-9-3+14
=-14+14
=0
Thus it can be said that (x+1) is a factor of the above equation

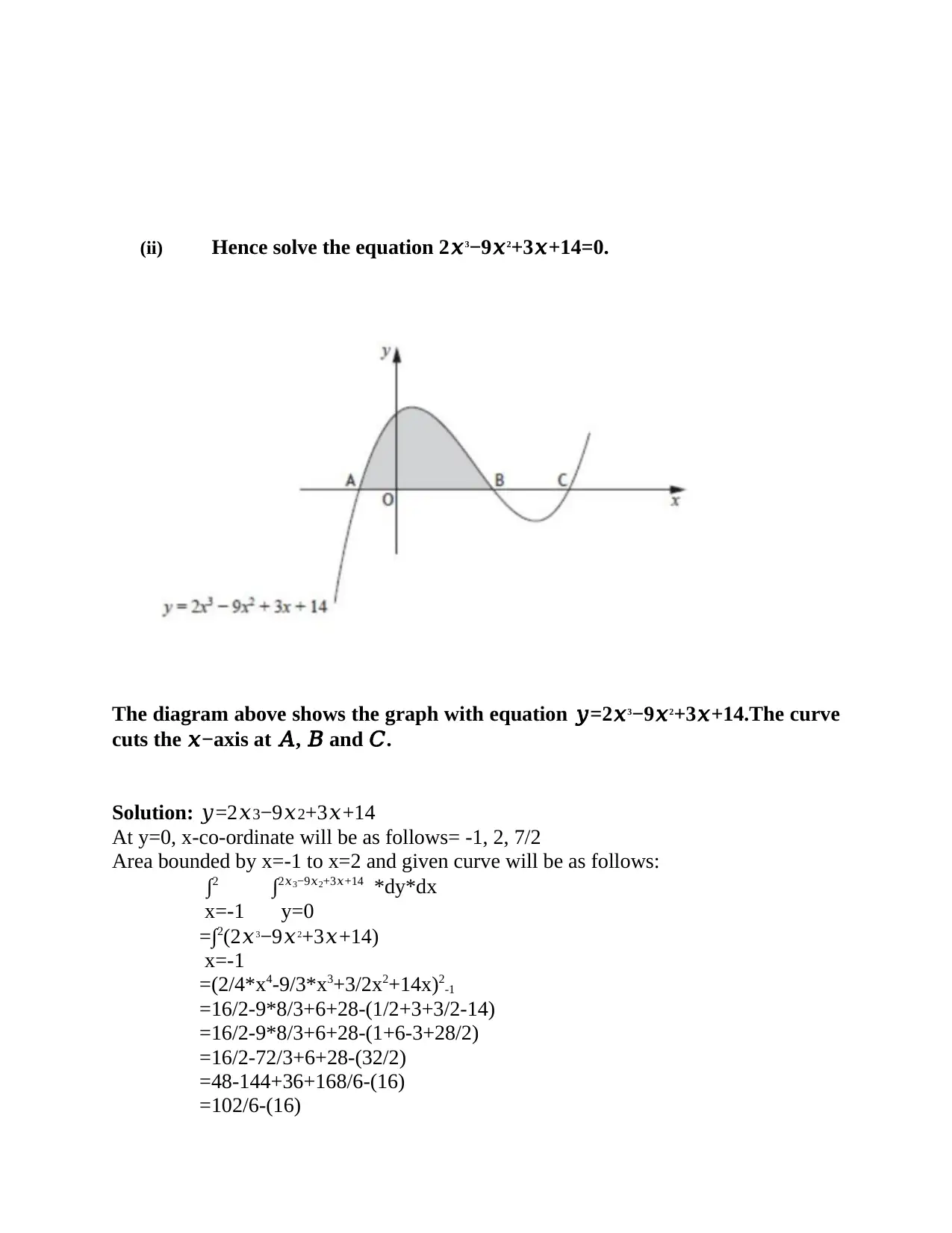
(ii) Hence solve the equation 2𝑥3−9𝑥2+3𝑥+14=0.
The diagram above shows the graph with equation 𝑦=2𝑥3−9𝑥2+3𝑥+14.The curve
cuts the 𝑥−axis at 𝐴, 𝐵 and 𝐶.
Solution: 𝑦=2𝑥3−9𝑥2+3𝑥+14
At y=0, x-co-ordinate will be as follows= -1, 2, 7/2
Area bounded by x=-1 to x=2 and given curve will be as follows:
∫2 ∫2𝑥3−9𝑥2+3𝑥+14 *dy*dx
x=-1 y=0
=∫2(2𝑥3−9𝑥2+3𝑥+14)
x=-1
=(2/4*x4-9/3*x3+3/2x2+14x)2-1
=16/2-9*8/3+6+28-(1/2+3+3/2-14)
=16/2-9*8/3+6+28-(1+6-3+28/2)
=16/2-72/3+6+28-(32/2)
=48-144+36+168/6-(16)
=102/6-(16)

=17-16
=1
(iii) Write down the coordinates of the points 𝐴and 𝐵.
Solution: y=2x3-9x2+3x+14
y=0 for the roots of the equation
(x-2) 2x3-9x2+3x+14 2x2-5x-7
-2x3-4x2
+
0-5x2+3x+14
-5x2+10x
+ -
0-7x+14
-7x+14
+ -
0 0
By using hit and trial method:
Putting x=2
y=2(8)-9(4)+3(2)+14
=16-36+6+14
=0
Hence it can be said from the above that x=2 and (x-2) is one root!
Thus 0=(2x2-5x-7)(x-2)
2x2-5x-7=0
The calculation of root is shown below:

=+5± 25-4(2)(-7)
2(2)
=5±9/4
=(x=3.5) and (x=-1)
So, (x-3.5) (x+1)
Thus, the co-ordinates are as follows:
A=(-1,0)
B=(2,0)
C=(3.5,0)
(iv) Hence calculate the shaded area in the diagram.
Solution: -1∫2 (2𝑥3−9𝑥2+3𝑥+14)
=2 2-1[x4/4] -9 2-1[x3/3] +3 2-1[x2/2] +14 2-1[x]
=2[16-1/4] -9[8+1/3]+3[4-1/2]+14[2+1]
=15/2-27+9-2+42
=12+42-27
Area of the shaded region= 27
5a. Find the volume of the solid formed when the area between the line
𝑦=𝑥and the 𝑥−axis from 𝑥=0and 𝑥=2, perform one revolution about the 𝑥−axis.
Solution: Volume of the solid revolved around x-axis

In the above figure x=2 and y=x can be seen
=n1∫n2 π y2 dx
= π ∫2 y2 dx
n=0
= π 0∫2 x2 dx (because y=x)
= π x3/3 0∫2
=8 π/3

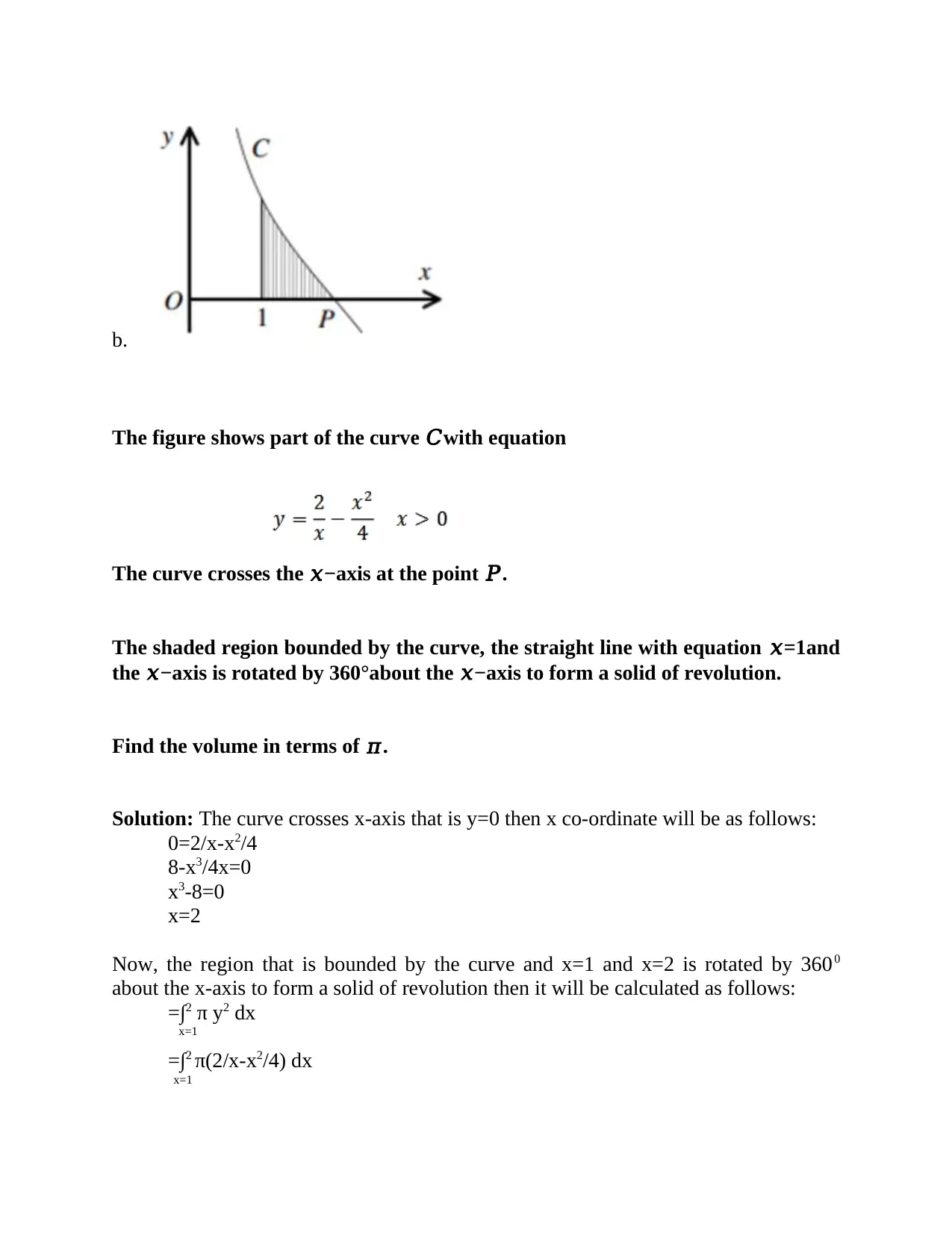
b.
The figure shows part of the curve 𝐶with equation
The curve crosses the 𝑥−axis at the point 𝑃.
The shaded region bounded by the curve, the straight line with equation 𝑥=1and
the 𝑥−axis is rotated by 360°about the 𝑥−axis to form a solid of revolution.
Find the volume in terms of 𝜋.
Solution: The curve crosses x-axis that is y=0 then x co-ordinate will be as follows:
0=2/x-x2/4
8-x3/4x=0
x3-8=0
x=2
Now, the region that is bounded by the curve and x=1 and x=2 is rotated by 3600
about the x-axis to form a solid of revolution then it will be calculated as follows:
=∫2 π y2 dx
x=1
=∫2 π(2/x-x2/4) dx
x=1

=∫2 π(4/x2+x4/16-4x/4) dx
x=1
= π(4(-2)/x3+x5/5*16-x2/2)21
= π(-8/8+32/5*16-2-[-8+1/5*16-1/2])
= π(-1+2/5-2+8-1/80+1/2)
= π(-80+32+160+640-1+40/80)
= π(791/80)
Volume= 791 π/80
6. Using integration by inspection find
Solution:
x2-1=t
2xdx=dt
xdx=dt/2
=∫ 1/t3*dt
=(t-3+1/-3+1)+c
=t-2/-2
=1/-2(x2-1)2+c

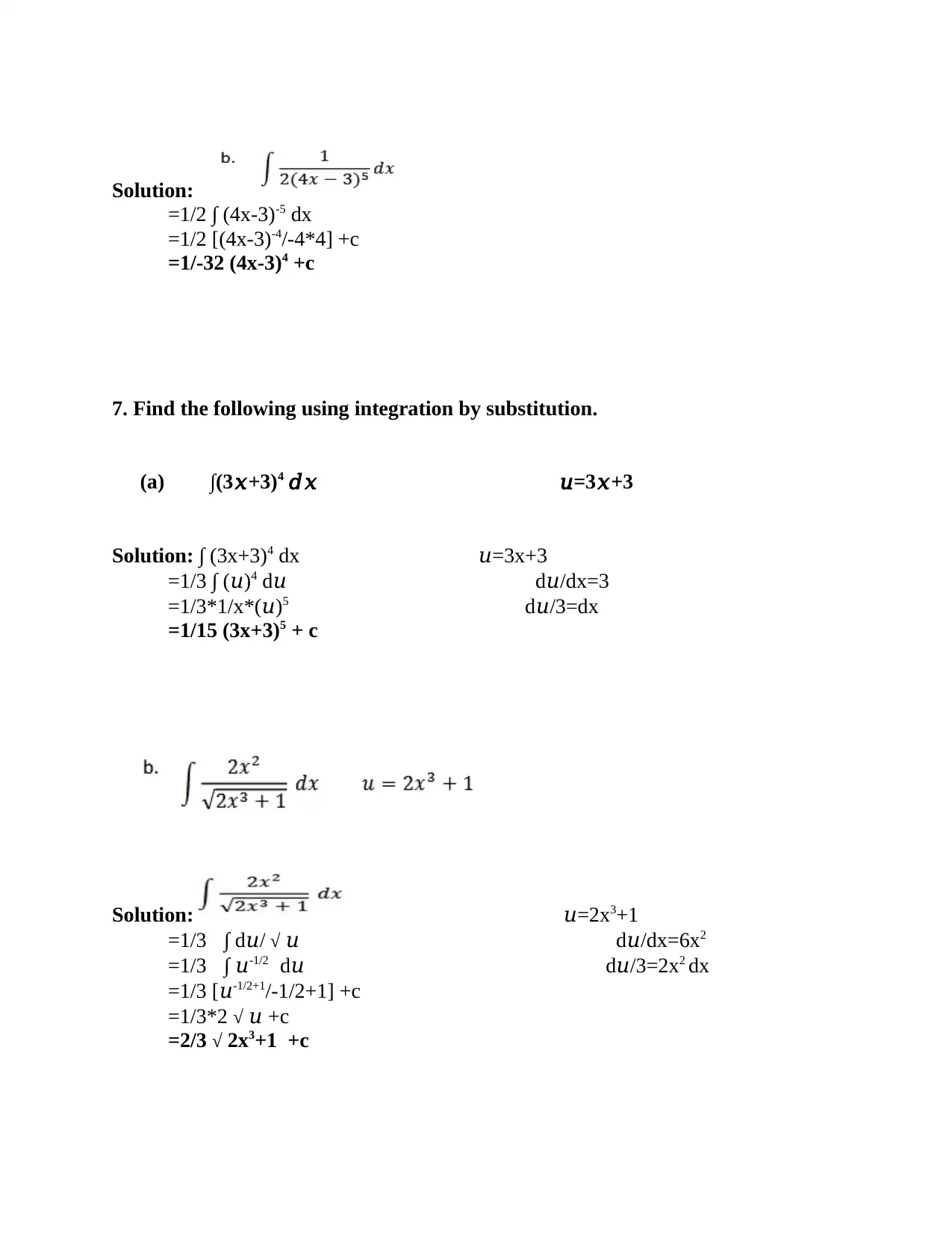
Solution:
=1/2 ∫ (4x-3)-5 dx
=1/2 [(4x-3)-4/-4*4] +c
=1/-32 (4x-3)4 +c
7. Find the following using integration by substitution.
(a) ∫(3𝑥+3)4 𝑑𝑥 𝑢=3𝑥+3
Solution: ∫ (3x+3)4 dx 𝑢=3x+3
=1/3 ∫ (𝑢)4 d𝑢 d𝑢/dx=3
=1/3*1/x*(𝑢)5 d𝑢/3=dx
=1/15 (3x+3)5 + c
Solution: 𝑢=2x3+1
=1/3 ∫ d𝑢/ √ 𝑢 d𝑢/dx=6x2
=1/3 ∫ 𝑢-1/2 d𝑢 d𝑢/3=2x2 dx
=1/3 [𝑢-1/2+1/-1/2+1] +c
=1/3*2 √ 𝑢 +c
=2/3 √ 2x3+1 +c

8. Carry out the following integrations using integration by parts.
(a) ∫ 1/2𝑥𝑒4𝑥 𝑑𝑥
Solution: ∫1/2𝑥𝑒4𝑥𝑑𝑥
=x∫1/2𝑒4𝑥𝑑𝑥-∫(dx/dx ∫𝑒4𝑥𝑑𝑥) dx+c
=xe4x/4-∫ 1*e4x/4*dx +c
= xe4x/4- e4x/16 +c
(b) ∫ 5𝑥 sin 4𝑥 𝑑𝑥
Solution: ∫ 5𝑥 sin 4𝑥 𝑑𝑥
=5(x ∫ sin 4x dx- ∫ dx/dx ∫ sin4x dx)
=5(x [-cos 4x]/4 - ∫ [-cos 4x]/4 dx +c)
=-5/4 x cos 4x + sin 4x /16 +c
(c) ∫ ln 𝑥/𝑥3*𝑑𝑥
Solution: ∫ ln 𝑥/𝑥3*𝑑𝑥
= ln x ∫ x3 dx - ∫ 1/x*x4/4*dx+c
= ln x (x4/4-x4/16 +c)
= x4/4 (ln x -1/4) +c

(d) ∫𝑥2𝑐𝑜𝑠1/3𝑥 𝑑𝑥
Solution: ∫𝑥2𝑐𝑜𝑠1/3𝑥 𝑑𝑥
=x2 ∫ cos x/3 dx- ∫ (d/dn* x2 ∫ cos x/3) dx
=x2 3 sin x/3 - ∫ 6 x sin x/3 dx
=3x2 sin x/3 -6 (3x [-cosx/3]- ∫ -3 cosx/3 dx)
=3 x2 sin x/3 +18 x cos x/3 -9 sin x/3 +c