Solved Maths Assignments and Study Material

Find a wide range of solved Maths assignments and study material on Desklib. Get help with your Maths homework and improve your grades.

MATHS
STUDENT ID:
[Pick the date]

Question 1
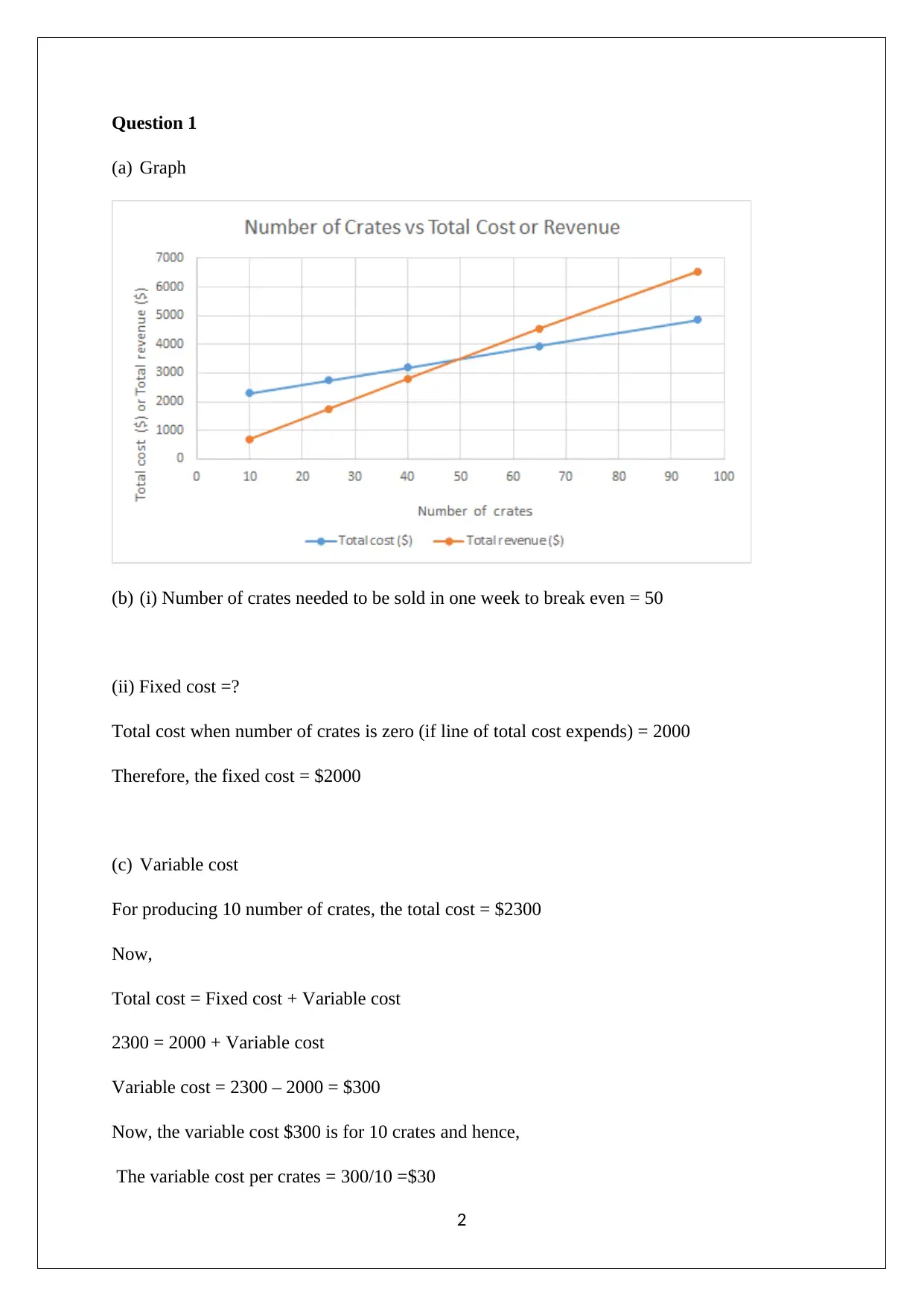
(a) Graph
(b) (i) Number of crates needed to be sold in one week to break even = 50
(ii) Fixed cost =?
Total cost when number of crates is zero (if line of total cost expends) = 2000
Therefore, the fixed cost = $2000
(c) Variable cost
For producing 10 number of crates, the total cost = $2300
Now,
Total cost = Fixed cost + Variable cost
2300 = 2000 + Variable cost
Variable cost = 2300 – 2000 = $300
Now, the variable cost $300 is for 10 crates and hence,
The variable cost per crates = 300/10 =$30
2

Question 2
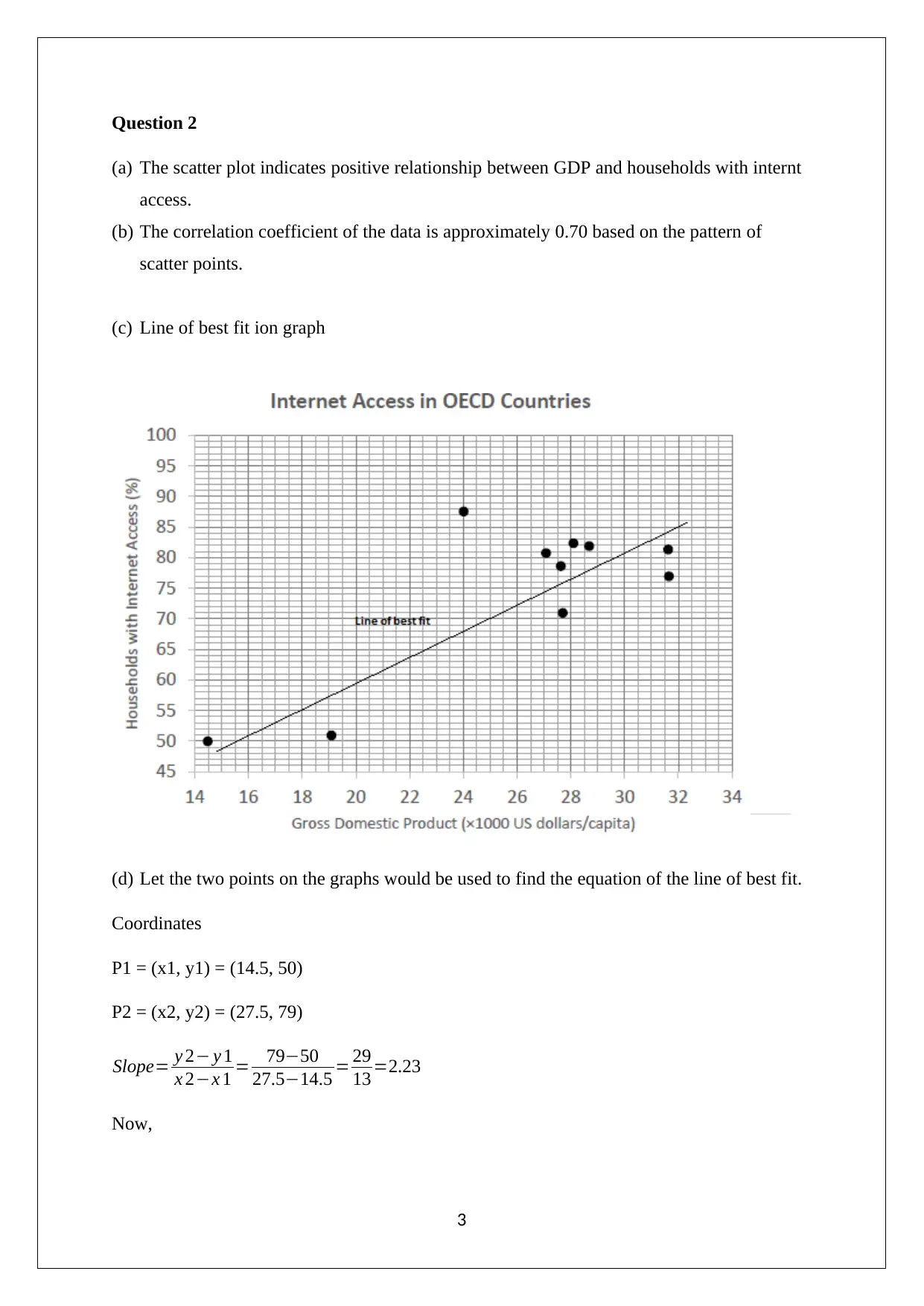
(a) The scatter plot indicates positive relationship between GDP and households with internt
access.
(b) The correlation coefficient of the data is approximately 0.70 based on the pattern of
scatter points.
(c) Line of best fit ion graph
(d) Let the two points on the graphs would be used to find the equation of the line of best fit.
Coordinates
P1 = (x1, y1) = (14.5, 50)
P2 = (x2, y2) = (27.5, 79)
Slope= y 2− y 1
x 2−x 1 = 79−50
27.5−14.5 = 29
13 =2.23
Now,
3

( y−50 )= 29
13 ( x−14.5 )
13 y−650=29 x−420.5
13 y=29 x−420.5+650
13 y=29 x +229.5
y=2.23 x +17.65
Households with internet access ( % ) =(2.23∗Grossdomestic product )+17.65
(e) Households with internet access (%) =?
Gross domestic product per capita = $3876 US
Equation
y=2.23 x +17.65
y= ( 2.23∗3876
1000 )+17.65=26.30 %
Therefore, the households with internet access (%) would be 27%.
(f) The above estimate would not be reliable considering that the GDP value used as the
independent variable does not lie in the range of GDP values which have been used for the
estimation of the linear regression lines.
Question 3
(a) y=2 ( 6 x +4 ) ( x −9 )
y=2{6 x2−¿54x +4x – 36}
y=2 ( 6 x2−50 x−36 )
y=12 x2−100 x−72
4

(b) Null factor law
y=2 ( 6 x +4 ) ( x −9 )
Let,
A = 6x+4
B = x-9
Now,
Put A = 0 (Null factor law)
6 x +4=0
x=−4
6 =−2
3
Now,
Put B = 0(Null factor law)
x−9=0
x=9
Thus, x = -2/3 and 9
(c) Factorise
y=24 x2 −26 x−8
y=2 ( 12 x2−13 x −4 )
y=2 ( 12 x2−16 x+3 x−4 )
y=2 ( 12 x2+3 x−16 x−4 )
y=2 { ( 12 x2 +3 x ) − ( 16 x+ 4 ) }
y=2 { 3 x ( 4 x +1 ) −4 ( 4 x +1 ) }
y=2 { ( 4 x+1 ) ( 3 x−4 ) }
y=2 ( 4 x+1 ) (3 x −4)
5

Question 4
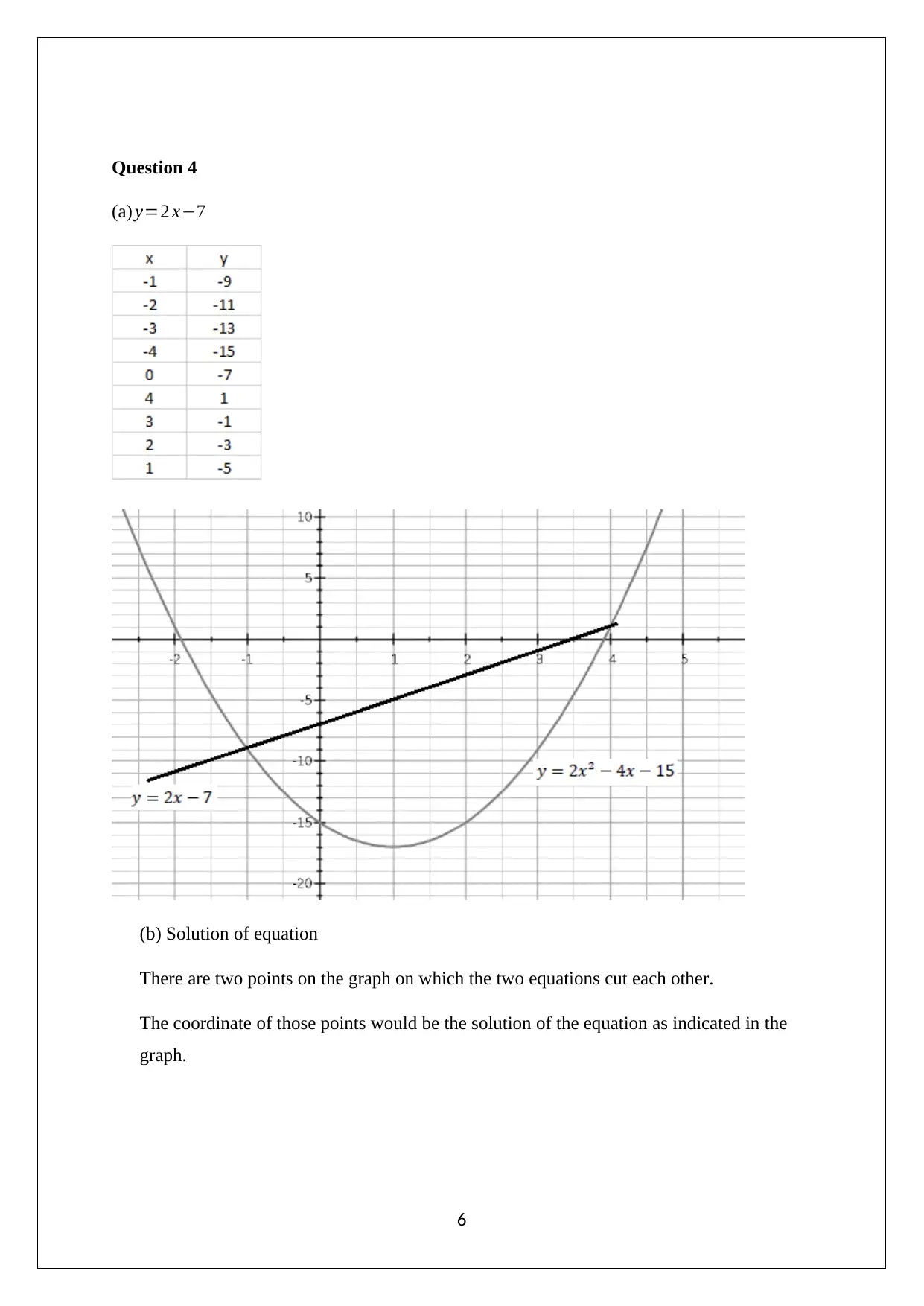
(a) y=2 x−7
(b) Solution of equation
There are two points on the graph on which the two equations cut each other.
The coordinate of those points would be the solution of the equation as indicated in the
graph.
6

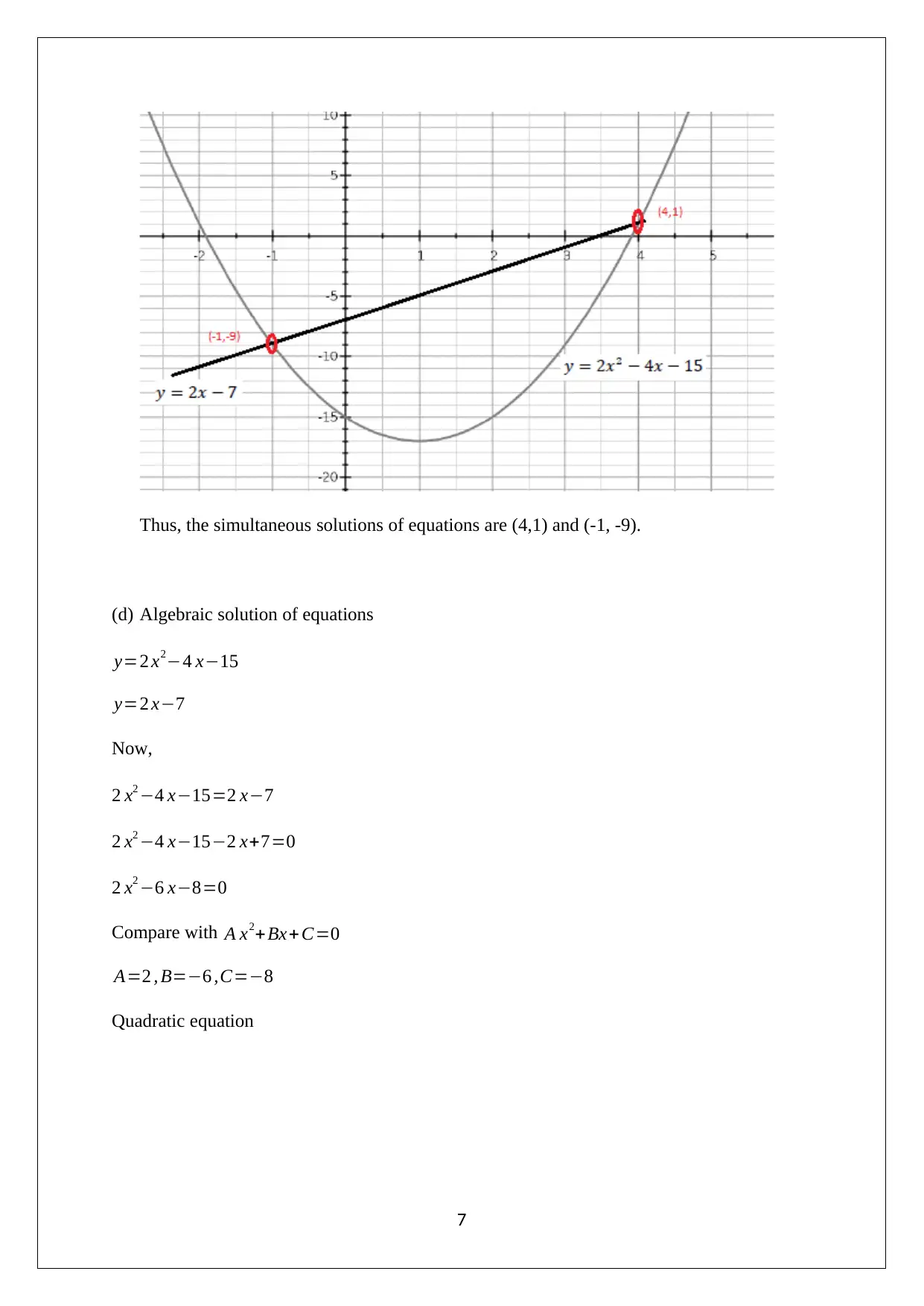
Thus, the simultaneous solutions of equations are (4,1) and (-1, -9).
(d) Algebraic solution of equations
y=2 x2−4 x−15
y=2 x−7
Now,
2 x2 −4 x−15=2 x−7
2 x2 −4 x−15−2 x+7=0
2 x2 −6 x−8=0
Compare with A x2+Bx+ C=0
A=2 , B=−6 ,C=−8
Quadratic equation
7

Now ,
x= 6+10
4 = 16
4 =4
y=2 x−7=2∗4−7=1
( 4,1 )
Similary,
x= 6−10
4 =−4
4 =−1
y=2 x−7= ( 2∗( −1 ) ) −7=−2−7=−9
(−1 ,−9 )
Thus, the solution of the equations are x = 4, -1
Question 5
(a) Maximum value of invrstment
Value of an investment
V =−0.6 t2 +15 t+ 200
dV
dt = d
dt ( −0.6 t 2+15 t +200 ) =−1.2t +15
For maximum investment
8

dV
dt =0
−1.2 t +15=0
t= 15
1.2 =12.5
t (max)=12.5
Now,
V ( max ) at t max
V (max)=−0.6 t2+15 t +200
V (max)=−0.6 ( 12.5 )2 +15(12.5)+200 = 293.75
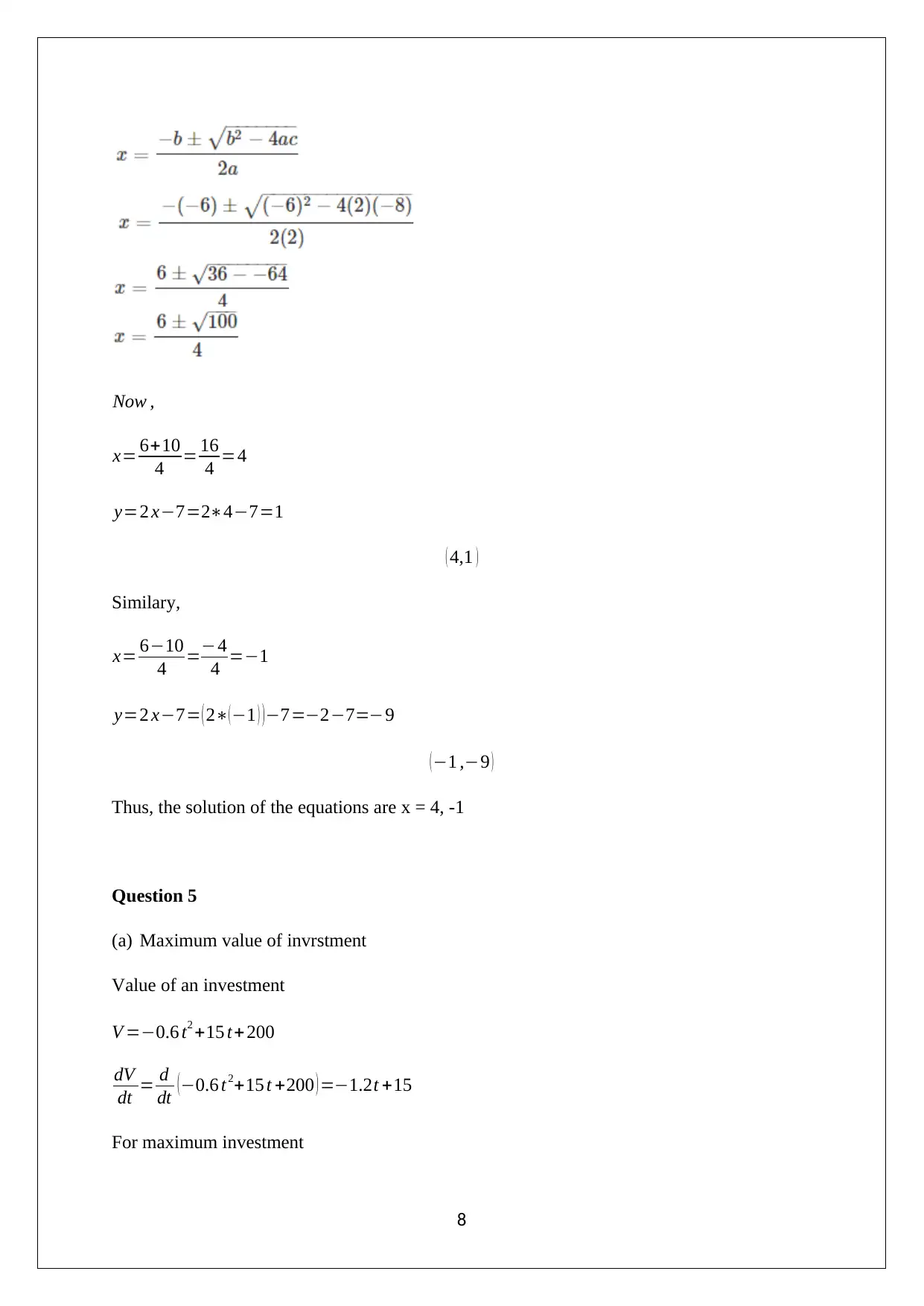
Therfore, the maximum value of investment would be $293,750.
(b) Graph
9

(c) V = $250,000
Value of an investment
V =−0.6 t2 +15 t+ 200
250=−0.6 t2+15 t +200
−0.6 t 2+15t−50=0
x=3.9∨21.03 4∨22 years
Question 6
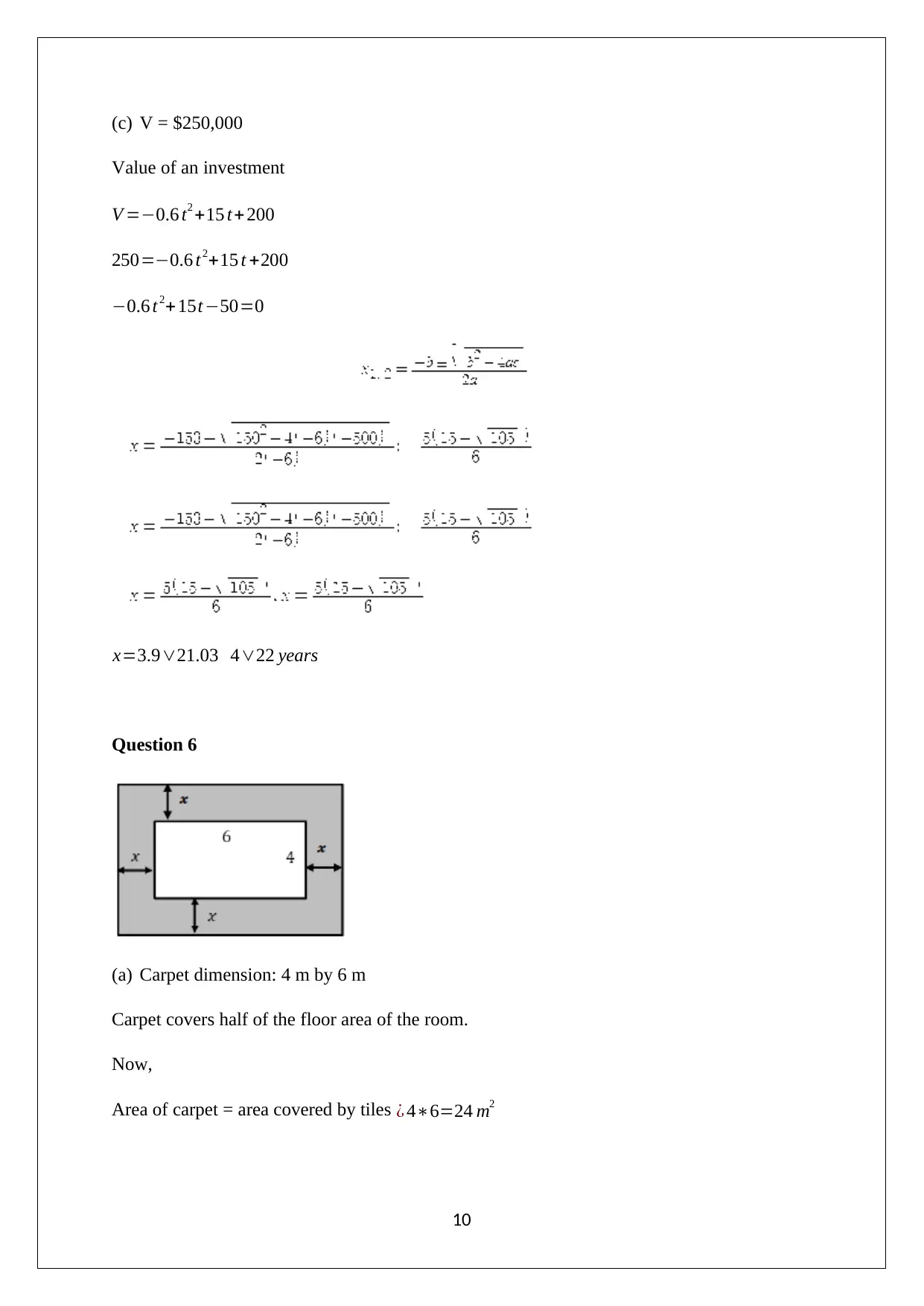
(a) Carpet dimension: 4 m by 6 m
Carpet covers half of the floor area of the room.
Now,
Area of carpet = area covered by tiles ¿ 4∗6=24 m2
10

Hence, 24 m2 of the room would be covered by tiles also since area covered by tiles is same
as that by carpet.
(b) Area covered by tiles
Area of room
Length = 4+2x
Width = 6 +2x
Total areaof room= ( 4 +2 x )∗( 6+2 x )
¿ 24+ 8 x+12 x+ 4 x2
¿ 4 x2 +20 x +24
Area of carpet ¿ 24 m2
Now,
Area covered by tiles ¿ 4 x2 +20 x +24−24=4 x2 +20 x
The area covered by tiles in standard form would be4 x2 +20 x .
(c) Area of the tiled portion is 24m2,
Hence, 4 x2 +20 x .−24=0
The equation can be divided by 4 throughout resulting in the following.
x2+ 5 x .−6=0
(x+6) (x-1) = 0
As a result, x = -6 or 1
Since x is length, hence it cannot be negative. Therefore, x = 1m
Thus, the dimensions of the room are (4+2*1) = 6 m and (6+2*1) = 8 m.
11