MATLAB Assignment
VerifiedAdded on 2023/06/14
|17
|2805
|311
AI Summary
This assignment covers topics such as Laplace transform, impulse response, transfer function, and more using MATLAB. Solutions are provided for various problems including finding the steady-state amplitude, drawing free body diagrams, and partial fraction expansion.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

Running head: MATLAB 1
MATLAB
Name
Institution
MATLAB
Name
Institution
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

MATLAB 2
MATLAB Assignment
Question 1
Part a
x ' ' +2 x ' + x=5 e−t + t
Apply Laplace transform on both sides
L { x' ' }+2 L { x' }+L { X }=5 L {e−t }+L {t }
s2 ˙x−sx ( 0 ) − ˙x ( 0 ) +2 ( s ˙x−x ( 0 ) ) + ˙x= 5
s +1 + 1
s2
But we know that x ( 0 )=0∧ ˙x ( 0 )=1. Substituting these into the above equation we get:
s2 ˙x−1+ s ( s ˙x ) + ˙x= 5
s +1 + 1
s2
˙x ( s2+ s+ 1 )=1+ 5
s +1 + 1
s2
˙x (s+1)2=1+ 5
s+1 + 1
s2
˙x= 1
(s+1)2 + 5
( s+1)3 + 1
s2 ( s+1)2
x=L−1
{ 1
(s+ 1)2 }+5 L−1
{ 1
(s+ 1)3 }+ L−1 { 1
s2 (s +1)2 }
L−1
{ 1
(s+ 1)2 }=e−t t
MATLAB Assignment
Question 1
Part a
x ' ' +2 x ' + x=5 e−t + t
Apply Laplace transform on both sides
L { x' ' }+2 L { x' }+L { X }=5 L {e−t }+L {t }
s2 ˙x−sx ( 0 ) − ˙x ( 0 ) +2 ( s ˙x−x ( 0 ) ) + ˙x= 5
s +1 + 1
s2
But we know that x ( 0 )=0∧ ˙x ( 0 )=1. Substituting these into the above equation we get:
s2 ˙x−1+ s ( s ˙x ) + ˙x= 5
s +1 + 1
s2
˙x ( s2+ s+ 1 )=1+ 5
s +1 + 1
s2
˙x (s+1)2=1+ 5
s+1 + 1
s2
˙x= 1
(s+1)2 + 5
( s+1)3 + 1
s2 ( s+1)2
x=L−1
{ 1
(s+ 1)2 }+5 L−1
{ 1
(s+ 1)3 }+ L−1 { 1
s2 (s +1)2 }
L−1
{ 1
(s+ 1)2 }=e−t t

MATLAB 3
5 L−1
{ 1
( s+1)3 }=5 e−t t 2
2
L−1
{ 1
s2 ( s+1 )2 }=t+2 e−t +e−t t−2
x=e−t t +5 e−t t2
2 +t +2 e−t +e−t t−2
Therefore, x=2 e−t t+2e−t + 5 e−t t2
2 +t−2
We can prove the answer using the MATLAB code,
syms y(t)
Dy = diff(y);
ode = diff(y,t,2)+2*diff(y,'t',1)+y == 5*exp(-t)+t;
cond1 = y(0) == 0;
cond2 = Dy(0) == 1;
conds = [cond1 cond2];
ySol(t) = dsolve(ode,conds);
ySol = simplify(ySol)
Part b⃛
y +3 ¨y +5 ˙y=⃛ x +4 ¨x +6 ˙x +8 x
Apply Laplace transform on both sides (remember all initial conditions are zero)
L {⃛ y }+ 3 L { ¨y } +5 L { ˙y }=L{⃛ x }+ 4 L { ¨x }+6 L { ˙x }+8 L {x }
L {⃛ y }=s3 Y ( s ) −s2 y ( 0 ) −s ˙y ( 0 ) − ¨y ( 0 ) =s3 Y ( s )
3 L { ¨y }=3 ¿
5 L { ˙y } =5 ¿
5 L−1
{ 1
( s+1)3 }=5 e−t t 2
2
L−1
{ 1
s2 ( s+1 )2 }=t+2 e−t +e−t t−2
x=e−t t +5 e−t t2
2 +t +2 e−t +e−t t−2
Therefore, x=2 e−t t+2e−t + 5 e−t t2
2 +t−2
We can prove the answer using the MATLAB code,
syms y(t)
Dy = diff(y);
ode = diff(y,t,2)+2*diff(y,'t',1)+y == 5*exp(-t)+t;
cond1 = y(0) == 0;
cond2 = Dy(0) == 1;
conds = [cond1 cond2];
ySol(t) = dsolve(ode,conds);
ySol = simplify(ySol)
Part b⃛
y +3 ¨y +5 ˙y=⃛ x +4 ¨x +6 ˙x +8 x
Apply Laplace transform on both sides (remember all initial conditions are zero)
L {⃛ y }+ 3 L { ¨y } +5 L { ˙y }=L{⃛ x }+ 4 L { ¨x }+6 L { ˙x }+8 L {x }
L {⃛ y }=s3 Y ( s ) −s2 y ( 0 ) −s ˙y ( 0 ) − ¨y ( 0 ) =s3 Y ( s )
3 L { ¨y }=3 ¿
5 L { ˙y } =5 ¿

MATLAB 4
L {⃛ x }=s3 X ( s )−s2 x ( 0 ) −s ˙x ( 0 )− ¨x ( 0 ) =s3 X ( s )
4 L { ¨x }=4 ¿
6 L { ˙x }=6 ¿
8 L { x ) =8 X ( s )
s3 Y ( s ) +3 s2 Y ( s ) +5 sY ( s ) =s3 X ( s ) + 4 s2 X ( s ) + 6 sX ( s ) +8 X ( s )
Simplifying the above equation:
Y ( s ) ( s3+3 s2+5 s ) =X ( s ) ( s3 + 4 s2 +6 s +8)
G ( s ) = Y ( s )
X ( s ) =(s3 +4 s2+ 6 s +8)
( s3 +3 s2 +5 s )
Using the final Value theorem,
lim
n → ∞
¿ ¿
Using MATLAB, the impulse response of G ( s ) is shown below.
L {⃛ x }=s3 X ( s )−s2 x ( 0 ) −s ˙x ( 0 )− ¨x ( 0 ) =s3 X ( s )
4 L { ¨x }=4 ¿
6 L { ˙x }=6 ¿
8 L { x ) =8 X ( s )
s3 Y ( s ) +3 s2 Y ( s ) +5 sY ( s ) =s3 X ( s ) + 4 s2 X ( s ) + 6 sX ( s ) +8 X ( s )
Simplifying the above equation:
Y ( s ) ( s3+3 s2+5 s ) =X ( s ) ( s3 + 4 s2 +6 s +8)
G ( s ) = Y ( s )
X ( s ) =(s3 +4 s2+ 6 s +8)
( s3 +3 s2 +5 s )
Using the final Value theorem,
lim
n → ∞
¿ ¿
Using MATLAB, the impulse response of G ( s ) is shown below.
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
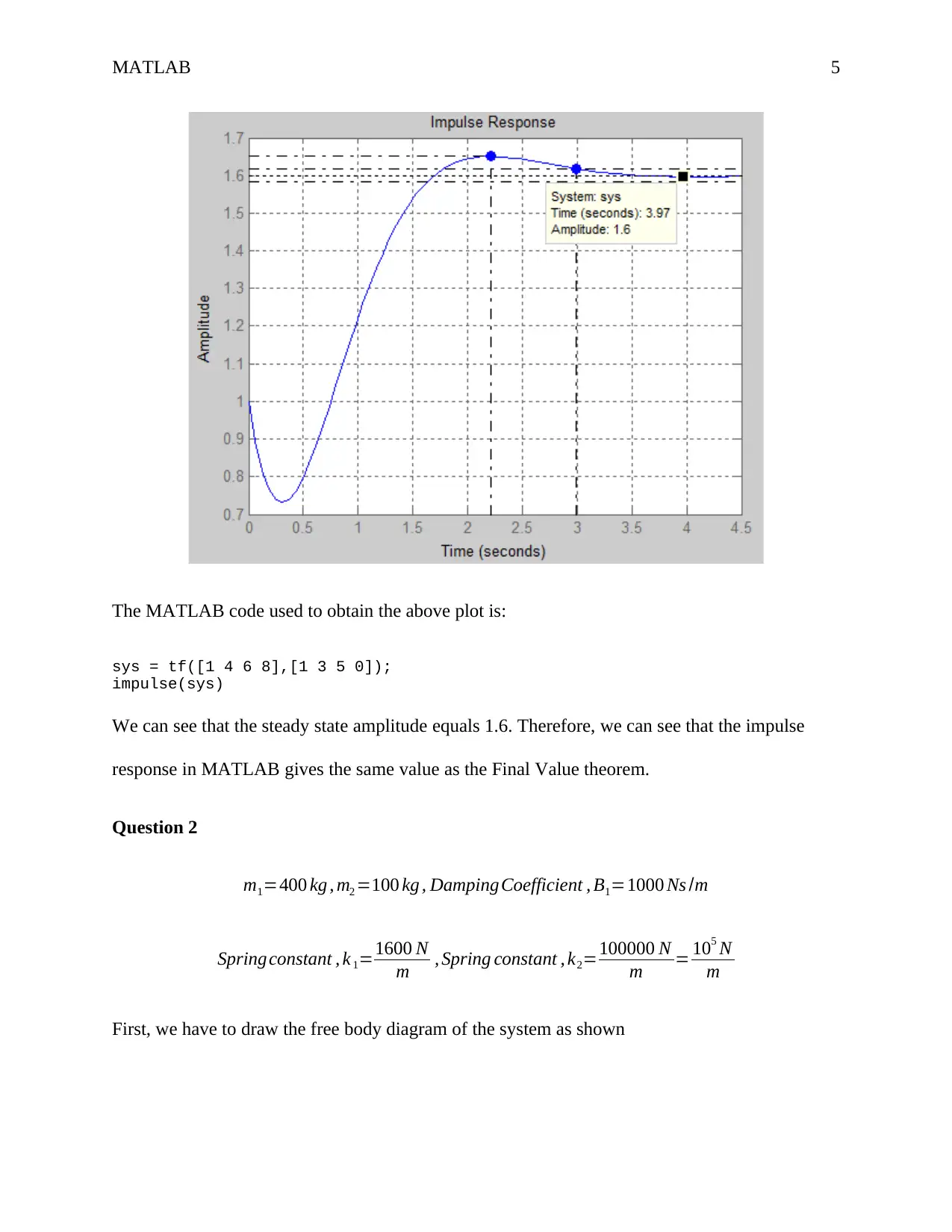
MATLAB 5
The MATLAB code used to obtain the above plot is:
sys = tf([1 4 6 8],[1 3 5 0]);
impulse(sys)
We can see that the steady state amplitude equals 1.6. Therefore, we can see that the impulse
response in MATLAB gives the same value as the Final Value theorem.
Question 2
m1=400 kg , m2 =100 kg , DampingCoefficient , B1=1000 Ns /m
Spring constant , k 1=1600 N
m , Spring constant , k2=100000 N
m = 105 N
m
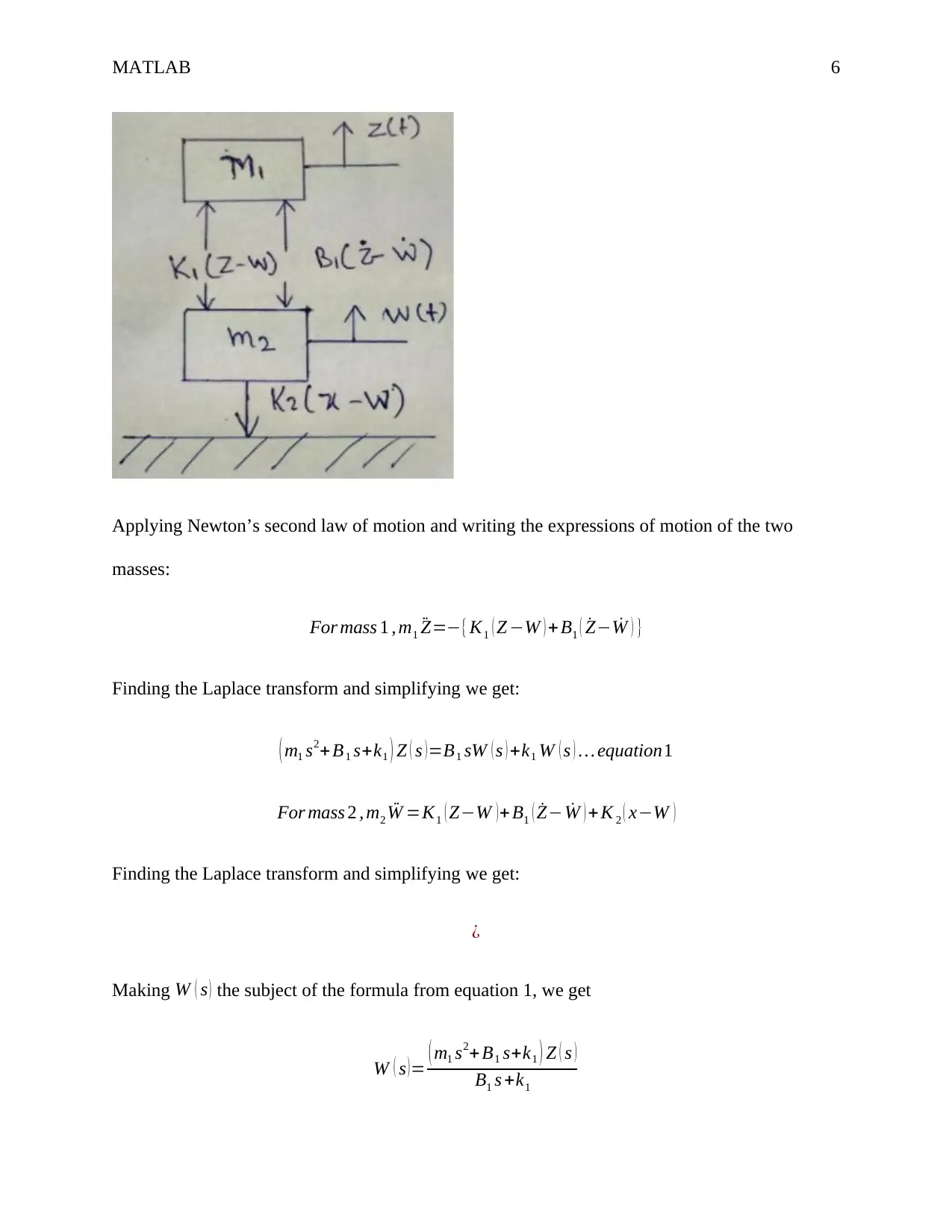
First, we have to draw the free body diagram of the system as shown
The MATLAB code used to obtain the above plot is:
sys = tf([1 4 6 8],[1 3 5 0]);
impulse(sys)
We can see that the steady state amplitude equals 1.6. Therefore, we can see that the impulse
response in MATLAB gives the same value as the Final Value theorem.
Question 2
m1=400 kg , m2 =100 kg , DampingCoefficient , B1=1000 Ns /m
Spring constant , k 1=1600 N
m , Spring constant , k2=100000 N
m = 105 N
m
First, we have to draw the free body diagram of the system as shown
MATLAB 6
Applying Newton’s second law of motion and writing the expressions of motion of the two
masses:
For mass 1 , m1 ¨Z=−{K1 ( Z −W ) + B1 ( ˙Z− ˙W ) }
Finding the Laplace transform and simplifying we get:
( m1 s2+B1 s+k1 ) Z ( s ) =B1 sW ( s ) +k1 W ( s ) … equation1
For mass 2 , m2 ¨W =K1 ( Z−W ) + B1 ( ˙Z− ˙W ) + K 2 ( x−W )
Finding the Laplace transform and simplifying we get:
¿
Making W ( s ) the subject of the formula from equation 1, we get
W ( s )= ( m1 s2+ B1 s+k1 ) Z ( s )
B1 s +k1
Applying Newton’s second law of motion and writing the expressions of motion of the two
masses:
For mass 1 , m1 ¨Z=−{K1 ( Z −W ) + B1 ( ˙Z− ˙W ) }
Finding the Laplace transform and simplifying we get:
( m1 s2+B1 s+k1 ) Z ( s ) =B1 sW ( s ) +k1 W ( s ) … equation1
For mass 2 , m2 ¨W =K1 ( Z−W ) + B1 ( ˙Z− ˙W ) + K 2 ( x−W )
Finding the Laplace transform and simplifying we get:
¿
Making W ( s ) the subject of the formula from equation 1, we get
W ( s )= ( m1 s2+ B1 s+k1 ) Z ( s )
B1 s +k1

MATLAB 7
Substituting this value in equation 2,
¿
Upon simplification we get:
Z ( s )
R ( s ) = (B¿¿ 1 s+ k1 ) K2
m1 m2 s4 +(m¿¿ 1+m2 )B1 s3 +¿ ¿ ¿ ¿ ¿
Substituting the values in the above equation:
Z ( s )
R ( s ) = (1000 s +1600)105
40000 s4 +500000 s3+(4 ×107 +(500)1600) s2 +108 s+16 ×107
Simplifying
Hence , Z ( s )
R ( s ) = 2500 s +4000
s4 +12.5 s3 +1020 s2+ 6500
Part b
We can use the MATLAB code to plot the transfer function.
H = tf([2500 4000],[1 12.5 1020 0 6500]);
bode(H)
The resulting plot is shown in figure 1 below
Substituting this value in equation 2,
¿
Upon simplification we get:
Z ( s )
R ( s ) = (B¿¿ 1 s+ k1 ) K2
m1 m2 s4 +(m¿¿ 1+m2 )B1 s3 +¿ ¿ ¿ ¿ ¿
Substituting the values in the above equation:
Z ( s )
R ( s ) = (1000 s +1600)105
40000 s4 +500000 s3+(4 ×107 +(500)1600) s2 +108 s+16 ×107
Simplifying
Hence , Z ( s )
R ( s ) = 2500 s +4000
s4 +12.5 s3 +1020 s2+ 6500
Part b
We can use the MATLAB code to plot the transfer function.
H = tf([2500 4000],[1 12.5 1020 0 6500]);
bode(H)
The resulting plot is shown in figure 1 below
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
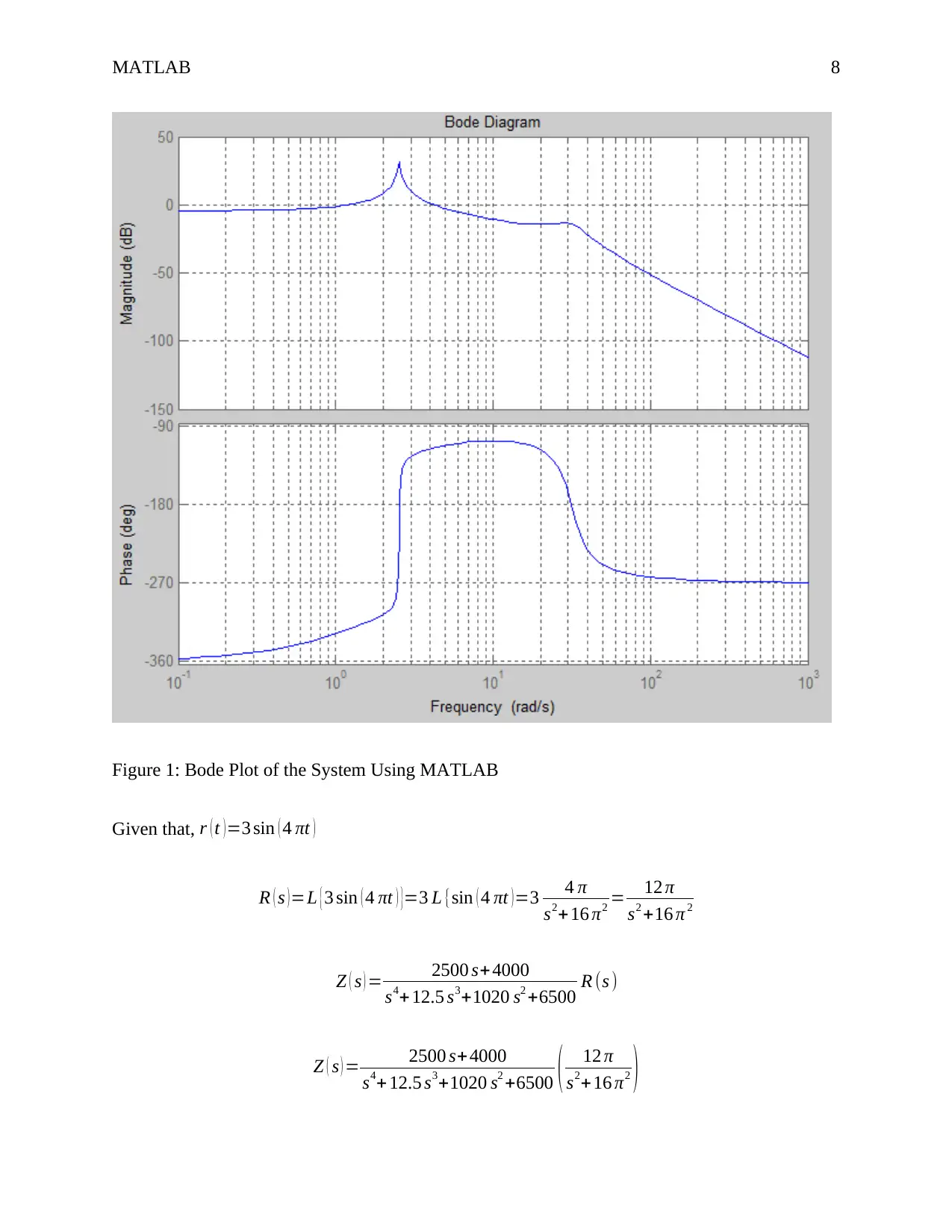
MATLAB 8
Figure 1: Bode Plot of the System Using MATLAB
Given that, r ( t )=3 sin ( 4 πt )
R ( s )=L {3 sin ( 4 πt ) }=3 L {sin ( 4 πt )=3 4 π
s2+16 π2 = 12 π
s2 +16 π 2
Z ( s ) = 2500 s+4000
s4+ 12.5 s3+1020 s2 +6500 R (s )
Z ( s ) = 2500 s+4000
s4+ 12.5 s3+1020 s2 +6500 ( 12 π
s2+16 π2 )
Figure 1: Bode Plot of the System Using MATLAB
Given that, r ( t )=3 sin ( 4 πt )
R ( s )=L {3 sin ( 4 πt ) }=3 L {sin ( 4 πt )=3 4 π
s2+16 π2 = 12 π
s2 +16 π 2
Z ( s ) = 2500 s+4000
s4+ 12.5 s3+1020 s2 +6500 R (s )
Z ( s ) = 2500 s+4000
s4+ 12.5 s3+1020 s2 +6500 ( 12 π
s2+16 π2 )
MATLAB 9
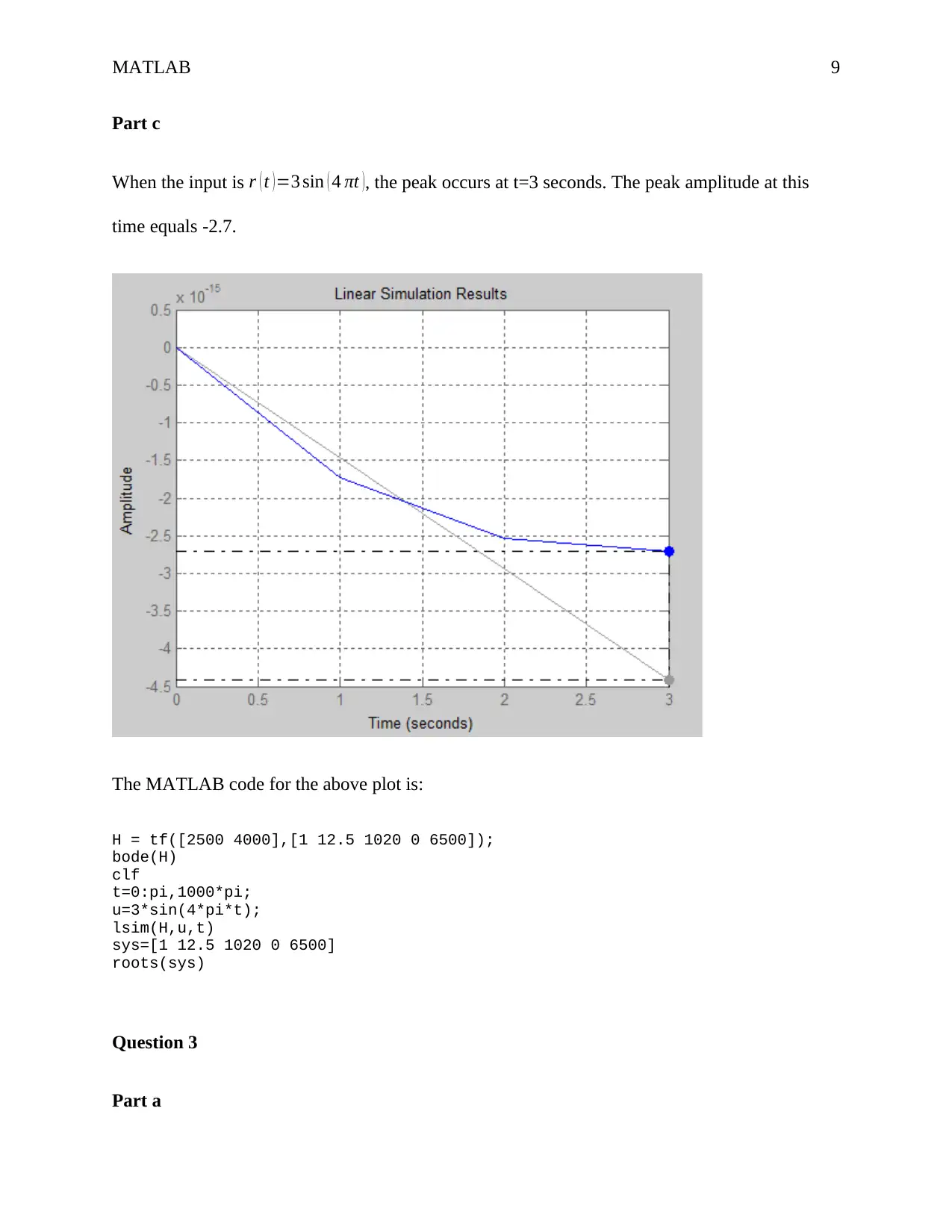
Part c
When the input is r ( t )=3 sin ( 4 πt ), the peak occurs at t=3 seconds. The peak amplitude at this
time equals -2.7.
The MATLAB code for the above plot is:
H = tf([2500 4000],[1 12.5 1020 0 6500]);
bode(H)
clf
t=0:pi,1000*pi;
u=3*sin(4*pi*t);
lsim(H,u,t)
sys=[1 12.5 1020 0 6500]
roots(sys)
Question 3
Part a
Part c
When the input is r ( t )=3 sin ( 4 πt ), the peak occurs at t=3 seconds. The peak amplitude at this
time equals -2.7.
The MATLAB code for the above plot is:
H = tf([2500 4000],[1 12.5 1020 0 6500]);
bode(H)
clf
t=0:pi,1000*pi;
u=3*sin(4*pi*t);
lsim(H,u,t)
sys=[1 12.5 1020 0 6500]
roots(sys)
Question 3
Part a
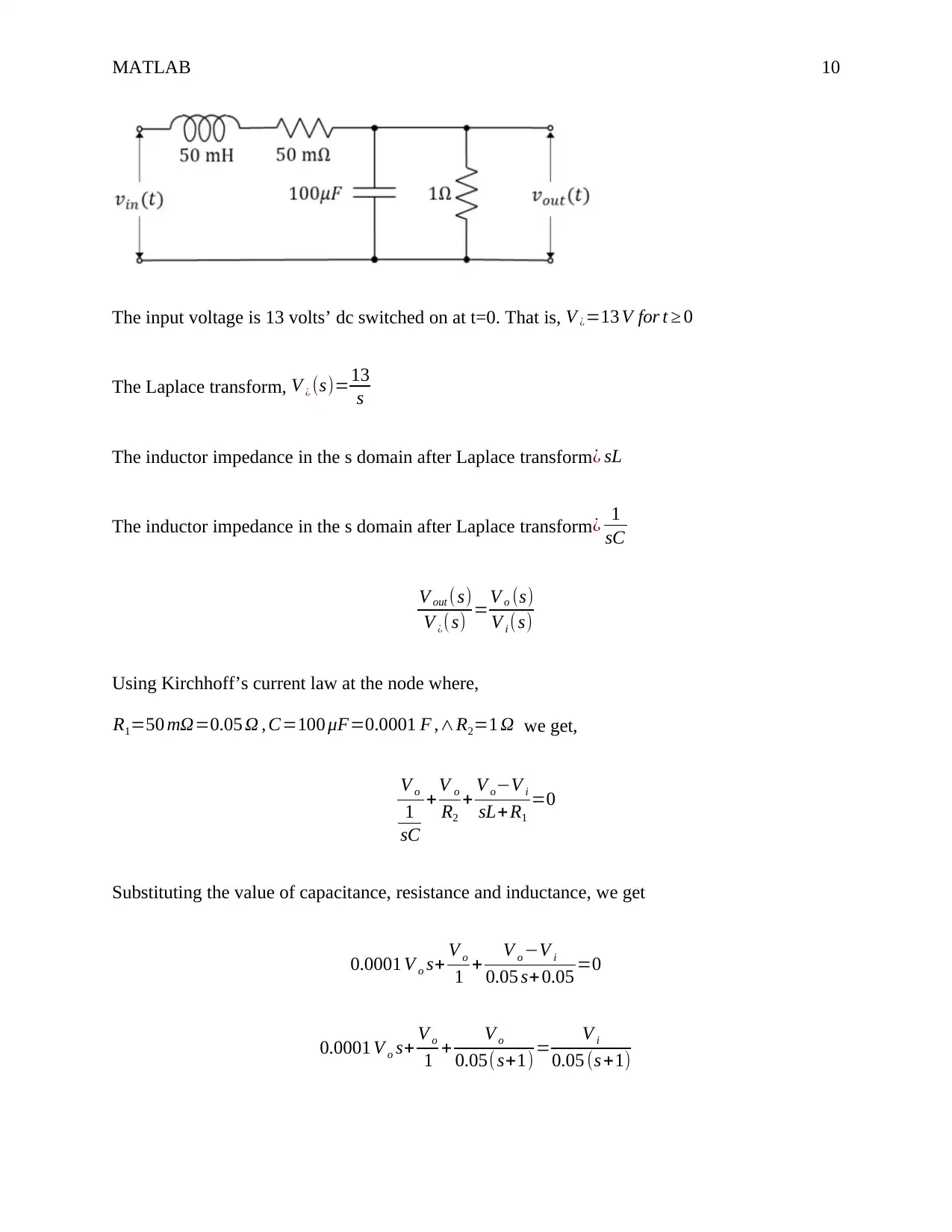
MATLAB 10
The input voltage is 13 volts’ dc switched on at t=0. That is, V ¿=13 V for t ≥ 0
The Laplace transform, V ¿ (s)=13
s
The inductor impedance in the s domain after Laplace transform ¿ sL
The inductor impedance in the s domain after Laplace transform¿ 1
sC
V out (s)
V ¿(s) =V o (s)
V i (s)
Using Kirchhoff’s current law at the node where,
R1=50 mΩ=0.05 Ω , C=100 μF=0.0001 F ,∧R2=1 Ω we get,
V o
1
sC
+ V o
R2
+ V o−V i
sL+ R1
=0
Substituting the value of capacitance, resistance and inductance, we get
0.0001 V o s+ V o
1 + V o −V i
0.05 s+ 0.05 =0
0.0001 V o s+ V o
1 + V o
0.05( s+1) = V i
0.05 (s +1)
The input voltage is 13 volts’ dc switched on at t=0. That is, V ¿=13 V for t ≥ 0
The Laplace transform, V ¿ (s)=13
s
The inductor impedance in the s domain after Laplace transform ¿ sL
The inductor impedance in the s domain after Laplace transform¿ 1
sC
V out (s)
V ¿(s) =V o (s)
V i (s)
Using Kirchhoff’s current law at the node where,
R1=50 mΩ=0.05 Ω , C=100 μF=0.0001 F ,∧R2=1 Ω we get,
V o
1
sC
+ V o
R2
+ V o−V i
sL+ R1
=0
Substituting the value of capacitance, resistance and inductance, we get
0.0001 V o s+ V o
1 + V o −V i
0.05 s+ 0.05 =0
0.0001 V o s+ V o
1 + V o
0.05( s+1) = V i
0.05 (s +1)
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
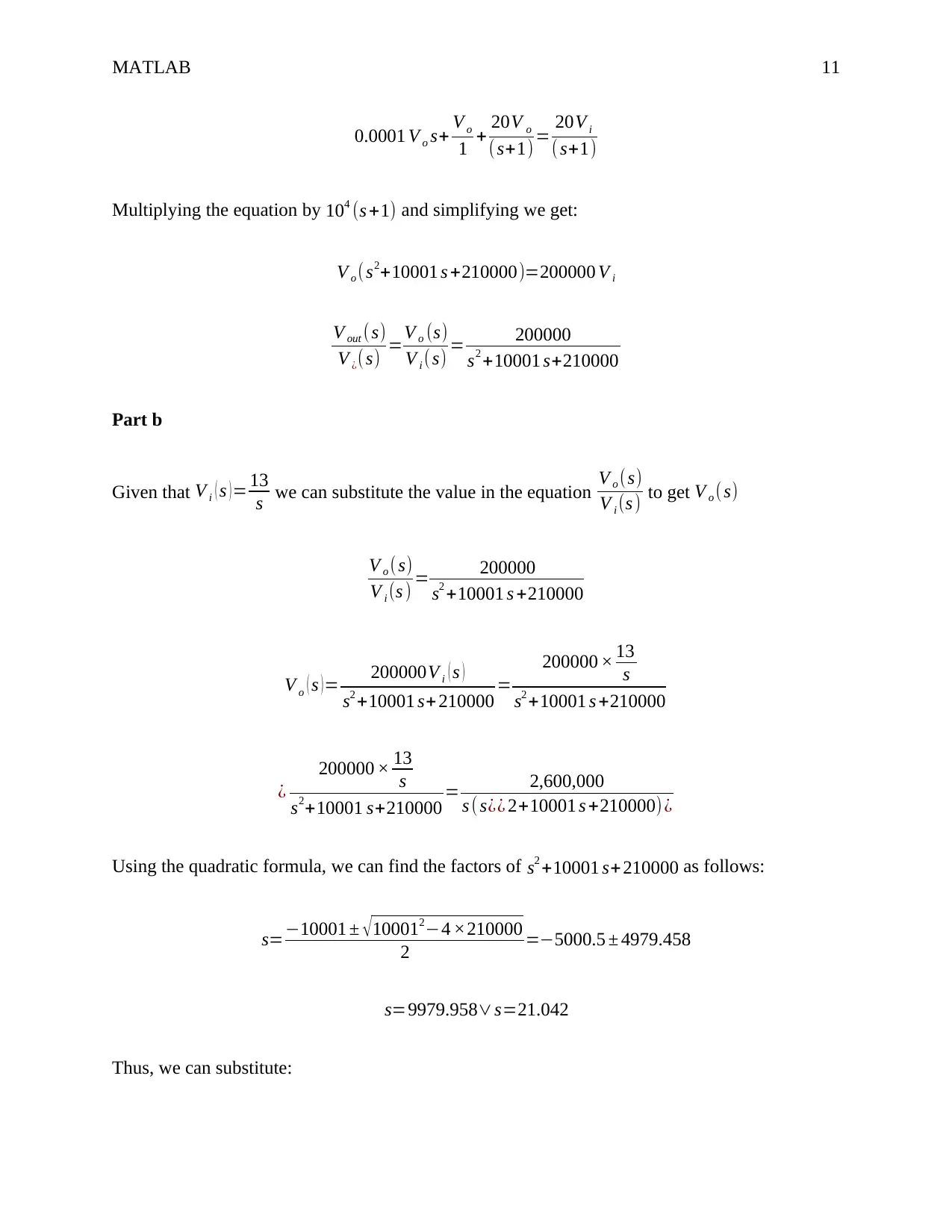
MATLAB 11
0.0001 V o s+ V o
1 + 20V o
( s+1)= 20V i
( s+1)
Multiplying the equation by 104 (s +1) and simplifying we get:
V o (s2+10001 s +210000)=200000 V i
V out ( s)
V ¿(s) =V o (s)
V i (s) = 200000
s2 +10001 s+210000
Part b
Given that V i ( s ) = 13
s we can substitute the value in the equation V o (s)
V i (s ) to get V o ( s)
V o ( s)
V i (s ) = 200000
s2 +10001 s +210000
V o ( s )= 200000V i ( s )
s2 +10001 s+ 210000 =
200000 × 13
s
s2 +10001 s +210000
¿
200000 × 13
s
s2+10001 s+210000 = 2,600,000
s ( s¿¿ 2+10001 s +210000) ¿
Using the quadratic formula, we can find the factors of s2 +10001 s+ 210000 as follows:
s=−10001 ± √100012−4 ×210000
2 =−5000.5 ± 4979.458
s=9979.958∨s=21.042
Thus, we can substitute:
0.0001 V o s+ V o
1 + 20V o
( s+1)= 20V i
( s+1)
Multiplying the equation by 104 (s +1) and simplifying we get:
V o (s2+10001 s +210000)=200000 V i
V out ( s)
V ¿(s) =V o (s)
V i (s) = 200000
s2 +10001 s+210000
Part b
Given that V i ( s ) = 13
s we can substitute the value in the equation V o (s)
V i (s ) to get V o ( s)
V o ( s)
V i (s ) = 200000
s2 +10001 s +210000
V o ( s )= 200000V i ( s )
s2 +10001 s+ 210000 =
200000 × 13
s
s2 +10001 s +210000
¿
200000 × 13
s
s2+10001 s+210000 = 2,600,000
s ( s¿¿ 2+10001 s +210000) ¿
Using the quadratic formula, we can find the factors of s2 +10001 s+ 210000 as follows:
s=−10001 ± √100012−4 ×210000
2 =−5000.5 ± 4979.458
s=9979.958∨s=21.042
Thus, we can substitute:

MATLAB 12
s2 +10001 s+ 210000=(s +9979.958)(s +21.042)
V o ( s )= 2,600,000
s(s+9979.958)( s+21.042)
2,600,000
s (s +9979.958)( s+21.042)= A
s + B
( s+ 9979.958) + C
(s +21.042)
We can do a partial fraction expansion of the RHS of the above equation as follows:
2,600,000= A ( s+9979.958 ) ( s+21.042 )+ B( s) ( s +21.042 ) +C( s)(s+ 9979.958)
If s=0
2,600,000= A ( 0+9979.958 ) ( 0+21.042 )= A (10001)
A= 2600000
209998.2762 =12.381
If s=−9979.258
2,600,000=B(−9979.258) ( −9979.258+ 21.042 )
B=0.0262
If s=−21.042
2,600,000=C (−21.042)(−21.042+9979.958)
C=−12.4072
We can rewrite the equation as:
V o ( s )= A
s + B
( s+9979.958) + C
(s +21.042)= 12.381
s + 0.0262
( s+ 9979.958) − 12.4072
( s+21.042)
Taking the inverse Laplace transform of the above equation we get:
V o ( t ) =12.381+0.0262 e−9979.958 t−12.4072 e−21.042t
Part c
How long it takes for V o ( t ) to reach 6V
V o ( t ) =12.381+0.0262 e−9979.958 t−12.4072 e−21.042t
6=12.381+ 0.0262 e−9979.958 t−12.4072 e−21.042t
Simplifying
s2 +10001 s+ 210000=(s +9979.958)(s +21.042)
V o ( s )= 2,600,000
s(s+9979.958)( s+21.042)
2,600,000
s (s +9979.958)( s+21.042)= A
s + B
( s+ 9979.958) + C
(s +21.042)
We can do a partial fraction expansion of the RHS of the above equation as follows:
2,600,000= A ( s+9979.958 ) ( s+21.042 )+ B( s) ( s +21.042 ) +C( s)(s+ 9979.958)
If s=0
2,600,000= A ( 0+9979.958 ) ( 0+21.042 )= A (10001)
A= 2600000
209998.2762 =12.381
If s=−9979.258
2,600,000=B(−9979.258) ( −9979.258+ 21.042 )
B=0.0262
If s=−21.042
2,600,000=C (−21.042)(−21.042+9979.958)
C=−12.4072
We can rewrite the equation as:
V o ( s )= A
s + B
( s+9979.958) + C
(s +21.042)= 12.381
s + 0.0262
( s+ 9979.958) − 12.4072
( s+21.042)
Taking the inverse Laplace transform of the above equation we get:
V o ( t ) =12.381+0.0262 e−9979.958 t−12.4072 e−21.042t
Part c
How long it takes for V o ( t ) to reach 6V
V o ( t ) =12.381+0.0262 e−9979.958 t−12.4072 e−21.042t
6=12.381+ 0.0262 e−9979.958 t−12.4072 e−21.042t
Simplifying

MATLAB 13
6.381=12.4072 e−21.042t −0.0262 e−9979.958 t
Using the Excel function “What if Analysis” by following the following steps
First, type the function =12.381+0.0262 {e} ^ {-9979.958t} -12.4072 {e} ^ {-21.042t in
cell B3 as shown in the screenshot below:
Second, type any value in cell B2.
Then, go to Data→ What If analysis → Goal Seek. After that, set the values as
shown in the screenshot below.
After clicking ok, Excel will return the value of t=0.031594411 as shown in
cell B2
t (V o=6V )=0.031594411 s ≈ 315.94 ×10−4 Seconds
Question 4
For the electrical part, we apply KVL to obtain
ea−Ra Ia−L d Ia
dt −Kb ωm=0
I a=
ea−L d Ia
dt −Kb ωm
Ra
But T m=Kt I a
6.381=12.4072 e−21.042t −0.0262 e−9979.958 t
Using the Excel function “What if Analysis” by following the following steps
First, type the function =12.381+0.0262 {e} ^ {-9979.958t} -12.4072 {e} ^ {-21.042t in
cell B3 as shown in the screenshot below:
Second, type any value in cell B2.
Then, go to Data→ What If analysis → Goal Seek. After that, set the values as
shown in the screenshot below.
After clicking ok, Excel will return the value of t=0.031594411 as shown in
cell B2
t (V o=6V )=0.031594411 s ≈ 315.94 ×10−4 Seconds
Question 4
For the electrical part, we apply KVL to obtain
ea−Ra Ia−L d Ia
dt −Kb ωm=0
I a=
ea−L d Ia
dt −Kb ωm
Ra
But T m=Kt I a
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
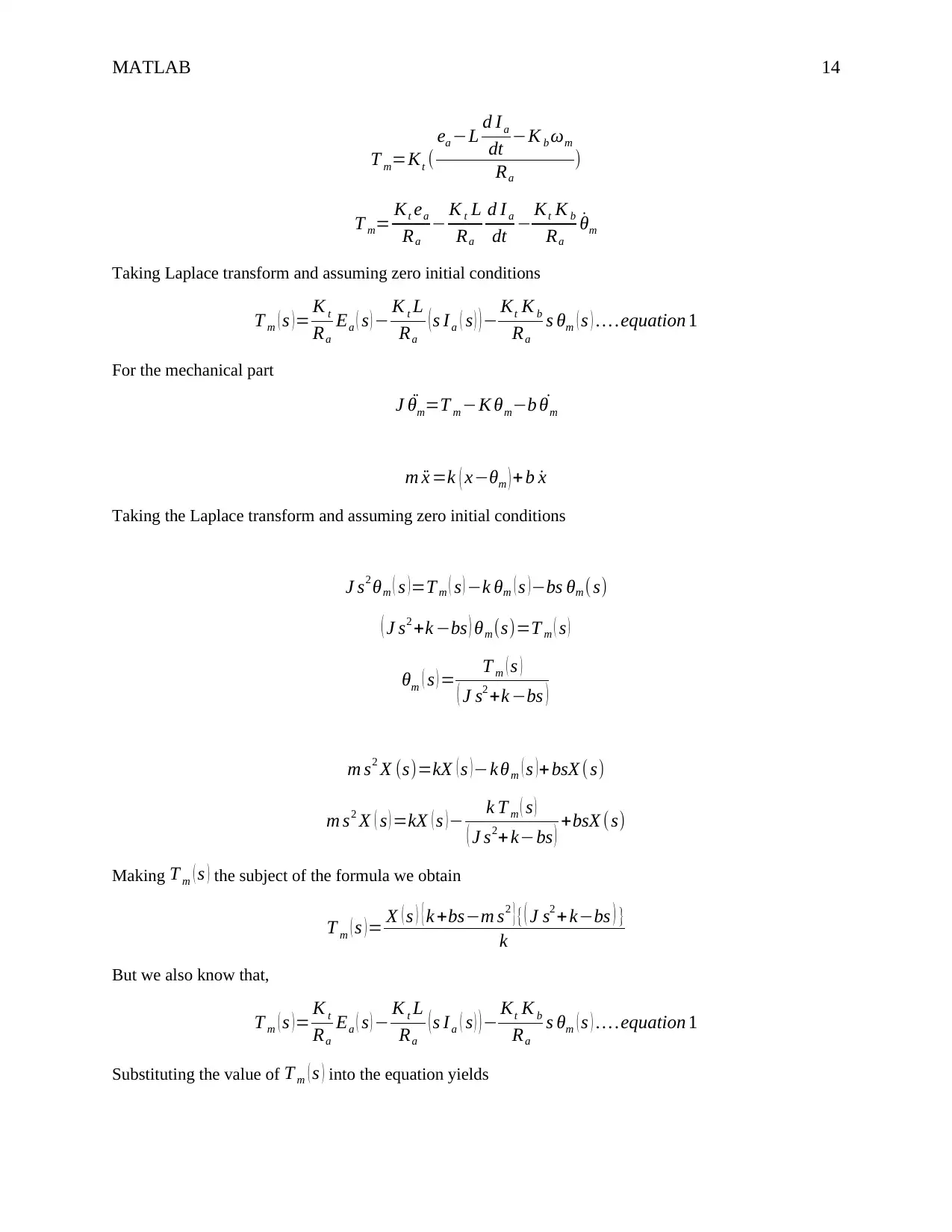
MATLAB 14
T m=Kt (
ea −L d I a
dt −K b ωm
Ra
)
T m= Kt ea
Ra
− K t L
Ra
d I a
dt − Kt K b
Ra
˙θm
Taking Laplace transform and assuming zero initial conditions
T m ( s )= K t
Ra
Ea ( s ) − K t L
Ra
(s Ia ( s ) )− Kt K b
Ra
s θm ( s ) … .equation 1
For the mechanical part
J ¨θm=T m −K θm−b ˙θm
m ¨x =k ( x−θm ) +b ˙x
Taking the Laplace transform and assuming zero initial conditions
J s2 θm ( s )=T m ( s ) −k θm ( s )−bs θm ( s)
( J s2 +k −bs ) θm(s)=T m ( s )
θm ( s ) = T m ( s )
( J s2 +k −bs )
m s2 X (s)=kX ( s )−k θm ( s )+ bsX ( s)
m s2 X ( s ) =kX ( s )− k T m ( s )
( J s2+ k−bs ) +bsX (s)
Making T m ( s ) the subject of the formula we obtain
T m ( s )= X ( s ) {k +bs−m s2 }{( J s2 + k−bs ) }
k
But we also know that,
T m ( s )= K t
Ra
Ea ( s ) − K t L
Ra
(s Ia ( s ) )− Kt K b
Ra
s θm ( s ) … .equation 1
Substituting the value of T m ( s ) into the equation yields
T m=Kt (
ea −L d I a
dt −K b ωm
Ra
)
T m= Kt ea
Ra
− K t L
Ra
d I a
dt − Kt K b
Ra
˙θm
Taking Laplace transform and assuming zero initial conditions
T m ( s )= K t
Ra
Ea ( s ) − K t L
Ra
(s Ia ( s ) )− Kt K b
Ra
s θm ( s ) … .equation 1
For the mechanical part
J ¨θm=T m −K θm−b ˙θm
m ¨x =k ( x−θm ) +b ˙x
Taking the Laplace transform and assuming zero initial conditions
J s2 θm ( s )=T m ( s ) −k θm ( s )−bs θm ( s)
( J s2 +k −bs ) θm(s)=T m ( s )
θm ( s ) = T m ( s )
( J s2 +k −bs )
m s2 X (s)=kX ( s )−k θm ( s )+ bsX ( s)
m s2 X ( s ) =kX ( s )− k T m ( s )
( J s2+ k−bs ) +bsX (s)
Making T m ( s ) the subject of the formula we obtain
T m ( s )= X ( s ) {k +bs−m s2 }{( J s2 + k−bs ) }
k
But we also know that,
T m ( s )= K t
Ra
Ea ( s ) − K t L
Ra
(s Ia ( s ) )− Kt K b
Ra
s θm ( s ) … .equation 1
Substituting the value of T m ( s ) into the equation yields
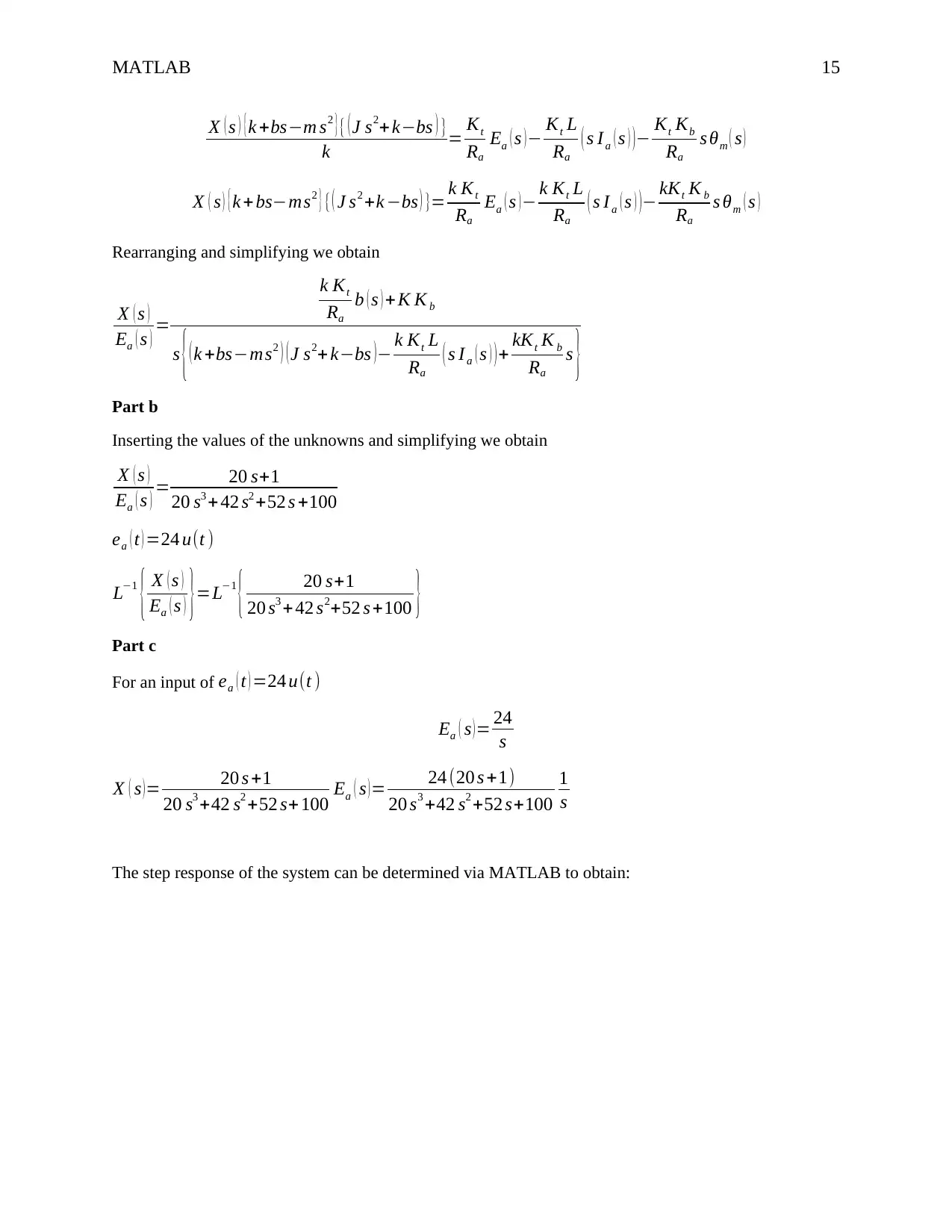
MATLAB 15
X ( s ) {k +bs−m s2 }{ ( J s2+ k−bs ) }
k = Kt
Ra
Ea ( s )− Kt L
Ra
( s Ia ( s ) )− Kt Kb
Ra
s θm ( s )
X ( s ) { k + bs−ms2 } {( J s2 +k −bs ) }= k Kt
Ra
Ea ( s ) − k Kt L
Ra
( s I a ( s ) )− kKt K b
Ra
s θm ( s )
Rearranging and simplifying we obtain
X ( s )
Ea ( s ) =
k Kt
Ra
b ( s ) + K K b
s {( k +bs−ms2 ) ( J s2+ k−bs )− k Kt L
Ra
( s I a ( s ) ) + kKt K b
Ra
s }
Part b
Inserting the values of the unknowns and simplifying we obtain
X ( s )
Ea ( s ) = 20 s+1
20 s3 + 42 s2 +52 s +100
ea ( t ) =24 u(t )
L−1
{ X ( s )
Ea ( s ) }=L−1
{ 20 s+1
20 s3 + 42 s2+52 s +100 }
Part c
For an input of ea ( t ) =24 u(t )
Ea ( s )= 24
s
X ( s )= 20 s +1
20 s3 +42 s2 +52 s+ 100 Ea ( s )= 24 (20 s +1)
20 s3 +42 s2 +52 s+100
1
s
The step response of the system can be determined via MATLAB to obtain:
X ( s ) {k +bs−m s2 }{ ( J s2+ k−bs ) }
k = Kt
Ra
Ea ( s )− Kt L
Ra
( s Ia ( s ) )− Kt Kb
Ra
s θm ( s )
X ( s ) { k + bs−ms2 } {( J s2 +k −bs ) }= k Kt
Ra
Ea ( s ) − k Kt L
Ra
( s I a ( s ) )− kKt K b
Ra
s θm ( s )
Rearranging and simplifying we obtain
X ( s )
Ea ( s ) =
k Kt
Ra
b ( s ) + K K b
s {( k +bs−ms2 ) ( J s2+ k−bs )− k Kt L
Ra
( s I a ( s ) ) + kKt K b
Ra
s }
Part b
Inserting the values of the unknowns and simplifying we obtain
X ( s )
Ea ( s ) = 20 s+1
20 s3 + 42 s2 +52 s +100
ea ( t ) =24 u(t )
L−1
{ X ( s )
Ea ( s ) }=L−1
{ 20 s+1
20 s3 + 42 s2+52 s +100 }
Part c
For an input of ea ( t ) =24 u(t )
Ea ( s )= 24
s
X ( s )= 20 s +1
20 s3 +42 s2 +52 s+ 100 Ea ( s )= 24 (20 s +1)
20 s3 +42 s2 +52 s+100
1
s
The step response of the system can be determined via MATLAB to obtain:
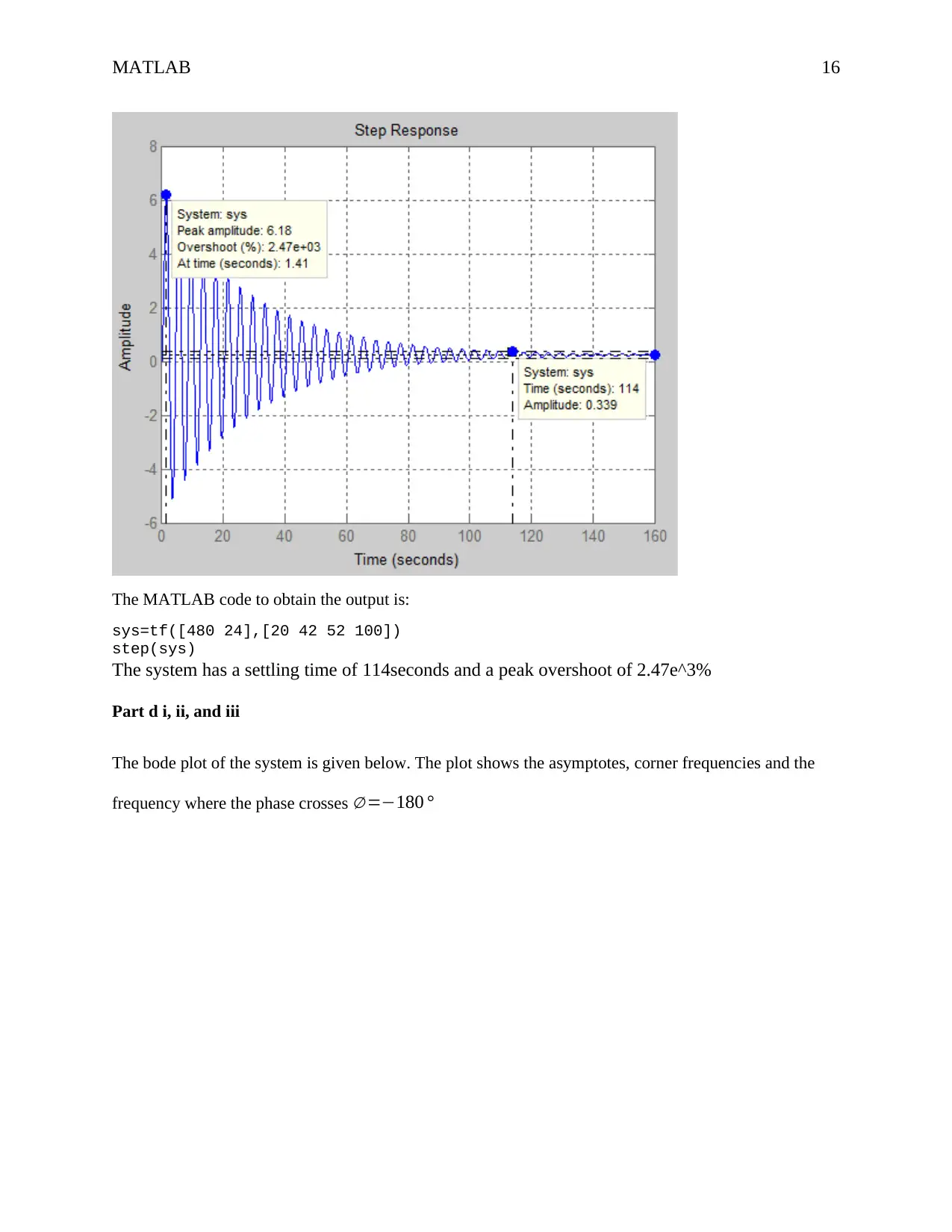
MATLAB 16
The MATLAB code to obtain the output is:
sys=tf([480 24],[20 42 52 100])
step(sys)
The system has a settling time of 114seconds and a peak overshoot of 2.47e^3%
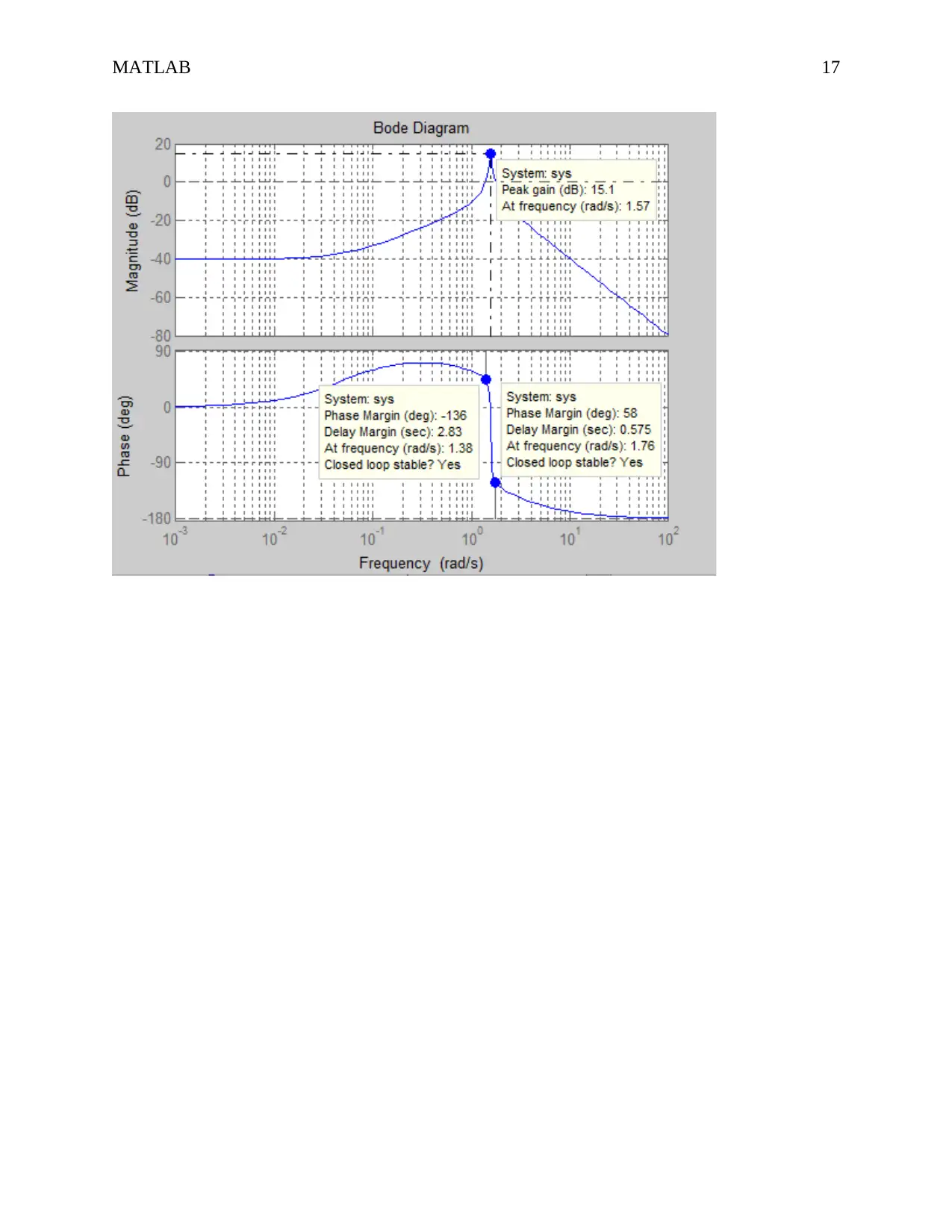
Part d i, ii, and iii
The bode plot of the system is given below. The plot shows the asymptotes, corner frequencies and the
frequency where the phase crosses ∅ =−180 °
The MATLAB code to obtain the output is:
sys=tf([480 24],[20 42 52 100])
step(sys)
The system has a settling time of 114seconds and a peak overshoot of 2.47e^3%
Part d i, ii, and iii
The bode plot of the system is given below. The plot shows the asymptotes, corner frequencies and the
frequency where the phase crosses ∅ =−180 °
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
MATLAB 17
1 out of 17
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.





