Mechanical Engineering Assignment: Solutions to Various Problems
VerifiedAdded on 2023/06/03
|16
|2191
|317
Homework Assignment
AI Summary
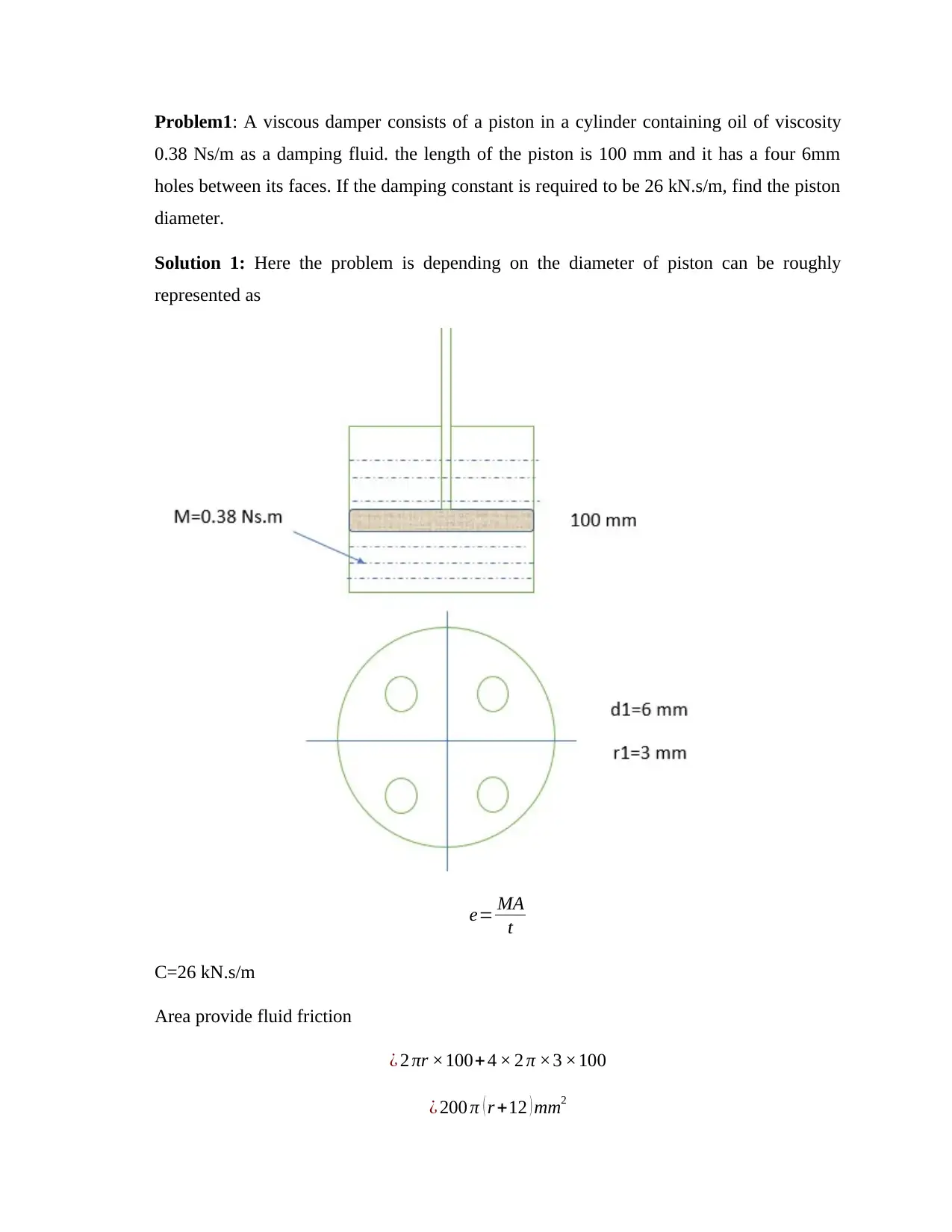
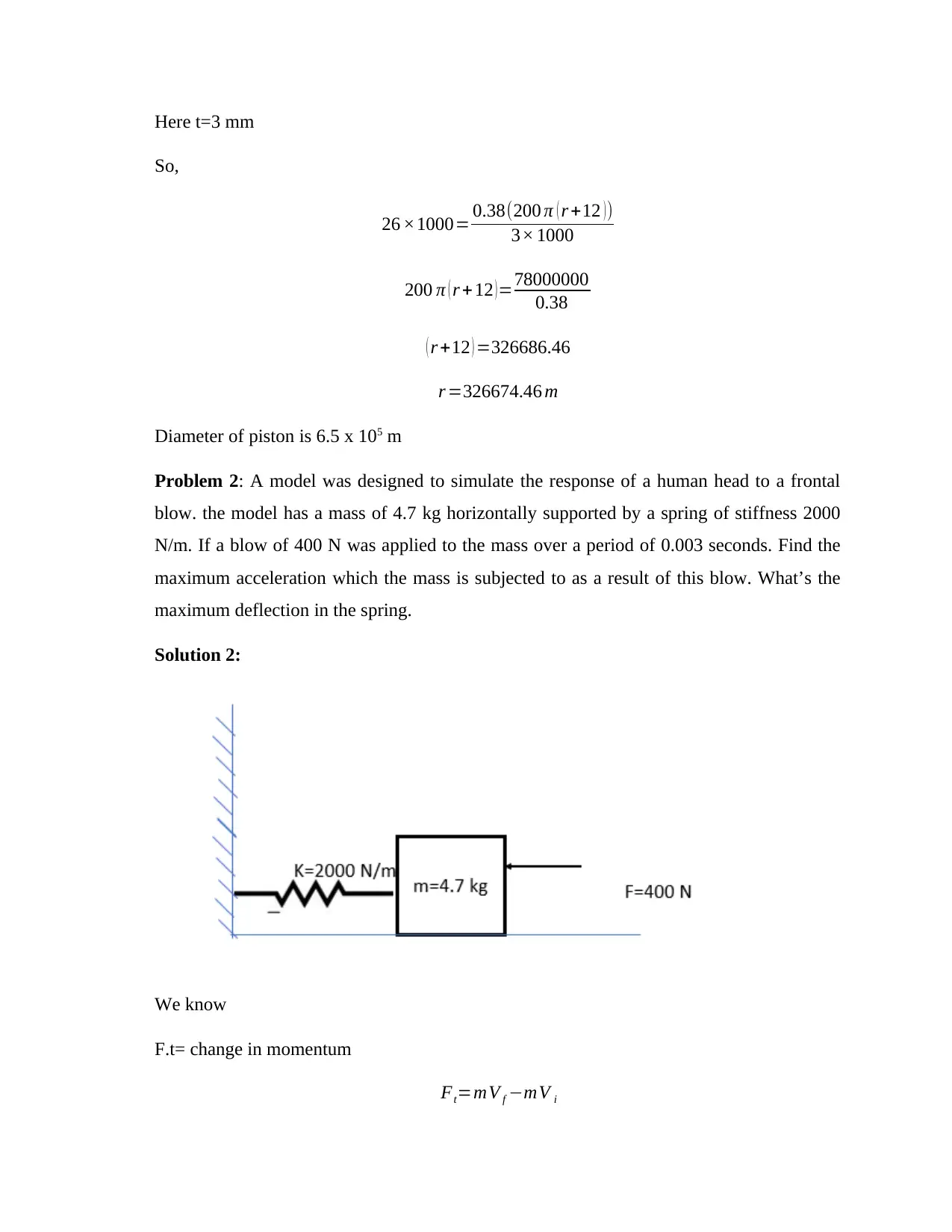
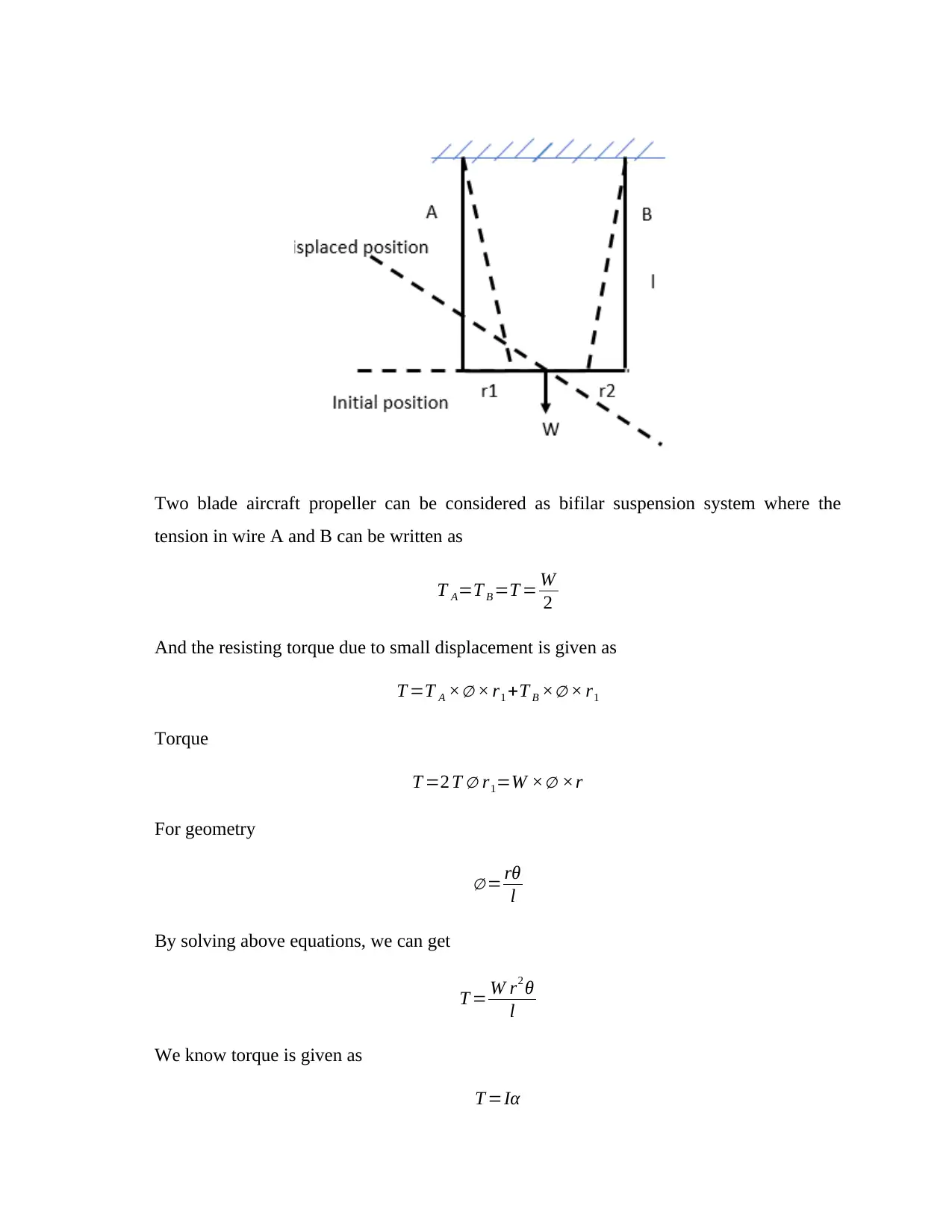
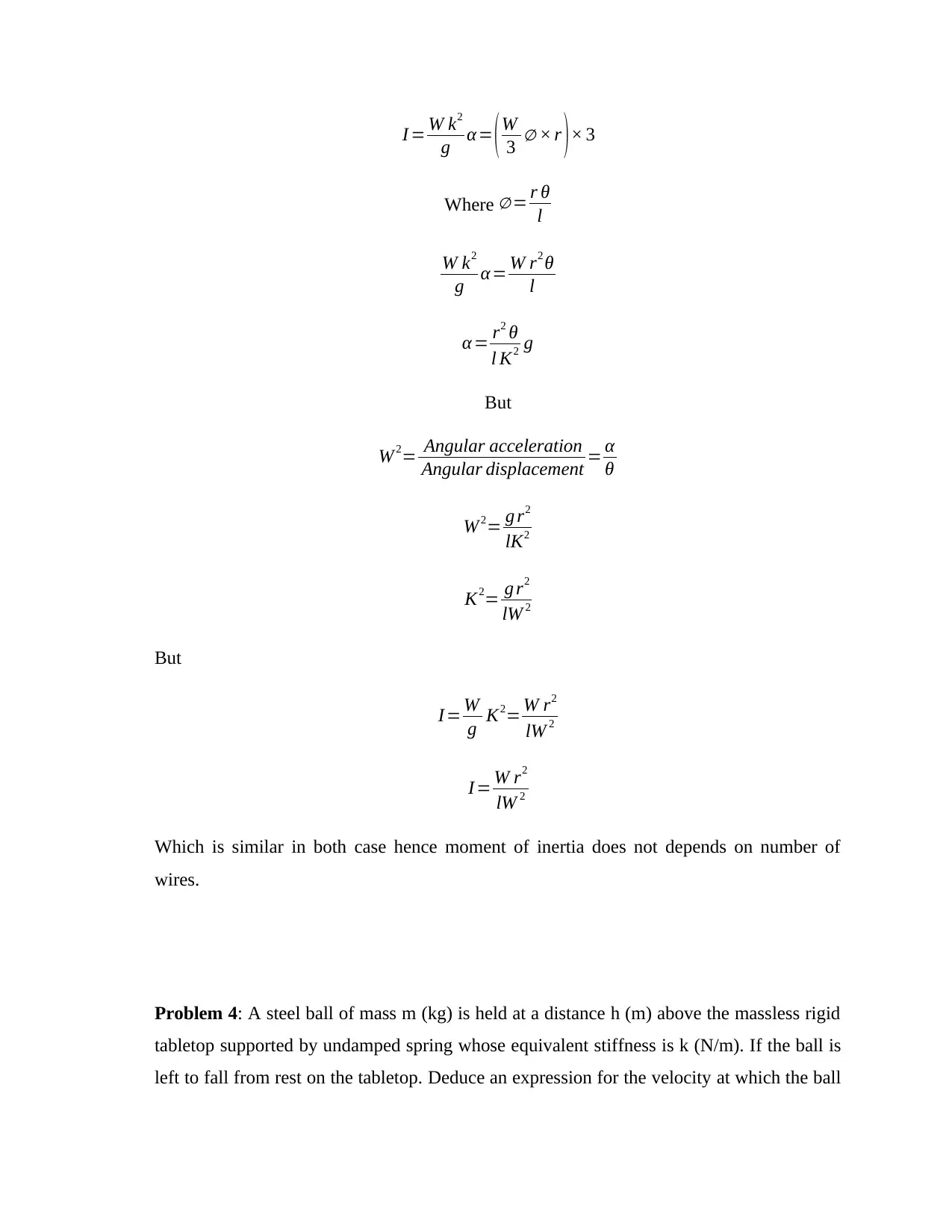
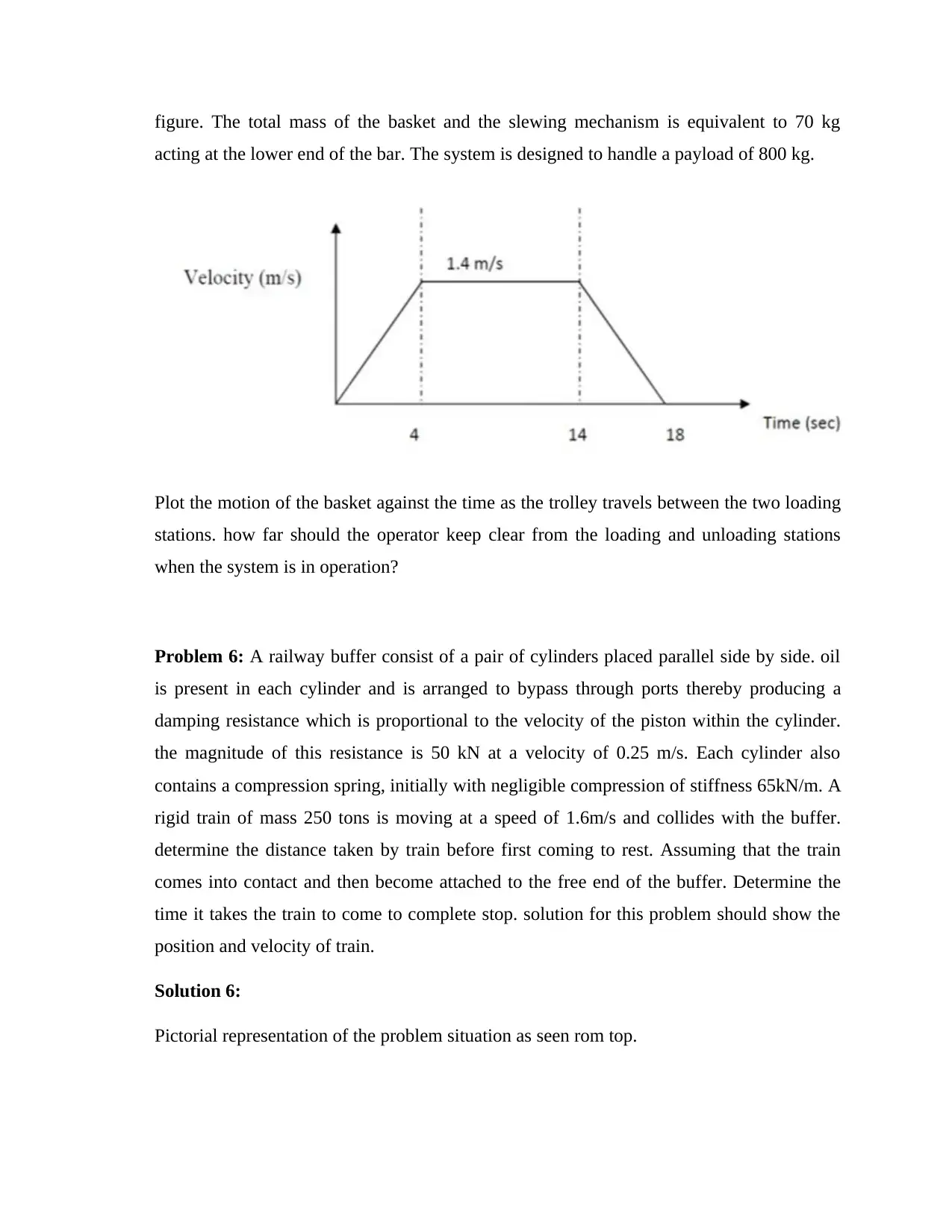
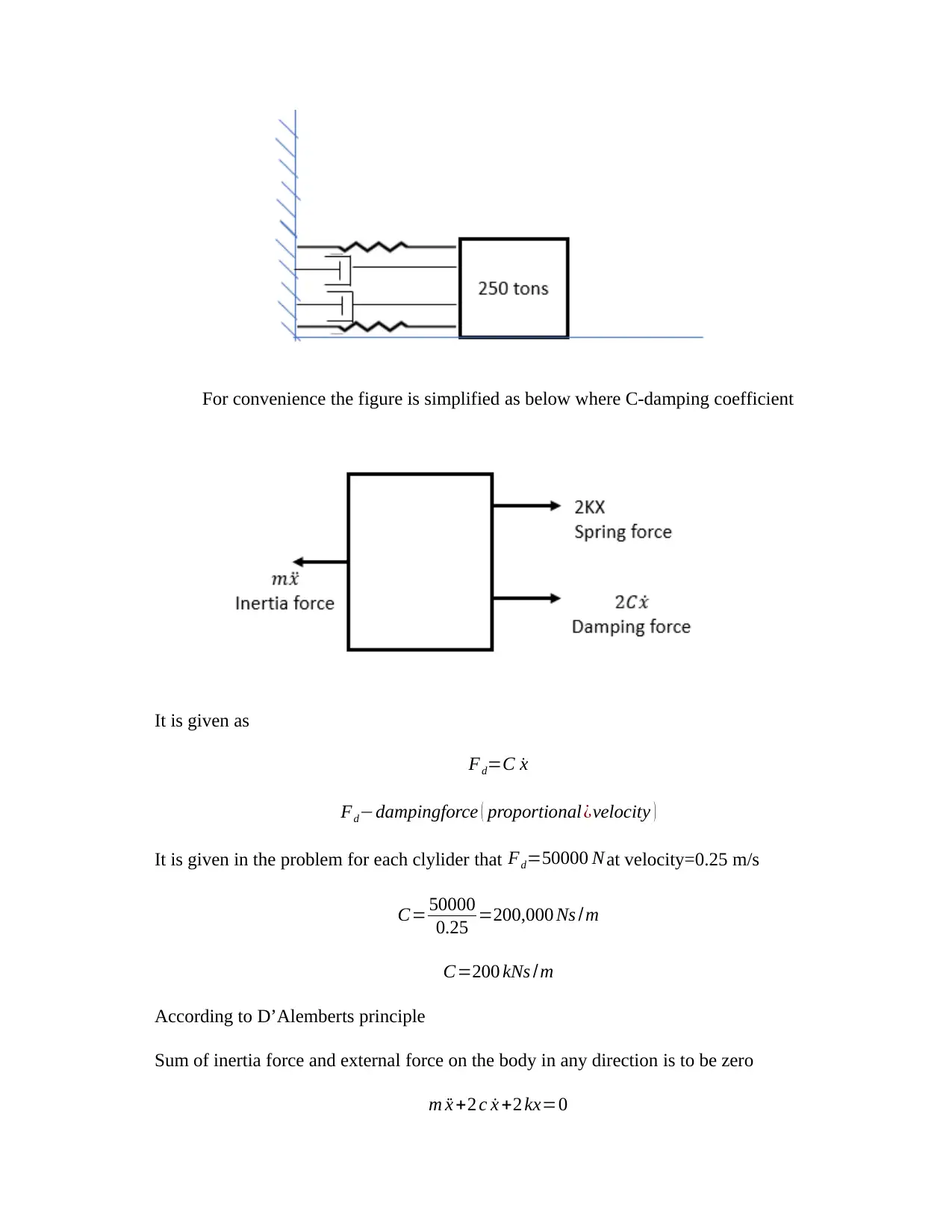
This mechanical engineering assignment presents solutions to a variety of problems covering different concepts in the field. The assignment begins with a problem on viscous dampers, calculating the piston diameter given damping constants and fluid properties. It then moves on to a model simulating a human head's response to a frontal blow, determining maximum acceleration and spring deflection. The assignment also includes a problem on determining the moment of inertia of aircraft propellers using bifilar suspension, and analyzes the behavior of a steel ball falling onto a spring-supported tabletop, deriving velocity expressions and discussing energy dissipation. Furthermore, the assignment presents problems related to a hoisting system and a railway buffer, analyzing the motion of a basket and the train's behavior during a collision, respectively. Finally, the assignment concludes with a problem on a door closing mechanism controlled by a spring and dashpot, deriving an expression for the door's motion and estimating the closing time. Each problem includes detailed step-by-step solutions, calculations, and explanations, making it a valuable resource for students studying mechanical engineering.
1 out of 16