Solved Problems in Mechanics and Thermodynamics

This document contains solved problems in mechanics and thermodynamics, including calculations related to beams, rafts, heat energy, and stress. The solutions are presented in a step-by-step format and cover topics such as moments, displacement, and Newton's laws of motion. The subject and course code are not mentioned.

ASSIGNMENT
Author
Department
Institution
City, Country
Address
Task 1
beam span=30 m
beam weight=10 kN /m=q1
uniformly distributed load =120 kN =q2 ( 8 m¿12 m)
Concentrated load=68 kN =p1 (at 12 m)
The sketch of the simply supported beam with the loads is
shown below:
We know that, the summation of moments about point A is 0.
That is, ∑ M A =0
∑ M A =−¿ q1 ( 30 ) ( 30
2 )−q2 ( 4 ) (8+ 4
2 )− p1 (12 )+RB ( 30 )=0 ¿
30 RB =450 q1+ 40 q2+12 p1
30 RB =450 (10)+40(120)+12(68)
RB= 4500+ 4800+816
30 = 10116
30 =337.2 kN
Also, the summation of moments about point B is 0. That is,
∑ M B=0
∑ M B=−RA (30 )+ ¿ q1 ( 30 ) (30−30
2 )−q2 ( 4 ) (22− 4
2 )+ p1 ( 18 )
30 RA =450 q1+ 80 q2 +18 p1
30 RA =450( 10)+80 (120)+18 (68)
RA = 4500+9600+1224
30 = 15324
30 =510.8 kN
Task 2
Total weight of cars 4 ×1600=6400 kg
weight of raft ¿ 4000 kg
mass of 1 barrel=15 kg
Density of foam=40 kg/m3
volume of 1barrel =π r2 h=π × 0.32 × 0.975=0.08775 π m3
mass of foam∈1 barrel=density × volume=40 × 0.08775 π =
Total mass of barrels=(3.51 π +15)kg × 70=1821.8893 kg
Total mass of raft =mass of raft +mass of barrels
¿ 1821.8893+4000=5821.8893 kg
Scenario 1: Empty raft
“According to the law of floatation, a floating object displaces
its own weight/mass.” Which implies that, the Mass of water
displaced equals 5821.8893 kg.
Volume of displaced water = mass
density =5821.8893
1029 =5.6578 m
S . A of raft base =7 ×6=42 m2

Height of water displaced=height of barrel displaced
¿ volume
S . A of raft base =5.6578
42 =0.1347 m
% displacement of barrels= 0.1347
0.975 × 100 %=13.82%
Scenario 2: Raft with the four cars on board
Mass of water displaced equals
( 5821.8893+6400 ) kg=12221.8893 kg
Volume of displaced water = mass
density =12221.8893
1029 =11.87744 m3
S . A of raft base =7 ×6=42 m2
Height of water displaced=height of barrel displaced
¿ volume
S . A of raft base =11.87744
42 =0.2828 m
% displacement of barrels= 0.2828
0.975 ×100 %=29 %
Task 3
We calculate the heat energy required to raise ice at −20 ℃
to steam at 130 ℃ in bits as follows.
First, energy required to raise 15kg of ice to 0 ℃
Specific heat of ice= 0.50 cal
g ℃
Heat required = 0.50 cal
g ℃ × ( 15 ×1000 ) g ×20 ℃=150000 cal=150 kcal
Second, energy required to melt 15kg of ice at 0 ℃
Latent heat of melting ice= 80 cal
g
Heat required= 80 cal
g × ( 15 ×1000 ) g=1200000 cal=1200 kcal
Third, energy required to raise 15kg of ice to 100 ℃
Specific heat of water =1 cal
g℃
Heat required=1 cal
g ℃ × ( 15 ×1000 ) g× 100 ℃=1500000 cal=
Then, energy required to vaporize 15kg of water at 100 ℃
Latent heat of boiling water= 540 cal
g
Heat required=540 cal
g × ( 15 ×1000 ) g=8100000 cal=8100
Finally, energy required to raise 15kg of steam to 130 ℃
Specific heat of steam= 0.48 al
g℃
Heat required= 0.48 cal
g ℃ × ( 15 ×1000 ) g ×30 ℃=216000 cal
Total heat required= ( 150+1200+1500+8100+216 ) kcal=11
We know that change in temperature results in a change in
volume of the material. As a result, a body exposed to
temperature variations develops stress. That is, expansion or
contraction stress.
Task 4
The free-body diagrams are shown below

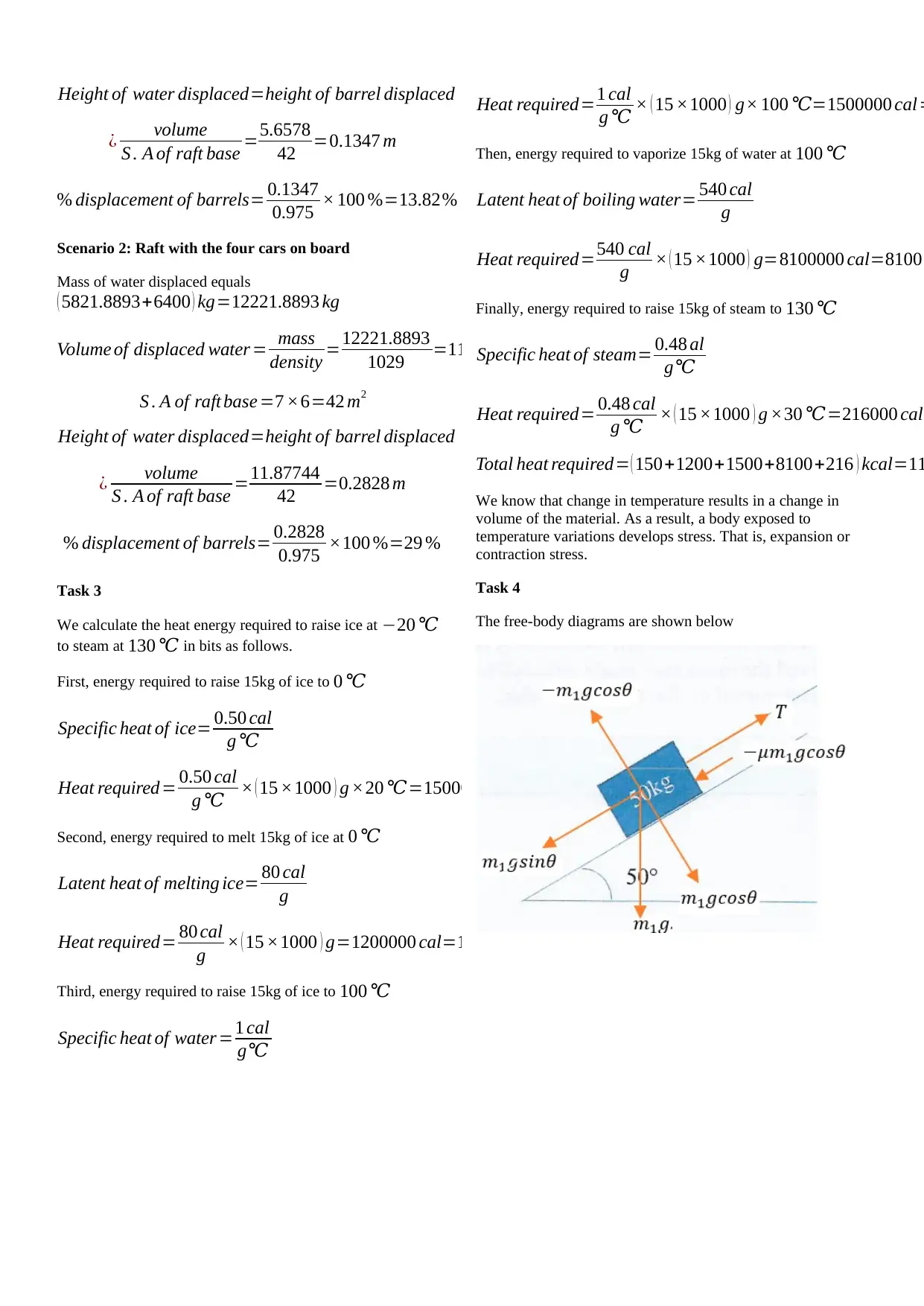
Let 50 kg mass=m1 and 10 kg mass=m2
Using Newton’s second law of motion and D’Alembert’s law
m2 g−T =m2 a …1 and
T −m1 gsinθ +μ m1 gcosθ=m1 a … 2
T =m2 g−m2 a
Substituting T into equation 2 we get:
m2 g−m2 a−m1 gsinθ+μ m1 gcosθ=m1 a
m1 a+m2 a=μ m1 gcosθ+ m2 g−m1 gsinθ
a= μ m1 gcosθ +m2 g−m1 gsinθ
(m¿¿ 1+m2)¿
All the forces shown on the free body diagrams above are
calculated as follows
μ m1 gcosθ=0.38 ×50 ×9.81 cos 50=119.8092 N
m2 g=10 ×9.81=98.1 N
m1 gsinθ=50 × 9.81sin 50=375.7448 N
a= 119.8092+ 98.1−375.7448
(50+10) =−157.8356
60 =2.6306 m s−2
T =m2 g−m2 a=m2 ( g−a )=10 ( 9.81− (−2.6306 ) )=124.406 N
Given that time=2 seconds ,
a= Final Velocity−Initial Velocity
time = V f −V i
t
But initial velocity=V i=0 so that
a= V f
t
V f =a × t=−2.6306 ×2=−5.2612m s−1
Displacement=V i t+ 1
2 at2=0+1
2 at2 =1
2 (−2.6306 ) ( 2 )2=−5.2
The negative sign in velocity, acceleration and displacement
denotes that the 10kg mass moves up.