Multirate Signal Processing Assignment: Problem Solutions

This document presents a solved assignment on multirate signal processing, addressing several key problems. The first question utilizes the noble identity to demonstrate an efficient implementation of a down-sampling process involving a filter. The second question involves drawing the discrete-time F...Read More

Multirate 1
Multirate signal processing
By
(Name)
(Course)
(Professor’s Name)
(Institution)
(State)
(Date)

Multirate 2
Question.1
Noble identity z- transform for down sampling is given by;
Ck [ n ] =δu ∨n [ n ] = 1
K ∑
k=0
k −1
e
j 2 kπn
K
X k [ n ]= {x [n ] k [ n ]
0 k ≠ n =Ck [ n ] x [ n ]
X k [ z ]=∑
n
X k [ n ] z−n= 1
K ∑
n
∑
k=0
k−1
e
j 2 πkn
K x [ n ] z−n= 1
K ∑
k=0
k−1
∑
n
x [n](e
j 2 πkn
K z)−n
¿ 1
K ∑
k=0
k−1
x (¿ e
− j2 πkn
K z )¿
Thus
X k ( z )=Y ( zk)
Y ( z )=X k (z
1
k )= 1
K ∑
k =0
k−1
x(e¿¿ − j 2 πkn
K z
1
k )¿
Given;
y [ n ] = ( ↓ 3 ) {h [ n ]∗x [n]} The Z-transform Y (Z) is given as;
Y ( Z )= ( ↓3 ) { H ( Z )∗X ( Z ) } But H ( Z )=1−z−1
1−z−3
Substituting, we get;Y ( Z )= ( ↓3 ) {1−z−1
1− z−3 ∗X ( Z ) } taking inverse z transform
y [ n ] = 1
1−z−1 ∗{ ( ↓ 3 ) ( 1−z−1∗x [ n ] ) } =h1 [ n ]∗{ ( ↓3 ) (h2 [ n ]∗x [n ])}
Hence the process can efficiently be implemented as shown.
Question.2
The discrete time Fourier transform of a signal is given by;
X ( w )=∑
−∞
∞
x (n)e− jwn =∑
− π
12
π
12
e− jwn
But w=π /12

Multirate 3
y1 ( w ) = 1
6 π ∑
−π / 12
π /12
e− jwn= 1
6 π e− jπ / 12n
| π /12
−π /12
y2 ( w ) = 2
π ∑
−π / 12
π /12
e− jwn= 2
π e− jπ / 12n
| π /12
−π /12
y1 ( )
Question.3
For interpolation, the cutoff frequency ¿ 2∗π /4
It takes into interpretation that after the interpolation procedure the signal is made up of (L–1)
zero coefficients, and the decimation progression infers that only one out of every M samples is
needed at the productivity of the converter. To ensure the system is more resourceful, the low-
pass filter in is substituted with a bank of filters organized in parallel. The sampling-rate
π /12−π /12

Multirate 4
conversion procedure is carried out by the multiplexer at the output by picking out every MT
L
samples.
From the Nyquist sampling theorem;
f sm= 1
MT = f s
M
Ωstop=2 π ( f s
2 M )T = π
M radians
f max< f s
2 M
Y z=H z Xz
Question.4
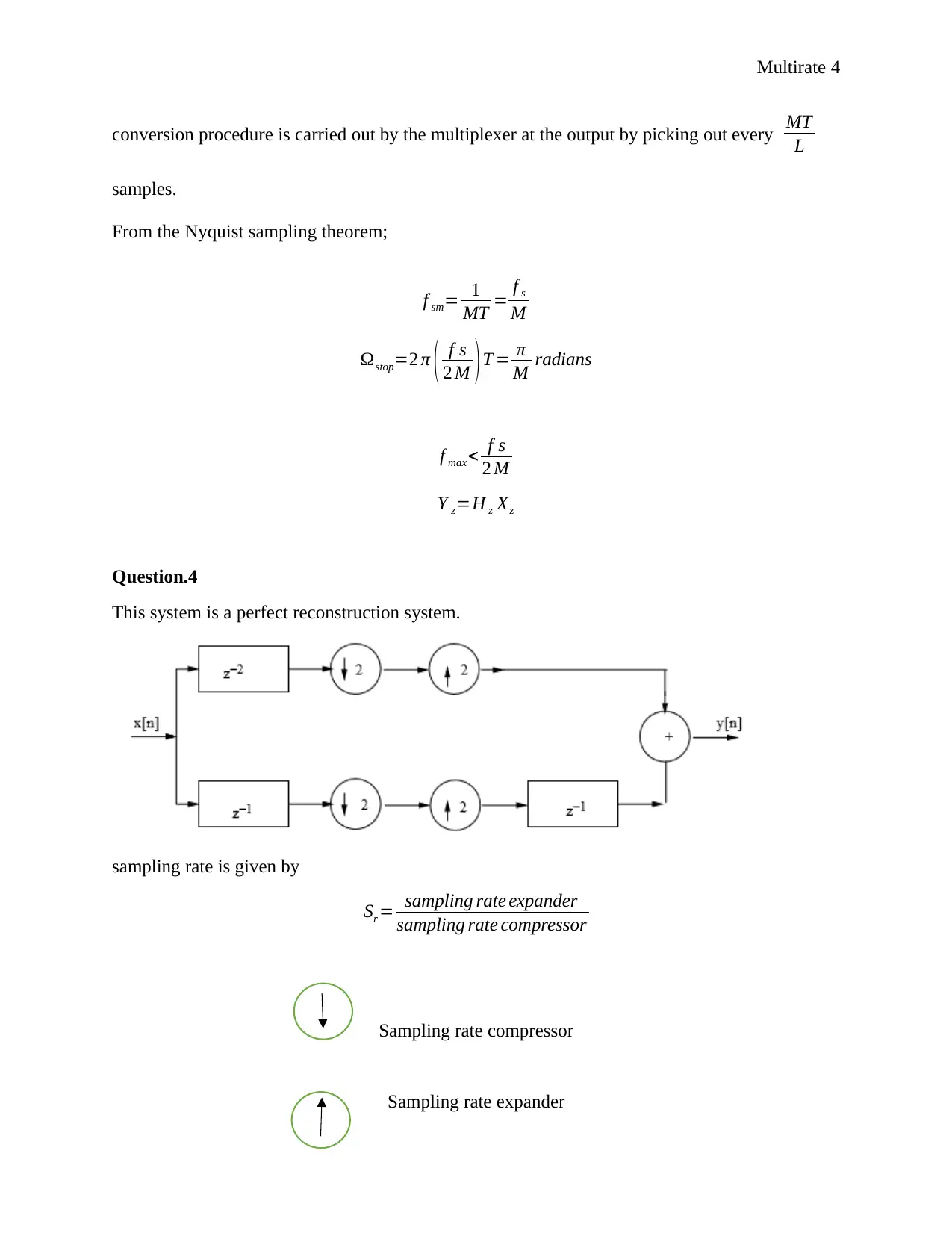
This system is a perfect reconstruction system.
sampling rate is given by
Sr = sampling rate expander
sampling rate compressor
Sampling rate compressor
Sampling rate expander

Multirate 5
Channel 1
z−2 x 2
2=z−2
Channel 2
z−1 x z−1 x 2
2 =z−2
The transfer function
z−2+ z−2
z−2 z−2 =2 z2
2 z2 >0
Thus, the system is perfectly reconstructive
Question.5
Determine the transfer function,
The ratio between the output and the input
h(z )= Y (z )
X(z)
The systems comprise of an expander (L) and compressor (M)
X(z )h(z )=Y (z )
For the blocks in series, the transfer function is given by the product of their values
h(z )=9 z−3

Multirate 6
Reference
Bandyopadhyay, B., & Janardhanan, S. (2006). Discrete-time Sliding Mode Control: a Multirate
Output Feedback Approach. Berlin Heidelberg, Springer-Verlag. Available from:
http://dx.doi.org/10.1007/11524083. [Accessed Date: 3rd May 2018].
Dolecek, G. J. (2018). Advances in Multirate Systems. Cham, Springer.
Fliege, N. (2005). Multirate digital signal processing: multirate systems, filter banks, wavelets.
Chichester, Wiley
Harris, F. (2008). Multirate signal processing for communication systems. Upper Saddle River,
N.J., Prentice Hall PTR.
Proakis, J. G., & Manolakis, D. G. (2014). Digital signal processing. Harlow, Essex, Pearson.
SPA (Conference: Institute of Electrical and Electronics Engineers). (2015). SPA 2015: Signal
Processing, Algorithms, Architectures, Arrangements, and Applications: conference
proceedings: Poznan, 23-25th September 2015. Available from:
http://ieeexplore.ieee.org/servlet/opac?punumber=7360272. [Accessed Date: 3rd May 2018]
Stranneby, D. (2001). Digital signal processing: DSP and applications. Oxford, Newnes.
Available from: http://site.ebrary.com/id/10186537. [Accessed Date: 3rd May 2018]
Yaroslavsky, L. (2011). Digital Signal Processing in Experimental Research Volume 1: Fast
Transform Methods in Digital Signal Processing. Sharjah, Bentham Science Publishers.
Available from: http://public.eblib.com/choice/publicfullrecord.aspx?p=864334. [Accessed Date:
3rd May 2018].

Multirate 7