SEO Expert for Desklib

Desklib is an online library that provides study material including solved assignments, essays, dissertations, and more. It offers a wide range of content for various subjects and courses. Whether you need help with assignments or want to explore different topics, Desklib has you covered.

Name…………………………………………………………. Personal Number……………
Problem 1
Solution
Suppose X maps W to R ,meaning the w ∈ Rand also X ( w)∈ R
Now Y(w)=X |w|3 also maps¿ Y : W → R
Now themeasure for cumulative distribution function will be as below
F ( x )=P ( X ≤ x )
We also consider y> 0
FY ( y )=P (Y ≤ y )
¿ P (|x|3 ≤ y )
¿ P(− 3
√ y )≤ X ≤− 3
√ y
Now
¿ FX (−3
√ y )−FX (−3
√ y )+P(X =−3
√ y)
since X is a random variable ,the |x|3 is alsoa random variable on the same space .
Problem 2
Part a
Solution
Using the formula∬
−∞
∞
f ( x , y ) dydx
E ( x , y ) =∫
0
2
∫
0
2
c ( y2 + xy ) dydx
∫
0
2
∫
0
2
c ( y2 + xy ) dydx=1

∫
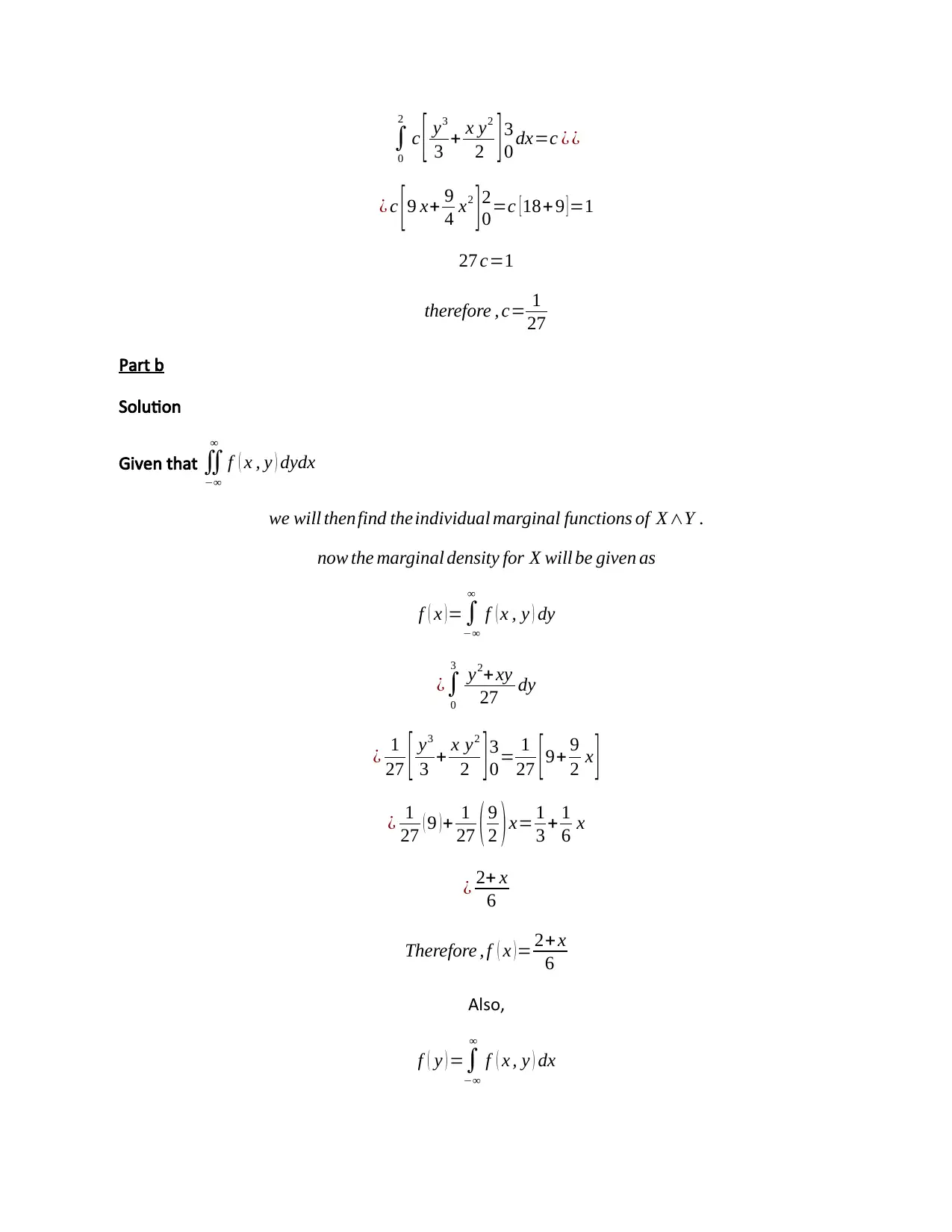
0
2
c [ y3
3 + x y2
2 ] 3
0 dx=c ¿ ¿
¿ c [ 9 x+ 9
4 x2
] 2
0=c [ 18+ 9 ] =1
27 c=1
therefore , c= 1
27
Part b
Solution
Given that ∬
−∞
∞
f ( x , y ) dydx
we will thenfind theindividual marginal functions of X∧Y .
now the marginal density for X will be given as
f ( x ) =∫
−∞
∞
f ( x , y ) dy
¿∫
0
3
y2+ xy
27 dy
¿ 1
27 [ y3
3 + x y2
2 ] 3
0= 1
27 [ 9+ 9
2 x ]
¿ 1
27 ( 9 )+ 1
27 ( 9
2 )x= 1
3 + 1
6 x
¿ 2+ x
6
Therefore , f ( x )= 2+ x
6
Also,
f ( y ) =∫
−∞
∞
f ( x , y ) dx

¿ 1
27 ∫
0
2
f ( y2+ xy ) dx
¿ 1
27 [ x y2+ x2 y
2 ] 2
0= 1
27 [ 2 y2+2 y ]
Therefore , f ( y ) = 2 y2+ 2 y
27
¿ the above obtained solutions , we can observe that f ( x , y ) ≠ f ( x ) f ( y )
Now we can confidently concludethat X ∧Y are not independent .
We now want ¿ calculte the xpected values ;
Expectedd values of 3 X −2 y is given by E [ 3 X −2Y ] =3 E [ X ] −2 E [ Y ] … … . A
Expected values of X will be givenby
E [ X ] =∫
−∞
∞
xf ( x ) dx
¿∫
0
2
1
6 x ( 2+x ) dx= 1
6 ∫
0
2
2 x + x2 dx
¿ 1
6 [x2 + x3
3 ]2
0= 1
6 (4+ 8
3 )=10
9
Therefore , E ( x ) =10
9
Also ,
E [ Y ] =∫
−∞
∞
yf ( x , y ) dy
¿∫
0
3
y ( 2 y2 + y
27 )dy = 2
27 ∫
0
3
( y3 + y2 ) dy
¿ 2
27 [ y4
4 + y3
3 ]3
0= 2
27 [ 81
4 + 27
3 ]=13
6
Therefore , E [ Y ] =13
6

Now ¿ our definition ¿ equation A
E [ 3 X−2 Y ]=3 ( 10
9 )−2 ( 13
6 )=10
3 − 13
3 =−1
Therefoe , E [ 3 X−2 Y ] =−1
Covariance
We wil consider a general formula for the covariance
Var [ X +Y ] =E [ ( X +Y ) 2 ] − [ E [ X +Y ] ] 2
Therefore , Var ( ax+ by ) =E [ ( ax +by ) 2 ] − [ E [ ax +by ] ] 2
¿ E [ a2 x2+ b2 y2 +2 abxy ]−¿
¿ a2 E [ x2 ]+b2 E [ Y 2 ] +2 ab [ XY ]−a2 x2−b2 y2−2 ab x y
Therefore , Var ( ax+by )=a2 σ2 x+b2 σ2 y2+2 abCxy … … … Equation B
Now we need ¿ find the σ 2 x ,σ 2 y ∧Cxy
E [ x2 ] =∫
−∞
∞
x2 f ( x ) dx=∫
0
2
x2 ( 2+ x
6 ¿¿) dx ¿ ¿
¿ 1
6 ∫
0
2
( 2 x2+x3 ) dx= 1
6 ( 16
3 + 4 ) = 8
9 + 2
3 = 14
9
E [ x2 ]=14
9
Var ( x )=σ2 x=E [ x2 ]− [ E [ x ] ]2
¿ ( 14
9 )− ( 10
9 )
2
¿ 14
9 − 100
81 =26
81
Hence , σ2 x=0.32099
E [ Y 2 ] =∫
−∞
∞
y2 f ( x ) dy=∫
0
3 y2 ( 2 y2 +2 y )
27 dy

¿ 1
27 ∫
0
3
( 2 y4 +2 y3 ) dy= 1
27 [ 2 y5
5 + 2 y4
4 ] 3
0
¿ 1
27 [ 486
5 + 81
2 ]= [ 18
5 + 3
2 ] = 51
10
Var ( y )=σ 2 y=E [ y2 ]− [ E [ y ] ]2
¿ 51
10 −( 13
6 )
2
= 73
180
hence , σ2 y =0.4056
The Covariance Cxy=E [ xy ] −x y
E [ XY ] =∫
−∞
∞
∫
−∞
∞
xyf ( x , y ) dydx
¿∫
0
2
∫
0
3 xy ( y2 + xy )
27 dydx
¿∫
0
2
∫
0
3
1
27 ( x ( y4 )
4 + x2 y2
)dydx
¿∫
0
2
1
27 [ 81
4 +9 x2
]3
0 dx
¿∫
0
2
( 3
4 x +9 x2
) dx
¿ [ 3
4 ( x2
2 ) + 1
3 ( x3
3 ) ] 2
0
¿ [ 3
2 + 8
9 ]= 43
18
E [ X , Y ] = 43
18 =2.3889
Therefore , Cxy= 43
18 −( 10
9 )( 13
6 )=−1
54
Hence Cxy=−0.01852

Now finding the variance of 3 X −2 Y ¿ equation B
But , we are given a=3∧b=−2
Therefore , Var ( 3 X −2 Y ) = ( 3 ) 2 ( 0.3210 ) + ( −2 ) 2 ( 0.4056 ) +2 ( 3 ) ( −2 ) ( −0.0185 )
¿ 4.7334
Part c
Solution
P [ X ≤|Y =1 ]=∫
−∞
1
2
f ( x , y ) dx
fy(1) dx∨ y =1
¿∫
0
1
2 y2 + xy
27
fy(1) dx aty=1
¿∫
0
1
2 ( 1+ x
27 )dx
f y (1)
¿ 1
27 [x + x2
2 ] 1
2
0
× 9
1
¿ 1
27 [ 0.5+0.125 ] × 9
¿ 5
24 =0.20833
Therefore , P [ X ≤ 1
2|Y =1 ]=0.20833
Part d
Solution
The conditional expected value of P [ Y |X=1 ] is givenby
P [ Y |X=1 ]=∫
−∞
∞
yf ( y
x =1 )dy wheref ( y|x=1 )= f (x , y)
f ( x )∨x=1

¿
( y ¿¿ 2+ xy ) 1
27
( 2
27 + x
27 ) when x=1
¿
¿ y2+ xy
27 × 9
1 = y2
3 + xy
3
Applyingexp ected value formula
E [ P [ Y ]|x=1 ] =∫
−∞
∞ y ( y2+ xy )
3 dy∨x =1
¿∫
0
3
y3+ y2
3 dy
¿ [ y4
4 + y3
9 ]3
0=81
4 +3
1 = 93
4 =23.25
Problem 4
Solution
Given that
Y = { x if |x|≤ a ⇒ a=0
−x if |a|<a ⟹ a=1 }since a >0
Now to show that the distribution has a standard distribution, we will compare
P ( Y ≤ x ) with P ( X ≤ x )
Starting with
F ( Y ) =P ( Y ≤ y )
¿ P ( Y ≤ y ;|x|=0 ) + P ( Y ≤ y ;|x|=1 )
¿ P ( X ≤ y ;|x |=0 ) + P ( −X ≤ y ;|x |=1 )
The ∑ of the individual probabilites should be 1
P ( X ≤ y ) ; p (|x|0 ) + p (−X ≤ y ; P (|x|=1 ) )
Now addingthe above probabilities

¿ 1 ¿
where 1is the∑ of probabilites of the two variables ¿
¿ P ( X ≤ y )
The variable x is not symmetric at any point
Fx ( y ) ∧f (x )≠ f (−x)
Therefore, the variable x and y do not have the same distribution function.
Since the variables don not share same function, Y has no standard normal distribution.
Problem 5
Given the function
f ( x ) = λ x−1 ( λ+1 ) for x ≥ 1
Looking at the given function above , we can confidently see say our function increases
supposethat t ∈ [ 0 , ∞ ) then
P ( Y ≤ t )=P ( x ≤ et )
¿ 1−e−λ ( λ x−1 ( λ+1 ) )
But y=lnx
then x=e y
¿ 1−e−( ( λ2 x−λ ) )
¿ 1−e−λ2 (X− ( λ+1 ) )
Now let the exponential distributionbe denoted by
Q ( x )=1−e−qx whereQ ( x ) is the function that decreases .
¿ 1−e−λ2
. X−( λ+1 ) is the exponetial function of th parameter λ .
Problem 6
Solution

A A charactiristic function of anr random variable is defined by :
Ψ ( t ) =∫
0
∞
( eitu ) dF ( u )
Ψ ( t )=∫
0
∞
( eitu ) f (u)d ( u )
Now given that x >0 , then functionwould be Ψ ( 0 )=1
dn Ψ ( t )
d tn but t=0 ∈ ( X n )
Ψ n =∈ ( X n )
The new function would be
Ψn ( t ) =∫
0
∞
Ψ ( tu ) + f ( u ) F (u)
Ψn ( t )=∫
0
∞
Ψ ( tu )+f ( u ) d (u)
As seen above the new characteristic function satisfies every condition for characteristic function
of a non-negative random variable.
Ψn ( t ) =∫
0
∞
Ψ ( tu ) dF (u)
Ψn ( t )=∫
0
∞
Ψ ( tu ) f (u ) d( u)
Ψ ' n ( t ) = d
dt ∫
0
∞
Ψ ( tu ) uf ( u ) d (u)
Now
Ψ m
n ( t ) = dm
d tm ∫
0
∞
Ψ ( u ) ( u ) m f ( u ) du
We can cle arly see that

Ψ ( 0 ) =∫
0
∞
Ψ ( 0 ) dF ( u )
Ψ ( 0 ) =∫
0
∞
( 1 ) f (u)d ( u )
Ψ ( 0 ) =1
Ψ m
n ( 0 ) =∫
0
∞
Ψ ( 0 ) um f ( u ) du
Ψ m
n ( 0 )=∫
0
∞
( 1 ) um f ( u ) du
Ψ m
n ( 0 ) =∫
0
∞
um f ( u ) d (u)
Ψ m
n ( 0 ) =∈(um )
Th erefore , Ψ ( t ) =∫
0
∞
etu f ( u ) du
sinceu >0 , thenfollows that um >0
It can be see that the new function Ψ ( t ) =∫
0
∞
etu f ( u ) dusatisfies all functions of the random
variable.
Problem 7
Solution
W e would solve this ¿ a known charateristic funtion of a prob . distribution P(x)
The function isexp (itz)
The function of the of P ( z ) will be givenby
Φ ( t )=E [ exp ( itz ) ]

Φ ( t ) =∫
−∞
∞
exp ( itz ) P ( z ) dz
Φ ( t )=∫
−∞
∞
e (itz ) .( 1
−z−3 ¿)dz+∫
1
∞
e
( itz )
( 1
z )3
dz ¿
Φ ( t ) =∫
1
∞
e− ( itz ) × ( 1
z3 ) +∫
1
∞
e
( itz ) × ( 1
z )
3
dz
Φ ( t ) =∫
1
∞
e− ( itz ) × ( 1
z3 ) +∫
1
∞
e
( itz ) × ( 1
z3 )
❑
dz
Φ ( t )= ( 1−t2 ) ׿
Therefore, the characteristic function has been proved.
Part b
Solution
We have to consider CLT
If the vraiables X 1 , X 2 , … Xn
Then
s
√ n → N ( 0,1 ) as n approaches ∞from the right
s
√ (nlogn) → N ( 0,1 ) asn approaches ∞ ¿ ¿
Now
lim
n → ∞
( √ n ) = lim
n→ ∞
¿ ¿
Thereby , proved .