Numeracy 2 (MAII3007) Coursework Portfolio
VerifiedAdded on 2023/06/15
|18
|2992
|121
AI Summary
This is the Numeracy 2 e-portfolio for the semester commencing February 2018 (Spring 2018). It consists of two sections: Section 1 is worth 75% of the final mark and consists of 8 questions (70%) and periodic Skills Audit (carrying 5%). Section 2 consists of 3 tasks. Combined they are worth 25% of the final mark. Get solved assignments, essays, dissertations for Numeracy 2 (MAII3007) Coursework Portfolio at Desklib.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

Student Name:
Student ID Number:
Tutor name:
This is your Numeracy 2 e-portfolio which you must submit by Wednesday 25th
April 2018 via the Student Portal.
Please read this carefully
Numeracy 2 (MAII3007) Coursework Portfolio
February 2018
Student ID Number:
Tutor name:
This is your Numeracy 2 e-portfolio which you must submit by Wednesday 25th
April 2018 via the Student Portal.
Please read this carefully
Numeracy 2 (MAII3007) Coursework Portfolio
February 2018
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

This is your Numeracy 2 e-portfolio for the semester commencing February 2018 (Spring 2018). Please
save a copy on your computer and back it up regularly (e.g. by saving it on your computer / in the cloud
(e.g. Google Drive) / emailing it to yourself. You should print a working copy and bring it to all lectures
and tutorials. However, at the end of the course, you will need to submit a completed electronic copy.
Please read carefully the module handbook, the marking criteria and the grade descriptors.
Academic Misconduct
You are responsible for ensuring you understand the policy and regulations about academic
misconduct. You must:
• Complete this work alone except where required or allowed by this assignment briefing paper and
ensure it has not been written or composed by or with the assistance of any other person.
• Make sure all sentences or passages quoted from other people’s work in this assignment (with or
without trivial changes) are in quotation marks, and are specifically acknowledged by reference to
the author, work and page.
This portfolio consists of two sections:
Section 1is worth 75% of the final mark and consists of 8 questions (70%) and periodic Skills
Audit (carrying 5%).
Section 2consists of 3 tasks. Combined they are worth 25% of the final mark.
Task 1 – Two Real life examples (8%)
Task 2 – Online Activity (10%)
Task 3 – Reflective log (7%)
save a copy on your computer and back it up regularly (e.g. by saving it on your computer / in the cloud
(e.g. Google Drive) / emailing it to yourself. You should print a working copy and bring it to all lectures
and tutorials. However, at the end of the course, you will need to submit a completed electronic copy.
Please read carefully the module handbook, the marking criteria and the grade descriptors.
Academic Misconduct
You are responsible for ensuring you understand the policy and regulations about academic
misconduct. You must:
• Complete this work alone except where required or allowed by this assignment briefing paper and
ensure it has not been written or composed by or with the assistance of any other person.
• Make sure all sentences or passages quoted from other people’s work in this assignment (with or
without trivial changes) are in quotation marks, and are specifically acknowledged by reference to
the author, work and page.
This portfolio consists of two sections:
Section 1is worth 75% of the final mark and consists of 8 questions (70%) and periodic Skills
Audit (carrying 5%).
Section 2consists of 3 tasks. Combined they are worth 25% of the final mark.
Task 1 – Two Real life examples (8%)
Task 2 – Online Activity (10%)
Task 3 – Reflective log (7%)
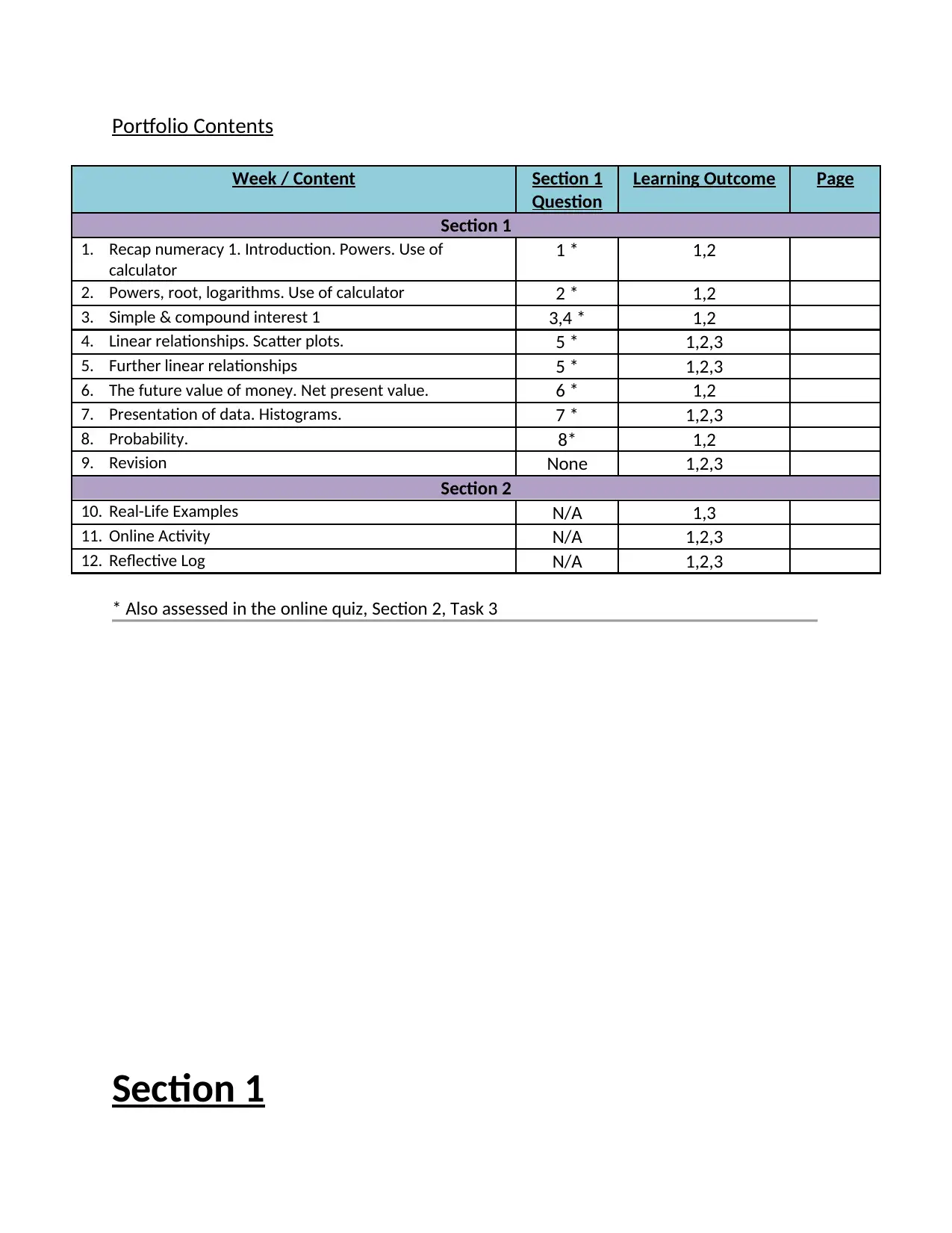
Portfolio Contents
Week / Content Section 1
Question
Learning Outcome Page
Section 1
1. Recap numeracy 1. Introduction. Powers. Use of
calculator
1 * 1,2
2. Powers, root, logarithms. Use of calculator 2 * 1,2
3. Simple & compound interest 1 3,4 * 1,2
4. Linear relationships. Scatter plots. 5 * 1,2,3
5. Further linear relationships 5 * 1,2,3
6. The future value of money. Net present value. 6 * 1,2
7. Presentation of data. Histograms. 7 * 1,2,3
8. Probability. 8* 1,2
9. Revision None 1,2,3
Section 2
10. Real-Life Examples N/A 1,3
11. Online Activity N/A 1,2,3
12. Reflective Log N/A 1,2,3
* Also assessed in the online quiz, Section 2, Task 3
Section 1
Week / Content Section 1
Question
Learning Outcome Page
Section 1
1. Recap numeracy 1. Introduction. Powers. Use of
calculator
1 * 1,2
2. Powers, root, logarithms. Use of calculator 2 * 1,2
3. Simple & compound interest 1 3,4 * 1,2
4. Linear relationships. Scatter plots. 5 * 1,2,3
5. Further linear relationships 5 * 1,2,3
6. The future value of money. Net present value. 6 * 1,2
7. Presentation of data. Histograms. 7 * 1,2,3
8. Probability. 8* 1,2
9. Revision None 1,2,3
Section 2
10. Real-Life Examples N/A 1,3
11. Online Activity N/A 1,2,3
12. Reflective Log N/A 1,2,3
* Also assessed in the online quiz, Section 2, Task 3
Section 1

This section should be filled in as you acquire the skills required for each question.
Answer all questions. Please show your workings and/or explain your results as required.
Marks will be awarded for good presentation. Please evaluate your progress using the skills
audits provided.
You may use your calculator as required.
You must show your working.
QUESTION 1 [6 marks]
Powers and Roots:
a) Simplify 75 x 72(2 marks)
b) Simplify 103 ÷102 (2 marks)
c) Evaluate ( 123 )4 (2 marks)
[TYPE YOUR ANSWER HERE]
a) 75×73 = 75+3 = 78 .
b) 103 ÷ 102 = 101 = 10
c) (123)4= 1212
Answer all questions. Please show your workings and/or explain your results as required.
Marks will be awarded for good presentation. Please evaluate your progress using the skills
audits provided.
You may use your calculator as required.
You must show your working.
QUESTION 1 [6 marks]
Powers and Roots:
a) Simplify 75 x 72(2 marks)
b) Simplify 103 ÷102 (2 marks)
c) Evaluate ( 123 )4 (2 marks)
[TYPE YOUR ANSWER HERE]
a) 75×73 = 75+3 = 78 .
b) 103 ÷ 102 = 101 = 10
c) (123)4= 1212
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
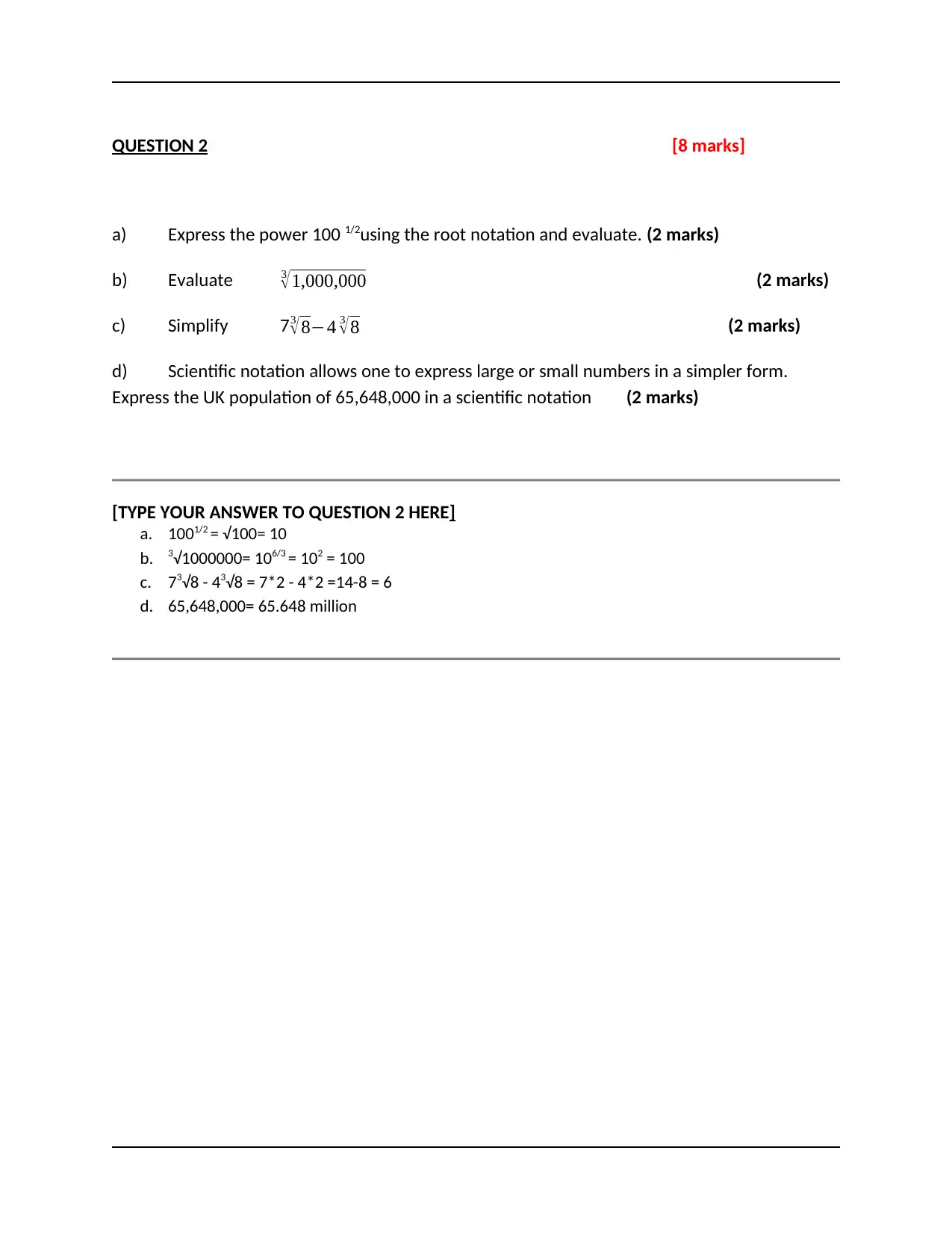
QUESTION 2 [8 marks]
a) Express the power 100 1/2using the root notation and evaluate. (2 marks)
b) Evaluate 3
√ 1,000,000 (2 marks)
c) Simplify 7 3
√ 8−4 3
√ 8 (2 marks)
d) Scientific notation allows one to express large or small numbers in a simpler form.
Express the UK population of 65,648,000 in a scientific notation (2 marks)
[TYPE YOUR ANSWER TO QUESTION 2 HERE]
a. 1001/2 = √100= 10
b. 3√1000000= 106/3 = 102 = 100
c. 73√8 - 43√8 = 7*2 - 4*2 =14-8 = 6
d. 65,648,000= 65.648 million
a) Express the power 100 1/2using the root notation and evaluate. (2 marks)
b) Evaluate 3
√ 1,000,000 (2 marks)
c) Simplify 7 3
√ 8−4 3
√ 8 (2 marks)
d) Scientific notation allows one to express large or small numbers in a simpler form.
Express the UK population of 65,648,000 in a scientific notation (2 marks)
[TYPE YOUR ANSWER TO QUESTION 2 HERE]
a. 1001/2 = √100= 10
b. 3√1000000= 106/3 = 102 = 100
c. 73√8 - 43√8 = 7*2 - 4*2 =14-8 = 6
d. 65,648,000= 65.648 million
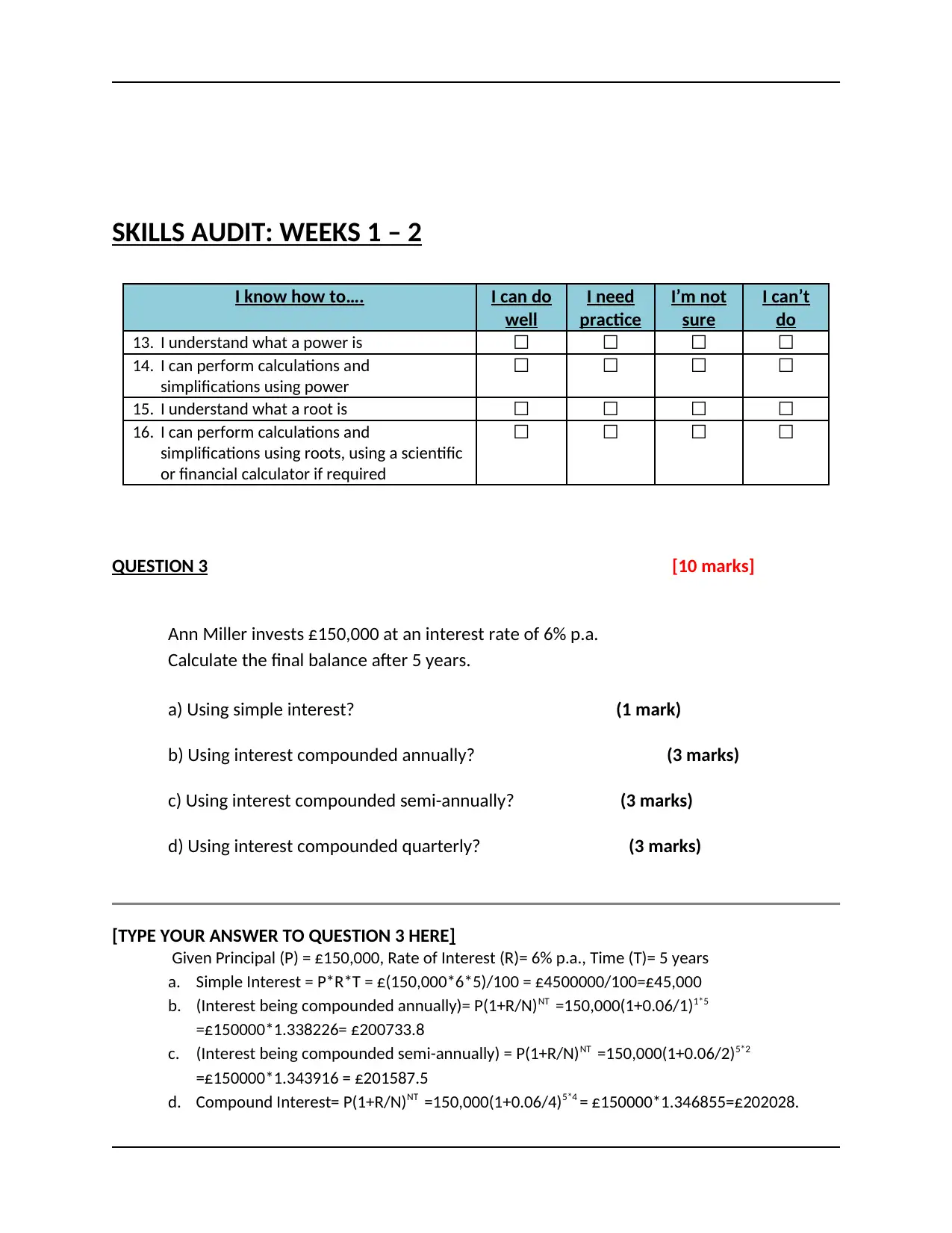
SKILLS AUDIT: WEEKS 1 – 2
I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
13. I understand what a power is ☐ ☐ ☐ ☐
14. I can perform calculations and
simplifications using power
☐ ☐ ☐ ☐
15. I understand what a root is ☐ ☐ ☐ ☐
16. I can perform calculations and
simplifications using roots, using a scientific
or financial calculator if required
☐ ☐ ☐ ☐
QUESTION 3 [10 marks]
Ann Miller invests £150,000 at an interest rate of 6% p.a.
Calculate the final balance after 5 years.
a) Using simple interest? (1 mark)
b) Using interest compounded annually? (3 marks)
c) Using interest compounded semi-annually? (3 marks)
d) Using interest compounded quarterly? (3 marks)
[TYPE YOUR ANSWER TO QUESTION 3 HERE]
Given Principal (P) = £150,000, Rate of Interest (R)= 6% p.a., Time (T)= 5 years
a. Simple Interest = P*R*T = £(150,000*6*5)/100 = £4500000/100=£45,000
b. (Interest being compounded annually)= P(1+R/N)NT =150,000(1+0.06/1)1*5
=£150000*1.338226= £200733.8
c. (Interest being compounded semi-annually) = P(1+R/N)NT =150,000(1+0.06/2)5*2
=£150000*1.343916 = £201587.5
d. Compound Interest= P(1+R/N)NT =150,000(1+0.06/4)5*4 = £150000*1.346855=£202028.
I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
13. I understand what a power is ☐ ☐ ☐ ☐
14. I can perform calculations and
simplifications using power
☐ ☐ ☐ ☐
15. I understand what a root is ☐ ☐ ☐ ☐
16. I can perform calculations and
simplifications using roots, using a scientific
or financial calculator if required
☐ ☐ ☐ ☐
QUESTION 3 [10 marks]
Ann Miller invests £150,000 at an interest rate of 6% p.a.
Calculate the final balance after 5 years.
a) Using simple interest? (1 mark)
b) Using interest compounded annually? (3 marks)
c) Using interest compounded semi-annually? (3 marks)
d) Using interest compounded quarterly? (3 marks)
[TYPE YOUR ANSWER TO QUESTION 3 HERE]
Given Principal (P) = £150,000, Rate of Interest (R)= 6% p.a., Time (T)= 5 years
a. Simple Interest = P*R*T = £(150,000*6*5)/100 = £4500000/100=£45,000
b. (Interest being compounded annually)= P(1+R/N)NT =150,000(1+0.06/1)1*5
=£150000*1.338226= £200733.8
c. (Interest being compounded semi-annually) = P(1+R/N)NT =150,000(1+0.06/2)5*2
=£150000*1.343916 = £201587.5
d. Compound Interest= P(1+R/N)NT =150,000(1+0.06/4)5*4 = £150000*1.346855=£202028.

Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
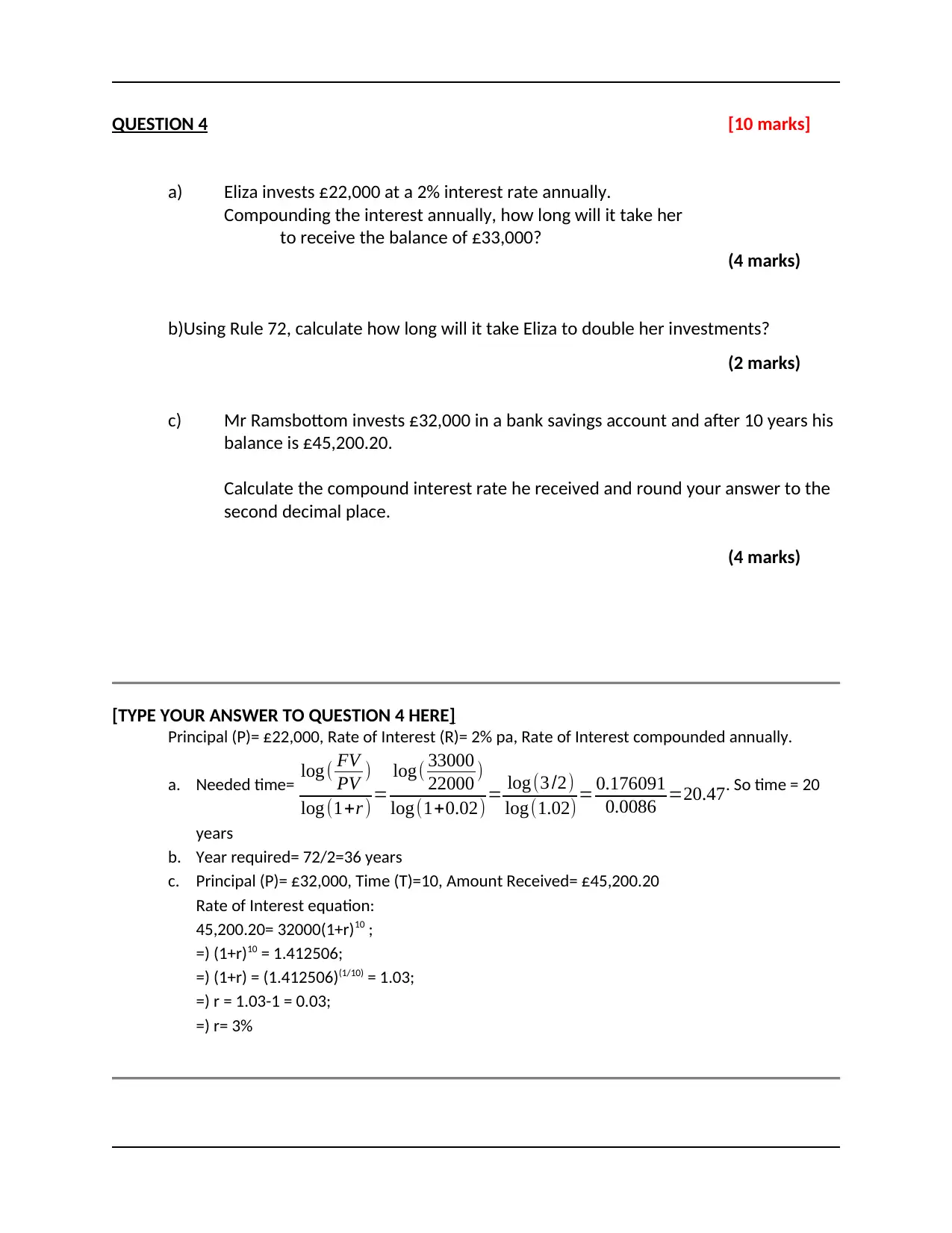
QUESTION 4 [10 marks]
a) Eliza invests £22,000 at a 2% interest rate annually.
Compounding the interest annually, how long will it take her
to receive the balance of £33,000?
(4 marks)
b)Using Rule 72, calculate how long will it take Eliza to double her investments?
(2 marks)
c) Mr Ramsbottom invests £32,000 in a bank savings account and after 10 years his
balance is £45,200.20.
Calculate the compound interest rate he received and round your answer to the
second decimal place.
(4 marks)
[TYPE YOUR ANSWER TO QUESTION 4 HERE]
Principal (P)= £22,000, Rate of Interest (R)= 2% pa, Rate of Interest compounded annually.
a. Needed time= log ( FV
PV )
log (1+r )=
log( 33000
22000 )
log(1+0.02)= log (3 /2)
log(1.02)= 0.176091
0.0086 =20.47. So time = 20
years
b. Year required= 72/2=36 years
c. Principal (P)= £32,000, Time (T)=10, Amount Received= £45,200.20
Rate of Interest equation:
45,200.20= 32000(1+r)10 ;
=) (1+r)10 = 1.412506;
=) (1+r) = (1.412506)(1/10) = 1.03;
=) r = 1.03-1 = 0.03;
=) r= 3%
a) Eliza invests £22,000 at a 2% interest rate annually.
Compounding the interest annually, how long will it take her
to receive the balance of £33,000?
(4 marks)
b)Using Rule 72, calculate how long will it take Eliza to double her investments?
(2 marks)
c) Mr Ramsbottom invests £32,000 in a bank savings account and after 10 years his
balance is £45,200.20.
Calculate the compound interest rate he received and round your answer to the
second decimal place.
(4 marks)
[TYPE YOUR ANSWER TO QUESTION 4 HERE]
Principal (P)= £22,000, Rate of Interest (R)= 2% pa, Rate of Interest compounded annually.
a. Needed time= log ( FV
PV )
log (1+r )=
log( 33000
22000 )
log(1+0.02)= log (3 /2)
log(1.02)= 0.176091
0.0086 =20.47. So time = 20
years
b. Year required= 72/2=36 years
c. Principal (P)= £32,000, Time (T)=10, Amount Received= £45,200.20
Rate of Interest equation:
45,200.20= 32000(1+r)10 ;
=) (1+r)10 = 1.412506;
=) (1+r) = (1.412506)(1/10) = 1.03;
=) r = 1.03-1 = 0.03;
=) r= 3%
WEEKS 3 – 4
I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
17. I understand the idea of simple interest ☐ ☐ ☐ ☐
18. I can perform simple interest calculations ☐ ☐ ☐ ☐
19. I understand the idea of compound interest ☐ ☐ ☐ ☐
20. I can perform compound interest
calculations using a calculator if required
☐ ☐ ☐ ☐
21. I understand the Rule of 72 (or 69 or 70)
and can apply it.
☐ ☐ ☐ ☐
QUESTION 5 [8 marks]
a) Find the value of x if 15 x−10=50. (1 mark)
b) Solve the equation X + 20 = 70. (1 mark)
c) Solve the equation x−6
4 = 10. (1 marks)
d) To plot the linear graph of y = 3x + 10complete the following table:
x - 8 -5 0 7 12 24
y
I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
17. I understand the idea of simple interest ☐ ☐ ☐ ☐
18. I can perform simple interest calculations ☐ ☐ ☐ ☐
19. I understand the idea of compound interest ☐ ☐ ☐ ☐
20. I can perform compound interest
calculations using a calculator if required
☐ ☐ ☐ ☐
21. I understand the Rule of 72 (or 69 or 70)
and can apply it.
☐ ☐ ☐ ☐
QUESTION 5 [8 marks]
a) Find the value of x if 15 x−10=50. (1 mark)
b) Solve the equation X + 20 = 70. (1 mark)
c) Solve the equation x−6
4 = 10. (1 marks)
d) To plot the linear graph of y = 3x + 10complete the following table:
x - 8 -5 0 7 12 24
y
(NO graphrequired)
(5 marks)
[TYPE YOUR ANSWER TO QUESTION 5 HERE]
a. 15x-10=50; =) 15x = 50+10= 60; =) x=60/15; =) x=4
b. X+20=70 =) X=70-20 = 50
c. x−6
4 = 10; =) x – 6 = 40; =) x=40+6 = 46; =) x = 46.
d. Solution:
x - 8 -5 0 7 12 24
y -14 -5 10 31 46 82
WEEK 5
I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
22. I understand the idea of a linear
relationship between two variables
☐ ☐ ☐ ☐
23. I can manipulate a linear equation to solve
for a variable
☐ ☐ ☐ ☐
24. I can construct a scatter plot from a set of
data (a linear relationship applies) and
apply a line of best fit.
☐ ☐ ☐ ☐
25. I understand the y-intercept and slope
(gradient) of a graph and their meaning to
real situations ( y=mx+c).
☐ ☐ ☐ ☐
(5 marks)
[TYPE YOUR ANSWER TO QUESTION 5 HERE]
a. 15x-10=50; =) 15x = 50+10= 60; =) x=60/15; =) x=4
b. X+20=70 =) X=70-20 = 50
c. x−6
4 = 10; =) x – 6 = 40; =) x=40+6 = 46; =) x = 46.
d. Solution:
x - 8 -5 0 7 12 24
y -14 -5 10 31 46 82
WEEK 5
I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
22. I understand the idea of a linear
relationship between two variables
☐ ☐ ☐ ☐
23. I can manipulate a linear equation to solve
for a variable
☐ ☐ ☐ ☐
24. I can construct a scatter plot from a set of
data (a linear relationship applies) and
apply a line of best fit.
☐ ☐ ☐ ☐
25. I understand the y-intercept and slope
(gradient) of a graph and their meaning to
real situations ( y=mx+c).
☐ ☐ ☐ ☐
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
26. I can use the scatter plot produced in part
(12) to derive a linear relationship between
two variables ( y=mx+c).
☐ ☐ ☐ ☐
27. I can use the relationship from part (14) to
extrapolate and interpolate
☐ ☐ ☐ ☐
(12) to derive a linear relationship between
two variables ( y=mx+c).
☐ ☐ ☐ ☐
27. I can use the relationship from part (14) to
extrapolate and interpolate
☐ ☐ ☐ ☐
Question 6 [10 marks]
Sarah Hair Saloon is considering an investment project to purchase and run a Hair Saloon
business. The initial cost is £55,000. The annual cash inflows (income) are projected to be as
follows:
Year 1 Year 2 Year 3 Year 4
£15,000 £25,000 £45,000 £15,000
The discount rate for this investment is 8% p.a., compounded annually.
a) Work out the Net Present Value (NPV) of this investment. (8 marks)
b) Should Sarah proceed with this project?
Explain your reasoning. (2 marks)
[TYPE YOUR ANSWER TO QUESTION 6 HERE]
a. Initial Cost= £55,000,
Annual Cash Flow=
Year 1 Year 2 Year 3 Year 4
£15,000 £25,000 £45,000 £15,000
Rate of Interest=8%
NPV= ∑
t =1
T Ct
( 1+ r)t - C0
=(15000/(1+0.08)) + (25000/(1+0.08)2) + (45000/(1+0.08)3) + (15000/(1+0.08)4) – 55000
=£(16200+29160+56687.04+20407.33-55000)
=£(122454.4 – 55000) = £67454.37.
b. Net Present value is the difference between the present value of cash inflow and present value
of cash outflow. A positive NPV would imply acceptation of the investment and negative NPV
would imply rejection. Since the value is quite high and positive. Hence, Sarah should proceed
with the investment. However, there is no option for comparison here, since no alternative NPV
value is available.
WEEK 6
Sarah Hair Saloon is considering an investment project to purchase and run a Hair Saloon
business. The initial cost is £55,000. The annual cash inflows (income) are projected to be as
follows:
Year 1 Year 2 Year 3 Year 4
£15,000 £25,000 £45,000 £15,000
The discount rate for this investment is 8% p.a., compounded annually.
a) Work out the Net Present Value (NPV) of this investment. (8 marks)
b) Should Sarah proceed with this project?
Explain your reasoning. (2 marks)
[TYPE YOUR ANSWER TO QUESTION 6 HERE]
a. Initial Cost= £55,000,
Annual Cash Flow=
Year 1 Year 2 Year 3 Year 4
£15,000 £25,000 £45,000 £15,000
Rate of Interest=8%
NPV= ∑
t =1
T Ct
( 1+ r)t - C0
=(15000/(1+0.08)) + (25000/(1+0.08)2) + (45000/(1+0.08)3) + (15000/(1+0.08)4) – 55000
=£(16200+29160+56687.04+20407.33-55000)
=£(122454.4 – 55000) = £67454.37.
b. Net Present value is the difference between the present value of cash inflow and present value
of cash outflow. A positive NPV would imply acceptation of the investment and negative NPV
would imply rejection. Since the value is quite high and positive. Hence, Sarah should proceed
with the investment. However, there is no option for comparison here, since no alternative NPV
value is available.
WEEK 6
I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
28. I understand the idea of the future value of
money
☐ ☐ ☐ ☐
29. I understand the idea the net present value
(NPV) of a project
☐ ☐ ☐ ☐
30. I can complete a net present value
calculation, using a calculator if required
☐ ☐ ☐ ☐
Question 7 [10 marks]
A set of test scores, marked out of 100, is as follows:
66 93 75 58 68
53 65 92 94 62
63 74 93 92 95
58 94 62 78 96
62 64 87 66 57
a) Produce a tally of this data set suitable for the production of a histogram (3 marks)
b) Draw a histogram of this data set (6 marks)
c) Comment on the distribution of these marks. (1 marks)
[TYPE YOUR ANSWER TO QUESTION 7 HERE]
The dataset is being divided into four groups and they are <63.75, >63.75 and <74.5, >74.5 and
<85.25, >85.25 and <96
a. Tally for data set-
X<63.75 IIII III
63.75 <X<74.5 IIII I
well
I need
practice
I’m not
sure
I can’t
do
28. I understand the idea of the future value of
money
☐ ☐ ☐ ☐
29. I understand the idea the net present value
(NPV) of a project
☐ ☐ ☐ ☐
30. I can complete a net present value
calculation, using a calculator if required
☐ ☐ ☐ ☐
Question 7 [10 marks]
A set of test scores, marked out of 100, is as follows:
66 93 75 58 68
53 65 92 94 62
63 74 93 92 95
58 94 62 78 96
62 64 87 66 57
a) Produce a tally of this data set suitable for the production of a histogram (3 marks)
b) Draw a histogram of this data set (6 marks)
c) Comment on the distribution of these marks. (1 marks)
[TYPE YOUR ANSWER TO QUESTION 7 HERE]
The dataset is being divided into four groups and they are <63.75, >63.75 and <74.5, >74.5 and
<85.25, >85.25 and <96
a. Tally for data set-
X<63.75 IIII III
63.75 <X<74.5 IIII I
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
74.5<X<85.25 IIII IIII
85.25<X<96 IIII IIII IIII
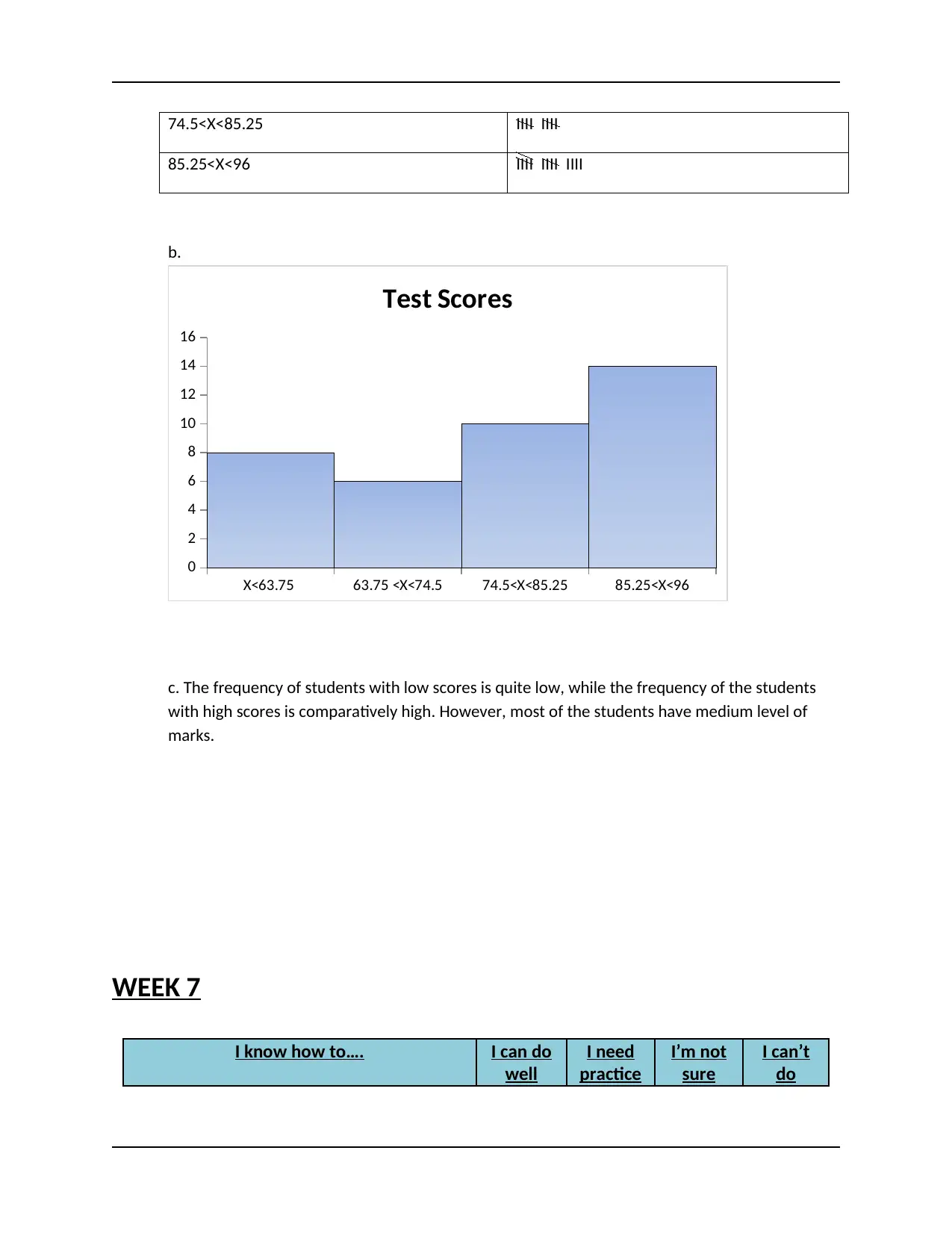
b.
X<63.75 63.75 <X<74.5 74.5<X<85.25 85.25<X<96
0
2
4
6
8
10
12
14
16
Test Scores
c. The frequency of students with low scores is quite low, while the frequency of the students
with high scores is comparatively high. However, most of the students have medium level of
marks.
WEEK 7
I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
85.25<X<96 IIII IIII IIII
b.
X<63.75 63.75 <X<74.5 74.5<X<85.25 85.25<X<96
0
2
4
6
8
10
12
14
16
Test Scores
c. The frequency of students with low scores is quite low, while the frequency of the students
with high scores is comparatively high. However, most of the students have medium level of
marks.
WEEK 7
I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
31. I understand the idea of frequency
distribution
☐ ☐ ☐ ☐
32. I can read and interpret a histogram ☐ ☐ ☐ ☐
33. I can construct a histogram from a set of
data
☐ ☐ ☐ ☐
Question 8 [8 marks]
Probability is a measure of the likelihood and can be stated as a ratio, percentage or generally
as a number between zero and one.
a)What is the probability when the likelihood is impossible? (1 mark)
b) What is the probability when the likelihood is certain? (1 mark)
c) Express the probability of 0.06 as a % (2 marks)
d) Josiah tossed a coin and thrown a die at the same time (simultaneously). Work out the
probability of getting a head on the coin and a 5 on the die.
(4 marks)
[TYPE YOUR ANSWER TO QUESTION 8 HERE]
a. An impossible likelihood has probability of 0.
b. A certain likelihood have probability of 1
c. Probability of 0.06 means the occurrence of event is 6% probable
d. Probability of getting Head in a coin is 1/2 and the probability of getting 5 on a dice is 1/6.
Hence, the probability of getting Head and a five on dice together simultaneously is 1/12.
distribution
☐ ☐ ☐ ☐
32. I can read and interpret a histogram ☐ ☐ ☐ ☐
33. I can construct a histogram from a set of
data
☐ ☐ ☐ ☐
Question 8 [8 marks]
Probability is a measure of the likelihood and can be stated as a ratio, percentage or generally
as a number between zero and one.
a)What is the probability when the likelihood is impossible? (1 mark)
b) What is the probability when the likelihood is certain? (1 mark)
c) Express the probability of 0.06 as a % (2 marks)
d) Josiah tossed a coin and thrown a die at the same time (simultaneously). Work out the
probability of getting a head on the coin and a 5 on the die.
(4 marks)
[TYPE YOUR ANSWER TO QUESTION 8 HERE]
a. An impossible likelihood has probability of 0.
b. A certain likelihood have probability of 1
c. Probability of 0.06 means the occurrence of event is 6% probable
d. Probability of getting Head in a coin is 1/2 and the probability of getting 5 on a dice is 1/6.
Hence, the probability of getting Head and a five on dice together simultaneously is 1/12.
WEEK 8
I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
34. I understand simple probabilities ☐ ☐ ☐ ☐
35. I can perform probability calculations, using
a calculator if required
☐ ☐ ☐ ☐
36. I understand and can perform exchange
rate calculations
☐ ☐ ☐ ☐
I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
34. I understand simple probabilities ☐ ☐ ☐ ☐
35. I can perform probability calculations, using
a calculator if required
☐ ☐ ☐ ☐
36. I understand and can perform exchange
rate calculations
☐ ☐ ☐ ☐
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Section 2
Task 1 - Two Real life examples (100 words each) [8 marks]
Give two real-life situations or problems in businesses that involve the topics studied in
this module (e.g. powers and roots, simple and compound interests, linear
relationships, graphs, probabilities and Net Present values (NPV)).
[TYPE YOUR ANSWERS TO TASK 1 HERE]
(1) (4 marks)
In a cricket match, the umpire tosses the coin and the captain calls for head or tail. The
probability that he would win the toss is ½.
(2) (4 marks)
The savings account in the bank provides us with 4% interest and it is compounded
annually. It is an example of Compound interest in daily life.
Task 2 - Online Activities [10 marks]
This relates to the quiz. Please complete and pass all three relevant quiz/activity;
screenshot and save the result’s screen ready to be pasted on the portfolio.
Ensure the followings are visible before the screenshot:
Your full names on the top right-hand corner of the screen
Your test result is any score from 40% to 100%
[PASTE YOUR SCREENSHOTS FOR TASK 2 HERE]
Task 1 - Two Real life examples (100 words each) [8 marks]
Give two real-life situations or problems in businesses that involve the topics studied in
this module (e.g. powers and roots, simple and compound interests, linear
relationships, graphs, probabilities and Net Present values (NPV)).
[TYPE YOUR ANSWERS TO TASK 1 HERE]
(1) (4 marks)
In a cricket match, the umpire tosses the coin and the captain calls for head or tail. The
probability that he would win the toss is ½.
(2) (4 marks)
The savings account in the bank provides us with 4% interest and it is compounded
annually. It is an example of Compound interest in daily life.
Task 2 - Online Activities [10 marks]
This relates to the quiz. Please complete and pass all three relevant quiz/activity;
screenshot and save the result’s screen ready to be pasted on the portfolio.
Ensure the followings are visible before the screenshot:
Your full names on the top right-hand corner of the screen
Your test result is any score from 40% to 100%
[PASTE YOUR SCREENSHOTS FOR TASK 2 HERE]

Task 3 - Reflective Log (150 words) [7 marks]
This reflective log should develop as the course proceeds, and may be the last part to
be completed. Reflect honestly on your experiences throughout the semester. Start
your reflective log from week one by completing the skills audits and by writing
personal weekly notes after each topic. Please ask for your Tutor’s support if needed.
You may wish to consider the following points when providing your reflective
comments:
Which topics do you feel most confident about? (e.g. powers and roots, interest rates,
NPV etc.)
Are there areas for improvement (e.g. in probability, I need do practice more or
research etc.)?
How would you evaluate your participation on the module (e.g. contribution to
classes, independent study etc.)?
[TYPE YOUR ANSWER TO TASK 3 HERE]
This reflective log should develop as the course proceeds, and may be the last part to
be completed. Reflect honestly on your experiences throughout the semester. Start
your reflective log from week one by completing the skills audits and by writing
personal weekly notes after each topic. Please ask for your Tutor’s support if needed.
You may wish to consider the following points when providing your reflective
comments:
Which topics do you feel most confident about? (e.g. powers and roots, interest rates,
NPV etc.)
Are there areas for improvement (e.g. in probability, I need do practice more or
research etc.)?
How would you evaluate your participation on the module (e.g. contribution to
classes, independent study etc.)?
[TYPE YOUR ANSWER TO TASK 3 HERE]
1 out of 18
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.





