Numeracy and Data Analysis: Practical Application with Temperature Data of London

This report explains the practical application of numeracy and data analysis with temperature data of London for 10 consecutive days. It covers the computation of measures of central tendency such as mean, median, mode, range and standard deviation. It also utilises the linear forecasting model to forecast the temperature for days 11 and 12. Subject: Numeracy and Data Analysis, Course Code: NA101, College/University: Not mentioned.

Numeracy and Data
Analysis

Contents
INTRODUCTION...........................................................................................................................1
MAIN BODY...................................................................................................................................1
1 . Arrange the temperature data of 10 consecutive days............................................................1
2 . Display the data in any two types of charts............................................................................2
3 . Compute the following along with the steps and highlight the final values of the same.......3
CONCLUSION ...............................................................................................................................6
REFERENCES................................................................................................................................7

INTRODUCTION
Numeracy refers to the ability of understanding,reasoning with and applying the simple
numerical ideas and concepts. It explains the ways in which mathematics is utilised in the actual
world scenario and is used to be able to utilize and make the best feasible decisions. Data
analysis explains the pattern of operating with data to gather some helpful information, that can
be utilized to form informed decisions. In this report, the concept of numeracy and data statistics
has been explained practically with the help of the temperature of London for 10 consecutive
days. The various measures such as mean, median and many more are computed to analyse the
data along with depiction of the same with the help of graph and line charts.
MAIN BODY
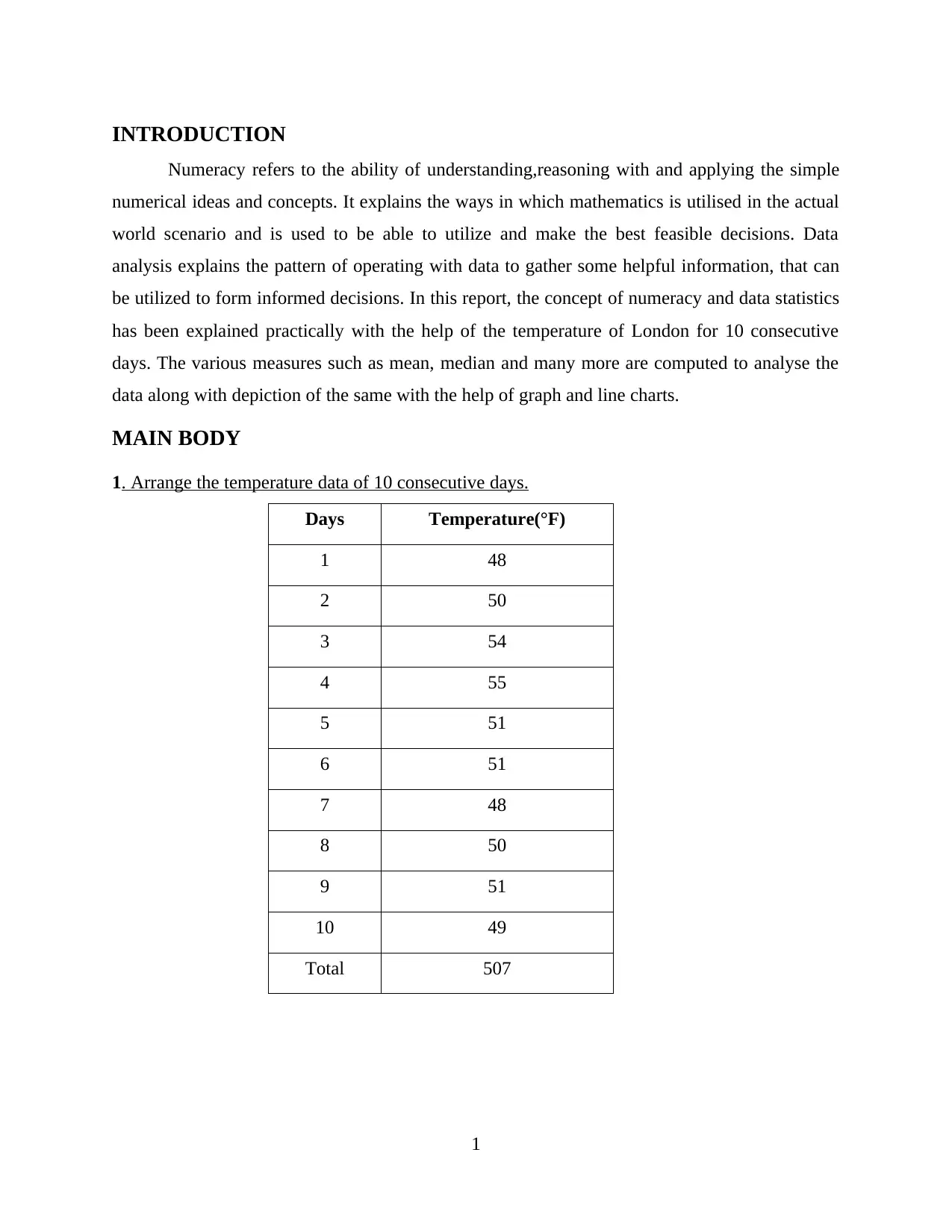
1. Arrange the temperature data of 10 consecutive days.
Days Temperature(°F)
1 48
2 50
3 54
4 55
5 51
6 51
7 48
8 50
9 51
10 49
Total 507
1

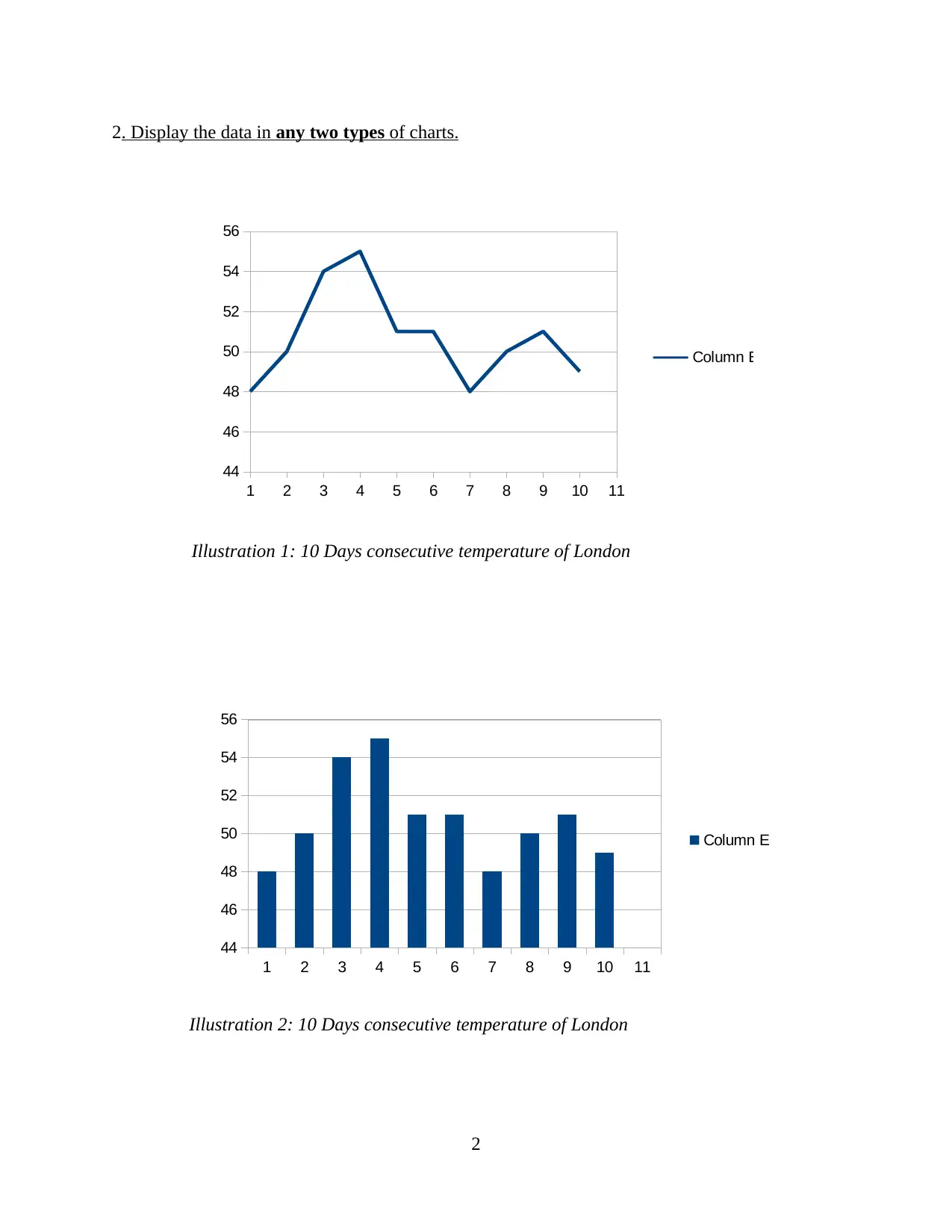
2. Display the data in any two types of charts.
2
1 2 3 4 5 6 7 8 9 10 11
44
46
48
50
52
54
56
Column E
Illustration 1: 10 Days consecutive temperature of London
1 2 3 4 5 6 7 8 9 10 11
44
46
48
50
52
54
56
Column E
Illustration 2: 10 Days consecutive temperature of London

3. Compute the following along with the steps and highlight the final values of the same.
Mean: It defines the average value of the given data set. Mean is computed by dividing the sum
of the numbers in given data set by the total number of values. It helps to summarize the entire
set of data with a single number which represents the central point of data set.
Mean = {Sum of Observation} ÷ {Total numbers of Observations}
= (48+50+54+55+51+51+48+50+51+49)/10
=507/10
Mean = 50.7
Steps:1. Ascertain the sum of values in the data set.2. Divide the sum obtained by he total number of values in the data set.
Median: Median is termed to be the middle most value of the entire data set after arranging the
data in an ascending order or descending order.
If data set has even number of values: Median = [(n/2)th term + {(n/2)+1}th]/2
Here the data set has 10 values so total number of values is even.
Median= [10/2th term + (10/2+1)th ]/2
= (5+6)th/2
=51+51/2
=102/2
Median= 51
Steps:
1. Organize and arrange the present data set in an ascending or descending order.
2. Count the number of values in data set.
3. If number of values are odd, apply the formula Median= {(n+1)/2}th term
4. If number of values are even, apply the formula Median=[(n/2)th term={(n/2)+1}th]/2
Mode: It is the value of highest frequency in the entire data set present. The value which is
termed as mode occurs in a given set of data the most often number of times.
Ascending order: 48 48 49 50 50 51 51 51 54 55
Mode= 51
Steps:
1. Arrange the entire data set in an ascending or descending order.
3

2. Count each number in the data set as to how many times it occurs in the data set.
3. The number which occurs most frequently and highest number of times is termed to b the
mode of the data set.
Range: It is the difference between the highest and the smallest value of data.
Range= (Highest value- Lowest value)
= (55-48)
Range = 7
Standard Deviation: It measures the dispersion of data when compared to the mean of data set.
Standard Deviation= √∑ (xi – μ) ^ 2 / N
= √(507-50.7)^2 / 10
= √208209.69/10
Standard Deviation=144.29
Steps:
1. Find the mean
2. For each value, find the square of its deviation from mean
3. Sum these values.
4. Divide them by total number of values.
5. Then take square root.
4

4. Utilise the linear forecasting model to compute the following: Calculation of m value. Calculation of c value.
Utilising calculated 'm' and 'c' values, forecast the humidity for day 11 and 12.
Linear forecasting model: It is a type of tool in statistics which is utilised to determine the future
values which are not present with the help of various past values. It is generally utilised as a
quantitative approach to ascertain the underlying trend in the entire data set.
y= mx+c
Calculation of 'm' value
= (10*2776)-(55*507)/(10*385)-(3025)
=-125/825
=-0.1515
Calculation of 'c' value
=507-(-0.1515*55)/10
=51.53
Temperature of day 11:
m = -0.1515, x=11, c=51.53
y = mx + C
=(-0.1515*11)+51.53
Temperature of day 11= 53.1
Temperature of day 14:
5

m = -0.1515, x=14, c=51.53
y = mx + C
=(-0.1515*14)+51.53
Temperature of day 14= 53.6
6

CONCLUSION
From the above report, it can be concluded that numeracy is an essential concept when
utilised for calculation and analysis of various factors to assists in decision making. The report
presents the various methods to calculate the measures of central tendency such as mean,
median, mode and many more to analyse the temperature data of London for 10 consecutive
days. In the end, it utilises the linear forecasting model and concludes upon the temperature for
days 11 and 12 with the help of already established values.
7

REFERENCES
Books and Journals:
Agnello, E. C., 2018. Why Are We Doing Math in English Class? Building Quantitative Literacy
to Improve Expository Text Comprehension. Numeracy: Advancing Education in
Quantitative Literacy, 11(2).
Hojnoski, R. L., Caskie, G. I. and Miller Young, R., 2018. Early numeracy trajectories: Baseline
performance levels and growth rates in young children by disability status. Topics in
Early Childhood Special Education, 37(4), pp.206-218.
Porciani, L. and Rondinella, T., 2019. Teaching official statistics in universities.
Recommendations from a direct experience. Statistical Journal of the IAOS, 35(3),
pp.425-433.
Sulak, T. N., and et.al., 2020. The relationships between numeracy scores and soft skills in
employed and unemployed Americans. New Horizons in Adult Education and Human
Resource Development, 32(2), pp.19-39.
Tout, D., 2020. Evolution of adult numeracy from quantitative literacy to numeracy: Lessons
learned from international assessments. International Review of Education, 66(2),
pp.183-209.
Tsagaroulis, P., 2020. Data Visualization, Numeracy and Graph Literacy: Seeing and Thinking
of Data Presented as Tables or Charts (Doctoral dissertation, The Chicago School of
Professional Psychology).
(Agnello, 2018)(Hojnoski, Caskie and Miller Young, 2018)(Porciani and Rondinella, 2019)
(Tout, 2020)(Tsagaroulis, 2020)(Sulak and et.al., 2020)
8