Assignment | Multiple Choice Questions

Provide Engineering Solutions to Problems in Complex Polyphase Power Circuits is the assignment,

Part 1 – Multiple-Choice Questions
For the following questions, select which answer is TRUE.
Question 1 (# marks)
The operator j = 1/90° and a = 1/120°:
a) j3 = 1
b) a4 = a
c) a3 = j2
d) a2 = 1+ a
[Insert answer here]
Ans: b)
Question 2 (# marks)
The per unit value of Z11kV line = (2.42 +j 3.63) Ω on a 10 MVA Base is:
a) (0.1 + j0.15) pu
b) (0.2 + j0.3) pu
c) (0.3 + j0.45) pu
d) (0.4 + j0.6) pu
[Insert answer here]
Ans: b)
Question 3 (# marks)
A 60 MVA transformer has R = 0.6% and X = 7.5%. Calculate in rectangular form the per unit
values on a 100MVA base:
a) (0.01 + j0.125)pu
b) (0.02 + j0.125) pu
c) (0.015 + j0.25) pu
d) (0.015 - j0.25) pu
[Insert answer here]
Ans: a)
Question 4 (# marks)
Which of the following is correct?
a) Z, R, X are phasors that can be represented by an impedance ∆
b) S, P, Q are phasors that can be represented by a Power ∆
c) θ, the angle in the impedance ∆, is the angle by which I lags or leads V
d) In the power triangle, Q = S cos θ and P = S sin θ°
[Insert answer here]
Ans: b)
Question 5 (# marks)
The voltage V = 10 ∟-15° Volts in the frequency domain is equivalent to which voltage in the time
domain:
a) V (t) = 10 cos (ωt + 75)
b) V (t) = 10 √2 cos (ωt + 75)
c) V (t) = 10 sin (ωt + 75)
d) V (t) = 10 √2 sin (ωt - 75)

[Insert answer here]
Ans: c)
Question 6 (# marks)
Which of the following is correct?
a) In- phase instantaneous power alternates between + ve and – ve for a 0PF load.
b) Quadrature instantaneous power alternates between + ve and – ve for a unity PF load.
c) In- phase instantaneous power for a lagging load is an offset sinusoidal varying sine wave.
d) Quadrature instantaneous power never goes – ve
[Insert answer here]
Ans: a)
Question 7 (# marks)
The frequency of in-phase instantaneous power for a 50Hz system is:
a) 50 Hz
b) 100 Hz
c) 150 Hz
d) Zero because it is an offset sine wave
[Insert answer here]
Ans: b)
Question 8 (# marks)
For VA = 240/30° and VB = 240/210°, the value of VAB is:
a) 0V
b) 480/30° V
c) 480/210° V
d) 240/30° V
[Insert answer here]
Ans: b)
Question 9 (# marks)
The frequency of V = 340 sin (2513.3t + 30°) is:
a) 50 Hz
b) 100 Hz
c) 200Hz
d) 400Hz
[Insert answer here]
Ans: d)
Question 10 (# marks)
For V = 250/0° V and I = 20/–36.87° A: The maximum value of in-phase instantaneous power (p) is:
a) 10 kW
b) 6 kW
c) 8 kW
d) 4 kW
[Insert answer here]
Ans: d)
Question 11 (# marks)

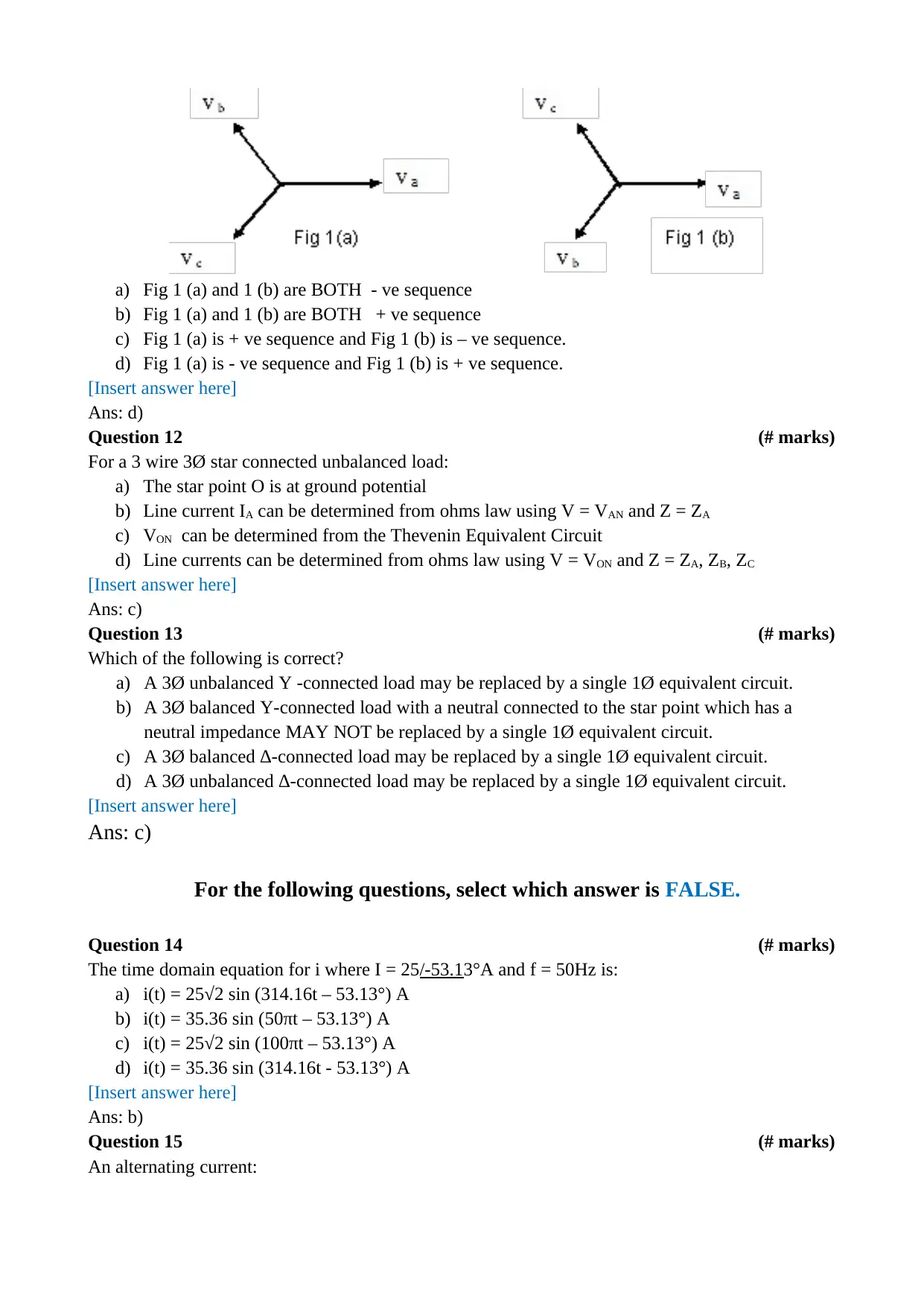
a) Fig 1 (a) and 1 (b) are BOTH - ve sequence
b) Fig 1 (a) and 1 (b) are BOTH + ve sequence
c) Fig 1 (a) is + ve sequence and Fig 1 (b) is – ve sequence.
d) Fig 1 (a) is - ve sequence and Fig 1 (b) is + ve sequence.
[Insert answer here]
Ans: d)
Question 12 (# marks)
For a 3 wire 3Ø star connected unbalanced load:
a) The star point O is at ground potential
b) Line current IA can be determined from ohms law using V = VAN and Z = ZA
c) VON can be determined from the Thevenin Equivalent Circuit
d) Line currents can be determined from ohms law using V = VON and Z = ZA, ZB, ZC
[Insert answer here]
Ans: c)
Question 13 (# marks)
Which of the following is correct?
a) A 3Ø unbalanced Y -connected load may be replaced by a single 1Ø equivalent circuit.
b) A 3Ø balanced Y-connected load with a neutral connected to the star point which has a
neutral impedance MAY NOT be replaced by a single 1Ø equivalent circuit.
c) A 3Ø balanced ∆-connected load may be replaced by a single 1Ø equivalent circuit.
d) A 3Ø unbalanced ∆-connected load may be replaced by a single 1Ø equivalent circuit.
[Insert answer here]
Ans: c)
For the following questions, select which answer is FALSE.
Question 14 (# marks)
The time domain equation for i where I = 25/-53.13°A and f = 50Hz is:
a) i(t) = 25√2 sin (314.16t – 53.13°) A
b) i(t) = 35.36 sin (50πt – 53.13°) A
c) i(t) = 25√2 sin (100πt – 53.13°) A
d) i(t) = 35.36 sin (314.16t - 53.13°) A
[Insert answer here]
Ans: b)
Question 15 (# marks)
An alternating current:

a) Is a vector quantity since, in polar form, current has magnitude and direction.
b) Can be shown in the form i(t) = Imax sin (ωt)
c) Is a phasor rotates at an angular frequency of ‘f’ revolutions per sec
d) Has RMS value of Peak divided by square root of two
[Insert answer here]
Ans: a)
Question 16 (# marks)
The current lags the associated voltage when:
a) Lagging power factor occurs
b) + j kVAr exists
c) The load is inductive
d) There is a heating load
[Insert answer here]
Ans: d)
Question 17 (# marks)
For a balanced 3Ø load
a) the 3 impedances are equal
b) the line currents are equal
c) |S| = √3|Vline|x|Iline|
d) The sum of the line currents = 0
[Insert answer here]
Ans: b)
Question 18 (# marks)
Which of the following is incorrect?
a) For a balanced 3Ø star connected system the line voltage is equal to √3 times the phase
voltage.
b) For a balanced 3Ø delta connected load the line voltage is equal to the phase voltage
c) In a star connected 3Ø system the line voltage EBC = EBN – ECN
d) For a balanced 3Ø delta connected load the line current is equal to the phase current.
[Insert answer here]
Ans: d)
Question 19 (# marks)
Larger consumers install capacitor banks at their premises so that:
a) There is a reduction in kVA demand
b) There is a reduction in kW demand
c) There is a reduction in kVAr demand
d) There is an improvement in pf to lower demand cost
[Insert answer here]
Ans: b)

Part 2 – Calculation Questions
Question 20 (# marks)
A series circuit comprises of a 12 ohm resistor, an inductor of 192 mH and a capacitor of 100 μF.
This is connected to an AC supply of 230 V 50 Hz. Calculate the following:
a) XL
b) Xc
c) Total impedance Z
d) I
e) VL
f) Vc
[Insert answer here]
a) XL= 2πfL= 2×π×50× 192
1000 = 60.32 Ω
b) X c= 1
2 πfC = 1
2 π × 50× 100× 10−6 =31.83 Ω
c) Z= √122+(60.32−31.83)2=30.91 ∠ 67.16 °Ω
d) I = V
Z = 230
√ 122 +(60.32−31.83)2 = 230
30.91 =7.44 ∠−67.16° A
e) V L=I X L=7.44 ∠−67.16 ° ×60.32 ∠90 °=448.78 ∠22.84 ° V
f) V C=I XC=7.44 ∠−67.16 ° ×31.83 ∠−90 °=236.82∠−157.16 °V
Question 21 (# marks)
A voltage source V (t) = 200 sin (314t + 30) is connected in series with a 12 ohm resistor and a
100μF capacitor. Calculate:
a) Xc
b) Total impedance - in polar and rectangular form
c) (c) Current Im
d) Average power
[Insert answer here]
a) 314=2 πf , f = 314
2 π =49.97 Hz , X C= 1
2 π ×49.97 × 100× 10−6 =31.85 Ω
b) Z= √ 122+31.852 =34.04 , phase angle=tan−1 31.85
12 =69.36 °
Polar form = 34.04 ∠69.36 ° Ω
Rectangular form = 12 + j31.85 Ω
c) I m= 200 ∠30°
34.04 ∠69.36 ° =5.88∠−39.36 ° A
d) Pavg =V m I m
2 cos ( θv−θi ) =(200)(5.88)
2 cos ¿
Question 22 (# marks)

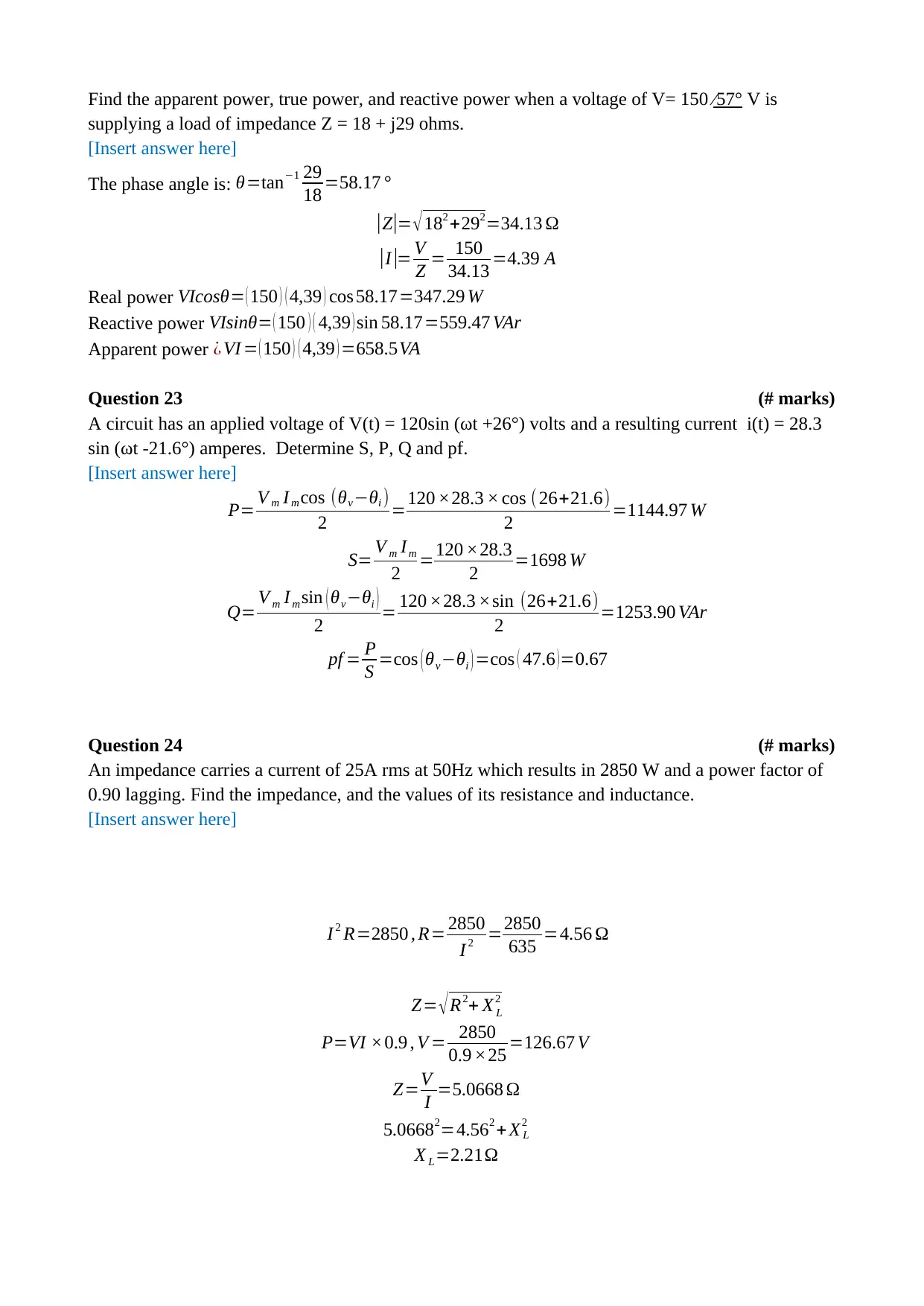
Find the apparent power, true power, and reactive power when a voltage of V= 150 ⁄57° V is
supplying a load of impedance Z = 18 + j29 ohms.
[Insert answer here]
The phase angle is: θ=tan−1 29
18 =58.17 °
|Z|= √ 182 +292=34.13 Ω
|I |= V
Z = 150
34.13 =4.39 A
Real power VIcosθ= ( 150 ) ( 4,39 ) cos 58.17=347.29 W
Reactive power VIsinθ= ( 150 ) ( 4,39 ) sin 58.17=559.47 VAr
Apparent power ¿ VI = ( 150 ) ( 4,39 ) =658.5VA
Question 23 (# marks)
A circuit has an applied voltage of V(t) = 120sin (ωt +26°) volts and a resulting current i(t) = 28.3
sin (ωt -21.6°) amperes. Determine S, P, Q and pf.
[Insert answer here]
P= V m I m cos (θv−θi )
2 =120 ×28.3 × cos (26+21.6)
2 =1144.97 W
S= V m Im
2 =120 ×28.3
2 =1698 W
Q= V m I m sin ( θv−θi )
2 = 120 ×28.3 ×sin (26+21.6)
2 =1253.90 VAr
pf = P
S =cos ( θv−θi ) =cos ( 47.6 ) =0.67
Question 24 (# marks)
An impedance carries a current of 25A rms at 50Hz which results in 2850 W and a power factor of
0.90 lagging. Find the impedance, and the values of its resistance and inductance.
[Insert answer here]
I 2 R=2850 , R= 2850
I 2 =2850
635 =4.56 Ω
Z= √ R2+ X L
2
P=VI ×0.9 , V = 2850
0.9 ×25 =126.67 V
Z=V
I =5.0668 Ω
5.06682=4.562 + X L
2
X L=2.21Ω

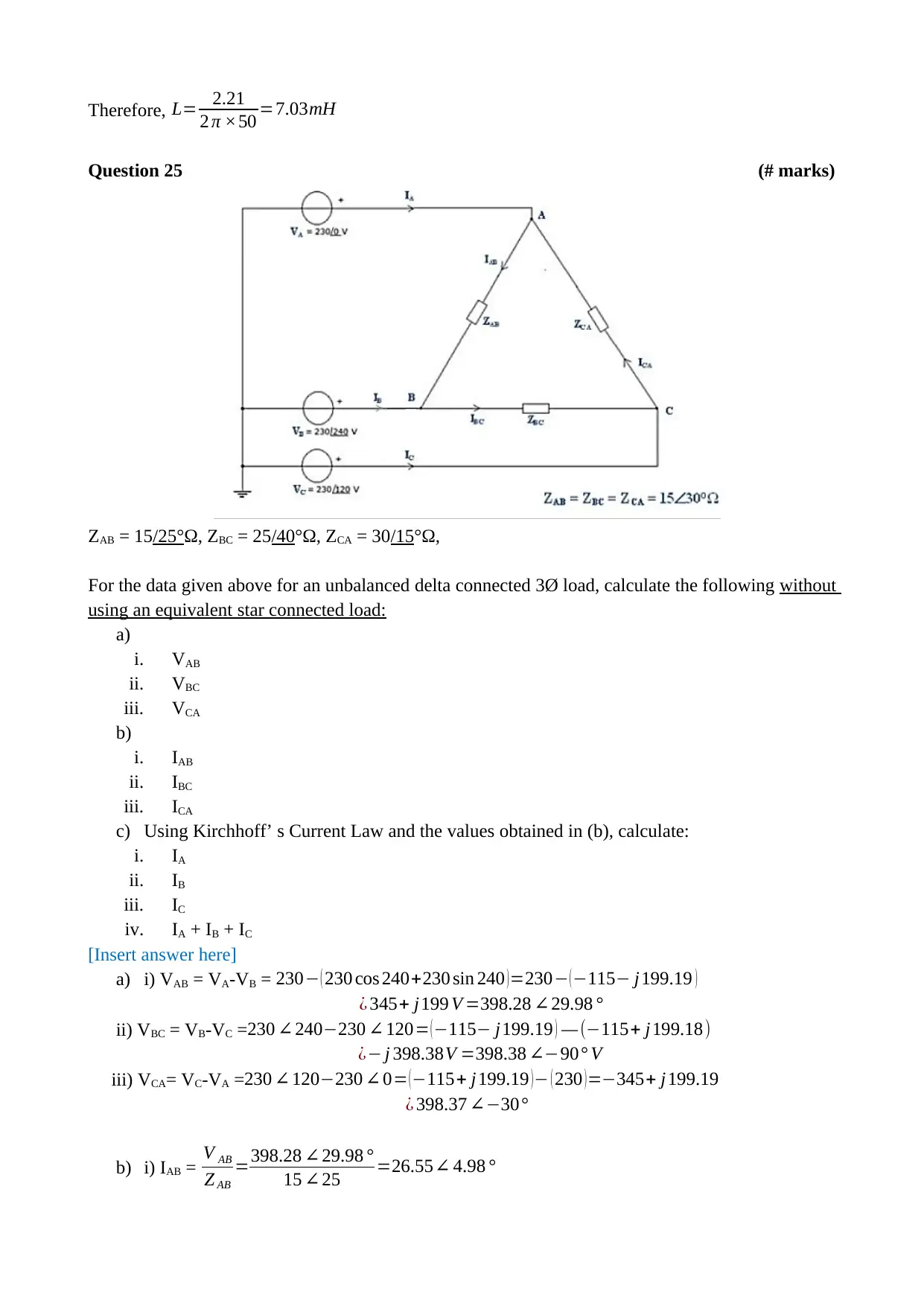
Therefore, L= 2.21
2 π ×50 =7.03mH
Question 25 (# marks)
ZAB = 15/25°Ω, ZBC = 25/40°Ω, ZCA = 30/15°Ω,
For the data given above for an unbalanced delta connected 3Ø load, calculate the following without
using an equivalent star connected load:
a)
i. VAB
ii. VBC
iii. VCA
b)
i. IAB
ii. IBC
iii. ICA
c) Using Kirchhoff’ s Current Law and the values obtained in (b), calculate:
i. IA
ii. IB
iii. IC
iv. IA + IB + IC
[Insert answer here]
a) i) VAB = VA-VB = 230− ( 230 cos 240+230 sin 240 )=230− (−115− j199.19 )
¿ 345+ j199 V =398.28 ∠29.98 °
ii) VBC = VB-VC =230 ∠ 240−230 ∠ 120= ( −115− j199.19 ) — (−115+ j199.18)
¿− j 398.38V =398.38 ∠−90° V
iii) VCA= VC-VA =230 ∠120−230 ∠ 0= (−115+ j199.19 )− ( 230 )=−345+ j199.19
¿ 398.37 ∠−30°
b) i) IAB = V AB
Z AB
=398.28 ∠ 29.98 °
15 ∠ 25 =26.55∠ 4.98 °

ii) IBC = V BC
ZBC
=398.38 ∠−90 °
25∠ 40 =15.94 ∠−130°
iii) ICA = V CA
ZCA
= 398.37 ∠−30°
30 ∠ 15 =13.28 ∠−45 °
c) i) IA = IAB- ICA = 26.55 ∠4.98 °−13.28 ∠−45 °=17.06+ j11.69
¿ 20.68 ∠34.42° A
ii) IB = IBC- IAB = 15.94 ∠−130 °−26.55 ∠ 4.98 °=−36.7− j 14.51
¿ 39.46 ∠201.57 °
iii) IC = ICA- IBC = 13.28 ∠−45 °−15.94 ∠−130 °=19.64+ j21.6
¿ 21.19 ∠47.72 °
iv) IA+IB+IC = j 18.72 A=18.72 ∠90 ° A
Question 26 (# marks)
A star connected unbalanced load of ZA= 2/20°Ω, ZB = 1.5/10°Ω, ZC= 3/25°Ω, is connected to a
4-wire 3-phase supply with VA= 230/0° and a positive phase sequence.
a) Calculate ZTHEV (the Thevenin Impedance).
b) INOR (the NORTON or S/C current)
c) VTHEV (the Thevenin Voltage)
d) Sketch the Thevenin EQUIVALENT CIRCUIT without any load connected showing the
terminals and N as well as VTHEV, ZTHEV and their values.
e) Sketch the Thevenin EQUIVALENT CIRCUIT with the load impedance ZN connected to 2
terminals O and N.
f) Using the THEVENIN equivalent circuit in (e), for ZN = (0.03 + j 0.05) Ω, i.e. neutral
conductor impedance, determine:
i. IN
ii. VON
iii. IA
iv. IB
v. IC
vi. Check that IA + IB + IC = IN.

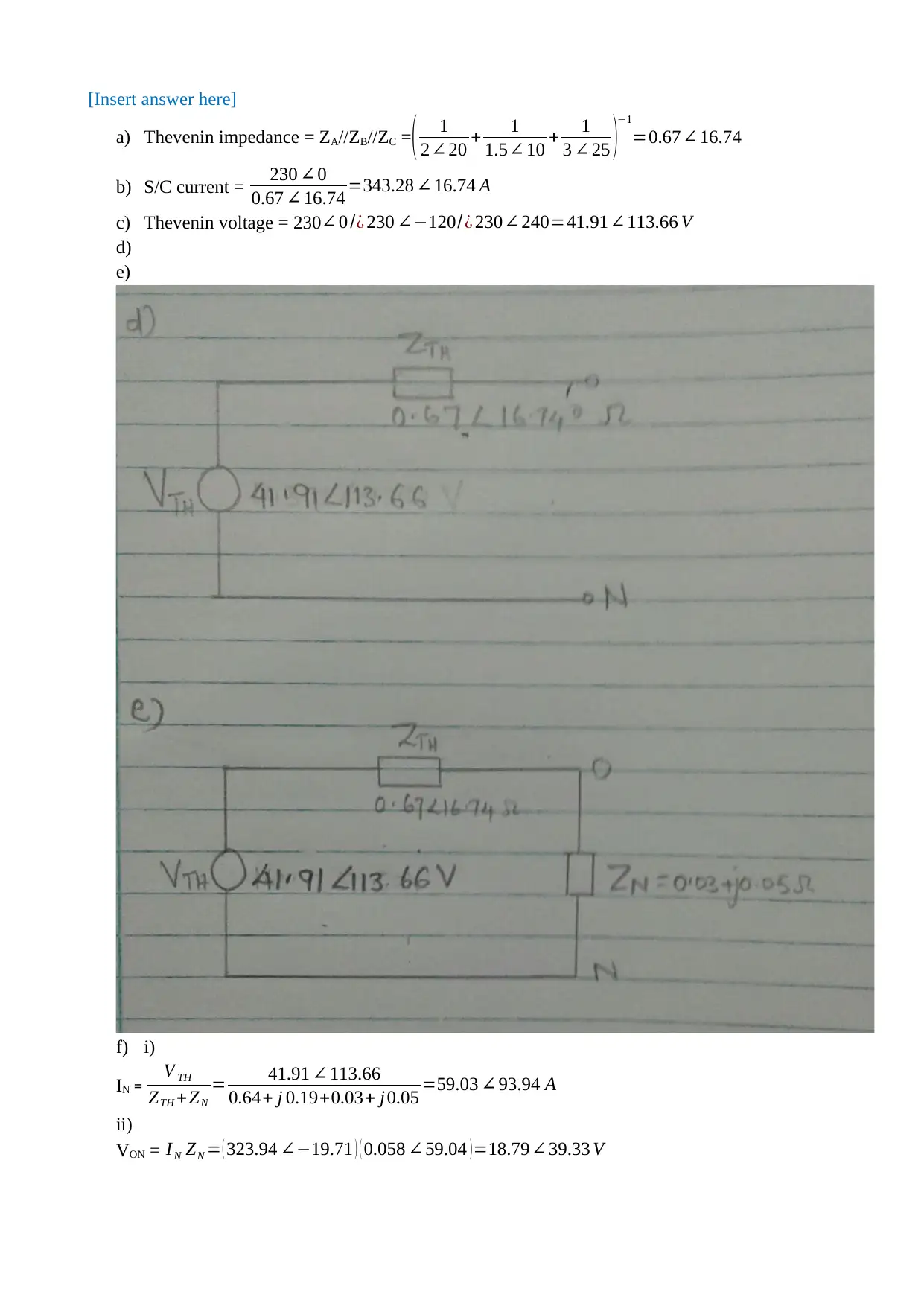
[Insert answer here]
a) Thevenin impedance = ZA//ZB//ZC =( 1
2∠20 + 1
1.5∠10 + 1
3 ∠ 25 )
−1
=0.67∠16.74
b) S/C current = 230 ∠0
0.67 ∠ 16.74 =343.28 ∠ 16.74 A
c) Thevenin voltage = 230∠ 0 /¿ 230 ∠−120/¿ 230∠ 240=41.91∠113.66 V
d)
e)
f) i)
IN = V TH
ZTH +ZN
= 41.91 ∠113.66
0.64+ j 0.19+0.03+ j0.05 =59.03 ∠ 93.94 A
ii)
VON = IN ZN = ( 323.94 ∠−19.71 ) ( 0.058 ∠59.04 ) =18.79∠39.33 V

iii)
IA = V A
Z A
= 230∠ 0
2∠20+ 0.058∠ 59.04 = 230 ∠0
2.04 ∠ 20.92 =112.75 ∠−20.92 A
IB = V B
ZB
= 230 ∠120
1.5∠ 10+ 0.058∠ 59.04 = 230∠120
1.54 ∠11.60 149.35∠108.4 A
IC = V C
ZC
= 230 ∠ 240
3 ∠25+0.058 ∠ 59.04 = 230 ∠ 240
3.05 ∠ 25.64 =75.41 ∠214.36 A
IA+ IB+ IC = 59.03 ∠ 93.95 A
END OF ASSESSMENT