Technical Selection of Penstock Material for Hydro-Electric Pumped Storage Piping System

This report discusses the design and selection of penstock material for a hydro-electric pumped storage piping system, including fracture assessment, NDT methods, and sensitivity tests. The report also includes steel material properties, design data, and fatigue assessment.

REPORT ON TECHNICAL SELECTION OF PENSTOCK MATERIAL
FOR HYDRO-ELECTRIC PUMPED STORAGE PIPING SYSTEM
PREPARED BY: ENG XYZ
DATED:

Contents
1. Introduction……………………………………………………………………………………………………3
2. Design and Selection……………………………………………………………………………………… 4
Design data……………………………………………………………………………………………….4
Design of the two steel material ………………………………………………………………
Fracture Assessment ……………………………………………………………………………….
NDT methods………………………………………………………………………………………….
3. Sensitivity Test………………………………………………………………………………………………11
Lifetime assessment………………………………………………………………………………..12
Revised assessment…………………………………………………………………………………14
4. Fatigue Assessment………………………………………………………………………………………14
5. Conclusion…………………………………………………………………………………………………….15

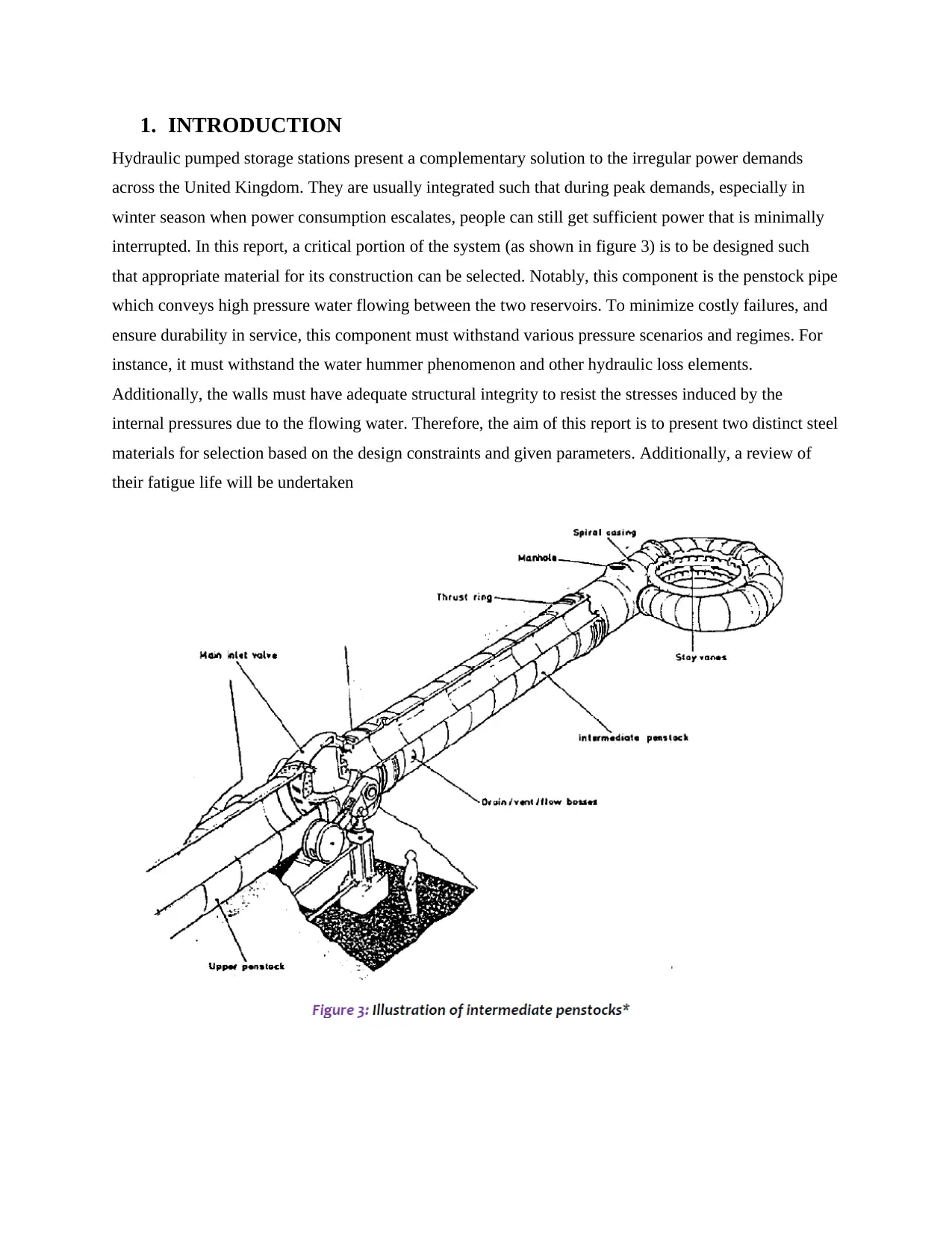
1. INTRODUCTION
Hydraulic pumped storage stations present a complementary solution to the irregular power demands
across the United Kingdom. They are usually integrated such that during peak demands, especially in
winter season when power consumption escalates, people can still get sufficient power that is minimally
interrupted. In this report, a critical portion of the system (as shown in figure 3) is to be designed such
that appropriate material for its construction can be selected. Notably, this component is the penstock pipe
which conveys high pressure water flowing between the two reservoirs. To minimize costly failures, and
ensure durability in service, this component must withstand various pressure scenarios and regimes. For
instance, it must withstand the water hummer phenomenon and other hydraulic loss elements.
Additionally, the walls must have adequate structural integrity to resist the stresses induced by the
internal pressures due to the flowing water. Therefore, the aim of this report is to present two distinct steel
materials for selection based on the design constraints and given parameters. Additionally, a review of
their fatigue life will be undertaken

2. DESIGN AND SELECTION
2.1 Design Data Provided
Table 1: Design Parameters
Crack length (mm) Pipe diameter (m) Static head (m) Water hummer (m)
3.0 2.5 501 765
Table 2: Steel Material Properties
PROPERTIES STEEL A QT 445 STEEL B BS150490LT50
C 0.15-0.21 <0.20
Si <0.90 0.10-0.50
S <0.04 <0.03
P <0.04 <0.03
Mn 0.80-1.10 0.9-1.60
Cr 0.50-0.80 <0.25
Mo 0.25-0.60 <0.10
Ni - <0.30
Zr 0.05-0.15 -
Cu - <0.30
B 0.0005 -
Nb - 0.01-0.06
Yield strength (MPa 700 350
UTS (MPa) 800 500
Fracture toughness Kic 100 130
Elongation (%) 18 20
Price of rolled plate (per
1000kg
965 525
Density (kg/m3) 9750 9750
2.2 Design of the two steel Material
It is noted that the maximum allowable stress (for the purpose of determining the thickness of
both steel materials) is fixed at 0.6 times the material yield strength. The penstock is normally
built in a similar fashion as the pressure vessels. Consideration is given for the thin walled
cylinders hence theories of hoop and circumferential stresses come in handy in this case:

Firstly, the wall thickness is determined by considering the loading conditions and the yield stress
Consider steel A:
The allowable design stress= Yield stress/factor of safety=0.6x 700= 420MPa
The effective head, he= hw+hwh= 501 + 765= 1266m
Next, internal pressure P in determined:
P= ρgh= 1000x 9.81x 1266= 12.419MN/m2
Radius of pipe= d/2= 2.5/2= 1.25m
Now, we assume the pipe is designed in a fashion similar to pressure vessel hence thickness
is given as:
Hoop stress (maximum principal stress) = Pr/t
Implying: tA= (1.25x12.419)/420 = 0.03696m=36.96mm
Now, considering Steel B:
The allowable design stress= Yield stress/factor of safety=0.6x 350= 210MPa
The effective head, he= hw+hwh= 501 + 765= 1266m
Next, internal pressure P is determined:
P= ρgh= 1000x 9.81x 1266= 12.419MN/m2
Radius of pipe= d/2= 2.5/2= 1.25m
Hoop stress (maximum principal stress) = Pr/t
Hence tB= (1.25x12.419)/210 = 0.07392m= 73.92mm
Steel B wall pipe will have to be thicker than that of steel A in order to withstand the same loading
conditions. Although, per a thousand kg, steel B is cheaper than steel A, however in the long run more
capital would be needed to purchase steel B material given the doubling of material quantity due to
increased thickness (Bannister, 1998).
In fact considering the cost implications:
Cost A and Cost B can be compared:
Cost A/Cost B= tA$A/tB$B
36.96x 96.5/52.5x73.92= 0.9190
This implies that Material A will cost about 92%relative to material B

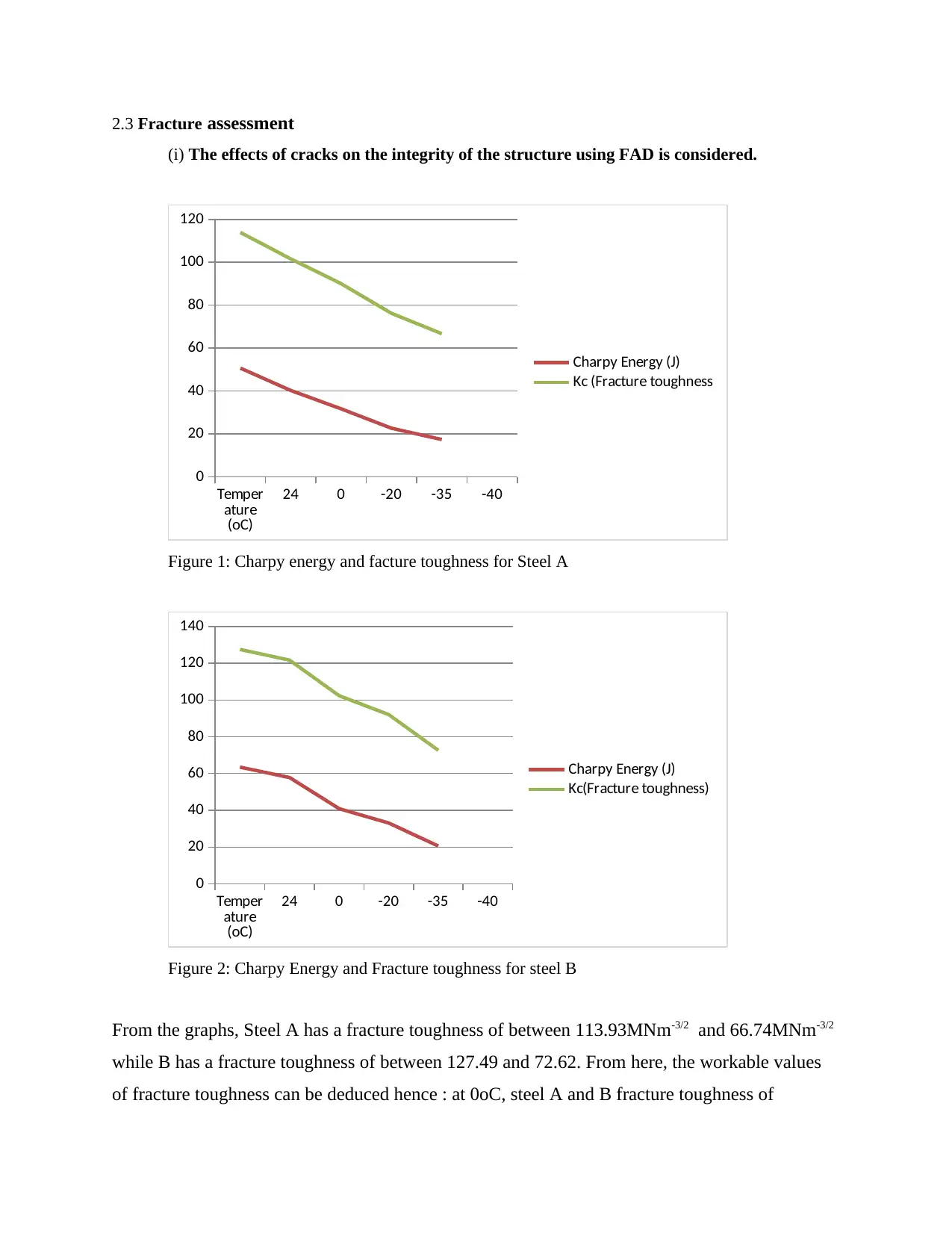
2.3 Fracture assessment
(i) The effects of cracks on the integrity of the structure using FAD is considered.
Temper
ature
(oC)
24 0 -20 -35 -40
0
20
40
60
80
100
120
Charpy Energy (J)
Kc (Fracture toughness
Figure 1: Charpy energy and facture toughness for Steel A
Temper
ature
(oC)
24 0 -20 -35 -40
0
20
40
60
80
100
120
140
Charpy Energy (J)
Kc(Fracture toughness)
Figure 2: Charpy Energy and Fracture toughness for steel B
From the graphs, Steel A has a fracture toughness of between 113.93MNm-3/2 and 66.74MNm-3/2
while B has a fracture toughness of between 127.49 and 72.62. From here, the workable values
of fracture toughness can be deduced hence : at 0oC, steel A and B fracture toughness of

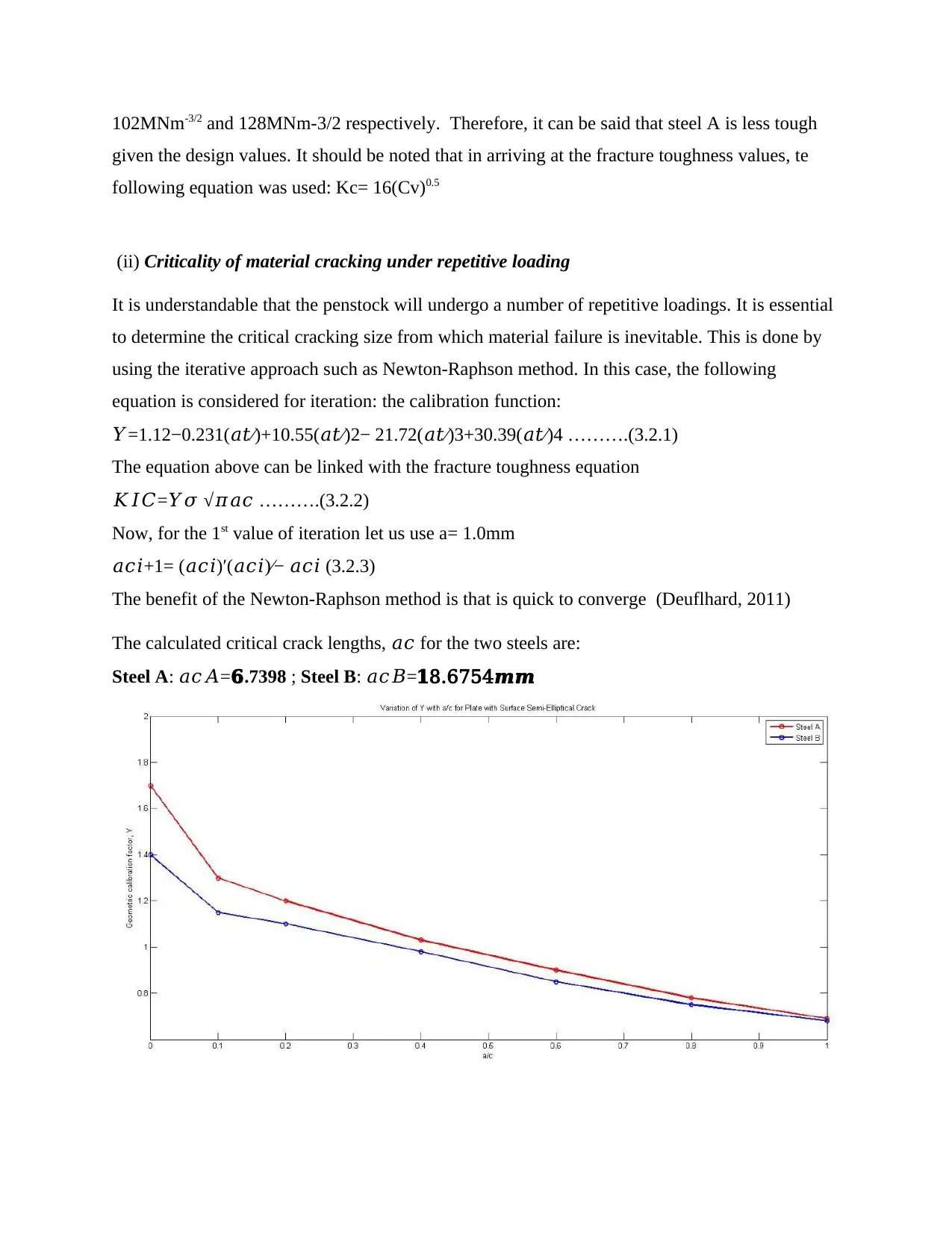
102MNm-3/2 and 128MNm-3/2 respectively. Therefore, it can be said that steel A is less tough
given the design values. It should be noted that in arriving at the fracture toughness values, te
following equation was used: Kc= 16(Cv)0.5
(ii) Criticality of material cracking under repetitive loading
It is understandable that the penstock will undergo a number of repetitive loadings. It is essential
to determine the critical cracking size from which material failure is inevitable. This is done by
using the iterative approach such as Newton-Raphson method. In this case, the following
equation is considered for iteration: the calibration function:
𝑌=1.12−0.231(𝑎𝑡⁄)+10.55(𝑎𝑡⁄)2− 21.72(𝑎𝑡⁄)3+30.39(𝑎𝑡⁄)4 ……….(3.2.1)
The equation above can be linked with the fracture toughness equation
𝐾𝐼𝐶=𝑌𝜎 √𝜋𝑎𝑐 ……….(3.2.2)
Now, for the 1st value of iteration let us use a= 1.0mm
𝑎𝑐𝑖+1= (𝑎𝑐𝑖)′(𝑎𝑐𝑖)⁄− 𝑎𝑐𝑖 (3.2.3)
The benefit of the Newton-Raphson method is that is quick to converge (Deuflhard, 2011)
The calculated critical crack lengths, 𝑎𝑐 for the two steels are:
Steel A: 𝑎𝑐𝐴=𝟔.7398 ; Steel B: 𝑎𝑐𝐵=𝟏8.6754𝒎𝒎

Since steel B gives a much bigger value of critical crack length, it can be stated that steel
B is structurally more resilient than A in terms of fracture toughness. The critical crack
size for Steel A is 𝟔.7398 mm, which is greater than 6mm, which is the assigned
detectable flaw size. With a total crack propagation length of only 0.7398mm, steel A
may not withstand repetitive loading as the critical crack size is nearer the design value of
6mm.
(iii) NDT methods and its accuracy
Accuracy of NDT Methods:
NDT methods like ultrasonic inspection often are used to detect flaws on the surface pipe
in a real-time fashion (Bannister, 1998). At minimum, a crack size of 6mm would be
perceived and this will facilitate repairs before cracking failure supersedes. Notably, with
time, the geometry calibration factor, Y would go down as the stress intensity ratio, Kr
and load ratio, Lr both increase with crack size. Thus assessment would focus on slightly
shifting along the failure curve so that crack size can be detected earlier than that of
6mm. Normally crack propagation is rapid and would bear catastrophic results if not
checked in real time. The NDT therefore provide tools of assessment of its status.
However, the accuracy of these methods would have to be increased so that chance of
failure can be fixed at the minimum level. For instance, as mentioned, one can reduce the
detectable flaw size by half which will then significantly thwart material failure (Hudson
& Rich,1986).
(iv) Failure assessment diagram (FAD) Analysis
The geometry calibration function factor for both steel materials A and B are: 𝑌𝐴=𝟏.7398
(𝑆𝑡𝑒𝑒𝑙 𝐴) 𝑌𝐵=𝟏.3𝟕𝟓𝟗 (𝑆𝑡𝑒𝑒𝑙 𝐵)
The resulting curve has an equation given by:
Y= 1.12-0.231t+10.67t2+ 30.39t3

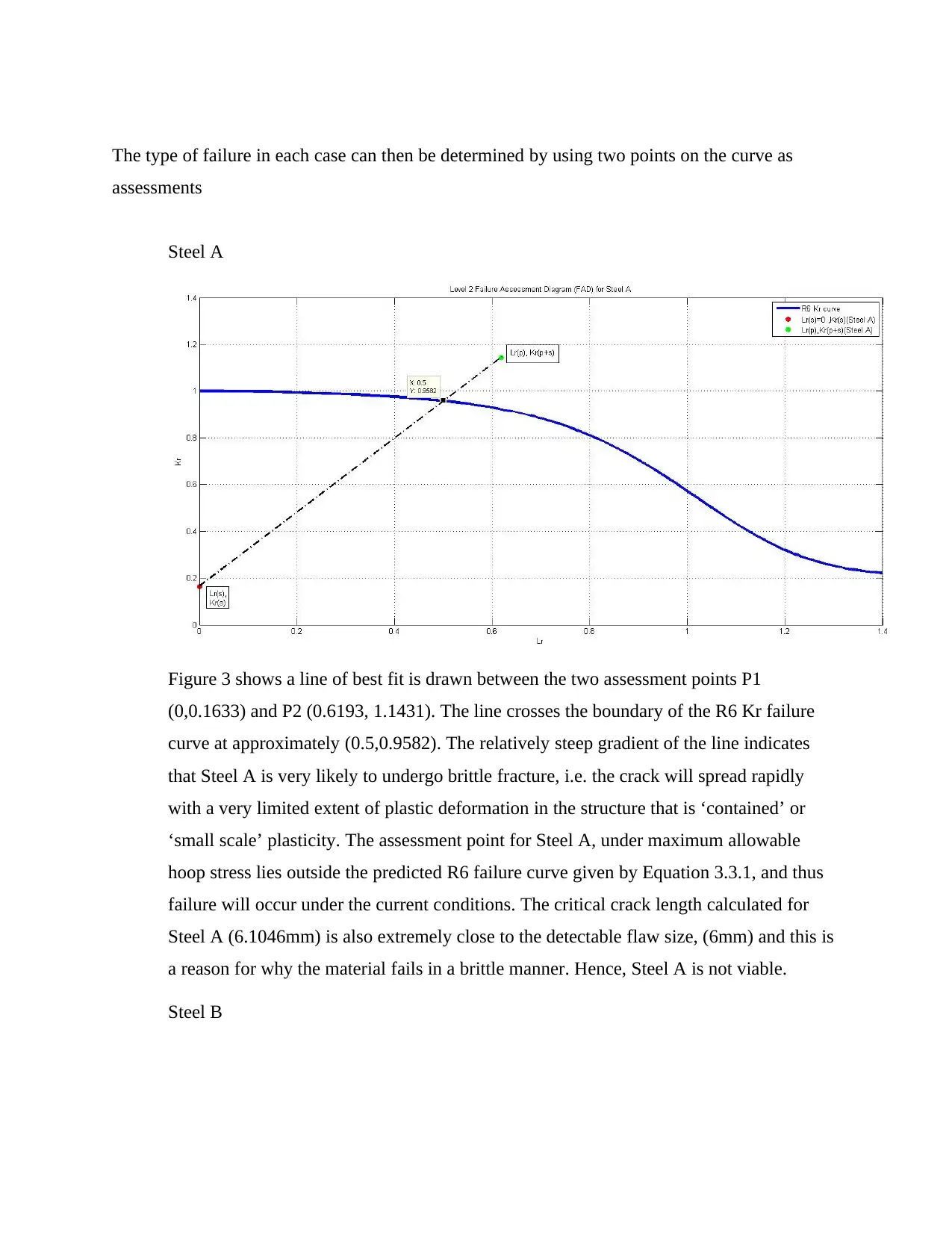
The type of failure in each case can then be determined by using two points on the curve as
assessments
Steel A
Figure 3 shows a line of best fit is drawn between the two assessment points P1
(0,0.1633) and P2 (0.6193, 1.1431). The line crosses the boundary of the R6 Kr failure
curve at approximately (0.5,0.9582). The relatively steep gradient of the line indicates
that Steel A is very likely to undergo brittle fracture, i.e. the crack will spread rapidly
with a very limited extent of plastic deformation in the structure that is ‘contained’ or
‘small scale’ plasticity. The assessment point for Steel A, under maximum allowable
hoop stress lies outside the predicted R6 failure curve given by Equation 3.3.1, and thus
failure will occur under the current conditions. The critical crack length calculated for
Steel A (6.1046mm) is also extremely close to the detectable flaw size, (6mm) and this is
a reason for why the material fails in a brittle manner. Hence, Steel A is not viable.
Steel B

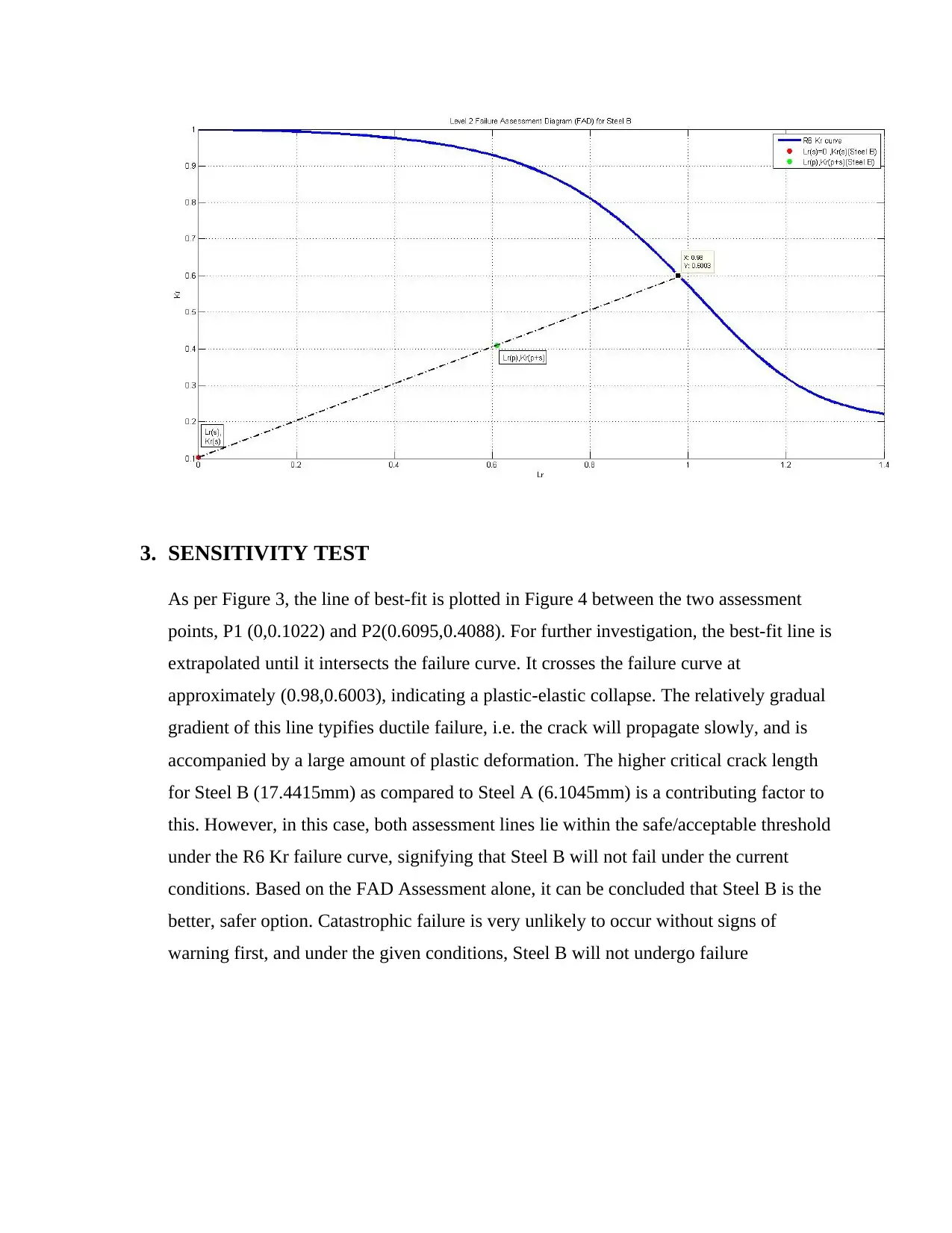
3. SENSITIVITY TEST
As per Figure 3, the line of best-fit is plotted in Figure 4 between the two assessment
points, P1 (0,0.1022) and P2(0.6095,0.4088). For further investigation, the best-fit line is
extrapolated until it intersects the failure curve. It crosses the failure curve at
approximately (0.98,0.6003), indicating a plastic-elastic collapse. The relatively gradual
gradient of this line typifies ductile failure, i.e. the crack will propagate slowly, and is
accompanied by a large amount of plastic deformation. The higher critical crack length
for Steel B (17.4415mm) as compared to Steel A (6.1045mm) is a contributing factor to
this. However, in this case, both assessment lines lie within the safe/acceptable threshold
under the R6 Kr failure curve, signifying that Steel B will not fail under the current
conditions. Based on the FAD Assessment alone, it can be concluded that Steel B is the
better, safer option. Catastrophic failure is very unlikely to occur without signs of
warning first, and under the given conditions, Steel B will not undergo failure

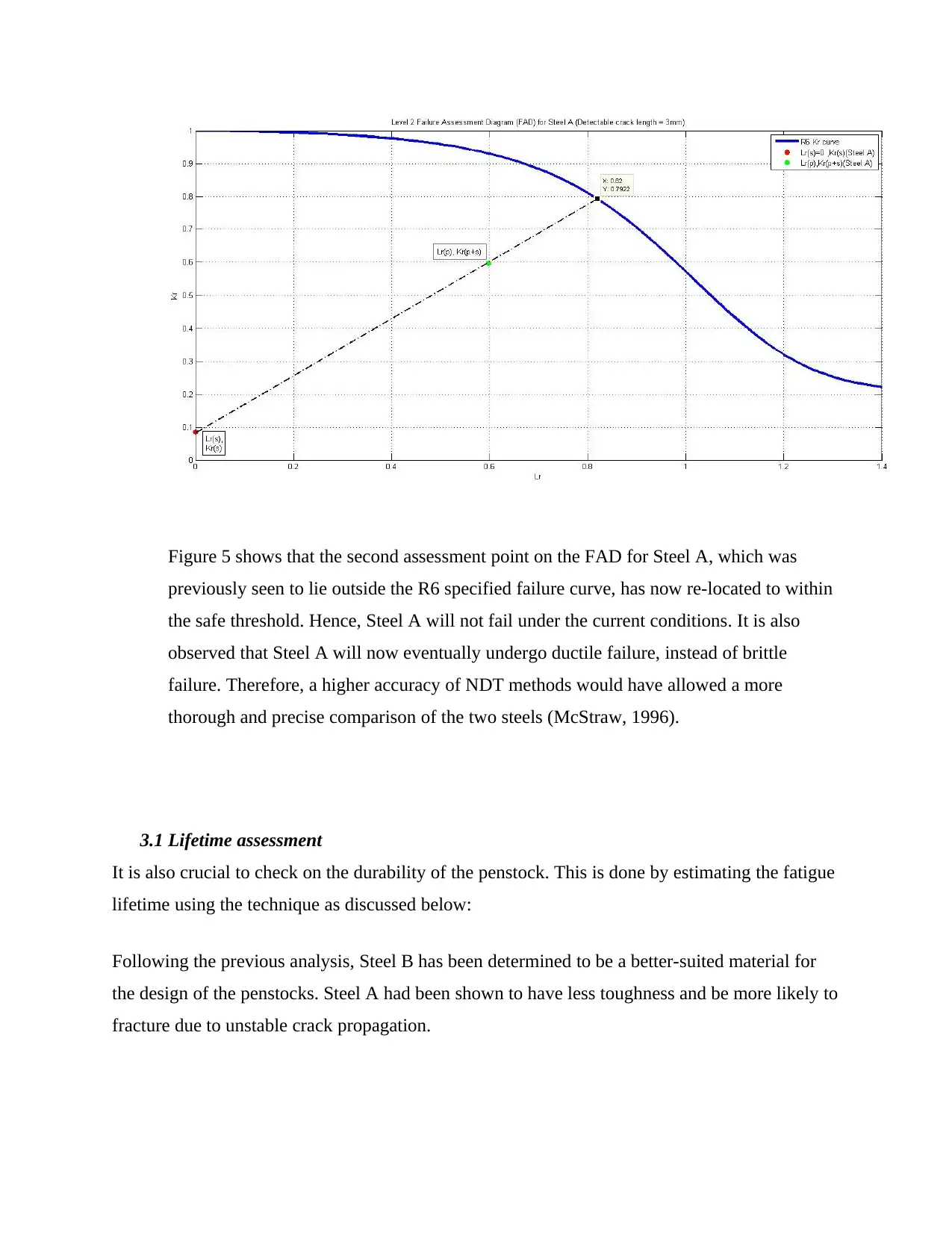
Figure 5 shows that the second assessment point on the FAD for Steel A, which was
previously seen to lie outside the R6 specified failure curve, has now re-located to within
the safe threshold. Hence, Steel A will not fail under the current conditions. It is also
observed that Steel A will now eventually undergo ductile failure, instead of brittle
failure. Therefore, a higher accuracy of NDT methods would have allowed a more
thorough and precise comparison of the two steels (McStraw, 1996).
3.1 Lifetime assessment
It is also crucial to check on the durability of the penstock. This is done by estimating the fatigue
lifetime using the technique as discussed below:
Following the previous analysis, Steel B has been determined to be a better-suited material for
the design of the penstocks. Steel A had been shown to have less toughness and be more likely to
fracture due to unstable crack propagation.

The crack propagation life can be estimated using the relation between the stress intensity range,
Δ and the crack growth rate (Paris’ Law)𝐾
The stress cycle arises from the pressure change from 0m to 525m head of water within the
pressure vessel. Using this information, the range of hoop stresses acting on the vessel can be
calculated.
…(4.1)
Likewise, for Steel A, Δ𝜎 is calculated to be 29.4 MPa.
The stress intensity range, Δ can be calculated using the value of Δ𝜎, using:𝐾
The change in crack sizes, ‘a’ can be calculated with respect to the number of cycles, ‘N’ by use
of Paris’ Law
By utilizing a small step size, (Δ ), an iterative numerical integration can be carried out to𝑎
determine the solution. The initial crack size, is equivalent to the given detectable crack/flaw𝑎𝑖
size of 6mm. The final crack size, , will be greater than the critical crack size, of the steel, as𝑎𝑐
calculated previously:

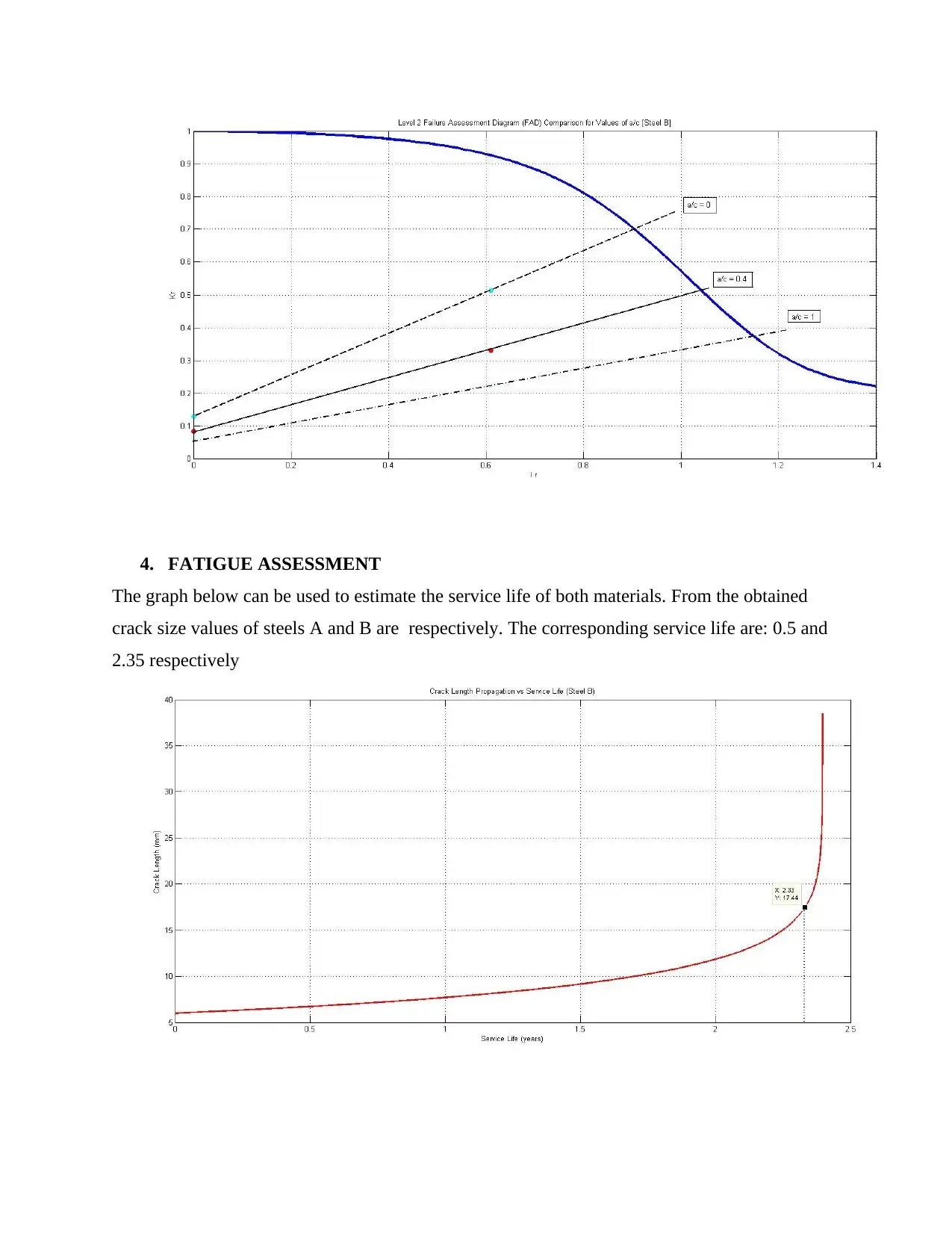
4. FATIGUE ASSESSMENT
The graph below can be used to estimate the service life of both materials. From the obtained
crack size values of steels A and B are respectively. The corresponding service life are: 0.5 and
2.35 respectively

.
The crack length propagation plot in Figure 10 shows that the time taken for the initial crack
to propagate to critical size within Steel B is 2.35 years, well below the required service life
of 50 years. Design improvements are to be made accordingly. The fatigue life plot for Steel
A predictably shows that failure due to fatigue will occur very quickly. However, it is noted
that the toughness data provided is for the parent material yet the point of propagation of
cracks are likely to be in the heat affected zones (Molzen & Hornbach, 2000).
5. CONCLUSION
Notably, the internal pressure P acting on the walls on the pipe greatly contributes to cracking.
However, the penstock is treated as a thin walled cylinder such that variations of stresses are not
considered. There are points along the pipe when stress would be maximum than the design
value. In order to accommodate these changes, the design engineer would design the pipe with
some factor of safety so as to cushion against catastrophic failure.
Now, there are techniques that are used to calculate the crack size. Matlab can be useful
especially for iteration. However, LEFM can be used as well although it is not strictly applicable
to the section thicknesses of Steels A and B, for values of K approaching KIC, as the plastic zone
at the crack tip is significant. By integrating the plastic zone correction factor, smaller values of
the critical crack length would be obtained. The size of the crack tip can either be calculated
using Irwin’s model, which estimates the elastic-plastic boundary using elastic stress analysis,
and the strip-yield model (O'Brien, 2015)
In the section ‘Sensitivity Analysis’, it was shown that the risk of failure could be substantially
reduced by improving the accuracy of the Non-Destructive Testing (NDT) methods. The
minimum detectable crack length should be reduced to 3mm, as this ensures that both Steels A
and B will not undergo failure under given conditions. Steel A can therefore be compared with
Steel B in a more critical and thorough manner and a more informed choice can be made. The
reduction of minimum detectable crack length also allows a more accurate estimation of crack

propagation, so that risks of failure can be identified in advance and dealt with accordingly
(Saadat, 2015).
The section on Fatigue Assessment does not take into account the damage caused by any
variation in the number of cycles, Nf. Variation in size, number and order of stress cycles will
lead to cumulative fatigue damage of the penstock. Therefore, fatigue damage must be evaluated
by adding the detrimental effects of each individual cycle, to ensure that any chance of failure is
minimized.
Cracks are to be repaired when they are approximately equal to 15mm, thus providing a safety
window of about 12 years to accommodate for the risk of failure. Maintenance of cracks of
smaller lengths than 15mm will increase the operational cost of the station, without any
substantial increase in safety.
The inspection frequency for the intermediate penstock must be between 1 and 5 years, but no
more than 5 years . Therefore, the inspection interval should be co-ordinate with the maintenance
interval, i.e. stripping of the walls and repainting.
REFERENCE
Anderson T. (2005). Fracture Mechanics. Hoboken: CRC Press.
Bannister A. (1998). Structural integrity assessment procedures for European Industry. Swindon:
British Steel plc
Deuflhard P. (2011). Newton Methods for Nonlinear Problems: Affine Invariance and Adaptive
Algorithms.
Hudson, C & Rich,T. (1986). Case histories involving fatigue and fracture mechanics.
Philadelphia, PA: ASTM.
Jivkov A. 2015. The Design of a Penstock Pipe for a Hydro-electric Pumped Storage Station. 1st
ed
Kaechele L. (1963). Review and Analysis of Cumulative Fatigue-Damage Theories.

McStraw, B. (1996). Inspection of Steel Penstocks and Pressure Conduits. United States
Department of the interior bureau of reclamation denver, colorado.
Molzen, M & Hornbach, D. (2000). Evaluation of Welding Residual Stress Levels Through Shot
Peening and Heat Treating. 1st ed.
O'Brien R. Welding handbook. (1991). Miami, Fla.: American Welding Society.
Saadat H. 2015. Power System Analysis [Internet]. Psapublishing.com. 2015 [cited 20
November. Available from: http://www.psapublishing.com/