Design of Plate Girder and Analysis of Structural Mechanisms

This article discusses the design considerations for plate girders, including depth and flange design. It also covers the analysis of structural mechanisms, including sway and beam mechanisms, and provides solved assignments, essays, and dissertations on the topic. Course codes, names, and universities are not mentioned.

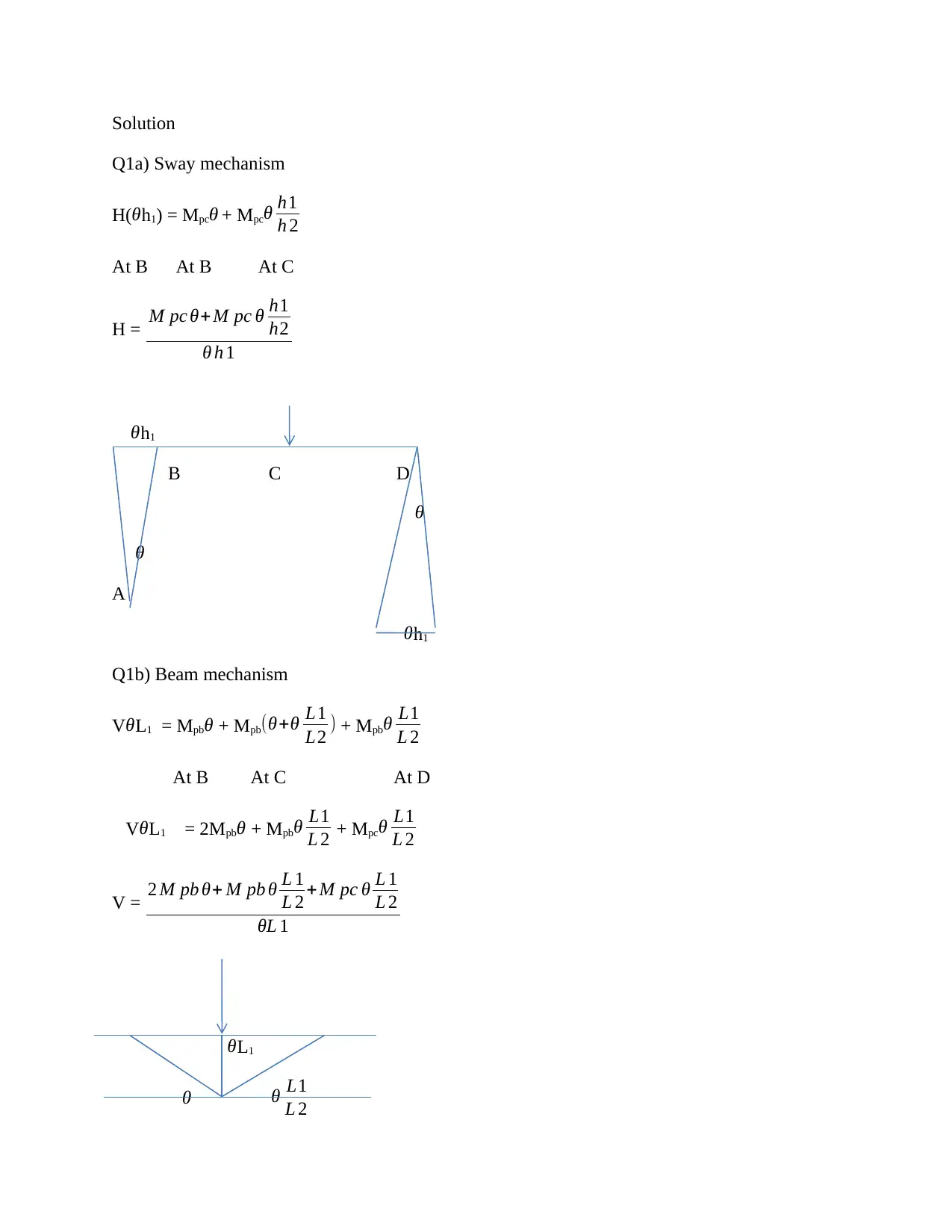
Solution
Q1a) Sway mechanism
H(θh1) = Mpcθ + Mpcθ h1
h 2
At B At B At C
H = M pc θ+ M pc θ h1
h2
θ h 1
θh1
B C D
θ
θ
A
θh1
Q1b) Beam mechanism
VθL1 = Mpbθ + Mpb(θ+θ L1
L2 ) + Mpbθ L1
L 2
At B At C At D
VθL1 = 2Mpbθ + Mpbθ L1
L 2 + Mpcθ L1
L 2
V = 2 M pb θ+ M pb θ L 1
L 2 +M pc θ L 1
L 2
θL 1
θL1
θ θ L1
L 2

Q1c) i) Combined sway
δW1 = Hθh1 + VθL1
= 2Mpbθ + Mpbθ L1
L 2 + Mpcθ L1
L 2 + Mpcθ + Mpcθ h1
h 2 - Mpcθ - Mpbθ
H(θh1) + VθL1 = Mpbθ + Mpbθ L1
L 2 + Mpcθ L1
L 2 + Mpcθ h1
h 2
Substituting
5*H* θ + V¿ θ*3 = 240*θ + 240* θ∗3
2 + 120* θ∗3
2 + 120*θ 5
3
5H θ + 3Vθ = 980 θ
ii) Interaction diagram
sway diagram
θh1
B C D
θ
θ
A
θh1
Beam diagram
θL1

θ θ L1
L 2
iii) Equilibrium
D Mpc
E HE
∑ M about D = 0
3HE – Mpc = 0
HE = Mpc
3 = 120
3 =40 KN
∑ Fx = 0
∑ M about E = 0
-2V + 2H + 3H = 0
5 * 80 = 2V
V = 200 KN
Thus the moment at E, from a free body diagram of ABC
∑ M About C = 0
2VA + 5HA – Mc = 0
Mc = 240KN.m
Since there is a plastic hinged at C of value MpC = 240 KNm, we have the equilibrium
H = 256 KN
V = 300 KN

Mpc = 120 KN
Mpb = 240 KN
L1 = 2, L2 = 3, h2 = 3, H1= 5 M
∑ M About C = 0
2*300 + 5*256 – Mc 0
Mc = 1880 KNM
∑ M About C = 0
3HE – 120 = 0
HE = 40 KN
Moment at B
256 *2.5 = 640 KNm
Moment at D
1880 – 300 *3 = 980 KNm
BMD
640 KNm 120 KNm
B C D
640 KNm
A 1880 KNm
Part B
Span of the welded girder = 15 m

It carries a load of approximately 500 KN each
The loads are at a distance of 4 m from each end of the girder
The girder carries uniform distributed load of 30 KN/m, which include the self-weight of girder
Designing the girder
500 KN 30 kn/m 500 KN
A C E D B
5 m 5 m 5 m
∑ M B = 0
RA * 15 = 500*10 + 500*5 + 20*15 * 15/2 = 650 KN
RB = 500 + 500 + 30 * 15 – 650 = 800 KN
Mc = 650 * 5 – 30 * 5 * 2.5 = 2875 KNm
MB = 650 * 7.5 – 500 * 2.5 – 30 *7.5 * 7.5/2 = 2781.25 KNm
The overall depth of girder = span/10 = 1500 mm
Assume that the girder has 30 mm thick therefore the allowance Pb = 140 N/mm2
If we assume that the flange plate resist the bending moment then the moment will be resisted by
lever arm of about 1460 mm
Flange area = (2781.25 *106)/(1460 *140) = 1.36 * 104 mm2
If we assume the flange that was used is of dimensions 150 mm by 100 mm
Assume the allowance shear stress is 100 N/mm2
Depth of web plate is 1300 mm and shear 650 KN at girder support
Thickness of web plate = (650 *103)/(1300 *100) = 4.64 mm
If a thickness of 20 mm was used
Checking the bending stress

IXX = (2 * 100 * 150 * 7002) + (20 *13003)/12 = 1.84 *1010 mm4
fbc = (2781.25 *106 *650)/ (1.84 * 1010) = 98.25 N/mm2 (it is safe)
load bearing stress are required at the support under the point loads
The spacing should not exceed
1.5d = 11950 mm
180t = 180 *20 = 3600
Stiffeners under 500 KN
Assuming the stiffeners each 100 mm by 30 mm
Bearing stress = (500 * 103)/(2*135 * 30) = 61.72 (safe )
The area of centerline of web
IXX = (30 * 3003)/12 = 6.75 * 107 mm4
A = (300 * 20) + (100 * 30*2) = 12, 000 mm2
rXX = √ 6.75∗107
12000 = 75 mm
l/rXX = (0.7 *1300)/75 = 12.1
Pc = 149.95 N/mm2
Fc = (650 *103)/(12000) = 54.17 N/mm2
A smaller size stiffener was used
End plate
Assume the end plate of 20 mm thick was used
Checking the bearing
Bearing stress = (650 * 103)/(450 *10) = 144.4 (safe )
Checking bthe section acting at the strut
A = 450 * 10 + (200 *10) = 6500 mm2
IXX = (10 * 4503)/12 = 7.6 * 107 mm4

rXX = √ 7.6∗107
6500 = 108.1 mm
l/rXX = (0.7 *1300)/108.1 = 8.4
Pc = 150.8 N/mm2
Fc = (650 *103)/(6500) = 100 N/mm2
Assume the weld stretch = 350 N/mm
Approximately the welded length = (650 *103)/350 = 1875 mm
Part B (ii)
Minimum yield strength at nominal thickness 16 mm
For steel S275 = 275 N/mm2
Tensile strength between 3 mm and 16 mm = 370 to 530 Mpa
Dead load
s.w = 20 KN
point load w1d = 200 KN, w2d = 200 Kn
imposed load
udl = 40 KN/m
point load, w1d = 300 KN, w2d = 300 KN
720 KN 40 kn/m 720 KN
B E K G J
9 m 7 m 9 m

Total weight = 1.2 D.L + 1.6 L.L
Load w1d = 1.2*200 + 1.6*300 = 720 KN
Load w2d = 1.2 * 200 + 1.6* 300 = 720 KN
∑ MJ = 0
RB * 25 = 720*16 + 720*9 + 40*25 * 25/2 = 1220 KN
RJ = 720 + 720 + 40 * 25 – 1220 = 1220 KN
ME = 1220 * 9 – 40 * 9 * 4.5 = 9360 KNm
MK = 1220 * 12.5 – 720 * 3.5 – 40 *12.5 * 12.5/2 = 9605 KNm
Girder section
The overall depth of girder = span/10 = 25000/10 = 2500 mm
Take the cover to be 40 mm thick therefore the allowance stress bending Pb = 275N/mm2
If we assume that the flange plate resist the bending moment then the moment will be resisted by
lever arm of about 2460 mm
Flange area = (9605 *106)/(2460 *275) = 1.42 * 104 mm2
If we assume the flange that was used is of dimensions 300 mm by 50 mm
Depth of web plate is 2400 mm and shears 1220 KN at girder support
Thickness of web plate = (1220 *103)/(2400 *100) = 5.08mm
If a thickness of 10 mm was used
Checking the bending stress
IXX = (2 * 50 * 300 * 12252) + (10 *24003)/12 = 1.156 *1010 mm4
fbc = (9605 *106 *1200)/ (1.156 * 1010) = 997 N/mm2 (it is safe)
for the web the ratio d/t = 2400/10 = 240 the for the intermediate stiffener must be provided
Load bearing stress is required at the support under the point loads

The spacing should not exceed
1.5d = 3600 mm
180t = 180 *10 = 1800
From the table of allowable shear stress assume 100 N/mm2
Stiffeners under 720 KN
Try two stiffeners each 200 mm by 20 mm
Bearing stress = (720 * 103)/(2*175 * 20) = 103 N/mm2 (safe )
The area of centerline of web
IXX = (20 * 3303)/12 = 6.0 * 107 mm4
A = (500 * 10) + (200 * 20*2) = 13, 000 mm2
rXX = √ 6.0∗107
13000 = 67.94 mm
l/rXX = (0.7 *2400)/67.94 = 24.7
Pc = 142.4 N/mm2
fc = (720 *103)/(13000) = 55.38 N/mm2
it is advisable to use smaller size stiffener
take weld strength approximately to 450 N/mm
length = (720*103)/450 = 1600 mm
End plate
Assume the end plate of 20 mm thick was used
Checking the bearing

Bearing stress = (1220 * 103)/(500 *20) = 22 N/mm2
Maximum stiffener = 11t = 11 * 20 = 220 mm (safe)
Checking the section acting at the strut
A = 500 * 20 + (1900 *10) = 11.9 * 103 mm2
IXX = (20 * 5003)/12 = 20/83 * 107 mm4
rXX = √ 20.83∗107
11900 = 132.3 mm
l/rXX = (0.7 *2400)/132.3 = 12.7
Pc = 147.2 N/mm2
Fc = (1220*103)/ (11900) = 102.5 N/mm2
Try the weld strength = 500 N/mm
Approximately the welded length = (1220 *103)/500 = 2440mm
Intermediate stiffeners
Use stiffeners of dimensions of each 100 mm by 10 mm
Maximum value = 10t = 10 *20 = 200
Moment of inertia = I = (10 *2103)/12 = 7.73 * 105 mm4
Distance between stiffeners = 1800 mm
Required thickness of web = 1300/ 180 = 7.22 mm
For shear strength, t = 5.08
I = (1.5 *12003 *7.353)/(104 *18002) = 3.2 *106 mm
Web to flange web
Horizontal shear per weld
= (1220 *103 *300 *50 *625)/(1.156 *1010 *2) = 495 N/mm ( hence ok)

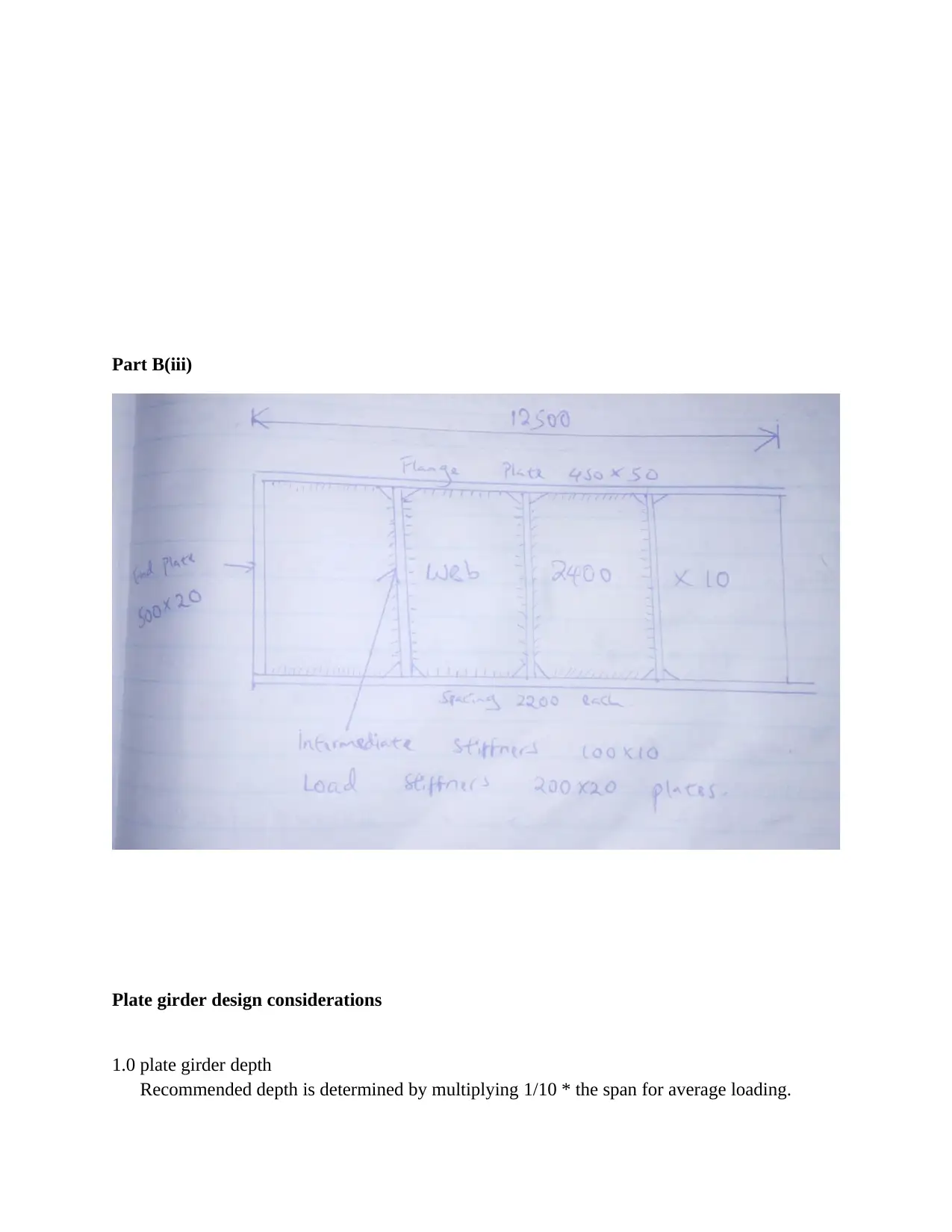
Part B(iii)
Plate girder design considerations
1.0 plate girder depth
Recommended depth is determined by multiplying 1/10 * the span for average loading.

The depth should be between 1
15 ¿ 1
20 if one is dealing with lightly loaded girders
In determination of the flange depth we multiply the overall depth by 1/3
Stiffeners are used to prevent Web buckling
2.0 plate girders deflections
The deflection of the plate girder is determined by referring to Clause 15 of BS449 in order to
determine the deflection requirement
3.0 permissible stresses
According to BS449 it is recommended that whenever the plate thickness will exceed 40 mm
a lower stress must be used
The allowable permissible bending stress to be used are provided in BS 449
4.0 Considering the types plate girder
Plate girder construction involves use of welded steel plate which together will form an I
section
5.0 Bending stresses
Bending stresses will be determined by referring to Clause 17, 27 and 32 of BS 449 sets
section area for the girders
In consideration all the characteristics of the plate girders that includes moment of inertia,
area, modulus of section and radii of gyration are calculated from the first principle.
Refer to BS 449 in determining maximum outstand for flange plate,
Where,
Compression flange = 16t
Tension flange = 20t
t is the thickness
6.0 Stresses and loads
Loads subjected to the plate girder are from stanchions, floor slab and floor beams, in which
it is carried by the girder
Whereas, flange of the plate will carry the bending moments while the web will have a
resistance to the shear force.

To avoid plate from failing and working effectively the rules from BS449 will be used to
govern the with or thickness ratios of the plates to ensure no buckling takes place, the rules
also will govern the positions of both the intermediate and stiffeners.
7.0 Shear stress of the web
Shear stress of the web is determined by the formula
fq = shear
depthof web plate∗thickness of the web
Allowable shear stress will depend on the value of d/t and stiffeners spacing
If the ratio of d/t more than 85 vertical stiffeners will be required at a distance which will not
exceed 1.5d
The thickness should be more than 1
180