Pre-Calculus Homework Assignment: Chapter 2 and Radical Functions
VerifiedAdded on 2023/06/09
|16
|1592
|352
Homework Assignment
AI Summary
This Pre-Calculus assignment solution covers a range of topics, including functions, graphing, and equations. The document provides solutions to multiple-choice questions, graphing problems involving transformations and determining domain and range. It also includes solutions for radical functions, polynomial equations, factoring, and solving for variables. The assignment further explores concepts such as the area of a tennis ball, inverse functions, and sketching graphs. Furthermore, it provides worked solutions for various problems, including factoring cubic and quartic equations and determining the dimensions of a swimming pool. The document is designed to aid students in understanding and solving complex Pre-Calculus problems.
1 out of 16



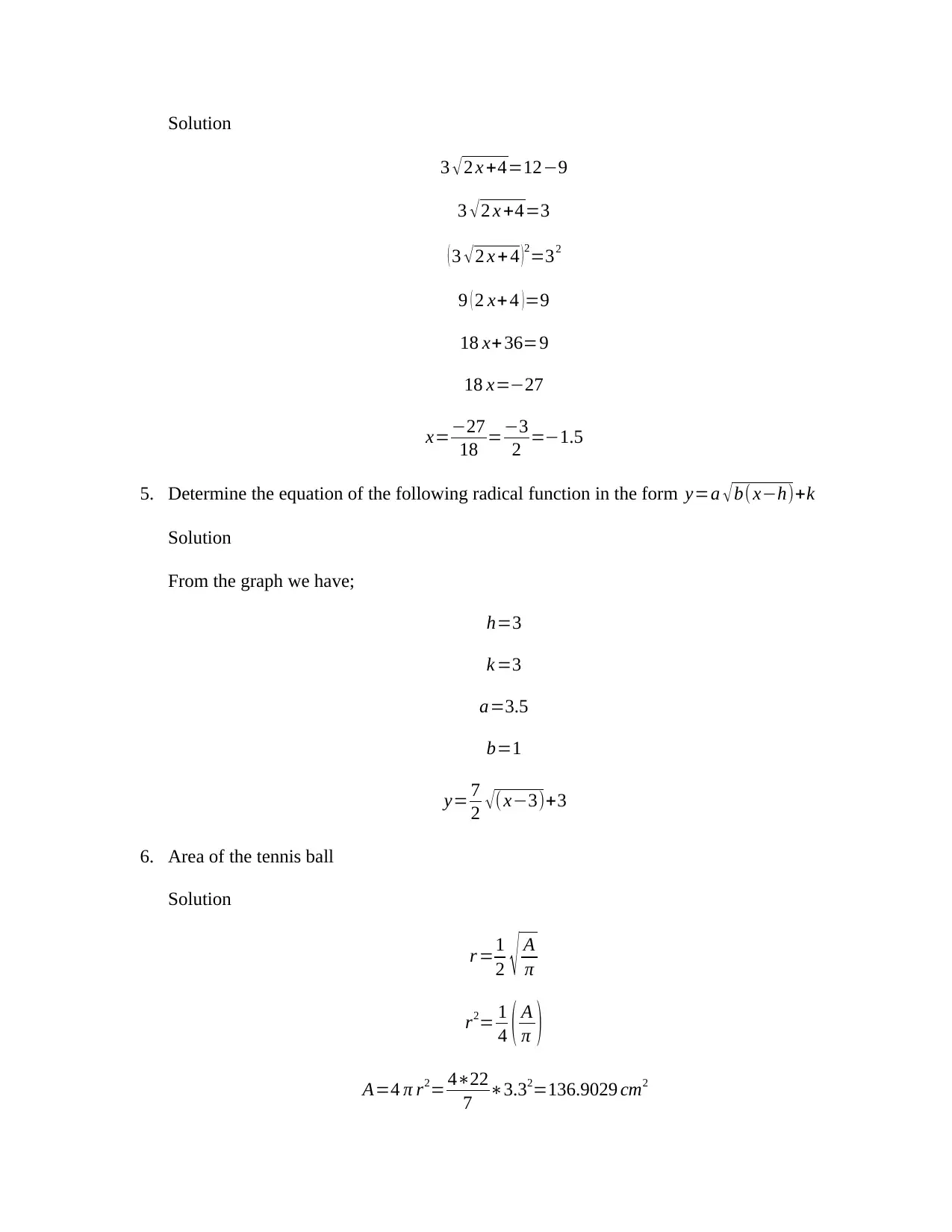
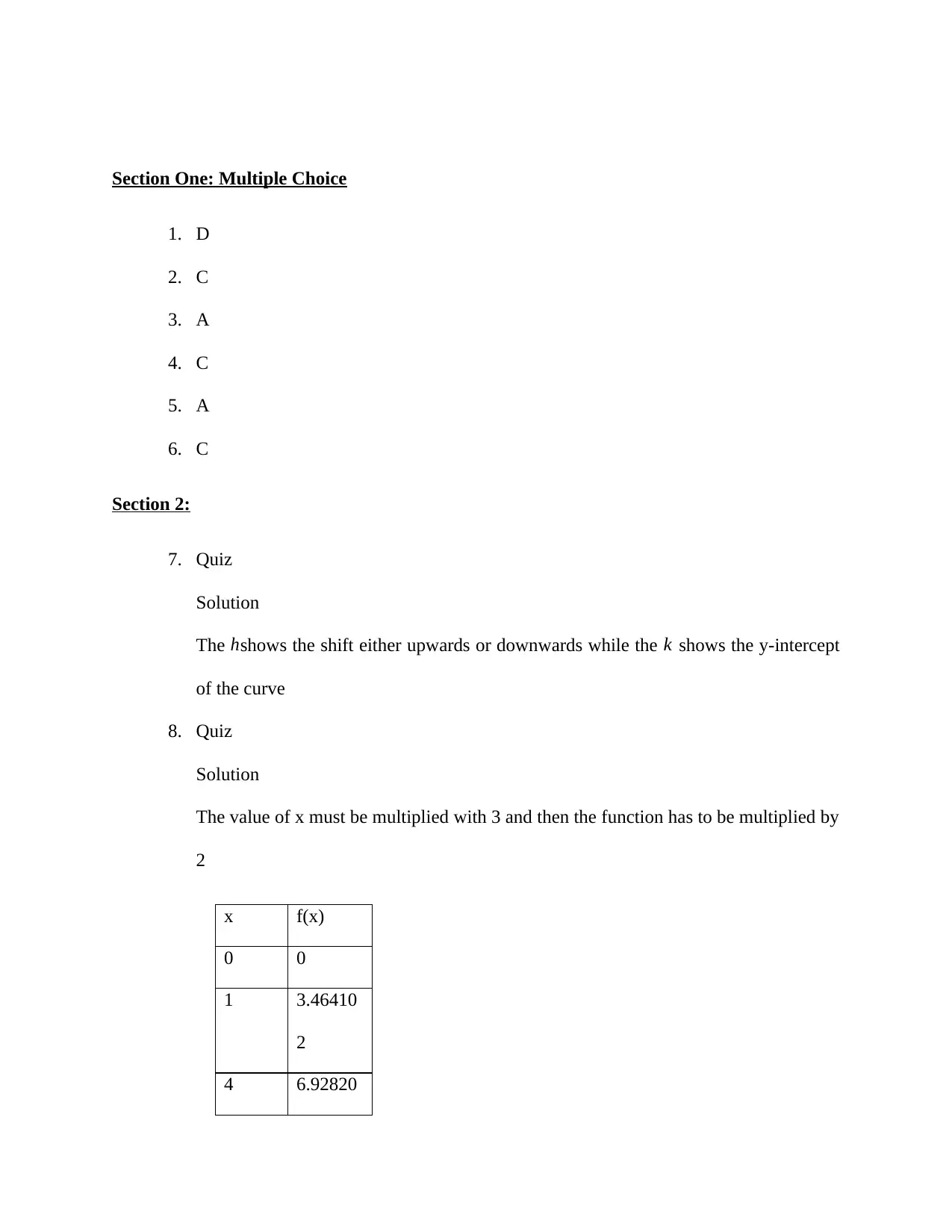
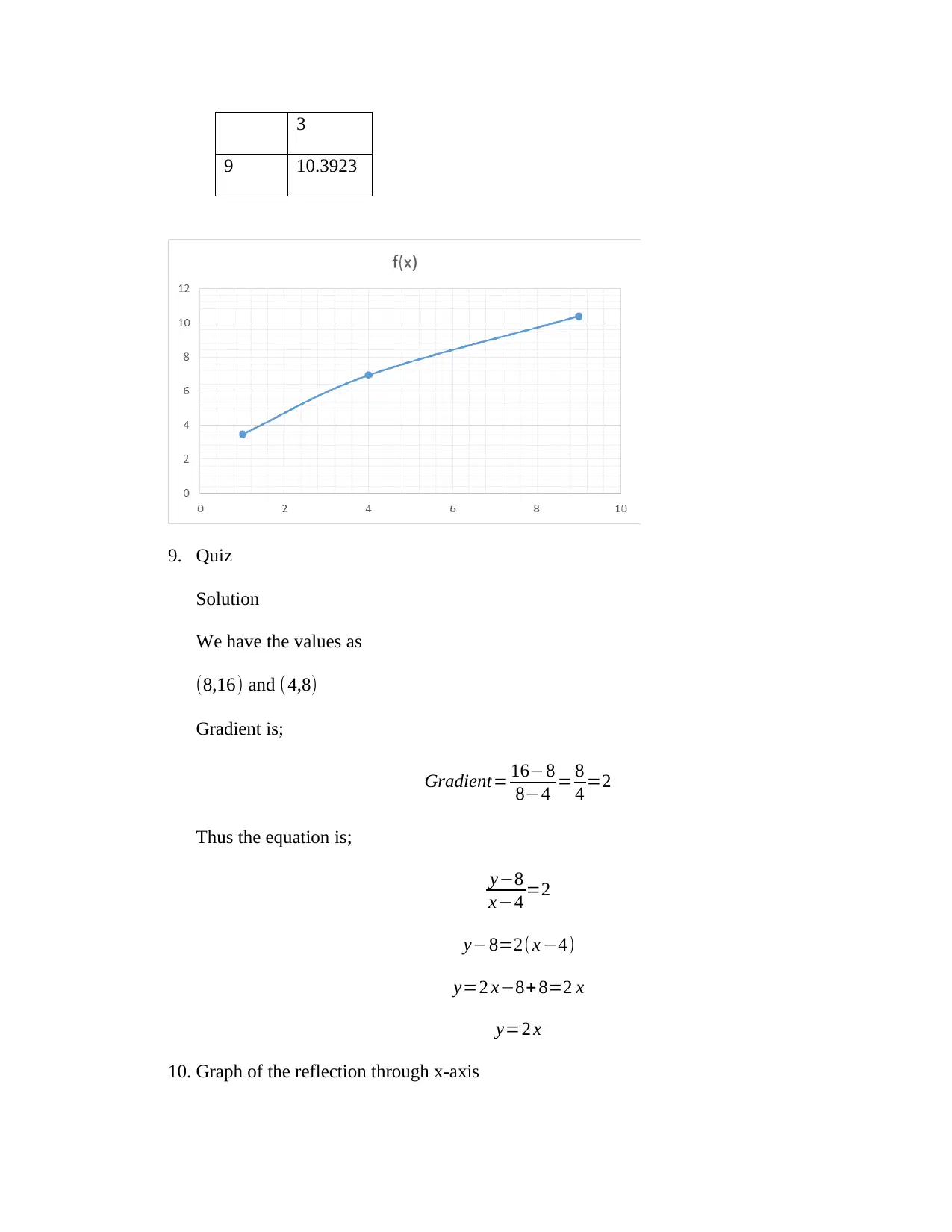
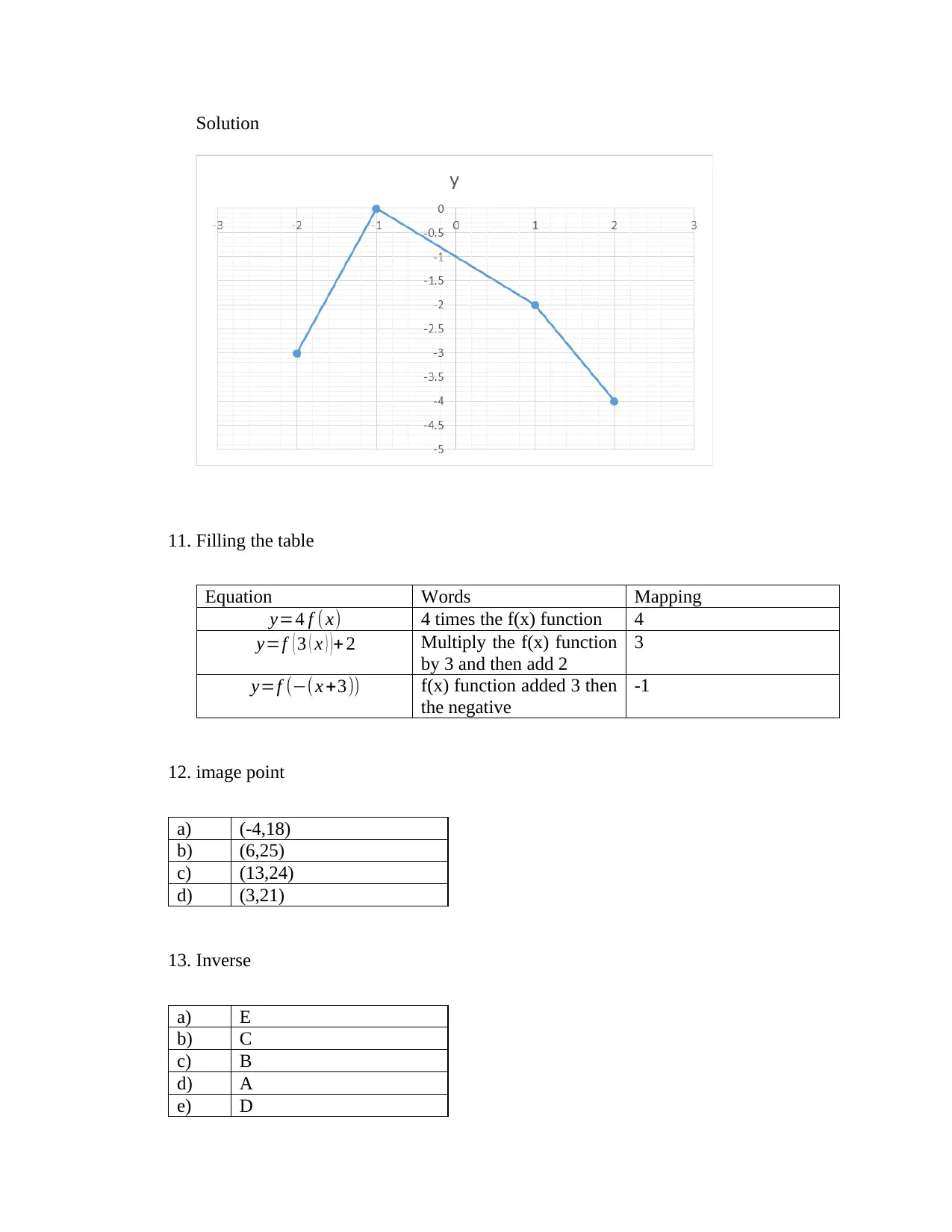





![[object Object]](/_next/static/media/star-bottom.7253800d.svg)