Probability Assignment - Statistical Probability and Genetic Analysis

This probability assignment explores various concepts including coin flips, probability calculations, and genetic disease analysis. The assignment begins with coin flip scenarios, calculating probabilities for different outcomes like getting heads at least four times or consecutive heads. It then delves into ...Read More

Probability 1
PROBABILITY
by Student’s Name
Code + Course Name
Professor’s Name
University Name
City, State
Date

Probability 2
Probability
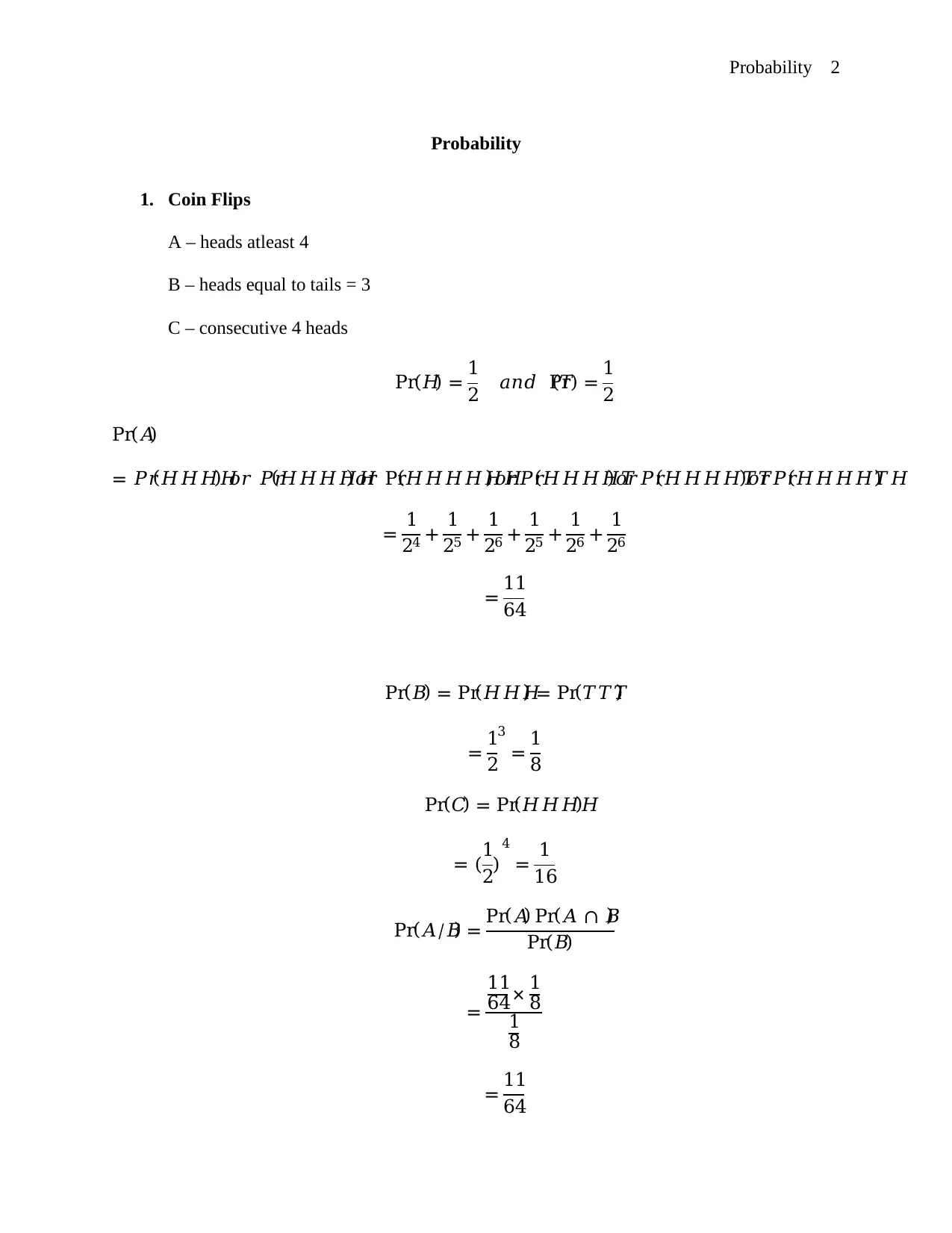
1. Coin Flips
A – heads atleast 4
B – heads equal to tails = 3
C – consecutive 4 heads
Pr(𝐻) = 1
2 𝑎𝑛𝑑 Pr(𝑇) = 1
2
Pr(𝐴)
= 𝑃𝑟(𝐻𝐻𝐻𝐻) 𝑜𝑟 𝑃𝑟(𝐻𝐻𝐻𝐻𝐻)𝑜𝑟 Pr(𝐻𝐻𝐻𝐻𝐻𝐻)𝑜𝑟𝑃𝑟(𝐻𝐻𝐻𝐻𝑇)𝑜𝑟𝑃𝑟(𝐻𝐻𝐻𝐻𝑇𝑇)𝑜𝑟𝑃𝑟(𝐻𝐻𝐻𝐻𝑇𝐻)
= 1
24 + 1
25 + 1
26 + 1
25 + 1
26 + 1
26
= 11
64
Pr(𝐵) = Pr(𝐻𝐻𝐻) = Pr(𝑇𝑇𝑇)
= 1
2
3
= 1
8
Pr(𝐶) = Pr(𝐻𝐻𝐻𝐻)
= (1
2)
4
= 1
16
Pr(𝐴/𝐵) = Pr(𝐴) Pr(𝐴 ∩ 𝐵)
Pr(𝐵)
=
11
64× 1
8
1
8
= 11
64

Probability 3
Pr(𝐶/𝐴) =
Pr(𝐶) Pr(𝐶 ∩ 𝐴)
Pr(𝐴)
=
1
16× 1
16
11
64
= 1
44
2. Probability of 5 before 7
A – d1 + d2 = 7
B – d1 + d2 = 5
C - 𝐴 ∪ 𝐵
Pr(1, … ,6) = 1
6
The first appearance of A = 1 and 6 or 2 and 5 or 3 and 4 or 4 and 3
While first appearance of B = 1 and 4 or 2 and 3 or 3 and 2 or 4 and 1
𝐴 ∪ 𝐵 = (1,6)(1,5)(1,4)(2,3)(2,4)(2,5)(3,2)(3,3)(3,4)(4,1)(4,2)(4,3)
Pr(𝐴) = 1
36+ 1
36+ 1
36+ 1
36 = 1
9
Pr(𝐵) = 1
36+ 1
36+ 1
36+ 1
36 = 1
9
Pr(𝐶) = 12
36
Pr(𝐶 ∩ 𝐴) = 4
36
Pr(𝐴/𝐶) =
Pr (𝐴) Pr(𝐶 ∩ 𝐴)
Pr(𝐶)
=
1
9 × 1
9
12
36
= 1
27

Probability 4
3. Four-Door Monte Hall
With four doors, probability of;
Goat door opened Pr(𝐺) = 3
4
Car door opened Pr(𝐶) = 1
4
i is the door closed but marked
j is a door with goat
i. Probability of winning is Pr(𝐶) = Pr(𝑖) = 1
4
ii. Probability of winning given {j} is;
Pr(𝐶/{𝑖}) 𝑖𝑚𝑝𝑙𝑖𝑒𝑠 𝑑𝑜𝑜𝑟𝑠 𝑎𝑟𝑒 𝑛𝑜𝑤 3
Pr(𝐶) =1
3
iii. Probability of winning given 2 doors,
Pr (𝐶 /{𝑖, 𝑗}) =1
2
4. Estimating Genetic Diseases
Pr(𝐶) = 1
25, Pr(𝐶𝐶) = 1
4, Pr(𝐶′ ) = 24
25, Pr(𝐶𝐶′ ) = 3
4
Where, C – Carrier parent
CC – Carrier child
i. Probability two uniformly-chosen healthy people have child with CF

Probability 5
Pr(𝐶𝐹) = Pr(𝐶, 𝐶𝐶) 𝑎𝑛𝑑 Pr(𝐶, 𝐶𝐶)
= 1
25× 1
4 × 1
25× 1
4 = 1
1000
ii. Probability two randomly – chosen parents have a non – carrier child
= Pr(𝐶, 𝐶𝐶′ )𝑎𝑛𝑑 Pr(𝐶, 𝐶𝐶′ )
= 1
25× 3
4 × 1
25× 3
4 = 9
1000
iii. Probability a carrier parent and a randomly chosen parent have a CF child
for a carrier parent,Pr(𝐶𝐶) = 1
2
𝑓𝑜𝑟 𝑎 𝑟𝑎𝑛𝑑𝑜𝑚𝑙𝑦 𝑐ℎ𝑜𝑠𝑒𝑛 𝑝𝑎𝑟𝑒𝑛𝑡,Pr(𝐶) = 1
25 𝑎𝑛𝑑 Pr(𝐶𝐶) = 1
2
Pr(𝐶𝐹) = 𝑃𝑟(𝐶𝐶)𝑎𝑛𝑑𝑃𝑟(𝐶, 𝐶𝐶)
= 1
2 × 1
25× 1
2 = 1
100
iv. The probability that a carrier parent and randomly – chosen parent have a carrier
child
for a carrier parent,Pr(𝐶𝐶) = 1
2 , 𝑎𝑛𝑑 Pr(𝐶𝐶′) = 1
2
𝑓𝑜𝑟 𝑎 𝑟𝑎𝑛𝑑𝑜𝑚𝑙𝑦 𝑐ℎ𝑜𝑠𝑒𝑛 𝑝𝑎𝑟𝑒𝑛𝑡,
Pr(𝐶) = 1
25 𝑎𝑛𝑑 Pr(𝐶𝐶) = 1
2, 𝑎𝑛𝑑 Pr(𝐶𝐶′) = 1
2
= Pr(𝐶𝐶) 𝑎𝑛𝑑 Pr(𝐶𝐶′ ) 𝑜𝑟 Pr(𝐶𝐶′ ) 𝑎𝑛𝑑 Pr(𝐶𝐶)
= 1
100+ 1
100= 1
50

Probability 6
v. The probability baby has CF from two uniformly chosen healthy people
Pr (
𝐶𝐹
𝐶𝐶
) = Pr(𝐶𝐹) Pr(𝐶𝐹 ∩ 𝐶𝐶)
Pr (𝐶𝐶)
= Pr(𝐶𝐹)
= 1
25× 1
4 × 1
25× 1
4 = 1
1000
5. Sampling With and Without Replacement
Probability of a cider = 2
𝑛
With replacement
From the geometric mean, 𝐸(𝑋) = 1
𝑝
= 1
( 2
𝑛 )
= 𝑛
2
Without replacement
We have 2
𝑛
Then 𝑛−2
𝑛 × 2
𝑛−1, 𝑛−2
𝑛 × 𝑛−3
𝑛−1 × 2
𝑛−2…
𝐸(𝑋) = 2
𝑛( 1 + 2 (
𝑛 − 2
𝑛 ) + 3 (
𝑛 − 2
𝑛 )
2
+ ⋯ ) =
= 2
𝑛( 2(𝑛 + 1)
𝑛 )
= 𝑛 + 1
3

Probability 7
6. Finding a Healthy Subring
1. 𝐸(𝑋)
We have people = n, s = sick people, 𝑝𝑠 = 𝑝𝑟𝑜𝑏𝑎𝑏𝑖𝑙𝑖𝑡𝑦 𝑜𝑓 𝑠𝑖𝑐𝑘 𝑝𝑒𝑟𝑠𝑜𝑛
𝑝ℎ = 𝑝𝑟𝑜𝑏𝑎𝑏𝑖𝑙𝑖𝑡𝑦 𝑜𝑓 ℎ𝑒𝑎𝑙𝑡ℎ𝑦 𝑝𝑒𝑟𝑠𝑜𝑛 𝑠𝑢𝑐ℎ 𝑡ℎ𝑎𝑡 𝑝𝑠 + 𝑝ℎ = 1
𝑝𝑠 = 𝑠
𝑛
Since 𝑛 = 𝑘 < 100 𝑖𝑛𝑡𝑒𝑟𝑔𝑒𝑟𝑠and k=s,
𝑋~𝐵𝑖𝑛(𝑘, 𝑝𝑠)
𝑝𝑠 = 𝑠
𝑘
𝑡ℎ𝑢𝑠 𝐸(𝑋) = 𝑘𝑝𝑠
2.
If n=70, s=39
We have, Pr(𝑝𝑠) = 39
70 and Pr (𝑝ℎ) = 31
70
From a sample of n= 7, we expect Pr(𝑝𝑠) = 39
70 × 7 = 4 𝑡𝑜 𝑏𝑒 𝑠𝑖𝑐𝑘
𝑎𝑛𝑑 Pr(𝑝ℎ) = 31
70× 7 = 3 𝑡𝑜 𝑏𝑒 ℎ𝑒𝑎𝑙𝑡ℎ𝑦
However, since the sample is random, a consecutive sample 𝑝0, 𝑝1, … , 𝑝6can have a
probability Pr(𝑝𝑠) ≤ 3
7 𝑎𝑛𝑑 Pr(𝑝ℎ) ≥ 4
7 while most of the sick people will be on the