Probability Problem Set

The assignment content discusses various concepts related to probability, including independent and dependent events, experimental probability, and theoretical probability. It provides examples and scenarios to illustrate these concepts, such as rolling a die, selecting candies, and testing the effectiveness of a cure for a pathogen in mice.

Probability
Question 1
Given
Number of respondents that have traveled to Europe (a) = 68
Number of respondents that have not traveled to Europe (b) = 124
Total number of respondents (n) = a + b = 68 + 124 = 192
If one of these respondents is randomly selected, the probability of getting someone who has
traveled to Europe
¿ a
n
¿ 68
192
¿ 0.35
Question 2
Total number of the members of a golf club = 20
Number of members who earn at least $100,000 = 12
The probability that a randomly selected member earns at least $100,000
¿ Number of memberswho earn at least $ 100,000
Total number of the members of a golf club
¿ 12
20
¿ 0.6

Question 3
(a)
Number of female students born in Orlando = 12
Total number of female students = 37
The probability that a female student was born in Orlando
¿ Number of female students born∈Orlando
Totalnumber of female students
¿ 12
37
¿ 0.32
(b)
Number of male students born in Miami = 7
Total number of male students = 22
The probability that a male student was born in Miami
¿ Number of male students born∈ Miami
Totalnumber of male students
¿ 7
22
¿ 0.32
(c)
Number of students born in Jacksonville = 26
Total number of students = 59
The probability that a student was born in Jacksonville
¿ Number of students born∈Jacksonville
Total number of students
¿ 26
59
¿ 0.44

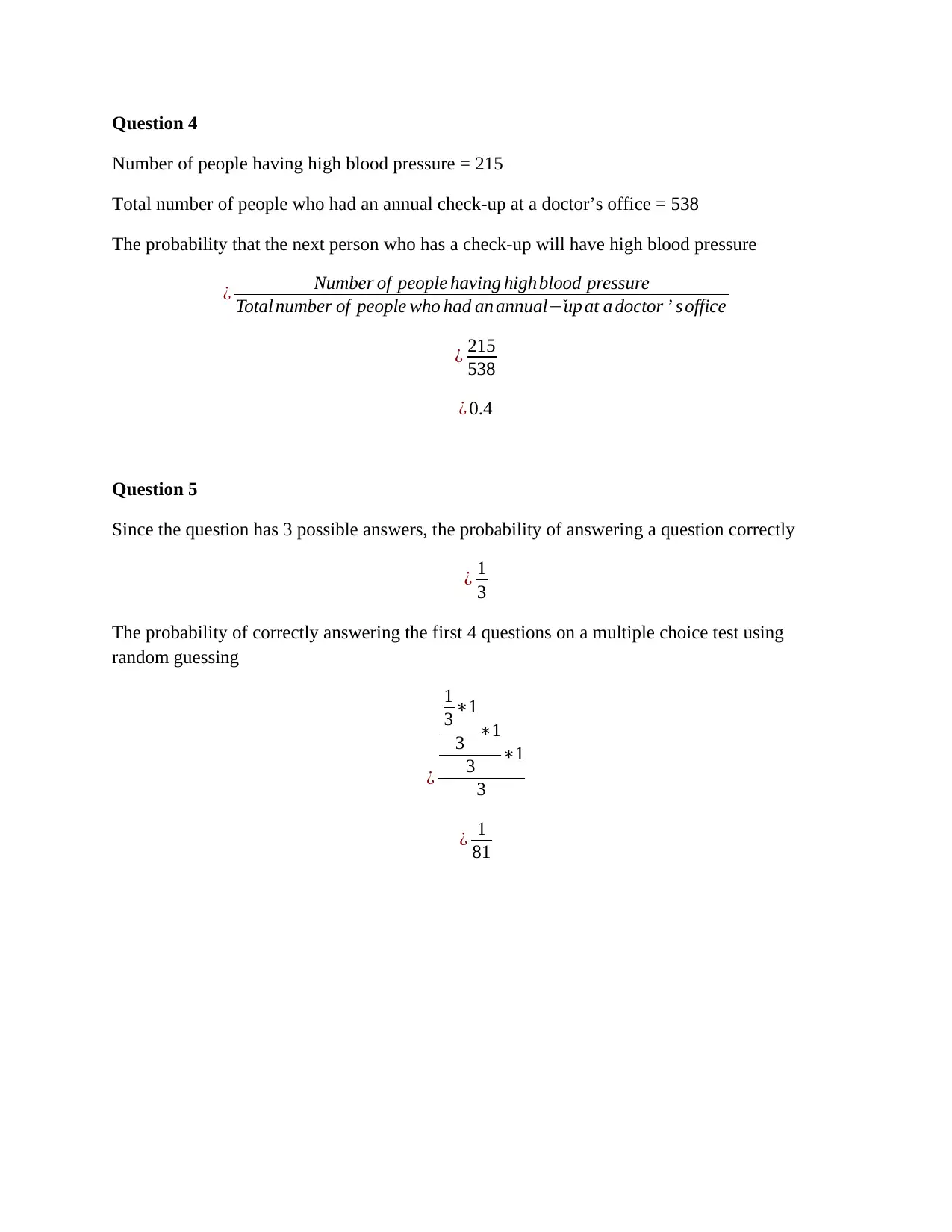
Question 4
Number of people having high blood pressure = 215
Total number of people who had an annual check-up at a doctor’s office = 538
The probability that the next person who has a check-up will have high blood pressure
¿ Number of people having highblood pressure
Total number of people who had an annual ˇ−up at a doctor ’ s office
¿ 215
538
¿ 0.4
Question 5
Since the question has 3 possible answers, the probability of answering a question correctly
¿ 1
3
The probability of correctly answering the first 4 questions on a multiple choice test using
random guessing
¿
1
3 ∗1
3 ∗1
3 ∗1
3
¿ 1
81

Question 6
The differences between independent and dependent events are:
1. Two events are independent if the outcome of one has no effect on the outcome of the
other whereas they are dependent if the outcome of one has an effect on the outcome of
the other.
2. Probabilities for independent events often involve exponents, while probabilities for
dependent events often involve factorials.
3. Example:
Independent event:
Event A: Rolling a number larger than four on a die in the first roll Event B: Rolling a 5 on
a die on the second roll. These events are independent because one roll of a dice
doesn't influence the outcome of another dice roll.
Dependent event:
Event A: Rolling a number larger than four on a die Event B: Rolling a 5 on a die on the
same roll. These events are dependent because, as we are in the same dice roll, the
occurrence of event B changes the probability of event A, actually it makes it 1.
4. Mathematically,
For Independent events:
P ( A ∪B ) =P ( A )+P ( B )−P ( A ) P ( B )
P ( A ∩B )=P ( A )∗P ( B )
P ( A∨B )=P ( A )
P ( A∨¬ B )=P ( A )
For Dependent events:
P ( A ∪B ) =P ( A ) + P ( B )
P ( A ∩B )=0
P ( A∨B )=0
P ( A∨¬ B )= P ( A )
1−P ( B )

Question 7
Experimental probability is the probability that an event occurred in the duration of an
experiment. It is calculated by dividing the number of event occurrences by the number of times
the trial was conducted.
For example, to find out how effective a given cure for a pathogen in mice is, we simply take a
number of mice with the pathogen and inject our cure.
We then find out how many mice were cured and this would give us the experimental probability
that a mouse is cured to be the ratio of number of mice cured to the total number of mice tested.
In this case, it is not possible to calculate the theoretical probability. We can then extend this
experimental probability to all mice.
Let the number of mice with pathogen be 1000 and the number of mice that have been cured be
579, then the experimental probability will be 579/1000 = 0.579.
It should be noted that in order for experimental probability to be meaningful in research, the
sample size must be sufficiently large.
In our above example, if we test our cure on 3 mice and all of these are cured, then the
experimental probability that a mouse is cured is 1. However, the sample size is too small to
conclude that the cure works in 100% of the cases.

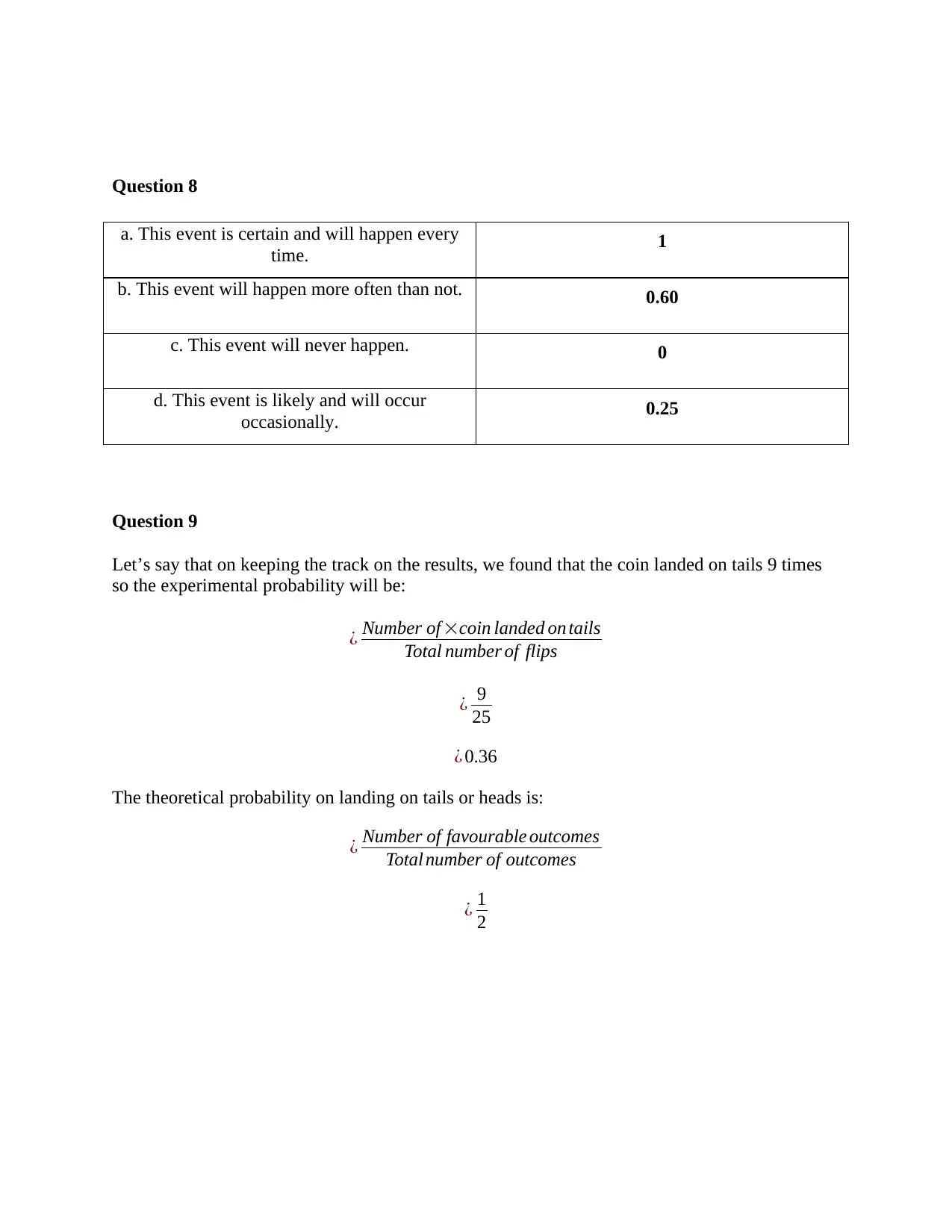
Question 8
a. This event is certain and will happen every
time. 1
b. This event will happen more often than not. 0.60
c. This event will never happen. 0
d. This event is likely and will occur
occasionally. 0.25
Question 9
Let’s say that on keeping the track on the results, we found that the coin landed on tails 9 times
so the experimental probability will be:
¿ Number of ×coin landed on tails
Total number of flips
¿ 9
25
¿ 0.36
The theoretical probability on landing on tails or heads is:
¿ Number of favourable outcomes
Total number of outcomes
¿ 1
2

Question 10
(a)
The probability of choosing a yellow candy = 0.20
(b)
The probability that the candy is blue, red, or green
¿ P ( blue ) + P ( red ) +P ( green )
¿ 0.30+0.10+ 0.15
¿ 0.55
(c)
Since the sum of the probabilities of all events in an experiment is always one.
Therefore, the probability of choosing an orange candy
¿ 1− ( P ( blue ) + P ( red ) + P ( green ) + P ( yellow ) )
¿ 1− ( 0.30+ 0.10+0.15+0.20 )
¿ 1−0.75
¿ 0.25