Mathematics Resit Coursework - Section A, Questions 1-3
VerifiedAdded on 2022/11/29
|4
|598
|500
Homework Assignment
AI Summary
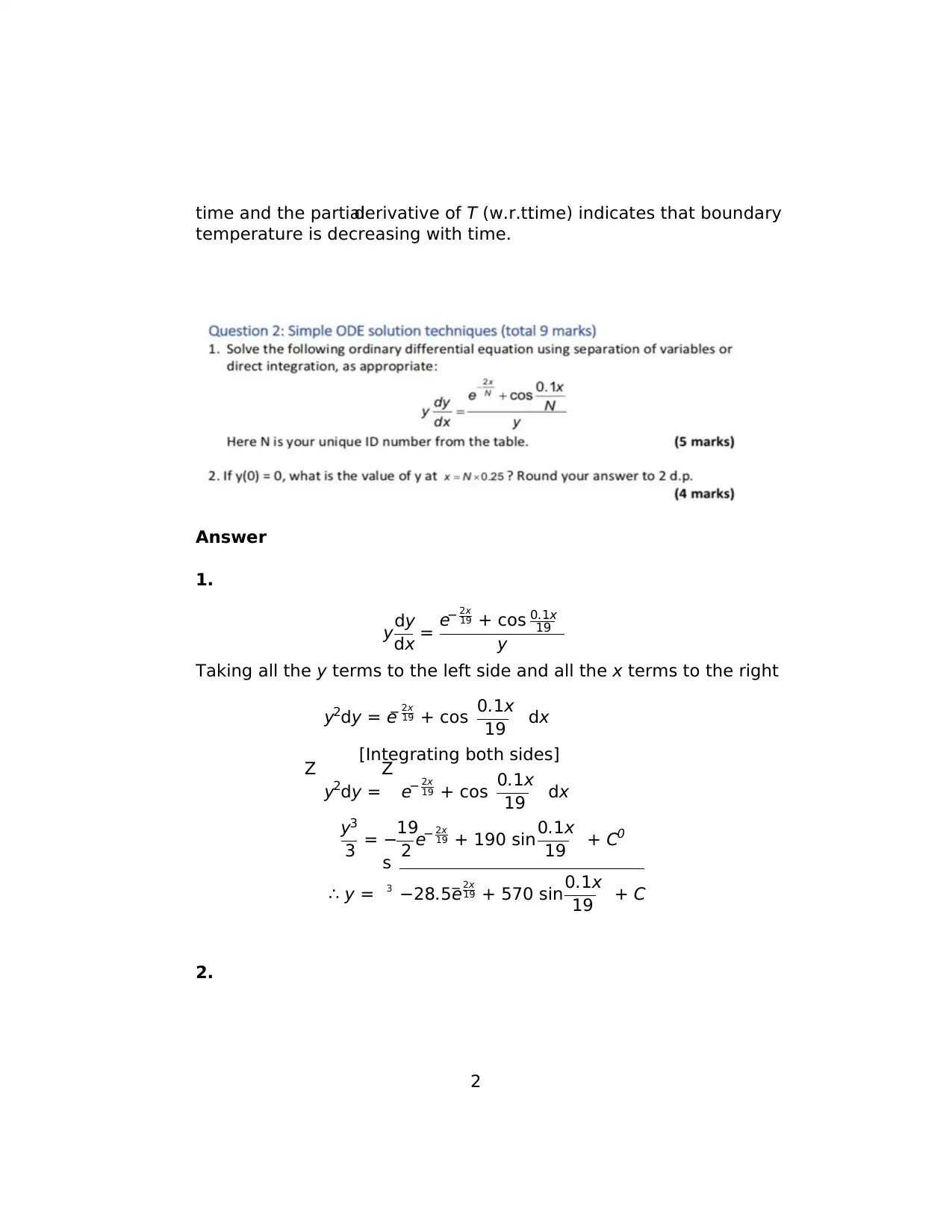
This document presents the solutions to the mathematics section (Section A) of a resit coursework. The solutions cover three questions, focusing on partial derivatives and differential equations. Question 1 involves finding the partial derivative of a given function with respect to time and evaluating it at a specific point, along with an interpretation of the result. Question 2 requires solving a differential equation using integration and finding a particular solution given initial conditions. Question 3 involves solving a linear differential equation using an integrating factor, and then determining the particular solution based on provided initial conditions. All steps and calculations are shown in detail, providing a comprehensive understanding of the solution process. This coursework is designed to assess the student's understanding of calculus and differential equations.
1 out of 4










![[object Object]](/_next/static/media/star-bottom.7253800d.svg)