Quantitative Methods in International Business

This article covers various quantitative methods used in international business, including hypothesis testing, normal distribution, sampling distribution, and more.

Running Head: QUANTITATIVE METHODS IN INTERNATIONAL BUSINESS
Quantitative Methods in International Business
Name of the Student
Name of the University
Author Note

1QUANTITATIVE METHODS IN INTERNATIONAL BUSINESS
Table of Contents
Table 1: Answer Matrix.............................................................................................................2
Answer 1....................................................................................................................................2
Answer 2....................................................................................................................................2
Answer 3....................................................................................................................................3
Answer 4....................................................................................................................................3
Answer 5....................................................................................................................................3
Answer 6....................................................................................................................................3
Answer 7....................................................................................................................................3
Answer 8....................................................................................................................................3
Answer 9....................................................................................................................................3
Answer 10..................................................................................................................................4
Answer 11..................................................................................................................................4
Answer 12..................................................................................................................................4
Answer 13..................................................................................................................................5
Answer 14..................................................................................................................................5
Answer 15..................................................................................................................................5

2QUANTITATIVE METHODS IN INTERNATIONAL BUSINESS
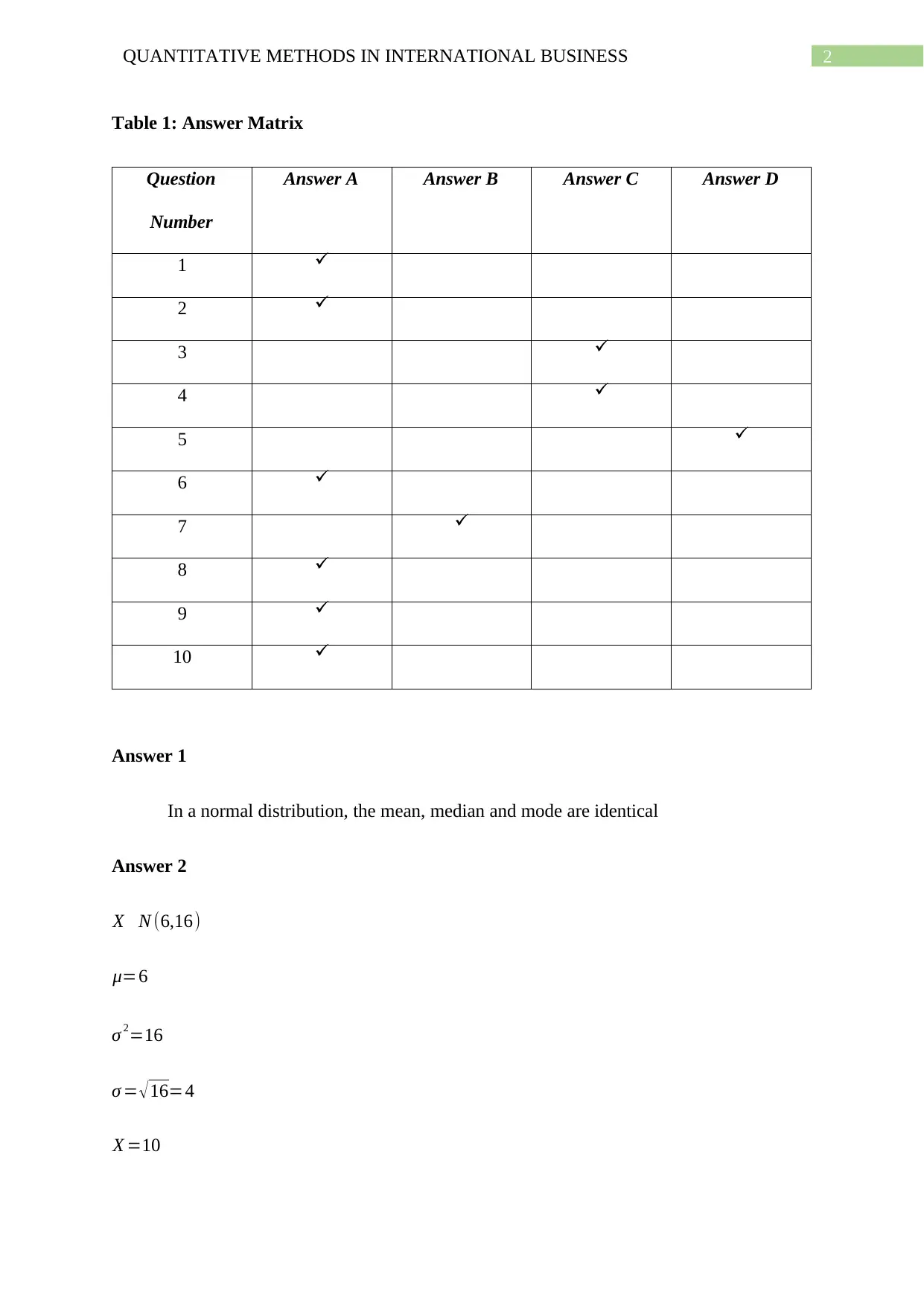
Table 1: Answer Matrix
Question
Number
Answer A Answer B Answer C Answer D
1 
2 
3 
4 
5 
6 
7 
8 
9 
10 
Answer 1
In a normal distribution, the mean, median and mode are identical
Answer 2
X N (6,16)
μ=6
σ 2=16
σ = √16=4
X =10

3QUANTITATIVE METHODS IN INTERNATIONAL BUSINESS
Z= X−μ
σ = 10−6
4 =1
Answer 3
A and B are two mutually exclusive events. This indicates that the two events are disjoint.
Therefore, P (A and B) = 0.
Answer 4
A histogram is not an ordinary bar graph because the horizontal axis of a histogram is
a continuous scale.
Answer 5
The standard deviation of the sampling distribution of the sample mean is (d) the
standard error
Answer 6
When testing hypothesis, if the p-value is smaller than the chosen significance level (
α), we then reject the null hypothesis (H0).
Answer 7
Type 1 error is committed when we reject a null hypothesis when it is true
Answer 8
Among the five steps needed for hypothesis testing are (a) State the hypothesis.
Answer 9
The sampling distribution of the mean of a small sample with sample size (n) < 30 is
approximated as a (a) Normal Distribution

4QUANTITATIVE METHODS IN INTERNATIONAL BUSINESS
Answer 10
If A and B are two Independent events, then,
P (A ∪ B) = P (A) + P(B)
Answer 11
A hypothesis which we set up and proceed to test with the help of sample
observations is called a null hypothesis.
For example, consider a company. It has to be tested whether there is a gender
discrimination in the company in terms of salary. To run this analysis, data has to be collected
on the male and the female salaries of the company. To conduct the test, at first the
hypothesis will have to be framed. In this case, the null hypothesis framed will be there exists
no difference in the male and the female salaries. Based on this this hypothesis, the analysis
will be conducted.
Answer 12
The error committed in rejecting the null hypothesis, when actually it is true is known
as type I error.
The error committed in accepting the null hypothesis, when actually it is false is
known as type II error.
The upper bound of the probability of type I error of a test is called the level of
significance of the test. In case of a simple hypothesis, the level of significance and the
probability of type I error are same. In most practical cases, as the level of significance, 0.01
or 0.05 is taken.

5QUANTITATIVE METHODS IN INTERNATIONAL BUSINESS
Let us consider the example where a criminal who have actually committed a crime
have not been put to jail or have not been punished. Thus, the criminal is roaming around free
and can commit more crimes. This is risky for other people around.
Answer 13
The company CoCo S.A. is concerned towards the time taken to react to the
complaints registered by the customers. Thus, a new set of procedures have been introduced
by the company for the staffs of the support centres. It has to be tested whether the average
time for responding to the complaints made by the customers is more than 28 days.
Only one sample is involved for this analysis. Thus, a one sample z-test will be used
to analyse this data. The distribution of the population is not known and the sample size is 42
which is greater than 30. Thus, the sample can be considered as a large sample and according
to the central limit theorem, this distribution can be assumed to be a normal distribution. The
null hypothesis for this test have been defined as the average time for responding to the
complaints made by the customers is less than equal to 28 days. The alternate hypothesis of
the test has been considered as the average time for responding to the complaints made by the
customers is more 28 days. Thus, the test conducted here will be an upper one tailed test. The
test conducted is a one tailed test at 5 percent level of significance. From the results of the
analysis, it can be seen that the p-value is 0.003 which is less than the level of significance
(0.05). Thus, the null hypothesis is rejected. Hence, it can be said that the average time for
responding to the complaints made by the customers is more than 28 days.
Answer 14
The concern of the University of Greenwich was about the implementation of a new
passport scheme among the undergraduate students of the university. A sample of 150
students were selected from all the undergraduate students of the Business School by the

6QUANTITATIVE METHODS IN INTERNATIONAL BUSINESS
passport team. Their total time spent on the passport activities during their second term of
year two has been recorded.
It can be seen clearly from the histogram and the frequency table that most of the
students have spent 31 – 35 hours doing the passport activities. 47 out of 150 students have
spent 31 – 35 hours doing passport activities. A minimum of 10 hours have been spent doing
the passport activities by the students. It has been observed from the value of the first quartile
(Q1) that 25 percent of the students spend less than 25 hours doing the passport activities.
The median value indicates that 50 percent of the students have spent more than 30 hours
doing the passport activities and from the value of the third quartile (Q3) that 25 percent of
the students have spent more than 34 hours doing the passport activities. The maximum time
spent doing passport activities by any undergraduate university student is 39 hours.
From the histogram, it can be seen clearly that the time spent doing passport activities
is negatively skewed. This indicates that time spent doing passport activities is mostly higher
than the average time of 29.24 hours. The mean is less than the median which is again less
than the mode. This also indicates negatively skewed. Again, the value of skewness can also
be seen to be negative. The value of kurtosis has been found to be positive. Thus, the time
spent doing passport activities is leptokurtic and have lesser tails and higher peaks.
Answer 15
The effectiveness of two TV advertisements has to be compared. For that reason, a
random sample of potential customers have been selected and their reactions have been
recorded on a scale of 1 to 25, where 1 is the most negative reaction and 25 is the most
positive reaction. It has to be tested whether the average reaction given by the customers on
both the type of advertisements are same. The reaction of 23 customers have been recorded

7QUANTITATIVE METHODS IN INTERNATIONAL BUSINESS
for advertisement type 1 and the reaction of 20 potential customers have been recorded for
advertisement type 2.
Here the difference of the means of two different samples have to be tested. In this
case there is no information about the population variance. Thus, independent sample t-test
has to be conducted. The test is conducted for the equality of means for the two groups
against the un-equality of the two means. Hence, the null hypothesis and the alternate
hypothesis is defined as:
H0: The average reaction score towards both the advertisements are equal
H1: The average reaction score towards both the advertisements are not equal
Thus, the test conducted is a two tailed test at 0.05 level of significance. From the results of
the t-test, it can be seen that the p-value is 0.31 which is greater than the level of significance
(0.05). Thus, the null hypothesis will be accepted. Thus, it can be said that there is no
difference in reaction of the customers on the two different types of advertisements.