Quantitative Methods: Solutions to Statistical Problems

This document provides solutions to statistical problems in Quantitative Methods, including calculating probabilities, confidence intervals, and population proportions. It also includes a summary of descriptive statistics and their interpretation. The subject, course code, course name, and college/university are not mentioned.

Quantitative methods
Quantitative methods
Student name:
Tutor name:
1 | P a g e

Quantitative methods
QUESTION ONE
Population mean = 150
Standard deviation = 21
Sample size, n = 49
a. p( x <147)
Z= x −μ
σ
Z=147−150
21
Z=−3
21 =−0.14
Z=−0.14
The probability corresponding to the Z-value is 44.43%
Thus p ( x<147 ) =44.43 %
b. p ( 152.5< x<157.5 )
p(x >152.5)
Z= x −μ
σ
Z=152.5−150
21
Z=2.5
21 =0.12
Z=0.12
The probability corresponding to the Z-value is 54.78%
p(x <157.5)
Z=157.5−150
21
2 | P a g e

Quantitative methods
Z=7.5
21 =0.36
Z=0.36
The probability corresponding to the Z-value is 64.06%
Therefore p ( 152.5<x< 157.5 ) =64.06 %−54.78 %=9.28 %
c.
p ( 148< x<158 )
p( x >148)
Z= x −μ
σ
Z=148−150
21
Z=−2.0
21 =−0.095
Z=−0.095
The probability corresponding to the Z-value is 46.41%
p( x <158)
Z=158−150
21
Z= 8
21 =0.38
Z=0.38
The probability corresponding to the Z-value is 64.8%
Therefore p ( 152.5<x< 157.5 ) =64.8 %−46.41%=18.39 %
3 | P a g e

Quantitative methods
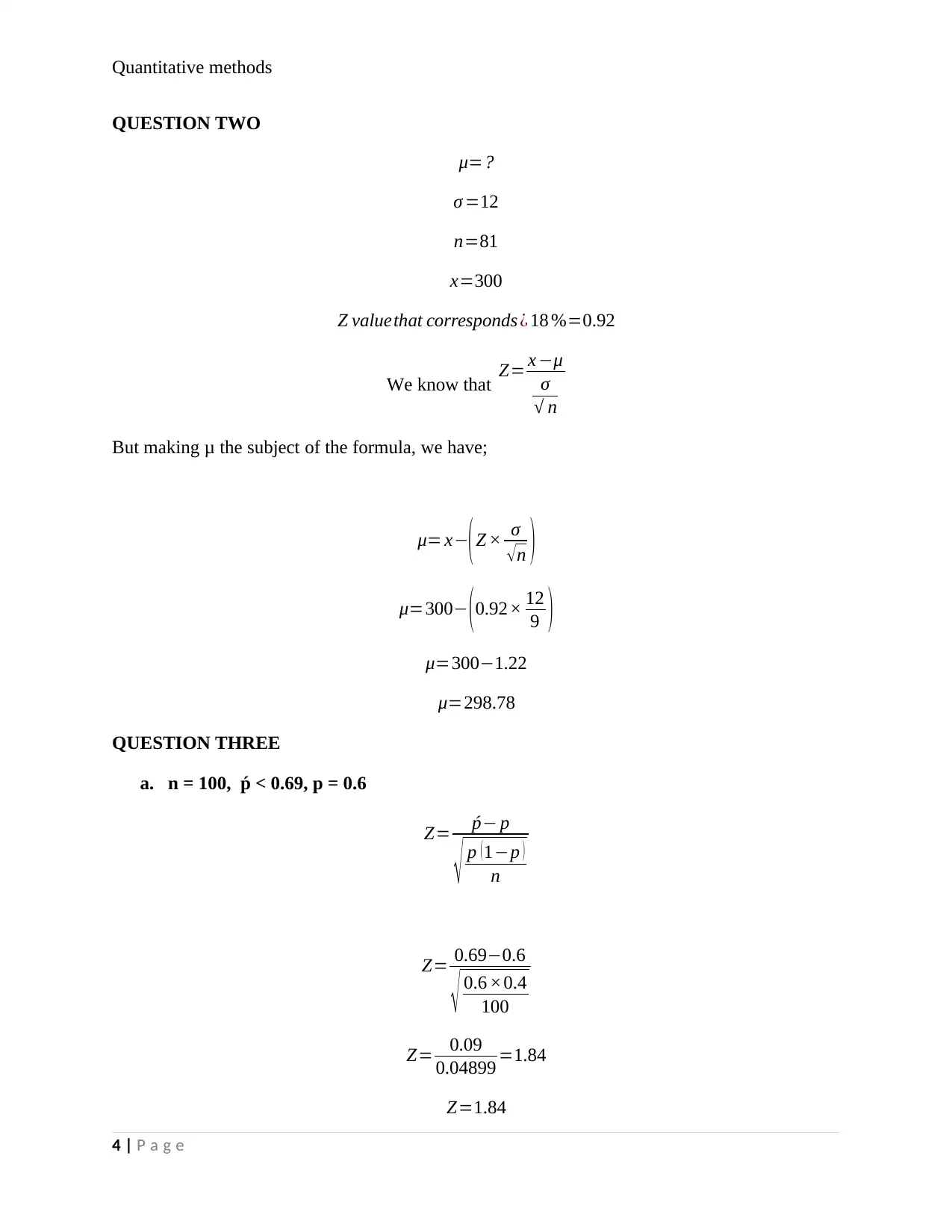
QUESTION TWO
μ=?
σ =12
n=81
x=300
Z valuethat corresponds ¿ 18 %=0.92
We know that Z= x −μ
σ
√ n
But making μ the subject of the formula, we have;
μ= x−
( Z × σ
√ n )
μ=300− (0.92× 12
9 )
μ=300−1.22
μ=298.78
QUESTION THREE
a. n = 100, ṕ < 0.69, p = 0.6
Z= ṕ− p
√ p ( 1−p )
n
Z= 0.69−0.6
√ 0.6 ×0.4
100
Z= 0.09
0.04899 =1.84
Z=1.84
4 | P a g e

Quantitative methods
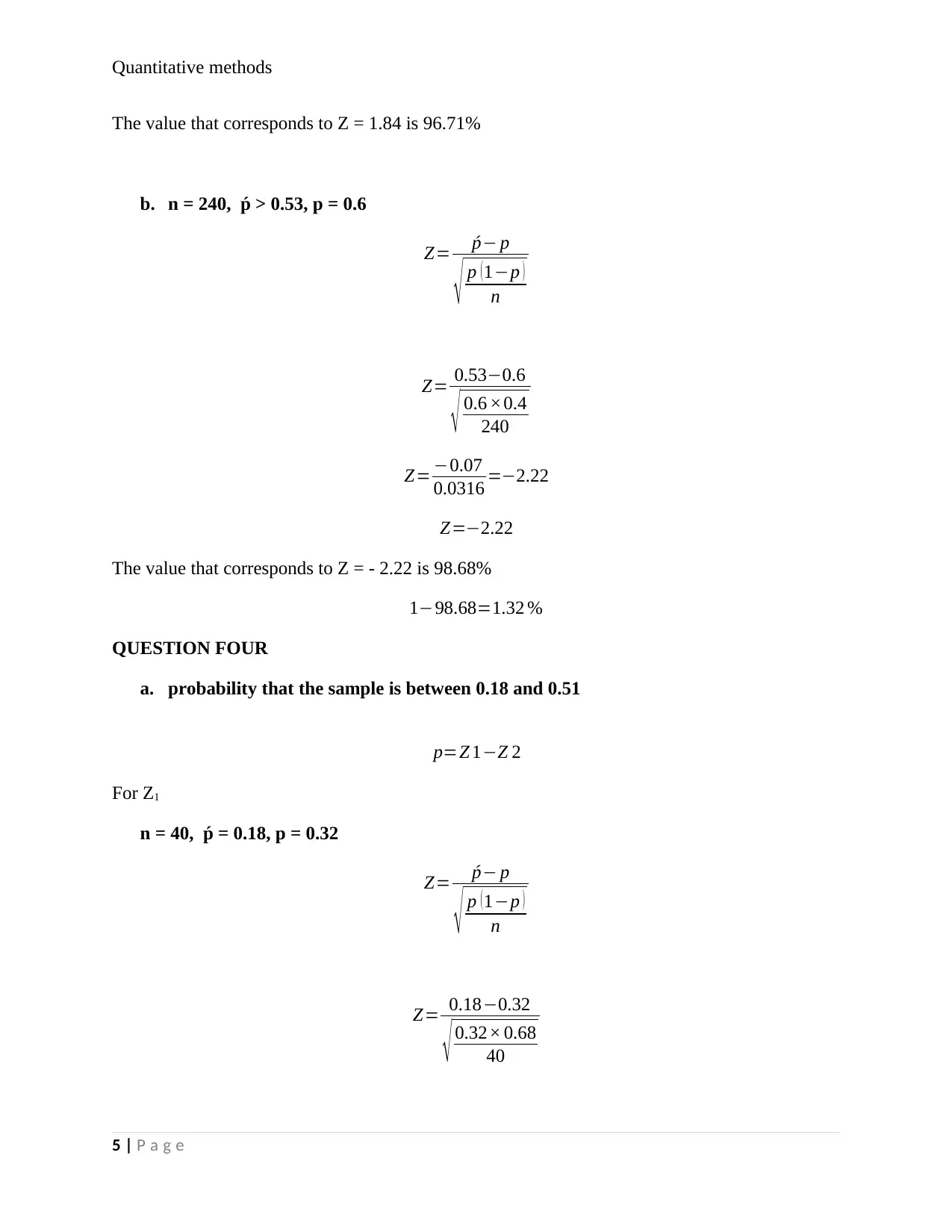
The value that corresponds to Z = 1.84 is 96.71%
b. n = 240, ṕ > 0.53, p = 0.6
Z= ṕ− p
√ p ( 1−p )
n
Z= 0.53−0.6
√ 0.6 ×0.4
240
Z= −0.07
0.0316 =−2.22
Z=−2.22
The value that corresponds to Z = - 2.22 is 98.68%
1−98.68=1.32 %
QUESTION FOUR
a. probability that the sample is between 0.18 and 0.51
p=Z 1−Z 2
For Z1
n = 40, ṕ = 0.18, p = 0.32
Z= ṕ− p
√ p ( 1−p )
n
Z= 0.18−0.32
√ 0.32× 0.68
40
5 | P a g e

Quantitative methods
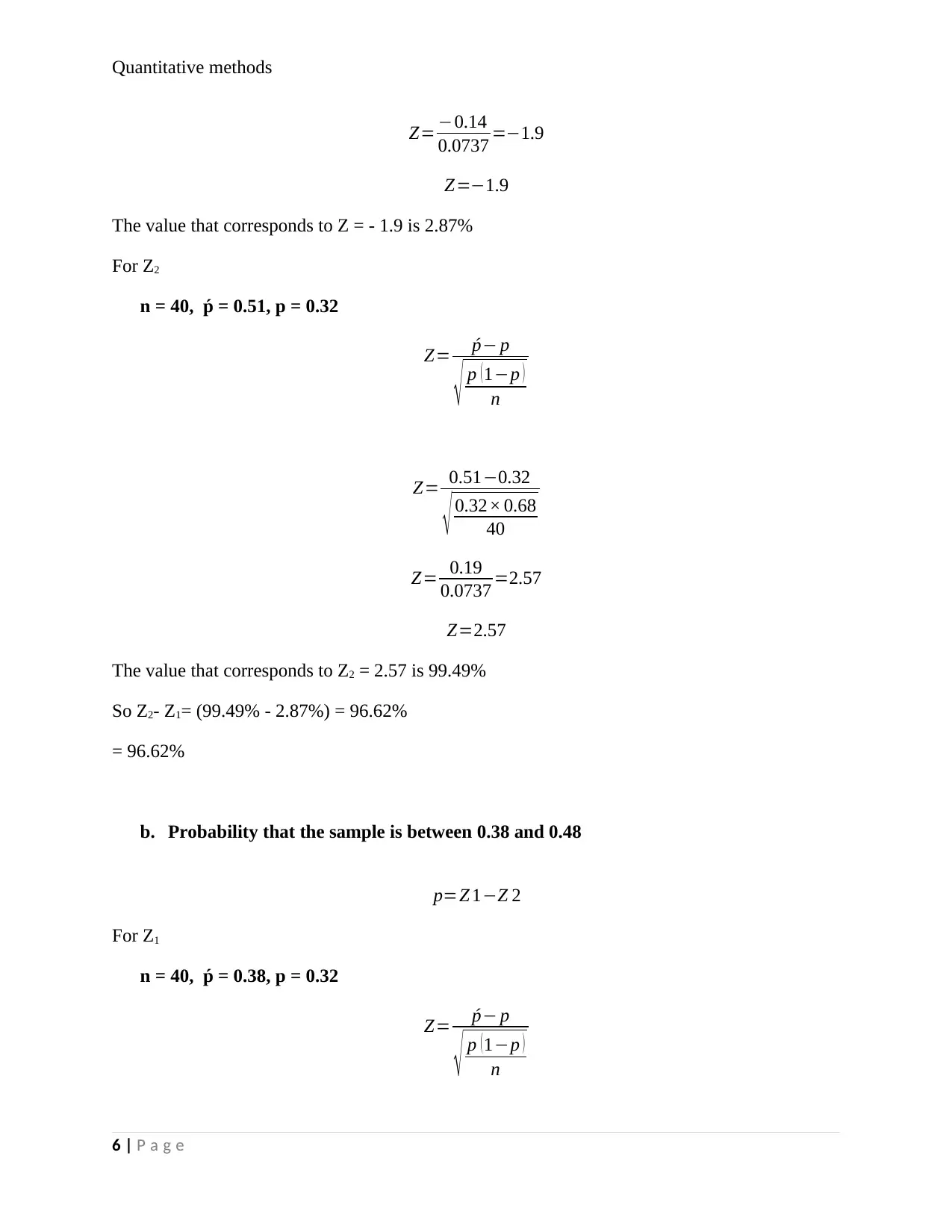
Z= −0.14
0.0737 =−1.9
Z=−1.9
The value that corresponds to Z = - 1.9 is 2.87%
For Z2
n = 40, ṕ = 0.51, p = 0.32
Z= ṕ− p
√ p ( 1−p )
n
Z= 0.51−0.32
√ 0.32× 0.68
40
Z= 0.19
0.0737 =2.57
Z=2.57
The value that corresponds to Z2 = 2.57 is 99.49%
So Z2- Z1= (99.49% - 2.87%) = 96.62%
= 96.62%
b. Probability that the sample is between 0.38 and 0.48
p=Z 1−Z 2
For Z1
n = 40, ṕ = 0.38, p = 0.32
Z= ṕ− p
√ p ( 1−p )
n
6 | P a g e

Quantitative methods
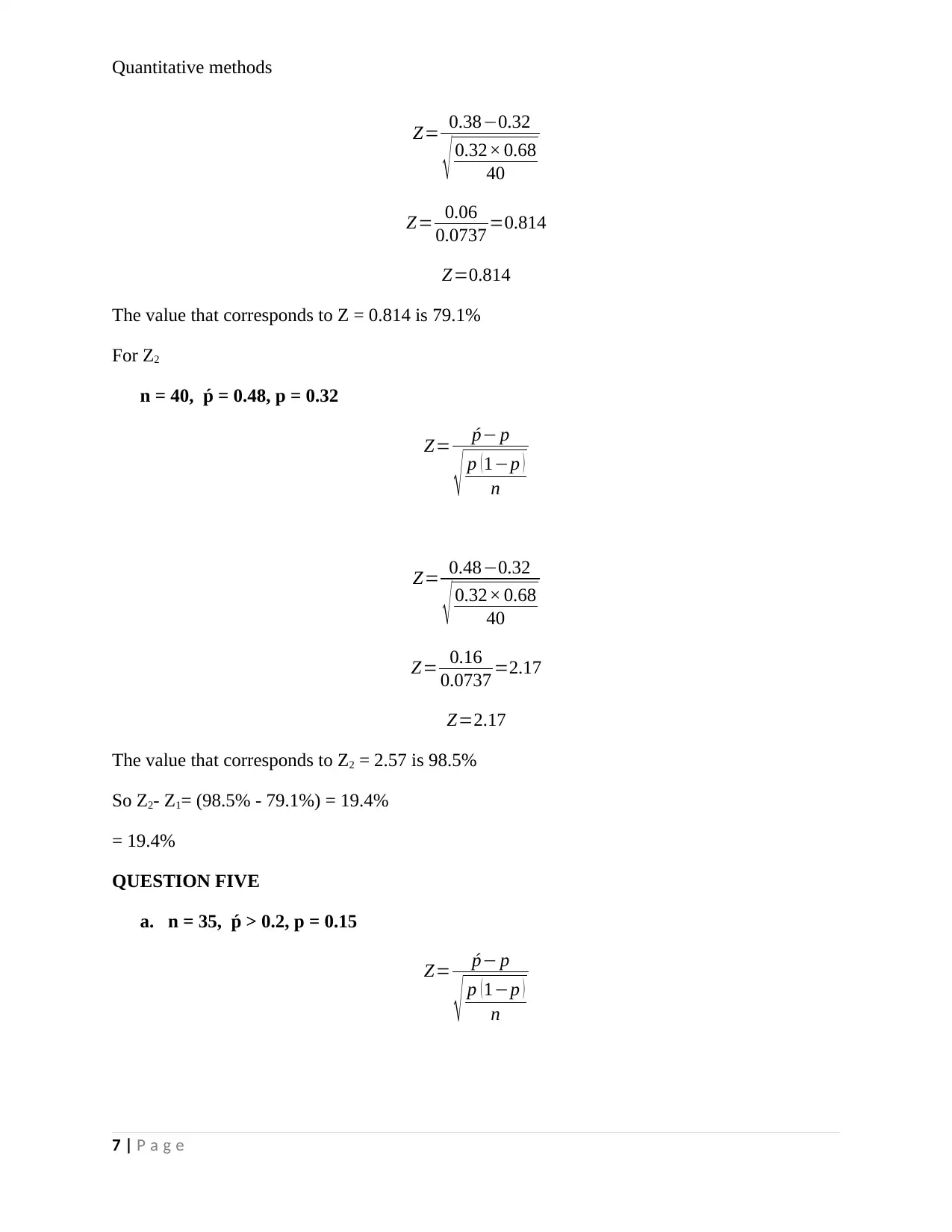
Z= 0.38−0.32
√ 0.32× 0.68
40
Z= 0.06
0.0737 =0.814
Z=0.814
The value that corresponds to Z = 0.814 is 79.1%
For Z2
n = 40, ṕ = 0.48, p = 0.32
Z= ṕ− p
√ p ( 1−p )
n
Z= 0.48−0.32
√ 0.32× 0.68
40
Z= 0.16
0.0737 =2.17
Z=2.17
The value that corresponds to Z2 = 2.57 is 98.5%
So Z2- Z1= (98.5% - 79.1%) = 19.4%
= 19.4%
QUESTION FIVE
a. n = 35, ṕ > 0.2, p = 0.15
Z= ṕ− p
√ p ( 1−p )
n
7 | P a g e

Quantitative methods
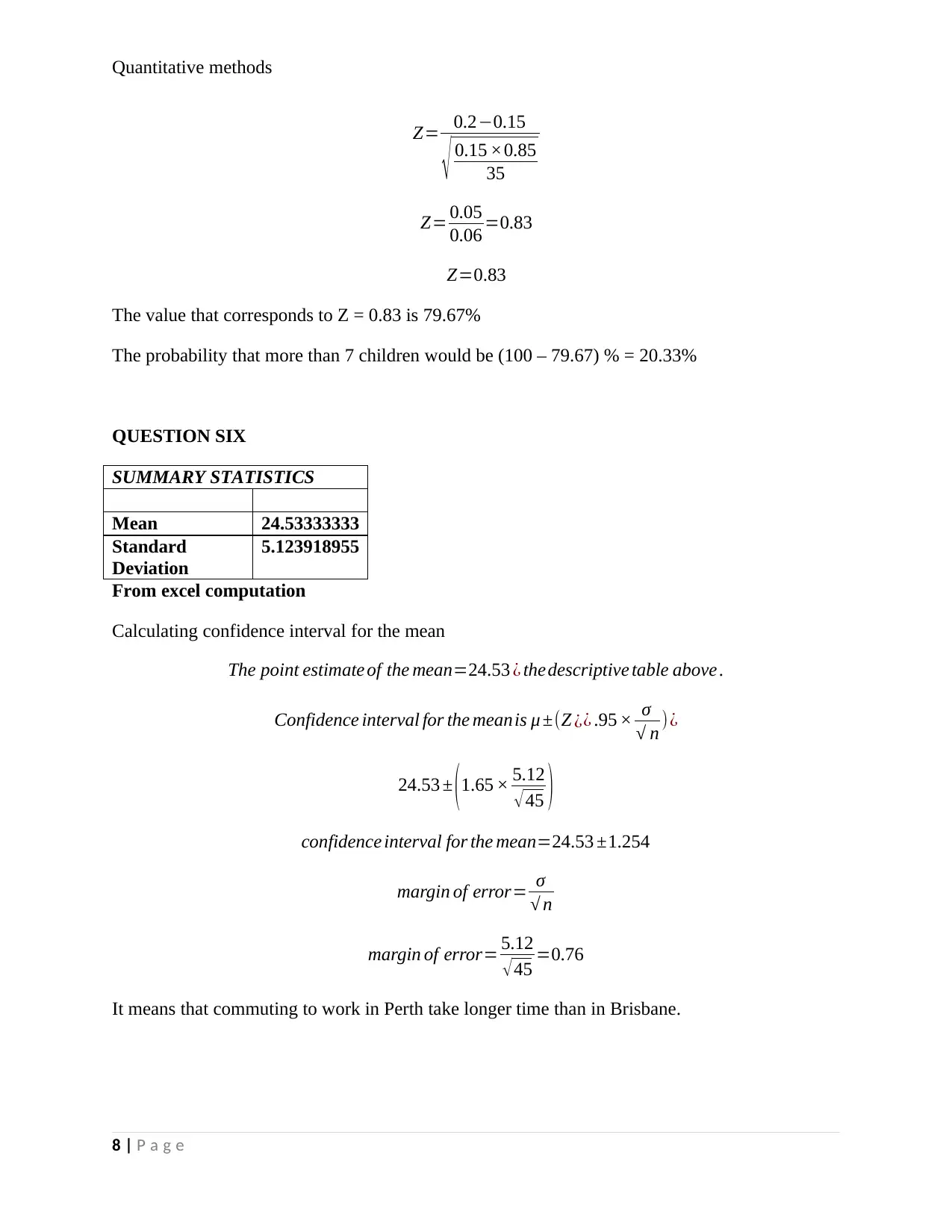
Z= 0.2−0.15
√ 0.15 ×0.85
35
Z= 0.05
0.06 =0.83
Z=0.83
The value that corresponds to Z = 0.83 is 79.67%
The probability that more than 7 children would be (100 – 79.67) % = 20.33%
QUESTION SIX
SUMMARY STATISTICS
Mean 24.53333333
Standard
Deviation
5.123918955
From excel computation
Calculating confidence interval for the mean
The point estimate of the mean=24.53 ¿ thedescriptive table above .
Confidence interval for the meanis μ ±(Z ¿¿ .95 × σ
√ n )¿
24.53 ± (1.65 × 5.12
√45 )
confidence interval for the mean=24.53 ±1.254
margin of error= σ
√ n
margin of error= 5.12
√45 =0.76
It means that commuting to work in Perth take longer time than in Brisbane.
8 | P a g e

Quantitative methods
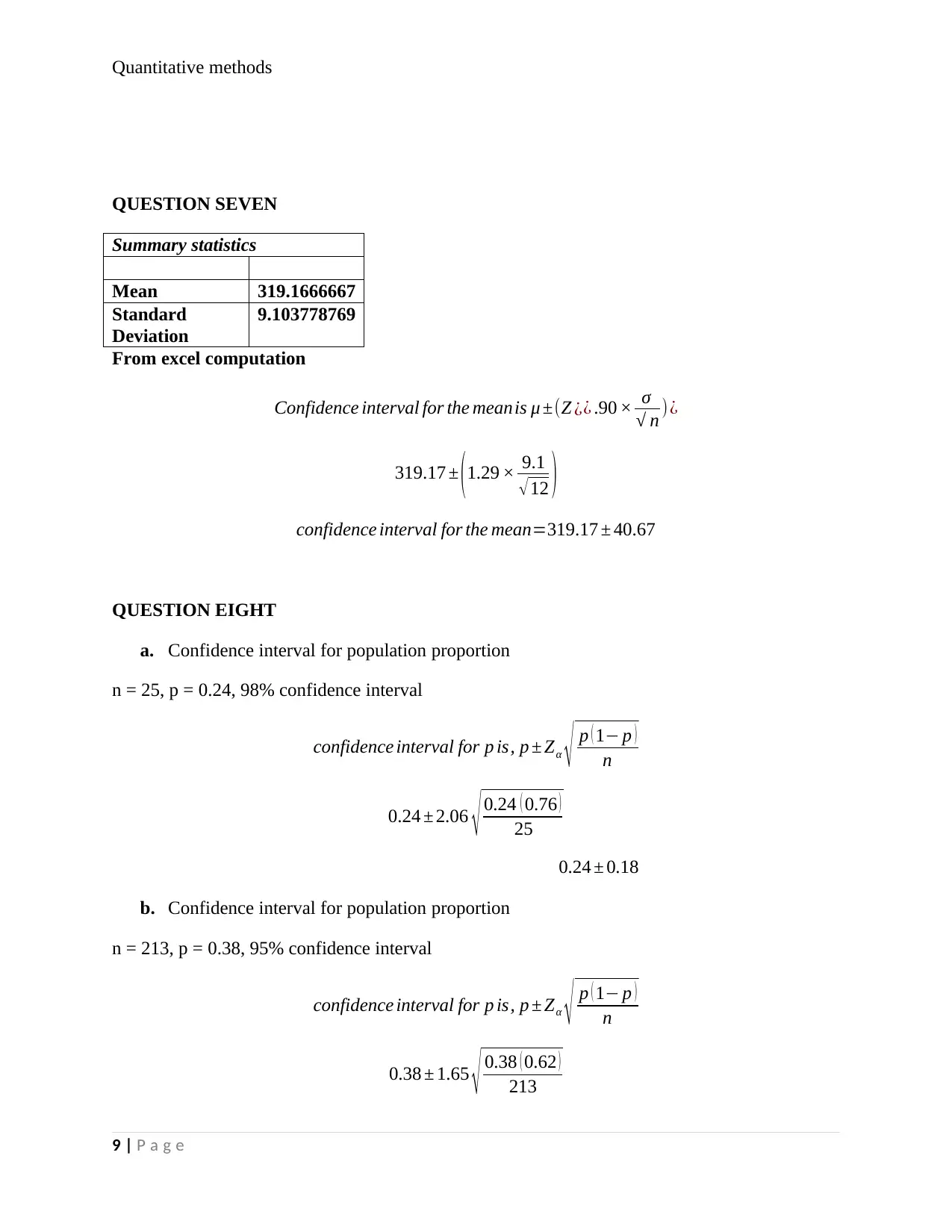
QUESTION SEVEN
Summary statistics
Mean 319.1666667
Standard
Deviation
9.103778769
From excel computation
Confidence interval for the meanis μ ±(Z ¿¿ .90 × σ
√ n )¿
319.17 ± (1.29 × 9.1
√12 )
confidence interval for the mean=319.17 ± 40.67
QUESTION EIGHT
a. Confidence interval for population proportion
n = 25, p = 0.24, 98% confidence interval
confidence interval for p is, p ± Zα √ p ( 1− p )
n
0.24 ± 2.06 √ 0.24 ( 0.76 )
25
0.24 ± 0.18
b. Confidence interval for population proportion
n = 213, p = 0.38, 95% confidence interval
confidence interval for p is, p ± Zα √ p ( 1− p )
n
0.38 ± 1.65 √ 0.38 ( 0.62 )
213
9 | P a g e

Quantitative methods
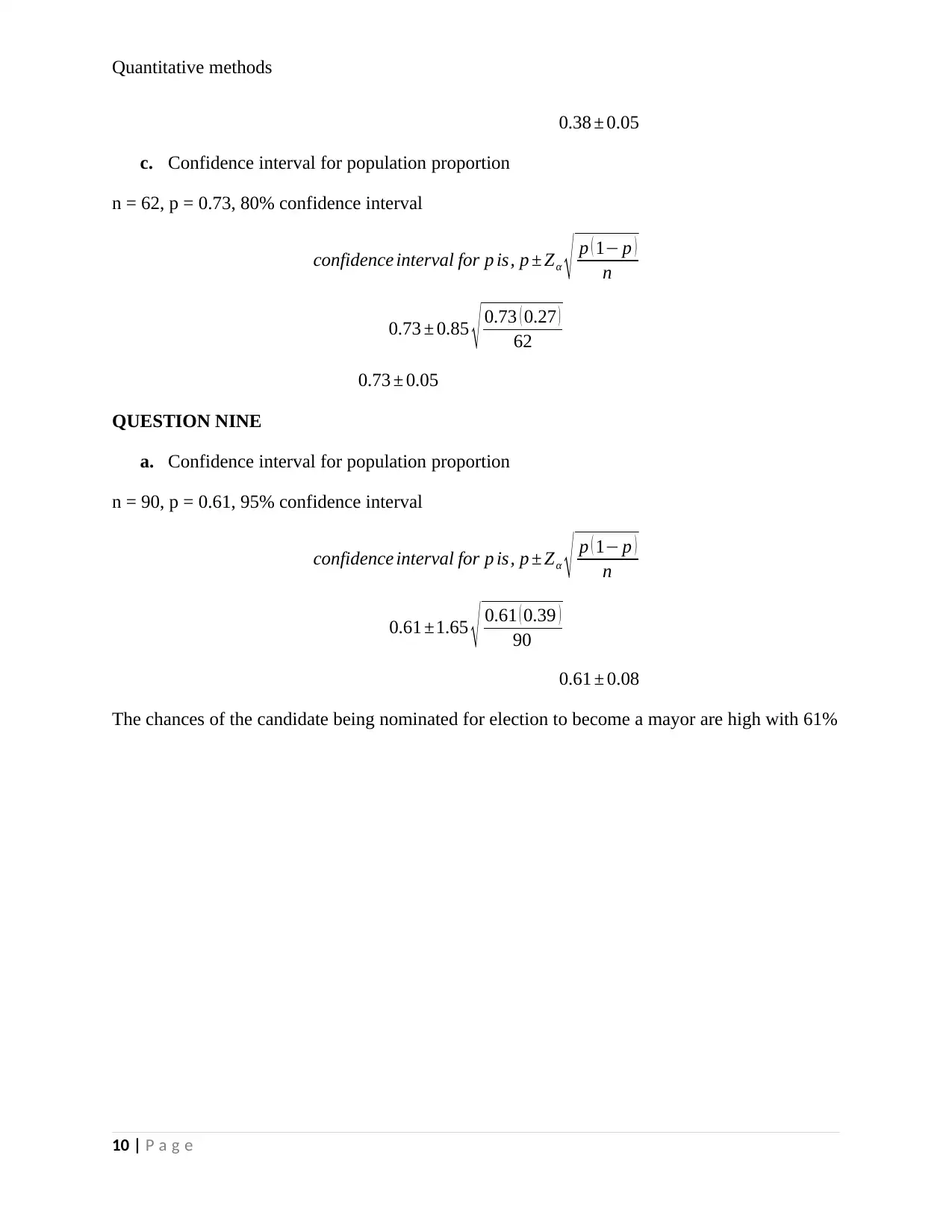
0.38 ± 0.05
c. Confidence interval for population proportion
n = 62, p = 0.73, 80% confidence interval
confidence interval for p is, p ± Zα √ p ( 1− p )
n
0.73 ± 0.85 √ 0.73 ( 0.27 )
62
0.73 ± 0.05
QUESTION NINE
a. Confidence interval for population proportion
n = 90, p = 0.61, 95% confidence interval
confidence interval for p is, p ± Zα √ p ( 1− p )
n
0.61 ±1.65 √ 0.61 ( 0.39 )
90
0.61 ± 0.08
The chances of the candidate being nominated for election to become a mayor are high with 61%
10 | P a g e