QUESTION AND ANSWER ON FINANCE
VerifiedAdded on 2022/08/29
|7
|1676
|14
AI Summary
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

FINANCE
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
Answer 1
The Invoice Price in the above exercise is $ 1000, followed by the accrued interest of $0. The
purchase price is $ 100.00 and the yield to maturity has been calculated to be 5%. The
purchase price is also referred to as the quoted price of the bonds that is the price on which
the bond can be bought from the market. In contrast to this, the invoice price is the sum of the
quoted price and the accrued interest on a bond. Thus, while the purchase price of the above
bond has been computed to be $100, the actual worth or the invoice price of the bond has
been $ 1000. The bond is stated to be at par.
Answer 2
The computation of the bond price using the formula is depicted as follows.
Computation of the price of the bond (Amount in $)
Yea
r Cash Flows PVF @ 5% Present Values
1 50 0.952 47.62
2 50 0.907 45.35
3 50 0.864 43.19
4 1050 0.823 863.84
SUM 1000
It can be noted that the price calculated on the Fidelity website and the price calculated as per
the theoretical formula of bond price calculation, is exactly the same. The price is exactly the
same that is $ 1000.
Answer 3
When the settlement date has been changed to tomorrow that is the April 4th 2020, the
changes are elaborated as follows. The Invoice Price has been changed to be $ 1000.14, and
the accrued interest has been changed to $ 0.14. The purchase price is still $ 100.00 and the
yield to maturity has been calculated to be 5%. Thus, while the purchase price and the yield
to maturity has been constant on the change of the settlement date of the above bond, the
invoice price of the bond and the yield to maturity has been changed just by one day shift in
the settlement date.
Answer 4
In this section, the yield to maturity rate has been changed to be 6 %. The result of the same
is elaborated as follows. The accrued interest is still $0 in this case. The Invoice price on the
The Invoice Price in the above exercise is $ 1000, followed by the accrued interest of $0. The
purchase price is $ 100.00 and the yield to maturity has been calculated to be 5%. The
purchase price is also referred to as the quoted price of the bonds that is the price on which
the bond can be bought from the market. In contrast to this, the invoice price is the sum of the
quoted price and the accrued interest on a bond. Thus, while the purchase price of the above
bond has been computed to be $100, the actual worth or the invoice price of the bond has
been $ 1000. The bond is stated to be at par.
Answer 2
The computation of the bond price using the formula is depicted as follows.
Computation of the price of the bond (Amount in $)
Yea
r Cash Flows PVF @ 5% Present Values
1 50 0.952 47.62
2 50 0.907 45.35
3 50 0.864 43.19
4 1050 0.823 863.84
SUM 1000
It can be noted that the price calculated on the Fidelity website and the price calculated as per
the theoretical formula of bond price calculation, is exactly the same. The price is exactly the
same that is $ 1000.
Answer 3
When the settlement date has been changed to tomorrow that is the April 4th 2020, the
changes are elaborated as follows. The Invoice Price has been changed to be $ 1000.14, and
the accrued interest has been changed to $ 0.14. The purchase price is still $ 100.00 and the
yield to maturity has been calculated to be 5%. Thus, while the purchase price and the yield
to maturity has been constant on the change of the settlement date of the above bond, the
invoice price of the bond and the yield to maturity has been changed just by one day shift in
the settlement date.
Answer 4
In this section, the yield to maturity rate has been changed to be 6 %. The result of the same
is elaborated as follows. The accrued interest is still $0 in this case. The Invoice price on the

change of the Yield to maturity has been computed to be $ 965.35. The purchase price as
calculated as per the fidelity calculator is $ 96.565. Thus, it is evident that the purchase price
of the bond has been dropped now, as the YTM has increased. The purchase price is less than
the par value of the bond, which means that the bond is now being traded at discount.
Answer 5
The results on changing the YTM to 4 % are listed as follows. The purchase price by
decreasing the yield to maturity has been computed out to be $ 103.63. The accrued interest
has been $ 0. It is to be noted here is that as the YTM drops, the price of the bond increases.
The price of the bond has increased from $ 100 of the initial bond to $ 103.63. Since, the
bond has crossed the par value, it can be stated that the bond is trading at premium. The
premium of the bond is $ 3.63.
Answer 6
On the comparison of the bond prices at the YTM of 6% and 4% respectively, it can be stated
that the prices of the bonds are not symmetrical around $ 1000. This is because, while the
increase in the YTM led to a fall of around $ 5, the decrease in the YTM led to the increase in
the prices by around $ 3. Thus, it can be stated that the increase and decrease of the bond
prices are not absolutely symmetrical.
Answer 7
As per the theoretical formula of the bond price calculation, the bond price has been
calculated as follows. The price is calculated at the semi annual YTM rate.
Computation of the price of the bond
Perio
d Cash Flows PVF @ 5% Present Values
1 25 0.976 24.39
2 25 0.952 23.80
3 25 0.929 23.21
4 25 0.906 22.65
5 25 0.884 22.10
6 25 0.862 21.56
7 25 0.841 21.03
8 1025 0.821 841.27
SUM 1020.52
calculated as per the fidelity calculator is $ 96.565. Thus, it is evident that the purchase price
of the bond has been dropped now, as the YTM has increased. The purchase price is less than
the par value of the bond, which means that the bond is now being traded at discount.
Answer 5
The results on changing the YTM to 4 % are listed as follows. The purchase price by
decreasing the yield to maturity has been computed out to be $ 103.63. The accrued interest
has been $ 0. It is to be noted here is that as the YTM drops, the price of the bond increases.
The price of the bond has increased from $ 100 of the initial bond to $ 103.63. Since, the
bond has crossed the par value, it can be stated that the bond is trading at premium. The
premium of the bond is $ 3.63.
Answer 6
On the comparison of the bond prices at the YTM of 6% and 4% respectively, it can be stated
that the prices of the bonds are not symmetrical around $ 1000. This is because, while the
increase in the YTM led to a fall of around $ 5, the decrease in the YTM led to the increase in
the prices by around $ 3. Thus, it can be stated that the increase and decrease of the bond
prices are not absolutely symmetrical.
Answer 7
As per the theoretical formula of the bond price calculation, the bond price has been
calculated as follows. The price is calculated at the semi annual YTM rate.
Computation of the price of the bond
Perio
d Cash Flows PVF @ 5% Present Values
1 25 0.976 24.39
2 25 0.952 23.80
3 25 0.929 23.21
4 25 0.906 22.65
5 25 0.884 22.10
6 25 0.862 21.56
7 25 0.841 21.03
8 1025 0.821 841.27
SUM 1020.52
It has been observed that the price computed using the theoretical formula in the case of the
semi annual payments of coupon is exactly equal to the price on the Fidelity website, that is $
1000 in both the cases.
Answer 8
It is to be noted that the prices of the bonds are inversely related to the market interest rates or
the yield to maturity. That means as the market interest rates or the yield to maturity
increases, the price of the bonds fall. The sensitivity of the price of the above bond with a
change in the interest rates on both sides, is depicted as follows.
Computation of the price of the bond ($)
Year Cash Flows PVF @ 6% Present Values
1 50 0.943 47.17
2 50 0.890 44.50
3 50 0.840 41.98 % change = 3.47%
4 1050 0.792 831.70 (price)
SUM 965.35
It must be noted that as the YTM increase from 5% to 6%, the price falls to $ 965, depicting a
percentage decrease of 3.47%. In contrast to this, as the YTM falls from 5% to 4%, the price
of the bond increases from $1000 to $1036. The percentage change in the price on such a
decrease is 3.63%. Hence, it can be stated that the price sensitivity of the bond to the YTM is
not “exactly equal.” There is a difference of 0.16% in the percentage changes. Further to note
that the reason for the inverse relationship is that while the bonds still paying the flat coupon
rate of 5% only, the market interest rate or a generalised value of another investment in the
market has been changing. As the market interest rate decreases, the proportional change in
price is more, than in the vice versa scenario. This depicts positive sentiments of the bond
holders.
Computation of the price of the bond ($)
Year Cash Flows PVF @ 4% Present Values
1 50 0.962 48.08
2 50 0.925 46.23
3 50 0.889 44.45
4 1050 0.855 897.54 % change = 3.63%
SUM 1036.30 (price)
semi annual payments of coupon is exactly equal to the price on the Fidelity website, that is $
1000 in both the cases.
Answer 8
It is to be noted that the prices of the bonds are inversely related to the market interest rates or
the yield to maturity. That means as the market interest rates or the yield to maturity
increases, the price of the bonds fall. The sensitivity of the price of the above bond with a
change in the interest rates on both sides, is depicted as follows.
Computation of the price of the bond ($)
Year Cash Flows PVF @ 6% Present Values
1 50 0.943 47.17
2 50 0.890 44.50
3 50 0.840 41.98 % change = 3.47%
4 1050 0.792 831.70 (price)
SUM 965.35
It must be noted that as the YTM increase from 5% to 6%, the price falls to $ 965, depicting a
percentage decrease of 3.47%. In contrast to this, as the YTM falls from 5% to 4%, the price
of the bond increases from $1000 to $1036. The percentage change in the price on such a
decrease is 3.63%. Hence, it can be stated that the price sensitivity of the bond to the YTM is
not “exactly equal.” There is a difference of 0.16% in the percentage changes. Further to note
that the reason for the inverse relationship is that while the bonds still paying the flat coupon
rate of 5% only, the market interest rate or a generalised value of another investment in the
market has been changing. As the market interest rate decreases, the proportional change in
price is more, than in the vice versa scenario. This depicts positive sentiments of the bond
holders.
Computation of the price of the bond ($)
Year Cash Flows PVF @ 4% Present Values
1 50 0.962 48.08
2 50 0.925 46.23
3 50 0.889 44.45
4 1050 0.855 897.54 % change = 3.63%
SUM 1036.30 (price)
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
Answer 9
The difference in the coupon amounts has significant impact interest rate sensitivity on the
bonds, as depicted from the following calculations.
When the coupon amounts are 35, the changes in the prices on the increase and decrease of
the YTM is as follows.
Computation of the price of the bond
Yea
r Cash Flows PVF @ 6% Present Values
1 35 0.943 33.02
2 35 0.890 31.15
3 35 0.840 29.39
4 1035 0.792 819.82
SUM 913.37
% change = 8.66%
(price)
Computation of the price of the bond
Yea
r Cash Flows PVF @ 4% Present Values
1 35 0.962 33.65
2 35 0.925 32.36
3 35 0.889 31.11
4 1035 0.855 884.72
SUM 981.85
% change = 1.81%
(price)
Thus, when the coupon amount is $35, and the YTM increases, the percentage change on
price is 8.66%. In contrast, when the YTM decreases, the percentage change is 1.81%.
Computation of the price of the bond
Yea
r Cash Flows PVF @ 6% Present Values
1 85 0.943 80.19
2 85 0.890 75.65
3 85 0.840 71.37
4 1085 0.792 859.42
SUM 1086.63
% change = 8.66%
The difference in the coupon amounts has significant impact interest rate sensitivity on the
bonds, as depicted from the following calculations.
When the coupon amounts are 35, the changes in the prices on the increase and decrease of
the YTM is as follows.
Computation of the price of the bond
Yea
r Cash Flows PVF @ 6% Present Values
1 35 0.943 33.02
2 35 0.890 31.15
3 35 0.840 29.39
4 1035 0.792 819.82
SUM 913.37
% change = 8.66%
(price)
Computation of the price of the bond
Yea
r Cash Flows PVF @ 4% Present Values
1 35 0.962 33.65
2 35 0.925 32.36
3 35 0.889 31.11
4 1035 0.855 884.72
SUM 981.85
% change = 1.81%
(price)
Thus, when the coupon amount is $35, and the YTM increases, the percentage change on
price is 8.66%. In contrast, when the YTM decreases, the percentage change is 1.81%.
Computation of the price of the bond
Yea
r Cash Flows PVF @ 6% Present Values
1 85 0.943 80.19
2 85 0.890 75.65
3 85 0.840 71.37
4 1085 0.792 859.42
SUM 1086.63
% change = 8.66%
(price)
Computation of the price of the bond
Yea
r Cash Flows PVF @ 4% Present Values
1 85 0.962 81.73
2 85 0.925 78.59
3 85 0.889 75.56
4 1085 0.855 927.46
SUM 1163.35
% change = 16.33%
(price)
When the coupon amount is $85, and the YTM increases, the percentage change on price is
still 8.66%. In contrast, when the YTM decreases, the percentage change is 16.33%.
It is clearly evident that on the fall of the YTM, the percentage change in the price of the
bonds with higher coupon rate is much more than that in the lower coupon amount.
Answer 10
As per the analysis conducted, the interest rate sensitivity to the time of maturity has been
depicted as follows.
Computation of the price of the bond
Period Cash Flows PVF @ 4% Present Values
1 25 0.980 24.51
2 25 0.961 24.03
3 25 0.942 23.56
4 25 0.924 23.10
5 25 0.906 22.64
6 25 0.888 22.20
7 25 0.871 21.76
8 1025 0.853 874.83
SUM 1036.63
% change = 3.66%
(price)
Computation of the price of the bond
Yea
r Cash Flows PVF @ 4% Present Values
1 85 0.962 81.73
2 85 0.925 78.59
3 85 0.889 75.56
4 1085 0.855 927.46
SUM 1163.35
% change = 16.33%
(price)
When the coupon amount is $85, and the YTM increases, the percentage change on price is
still 8.66%. In contrast, when the YTM decreases, the percentage change is 16.33%.
It is clearly evident that on the fall of the YTM, the percentage change in the price of the
bonds with higher coupon rate is much more than that in the lower coupon amount.
Answer 10
As per the analysis conducted, the interest rate sensitivity to the time of maturity has been
depicted as follows.
Computation of the price of the bond
Period Cash Flows PVF @ 4% Present Values
1 25 0.980 24.51
2 25 0.961 24.03
3 25 0.942 23.56
4 25 0.924 23.10
5 25 0.906 22.64
6 25 0.888 22.20
7 25 0.871 21.76
8 1025 0.853 874.83
SUM 1036.63
% change = 3.66%
(price)
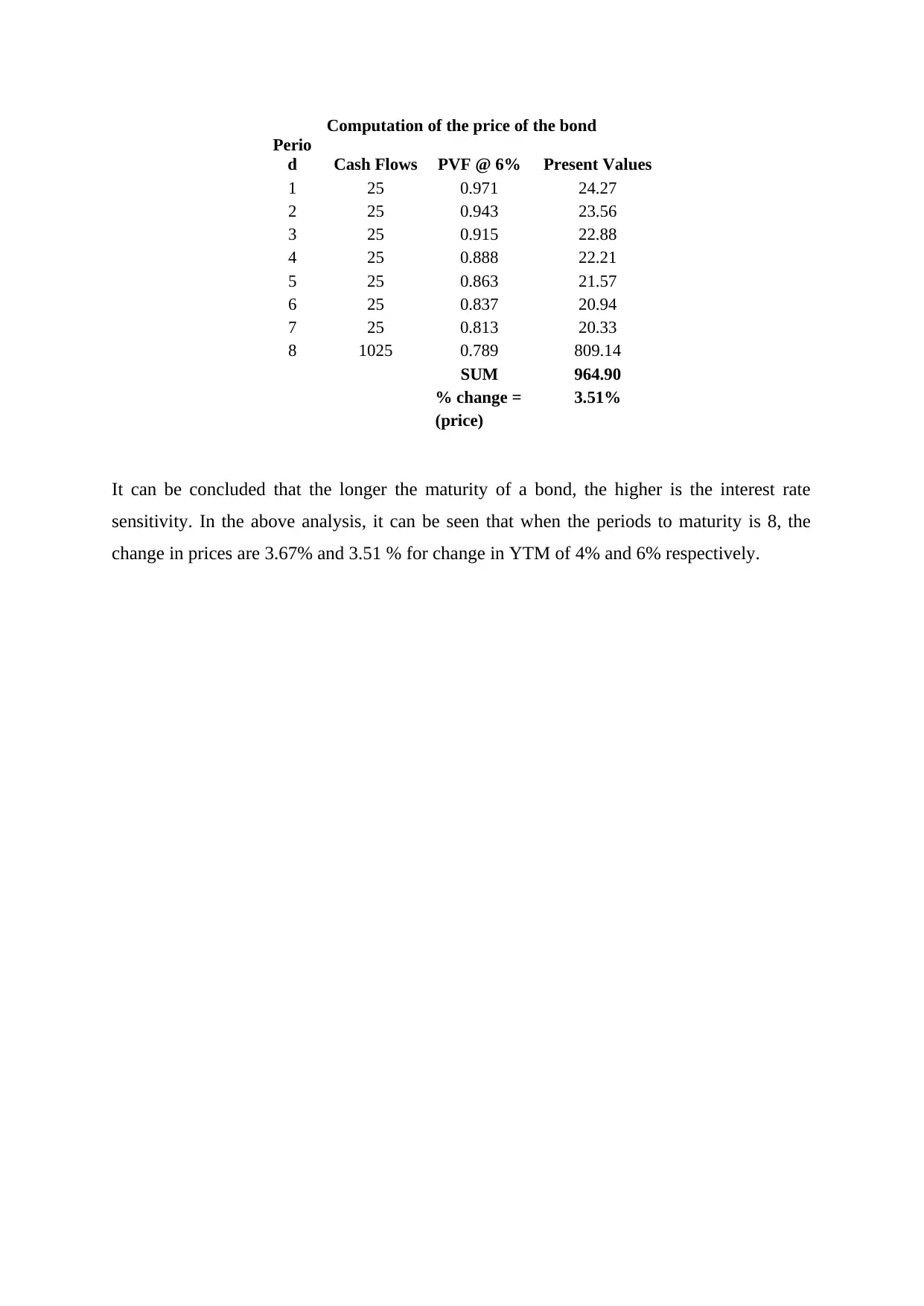
Computation of the price of the bond
Perio
d Cash Flows PVF @ 6% Present Values
1 25 0.971 24.27
2 25 0.943 23.56
3 25 0.915 22.88
4 25 0.888 22.21
5 25 0.863 21.57
6 25 0.837 20.94
7 25 0.813 20.33
8 1025 0.789 809.14
SUM 964.90
% change = 3.51%
(price)
It can be concluded that the longer the maturity of a bond, the higher is the interest rate
sensitivity. In the above analysis, it can be seen that when the periods to maturity is 8, the
change in prices are 3.67% and 3.51 % for change in YTM of 4% and 6% respectively.
Perio
d Cash Flows PVF @ 6% Present Values
1 25 0.971 24.27
2 25 0.943 23.56
3 25 0.915 22.88
4 25 0.888 22.21
5 25 0.863 21.57
6 25 0.837 20.94
7 25 0.813 20.33
8 1025 0.789 809.14
SUM 964.90
% change = 3.51%
(price)
It can be concluded that the longer the maturity of a bond, the higher is the interest rate
sensitivity. In the above analysis, it can be seen that when the periods to maturity is 8, the
change in prices are 3.67% and 3.51 % for change in YTM of 4% and 6% respectively.
1 out of 7
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.





