Statistical Proofs in Simple Linear Regression Analysis Model

This assignment provides detailed proofs for several key relationships in regression analysis. It demonstrates that the sum of the product of deviations from the mean for x and y can be expressed as the sum of x_i multiplied by the deviation of y_i from its mean. It also proves that the sum of observed ...Read More

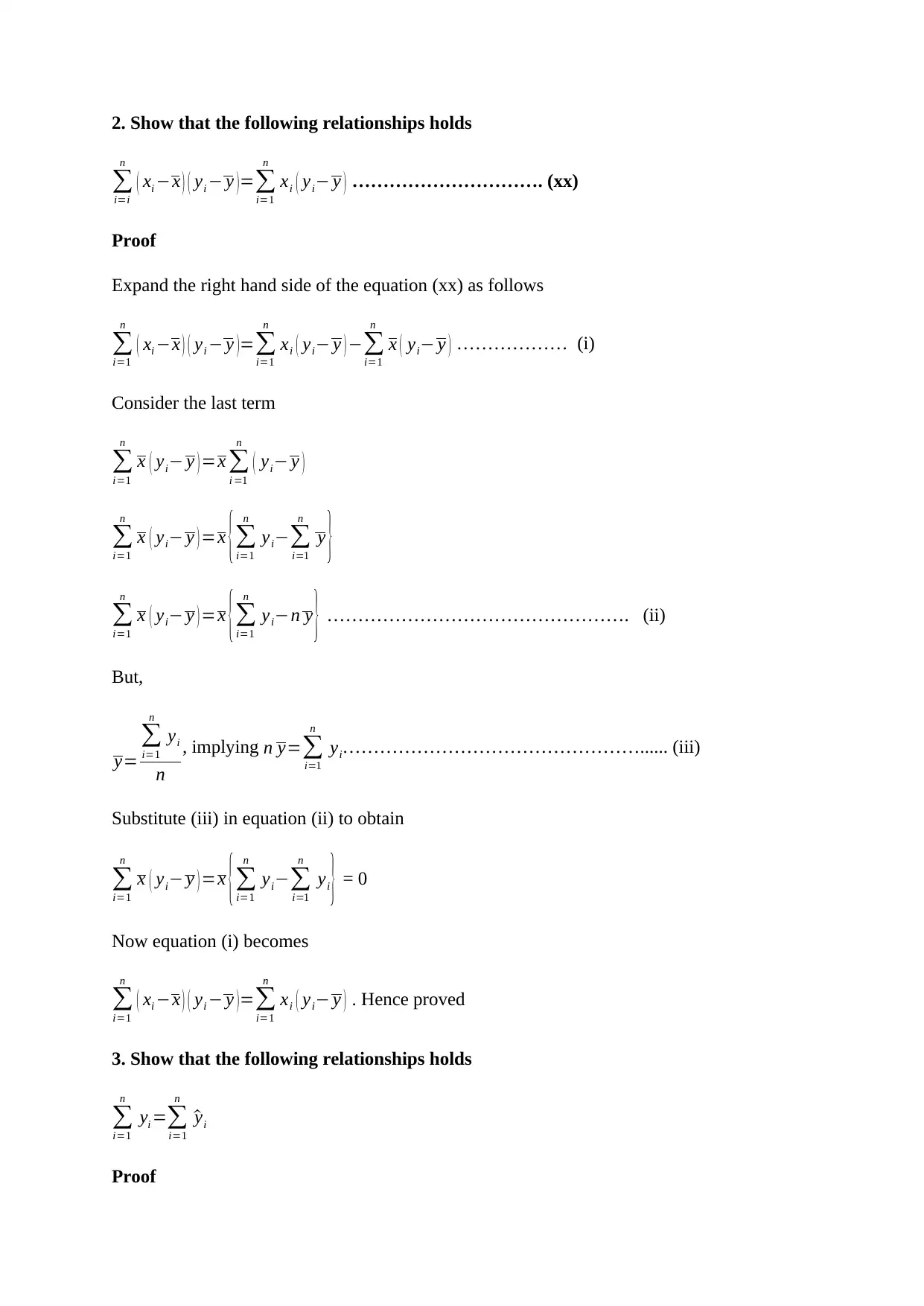
2. Show that the following relationships holds
∑
i=i
n
( xi −x ) ( yi − y )=∑
i=1
n
xi ( yi− y ) …………………………. (xx)
Proof
Expand the right hand side of the equation (xx) as follows
∑
i=1
n
( xi −x ) ( yi − y )=∑
i=1
n
xi ( yi− y ) −∑
i=1
n
x ( yi− y ) ……………… (i)
Consider the last term
∑
i=1
n
x ( yi− y ) =x ∑
i =1
n
( yi− y )
∑
i=1
n
x ( yi− y ) =x {∑
i=1
n
yi−∑
i=1
n
y }
∑
i=1
n
x ( yi− y ) =x {∑
i=1
n
yi−n y } …………………………………………. (ii)
But,
y=
∑
i=1
n
yi
n
, implying n y=∑
i=1
n
yi…………………………………………...... (iii)
Substitute (iii) in equation (ii) to obtain
∑
i=1
n
x ( yi− y ) =x {∑
i=1
n
yi−∑
i=1
n
yi } = 0
Now equation (i) becomes
∑
i=1
n
( xi −x ) ( yi − y )=∑
i=1
n
xi ( yi− y ) . Hence proved
3. Show that the following relationships holds
∑
i=1
n
yi =∑
i=1
n
^yi
Proof

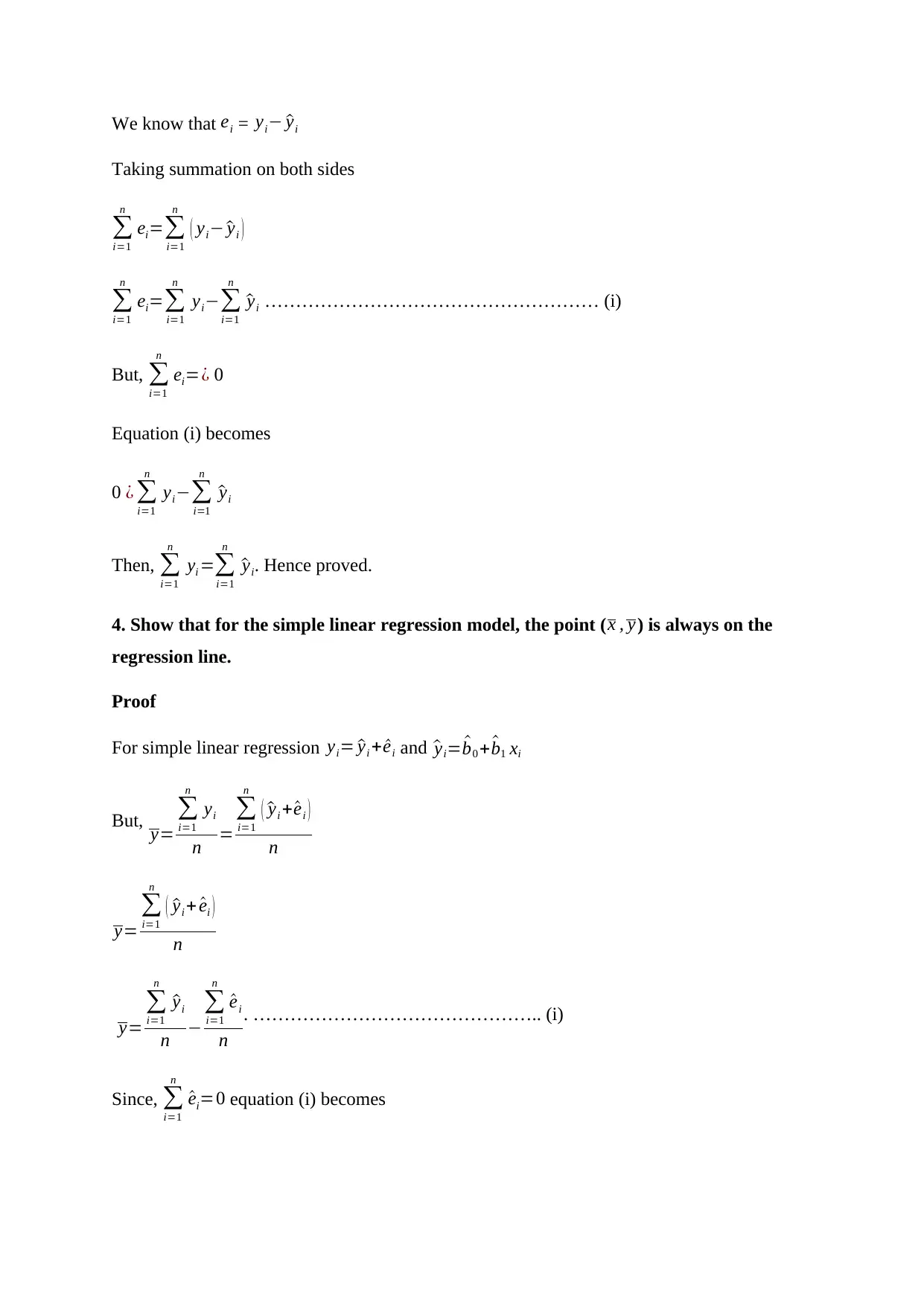
We know that ei = yi− ^yi
Taking summation on both sides
∑
i=1
n
ei=∑
i=1
n
( yi− ^yi )
∑
i=1
n
ei=∑
i=1
n
yi−∑
i=1
n
^yi ……………………………………………… (i)
But, ∑
i=1
n
ei=¿ 0
Equation (i) becomes
0 ¿ ∑
i=1
n
yi−∑
i=1
n
^yi
Then, ∑
i=1
n
yi =∑
i=1
n
^yi. Hence proved.
4. Show that for the simple linear regression model, the point ( x , y) is always on the
regression line.
Proof
For simple linear regression yi= ^yi + ^ei and ^yi= ^b0 + ^b1 xi
But, y=
∑
i=1
n
yi
n =
∑
i=1
n
( ^yi + ^ei )
n
y=
∑
i=1
n
( ^yi + ^ei )
n
y=
∑
i=1
n
^yi
n −
∑
i=1
n
^ei
n
. ……………………………………….. (i)
Since, ∑
i=1
n
^ei=0 equation (i) becomes

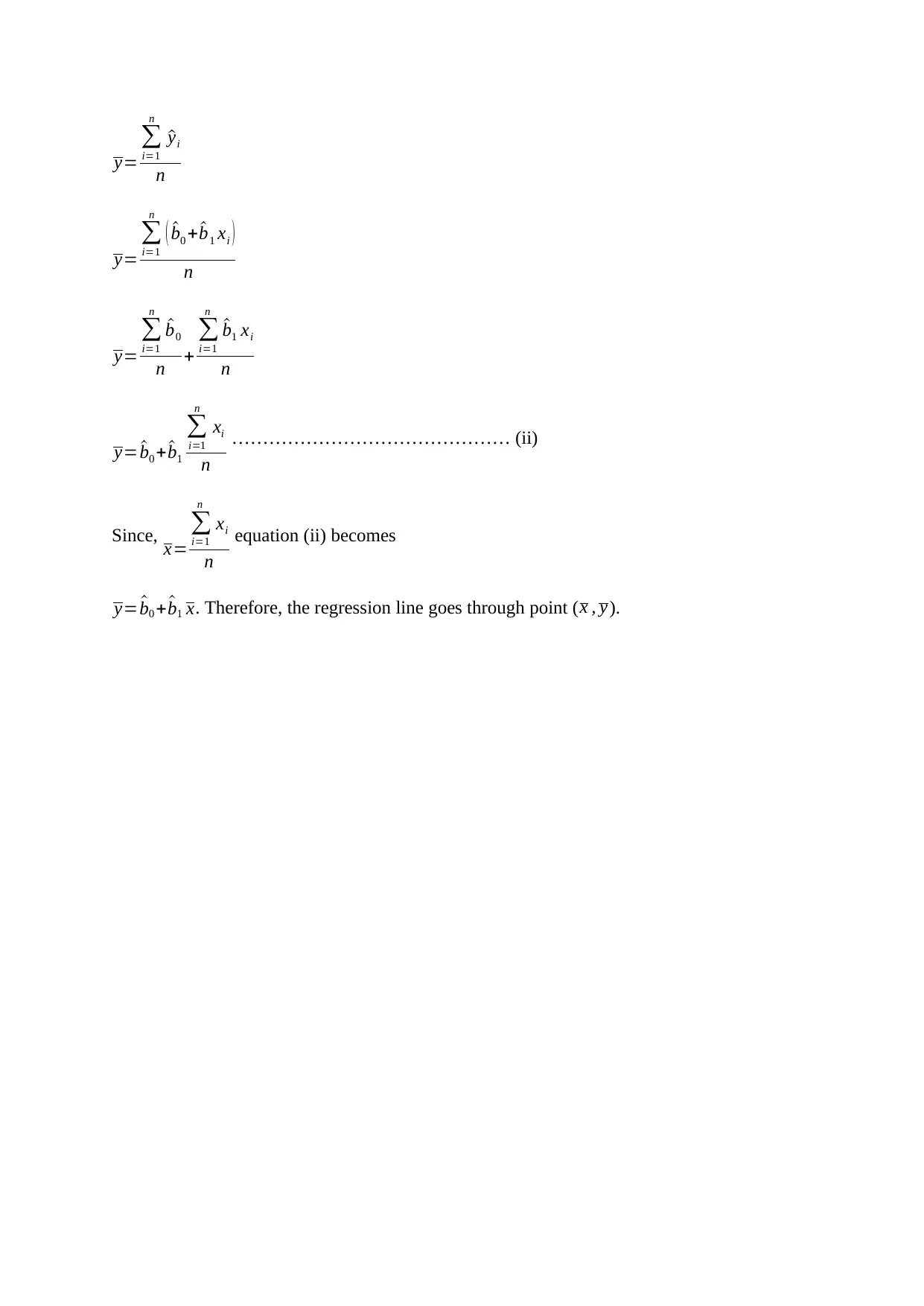
y=
∑
i=1
n
^yi
n
y=
∑
i=1
n
( ^b0 + ^b1 xi )
n
y=
∑
i=1
n
^b0
n +
∑
i=1
n
^b1 xi
n
y= ^b0 + ^b1
∑
i=1
n
xi
n
……………………………………… (ii)
Since, x=
∑
i=1
n
xi
n
equation (ii) becomes
y= ^b0 + ^b1 x. Therefore, the regression line goes through point ( x , y).