University of Portsmouth Engineering Analysis Coursework 3

This document presents a solved coursework assignment for Engineering Analysis, specifically for the School of Civil Engineering and Surveying at the University of Portsmouth. The assignment covers three main sections: vectors, differentiation and integration, and applications. Section A includ...Read More

Section A
Question 1⃗
a=3i+j ; ⃗ b=2i+j+3k⃗
a .⃗ b= |a||b|cosƟ
7= √ 10∗ √ 14 cosƟ
Ɵ=cos−1
( 7
√ 140 )
=cos−1 ( .591607 )
=53.72880
Approximately = 540
Question 2
F1=15N in direction j
F2=XY N in the direction of i+2j+k
Where XY=86
F1=15j ; F2=86(i +2 j + k)
F1+F2=86 i+187 j+86 k
Magnitude of this resultant= √ 862 +1872 +862
=223.071N
That is approximately = 223.1N
Question 3⃗
a=3i+j⃗
b=2i+j+3k
Vector perpendicular to these two vectors is the cross product of the two vectors.
That is we have to find ⃗ a ×⃗ b,

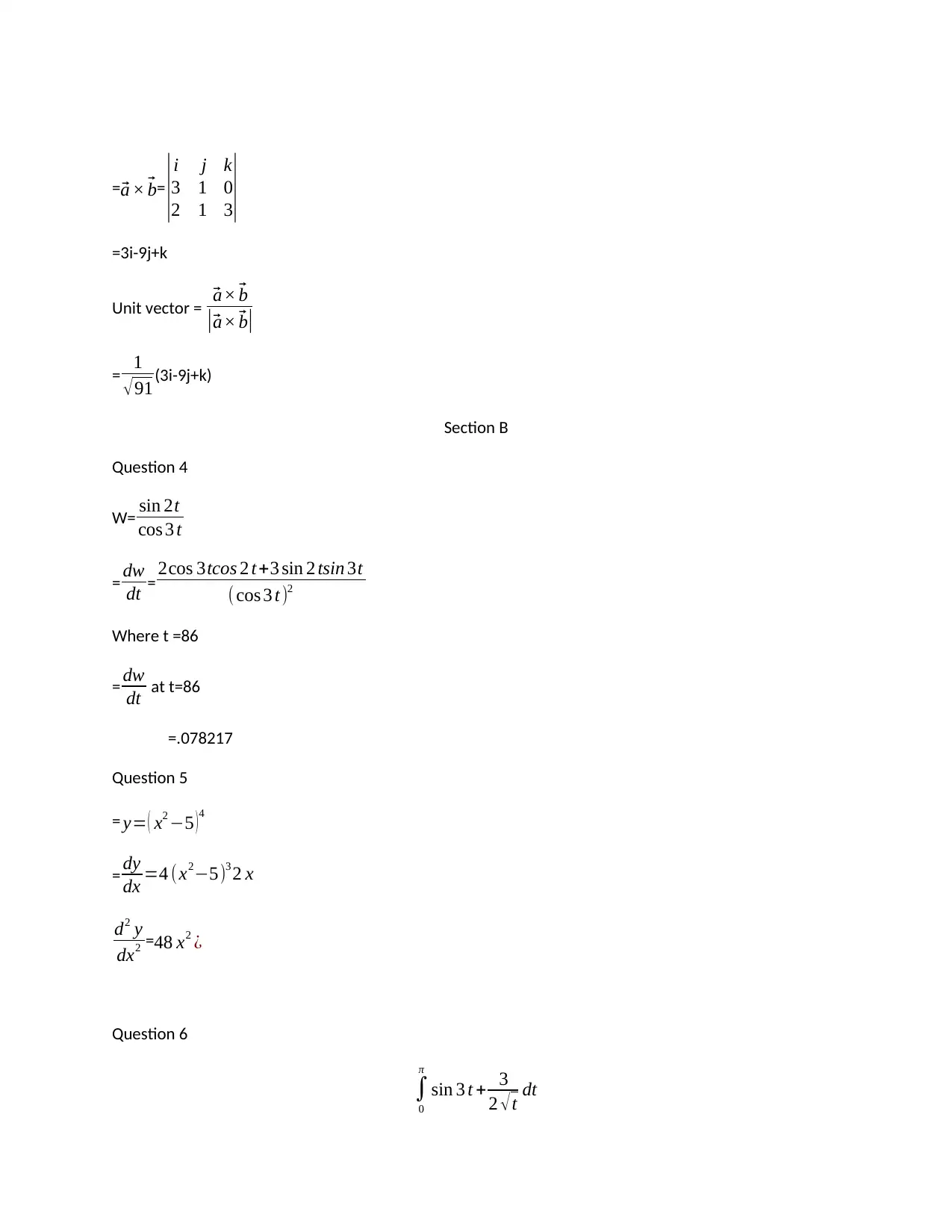
=⃗a ×⃗ b=
|i j k
3 1 0
2 1 3|
=3i-9j+k
Unit vector = ⃗ a×⃗ b
|⃗a×⃗ b|
= 1
√91(3i-9j+k)
Section B
Question 4
W= sin 2t
cos 3 t
= dw
dt = 2cos 3tcos 2 t+3 sin 2 tsin 3t
( cos 3 t)2
Where t =86
= dw
dt at t=86
=.078217
Question 5
= y= ( x2 −5 )4
= dy
dx =4 (x2−5)3 2 x
d2 y
dx2 =48 x2 ¿
Question 6
∫
0
π
sin 3 t + 3
2 √ t dt

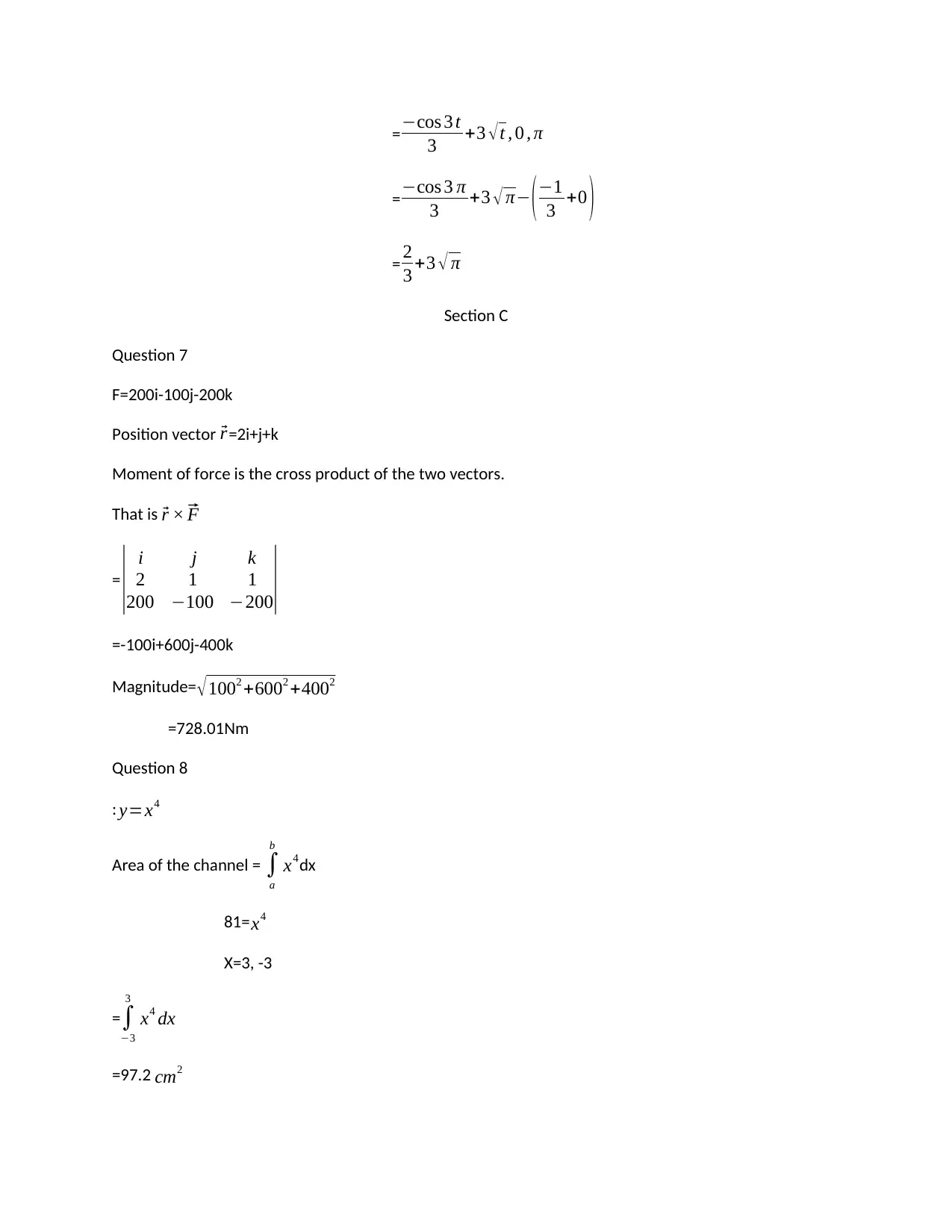
=−cos 3 t
3 +3 √ t , 0 , π
=−cos 3 π
3 +3 √ π− (−1
3 +0 )
= 2
3 +3 √ π
Section C
Question 7
F=200i-100j-200k
Position vector ⃗ r=2i+j+k
Moment of force is the cross product of the two vectors.
That is ⃗ r ×⃗ F
=
| i j k
2 1 1
200 −100 −200|
=-100i+600j-400k
Magnitude= √ 1002 +6002 +4002
=728.01Nm
Question 8
: y=x4
Area of the channel = ∫
a
b
x4dx
81=x4
X=3, -3
=∫
−3
3
x4 dx
=97.2 cm2

There for required area =81*6-97.2
=388.8 cm2
Question 9
y= x
XY ∗105 ( 7∗104−103 x2 +3 x4 ); XY=86
= x
86∗105 ( 7∗104 −103 x2 +3 x4 )……………………………………………………………1)
Putting y=0
x
86∗105 ( 7∗104 −103 x2 +3 x4 ) =0
7∗104 −103 x2 +3 x4=0
Let x2=t
So the given equation becomes,
3t2−10 t+7∗104=0
t=1.667
That is x2=1.667
x=1.2909m
Putting this value of x in equation 1)
We get y= 1.2909
86∗105 ( 7∗104 −103∗1.667 +3(1.667)4 )
=0.0102m
There fore the deflection is 0.0102m.