SOLID MECHANICS I ENG1066

This document contains solutions to problems related to solid mechanics, including free-body diagrams, conditions for block A to be raised up the incline, acceleration of block A, minimum magnitude of friction force, and pure rolling motion of a sphere. The subject is SOLID MECHANICS I ENG1066 and the document type is Solved Assignment.

1SOLID MECHANICS I ENG1066
Solid Mechanics I ENG1066
Name
The Name of the Class (Course)
Professor (Tutor)
The Name of the School (University)
The City and State where it is located
The Date

2SOLID MECHANICS I ENG1066
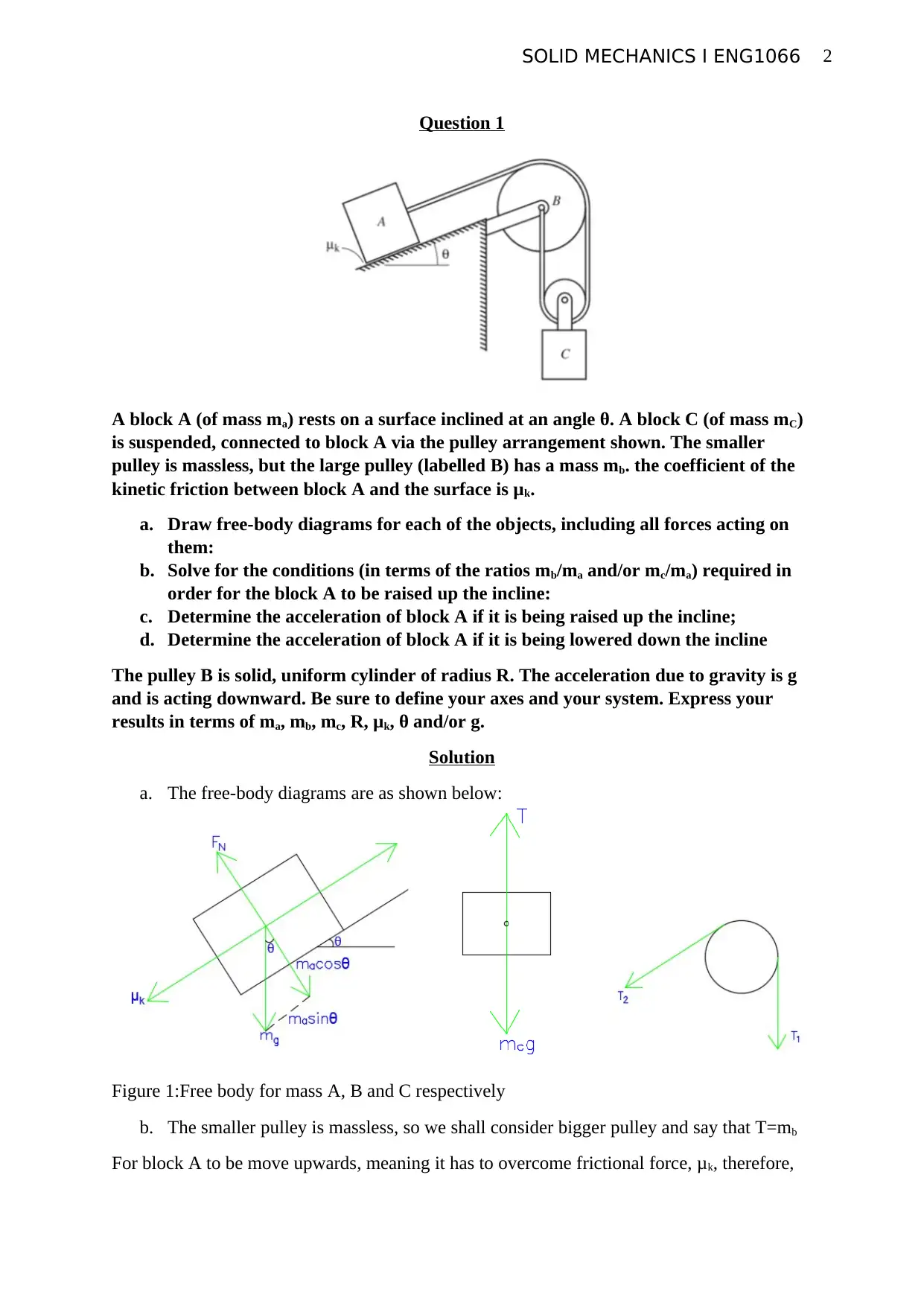
Question 1
A block A (of mass ma) rests on a surface inclined at an angle θ. A block C (of mass mC)
is suspended, connected to block A via the pulley arrangement shown. The smaller
pulley is massless, but the large pulley (labelled B) has a mass mb. the coefficient of the
kinetic friction between block A and the surface is μk.
a. Draw free-body diagrams for each of the objects, including all forces acting on
them:
b. Solve for the conditions (in terms of the ratios mb/ma and/or mc/ma) required in
order for the block A to be raised up the incline:
c. Determine the acceleration of block A if it is being raised up the incline;
d. Determine the acceleration of block A if it is being lowered down the incline
The pulley B is solid, uniform cylinder of radius R. The acceleration due to gravity is g
and is acting downward. Be sure to define your axes and your system. Express your
results in terms of ma, mb, mc, R, μk, θ and/or g.
Solution
a. The free-body diagrams are as shown below:
Figure 1:Free body for mass A, B and C respectively
b. The smaller pulley is massless, so we shall consider bigger pulley and say that T=mb
For block A to be move upwards, meaning it has to overcome frictional force, μk, therefore,

3SOLID MECHANICS I ENG1066
μk =ma g sin θ
That means that
mc >ma g sin θ
To satisfy the condition it means that condition in terms of the tension (which is mb),
and considering that the weight is moving in the negative Y direction, the expression
shall be:
∑ FY =mb−mc g
The net force in our case shall be ma, where a is the acceleration, therefore the
equation shall be:
−mc a=mb −mc g
mb=mc g−mc a
Alternatively: we can use the block A, where there are two forces now, the X (direction of μk)
and the Y (perpendicular force FN)
∑ F X =T −μk
Which can be written as:
ma=T −ma g sin θ
ma=mb −ma g sin θ
mb=ma + ma g sin θ
c. Therefore, the acceleration of block A moving upwards, shall be:
a= ( ∑ F )
m = mc g−ma g sin θ
mc+ ma
d. On a downward direction, the expression changes:
a= ( ∑ F )
m = mc g+ma g sin θ
mc+ ma
Question 2

4SOLID MECHANICS I ENG1066
A solid, uniform cylinder with radius R and mass m is released from rest on a surface
inclined at an angle θ relative to horizontal, such that it is allowed to roll without
slipping. The coefficient of the static friction between the object and the surface is μs,
and this happens to be exactly equal to the coefficient of kinetic friction μk .
a. Does there exist a minimum magnitude of θ below which the object will remain
‘stuck’ to the surface (and not roll down the surface)? Why or why not?
b. Determine the magnitude of the friction force acting on the object as it rolls.
What happens to the magnitude of the friction if the surface is vertical? What
happens to the magnitude of the friction if the surface is horizontal? Do these
results make sense? Why or why not?
The acceleration due to gravity is g and the acting downward. Be sure to define
your axes and your system. Express your results in terms of m , R , μs , μk , θ∧¿/or
g. Note that, for a cylinder
I zz= 1
2 m R2
Solution
a. Yes, and at this point θ=0. It is important to note that the frictional force μk , is what
makes the cylinder to roll down the plane. Therefore, the cylinder rolls when the
frictional force is sufficient. If the inclination angle is increased, the cylinder will not
roll but slip, this follows that the actual frictional force being equal to the maximum
frictional force. But when the angle is reduced, the cylinder will get to a point where it
will no longer roll, but be static, this means the kinetic friction will be equal to the
static friction. The frictional force, static is given by μs F
∑ F x=0
FN −mg ( sinθ ) =0
Therefore:
FN =mg ( sinθ )
That means when θ=0, then the frictional force is 0, and therefore, there’s no
movement. Also, it means that
μk =μs
b. The moment of inertia must take effect here and be considered, that is why F=ma is
not sufficient. Here, we shall consider torque, which is caused by the surface and the
rolling cylinder.
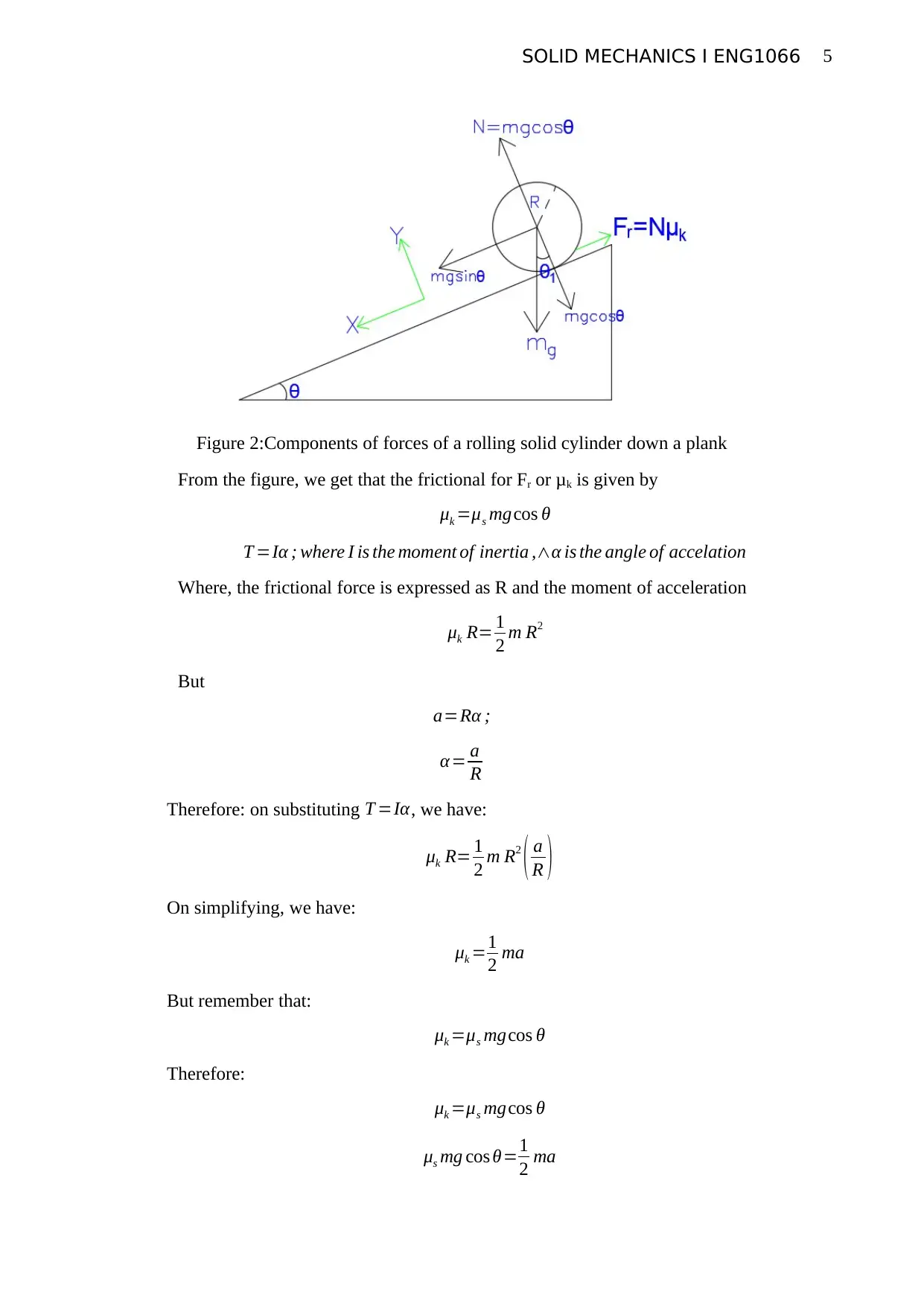
Rolling downwards, the force is mg(sinθ), the one acting perpendicular to the surface
is mg( cosθ) see the figure 2

5SOLID MECHANICS I ENG1066
Figure 2:Components of forces of a rolling solid cylinder down a plank
From the figure, we get that the frictional for Fr or μk is given by
μk =μs mgcos θ
T =Iα ; where I is the moment of inertia ,∧α is the angle of accelation
Where, the frictional force is expressed as R and the moment of acceleration
μk R= 1
2 m R2
But
a=Rα ;
α= a
R
Therefore: on substituting T =Iα, we have:
μk R= 1
2 m R2
( a
R )
On simplifying, we have:
μk =1
2 ma
But remember that:
μk =μs mgcos θ
Therefore:
μk =μs mgcos θ
μs mg cos θ=1
2 ma

6SOLID MECHANICS I ENG1066
μs g cos θ=1
2 a
μs = 1
2 g cos θ a
At a vertical angle, the inclination angle shall be 90O, therefore the equation shall be
μs = 1
2 g−0.448 a
It takes a negative value which is not the case, since the friction due to slip should be
zero and the frictional (kinetic) is infinity, and the body is not moving
At horizontal, the inclination angle is zero, and therefore, the friction magnitude
(kinetic) is should be infinity, at this moment the body is at rest and the slip should be
zero.
Therefore, both the first and second statements does not make sense when we use the
equation,
Question 3
A solid, uniform sphere of mass m and radius R is given an initial ‘backspin’ of angular
velocity ω0 before being released from rest on a surface inclined at an angle ϑ . The
coefficient of kinetic friction between the sphere and the surface is μk . Obtain an
expression for the distance travelled by the sphere at the instant that pure rolling
motion begins. Under what conditions (if any exist) will sphere:
a. Begin moving down the incline immediately upon release:
b. Undergo (at some point) pure rolling motion up the incline
c. Begin pure rolling motion at the same instant it begins moving down the incline?
The acceleration due to gravity is g and is acting downward. Be sure to define your
axes and your system. Express your results in terms of m , R , μk , θ∧¿ g. Note that for
a sphere
I zz= 2
5 m R2

7SOLID MECHANICS I ENG1066
Solution
In this case, there are two forms of energies, the one before the sphere is released and the
energy where it ends.
E0 =E1
Where E0 is potential energy (PE), and E1 is the potential energy at the instant when the
sphere has reached its maximum travelled distance and therefore has stopped, but before that,
there was a backspin, therefore that was a kinetic energy (which is split into know two)
PE0=PE1 + KE1 + KE2
1
2 μk x2 =mgh+ 1
2 m v2 + 1
2 I ω2
Since the sphere is rolling up the plank, it will form a triangle with an angle θ and that
distance there becomes the hypotenuse therefore h=dsinθ, also we know that I = 2
5 m R2,
therefore:
1
2 μk x2 =mgdsinθ+ 1
2 m v2 +1
2 ( V
R )
2
( 2
5 m R2
)
1
2 μk x2 =mgdsinθ+ 1
2 m v2 +1
5 mv2
5 μk x2 =10 mgdsinθ+5 m v2+ 2mv2=7 m v2
5 μk x2 =mgdsinθ+7 m v2
5 μk x2−7 m v2
10 mgsinθ =d
a. The sphere begins moving down the incline the moment it has reached the maximum
height and the frictional force/stalling force has acted on it and is equal to the force
making it move upwards. There, it will attain a potential energy, before rolling down
the incline. At this point μk =μs
b. There will be no pure rolling motion, the motion will be as a result of the force that
has released it to move up the incline.
The pure rolling motion is attained at the instance of down movement after it has
attained the maximum height and now the frictional force acting on it is enough to
make it begin rolling. When, the μs < μk

8SOLID MECHANICS I ENG1066