MAT 120 Exam 2 Solutions: Derivatives, Graphs, and Applications

This document presents the complete solutions to the MAT 120 Exam 2, a calculus assignment from Spring 2019. The solutions cover a range of calculus topics, including finding derivatives of various functions using the power rule, chain rule, and product rule, and also includes the differentiation of...Read More

Solution 1(a): Given . Differentiate with respect to x we get
Hence,
1(b): Given . Differentiate with respect to x we get
Hence,
1(c): Given . Differentiate with respect to x we get
Hence,
Solution 2(a): Given . Differentiate with respect to x we get
Hence,
2(b): Given . Differentiate with respect to x and use chain rule of
differentiation we get
Hence,
2(c): Given . Differentiate with respect to x and use product rule of
differentiation we get
Hence,
Solution 3(a): Given
The graph of the given piecewise function is shown below

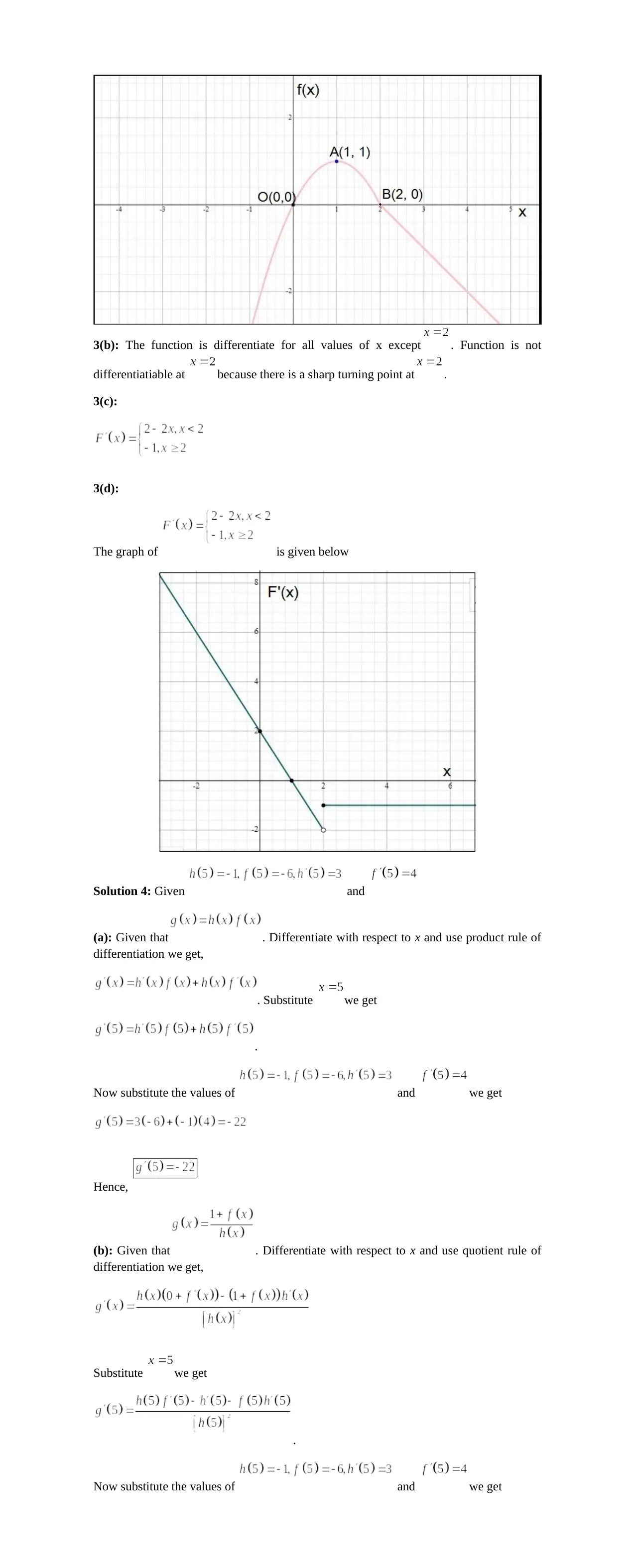
3(b): The function is differentiate for all values of x except . Function is not
differentiatiable at because there is a sharp turning point at .
3(c):
3(d):
The graph of is given below
Solution 4: Given and
(a): Given that . Differentiate with respect to x and use product rule of
differentiation we get,
. Substitute we get
.
Now substitute the values of and we get
Hence,
(b): Given that . Differentiate with respect to x and use quotient rule of
differentiation we get,
Substitute we get
.
Now substitute the values of and we get

Hence,
Solution 5: Given curve is . Differentiate with respect to x and solve
for we get
At ,
The equation of tangent line at point is
Hence, the equation of tangent line is
Solution 6:
(a): From the above table
(b): From the above table,
(c): From the table,
(d): We know that
Solution 7(a): The volume of the spherical balloon whose radius r is by
. Now differentiate with respect to t we get
Given that the spherical balloon is being inflated by a pump at the rate of 2 cubic inches
per second that is the volume of balloon increasing at the rate of that is
. We need to calculate the rate of change at which radius of balloon
increases when radius is inches this means that we need to calculate .
Substitute the value of and value of r in equation (1) we get,

Hence, the radius of balloon increases at the rate of inches per second.
7(b):
Use Pythagorean Theorem in above triangle we get
… (1)
Differentiate both sides with respect to t we get
Since x = 4 feet, use this value in equation we get feet. Given that feet
per second. So from equation (2) we get
.
Hence, the top of the ladder is sliding down (because of the negative sign in the result) at
a rate of .
Solution 8(a): The derivative of with respect to x is the function and is
defined as,
8(b): Given this implies that
Now,
So,

Hence,