Solutions to Differential Equations and Integrals

Get step-by-step solutions to differential equations and integrals. Solve initial value problems and evaluate integrals with Desklib. Expert assistance available 24/7.

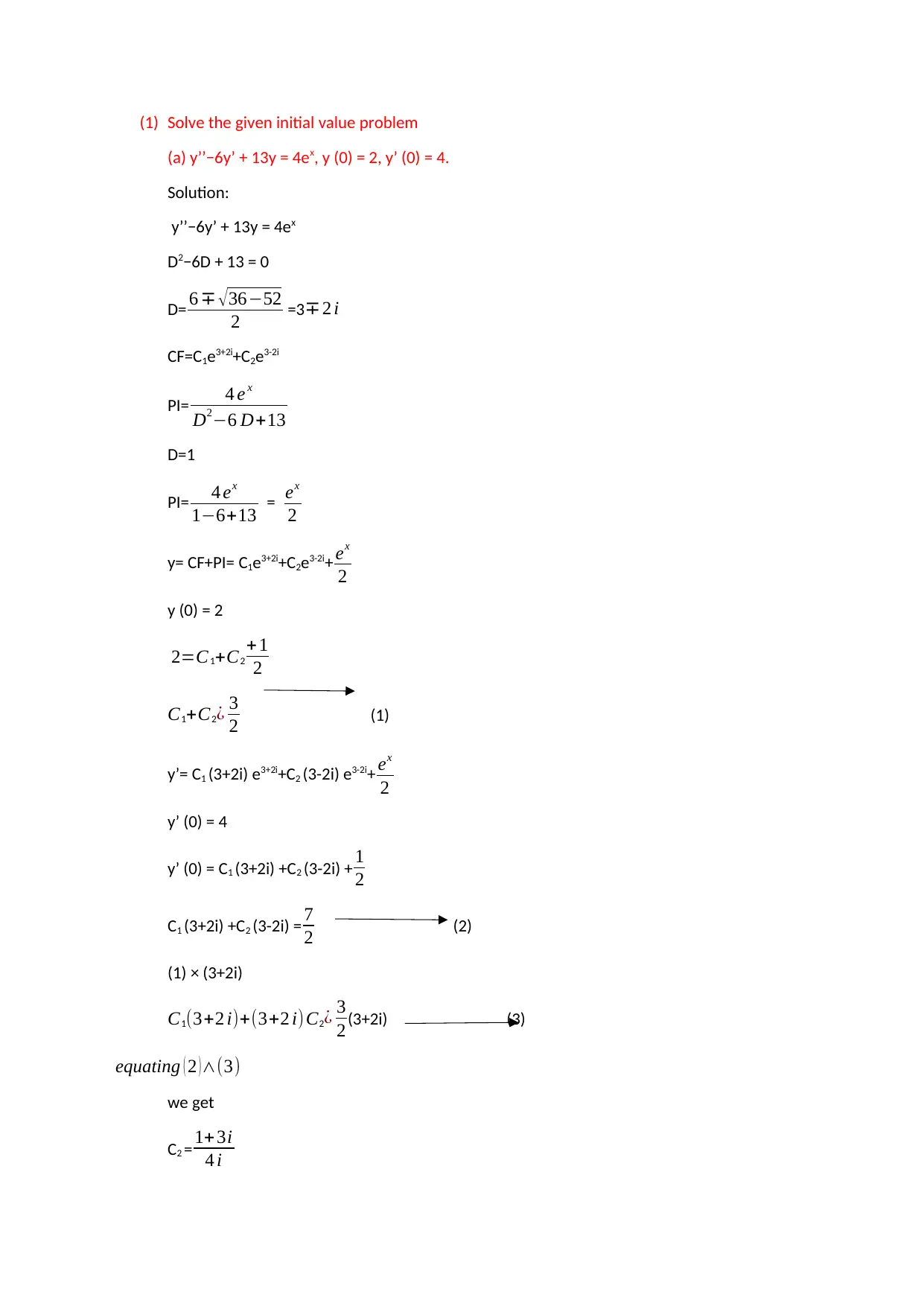
(1) Solve the given initial value problem
(a) y’’−6y’ + 13y = 4ex, y (0) = 2, y’ (0) = 4.
Solution:
y’’−6y’ + 13y = 4ex
D2−6D + 13 = 0
D= 6 ∓ √ 36−52
2 =3 ∓ 2 i
CF=C1e3+2i+C2e3-2i
PI= 4 e x
D2−6 D+13
D=1
PI= 4 ex
1−6+13 = ex
2
y= CF+PI= C1e3+2i+C2e3-2i+ ex
2
y (0) = 2
2=C1+C2
+ 1
2
C1+C2¿ 3
2 (1)
y’= C1 (3+2i) e3+2i+C2 (3-2i) e3-2i+ ex
2
y’ (0) = 4
y’ (0) = C1 (3+2i) +C2 (3-2i) + 1
2
C1 (3+2i) +C2 (3-2i) = 7
2 (2)
(1) × (3+2i)
C1(3+2 i)+(3+2 i)C2¿ 3
2(3+2i) (3)
equating ( 2 ) ∧(3)
we get
C2 = 1+3i
4 i

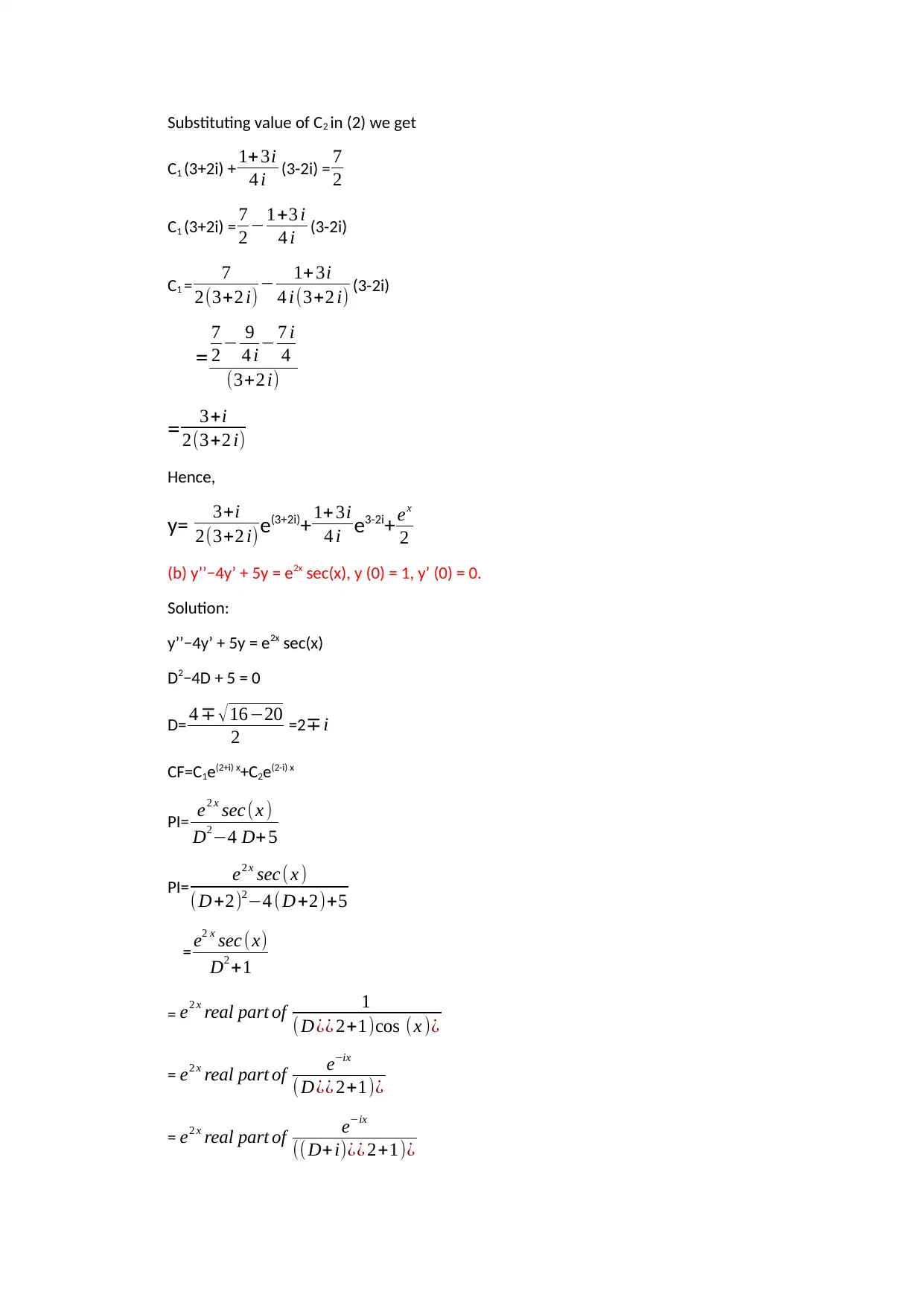
Substituting value of C2 in (2) we get
C1 (3+2i) + 1+ 3i
4 i (3-2i) = 7
2
C1 (3+2i) = 7
2 −1+3 i
4 i (3-2i)
C1 = 7
2(3+2 i) − 1+ 3i
4 i(3+2 i) (3-2i)
=
7
2 − 9
4 i− 7 i
4
(3+2 i)
= 3+i
2(3+2 i)
Hence,
y= 3+i
2(3+2 i)e(3+2i)+ 1+ 3i
4 i e3-2i+ex
2
(b) y’’−4y’ + 5y = e2x sec(x), y (0) = 1, y’ (0) = 0.
Solution:
y’’−4y’ + 5y = e2x sec(x)
D2−4D + 5 = 0
D= 4 ∓ √ 16−20
2 =2∓ i
CF=C1e(2+i) x+C2e(2-i) x
PI= e2 x sec (x )
D2−4 D+ 5
PI= e2 x sec ( x )
( D+2)2−4 ( D+2)+5
= e2 x sec(x)
D2 +1
= e2 x real part of 1
( D¿¿ 2+1)cos (x )¿
= e2 x real part of e−ix
( D¿¿ 2+1)¿
= e2 x real part of e−ix
(( D+i)¿¿ 2+1)¿

= e2 x real part of e−ix
D2−2 Di
= e2 x real part of e−ix 1
2 Di ¿
= e2 x real part of e−ix 1
2 Di [1+ D
2 i + ( D
2i )
2
+…]
= e2 x real part of e−ix (−1
2 i )∫1 dx
= e2 x real part of e−ix (−1
2 i ) x
= e2 x real part of ( cos ( x ) −isin ( x ) )(−1
2i ) x
¿ e2 x x sin ( x )
2
y= CF+PI= C1e(2+i) x+C2e(2-i) x+ e2 x x sin ( x )
2
y (0) = 1
1= C1+C2 (1)
y’ (0) = 0.
y’= (2+i) e(2+i) x+(2-i) e(2-i) x+ ¿ ¿ ¿
0= (2+i) e(2+i) x+(2-i) e(2-i) x (2)
(1) × (2+i)
(2+i) =(2+i) C1+(2+i) C2 (3)
equating ( 2 )∧(3)
we get
C2 = 2+i
2 i
Substituting value of C2 in (1) we get
C1+ 2+ i
2 i =1
C1=1- 2+ i
2 i
C1= i−2
2i

Hence,
y= i−2
2i e(2+i) x+ 2+ i
2 i e(2-i) x+ e2 x x sin ( x )
2
(2) (a) Show that any equation of the form
M’(x)N (y) + M(x)N’(y) dy
dx =0
is exact.
Show that the equation
exp ( x2 ) sin ( y2)
y + exp ( x2 ) cos ( y2 )
x
dy
dx =0
is not exact. Find a separable function T(x,y) = g(x)h(y) such that
T ( x , y ) exp ( x2 ) sin ( y2 )
y +T ( x , y ) exp ( x2 ) cos ( y2)
x
dy
dx =0
is exact and solve it.
Solution:
M’(x)N (y) + M(x)N’(y) dy
dx =0
du =M’(x)N (y) dx + M(x)N’(y)dy =0 (1)
du= ∂ u
∂ x dx += ∂u
∂ y dy (2)
∂ u
∂ x =M’(x)N (y) (3)
∂u
∂ y = M(x)N’(y) (4)
From (3) and (4)
∂(M ' ( x) N ( y ))
∂ y = ∂(M ( x) N ' ( y ))
∂ x
∂(M ' ( x) N ( y ))
∂ y = exp ( x2 ) sin ( y2)
y
=ex2 (2 y¿ ¿2 cosy 2−siny2 )
y2 ¿
∂(M (x) N ' ( y ))
∂ x = exp ( x2 ) cos ( y2 )
x

=cosy2 (2 x¿ ¿2 ex2
−ex2
)
x2 ¿
Multiplying both sides by I.F,
I.F=
e∫
∂ (M ( x)N ' (y ))
∂ x − ∂(M ' (x)N ( y))
∂ y
M ’(x)N ( y)
=
e
∫
ex2
cos y2(2 x2−1)
x2 − ex2
(2 y2 cos y2−sin y2 )
y2
ex2
sin y2
y
=
e
∫
y2 (e¿¿ x2 cos y2(2 x2−1))− x2 ex2
(2 y2 cos y2−sin y2)
x2 y2
ex2
sin y2
y
¿
=e∫( 1
y ¿− ycot y2)dy ¿
Put y2=t ;2 ydy =dt
=elogydy−log √ sint dt
= y
√sin y2
There fore, T(x,y)= y
√sin y2
(3) (a) Show that;
arcoth(x) = 1
2 ln (x +1)
( x−1),|x|>1.
Solution:
arcoth(x) = tanh-1x = 1
2 ln (x +1)
( x−1),|x|>1.
tanh-1x = y
x = tanh(y)
x = e y−e− y
e y+ e− y
( e y +e− y ¿ x = e y−e− y
x ey + x e− y = e y−e− y
x e2 y+ x
e y = e2 y −1
e y
x e2 y + x=e2 y−1

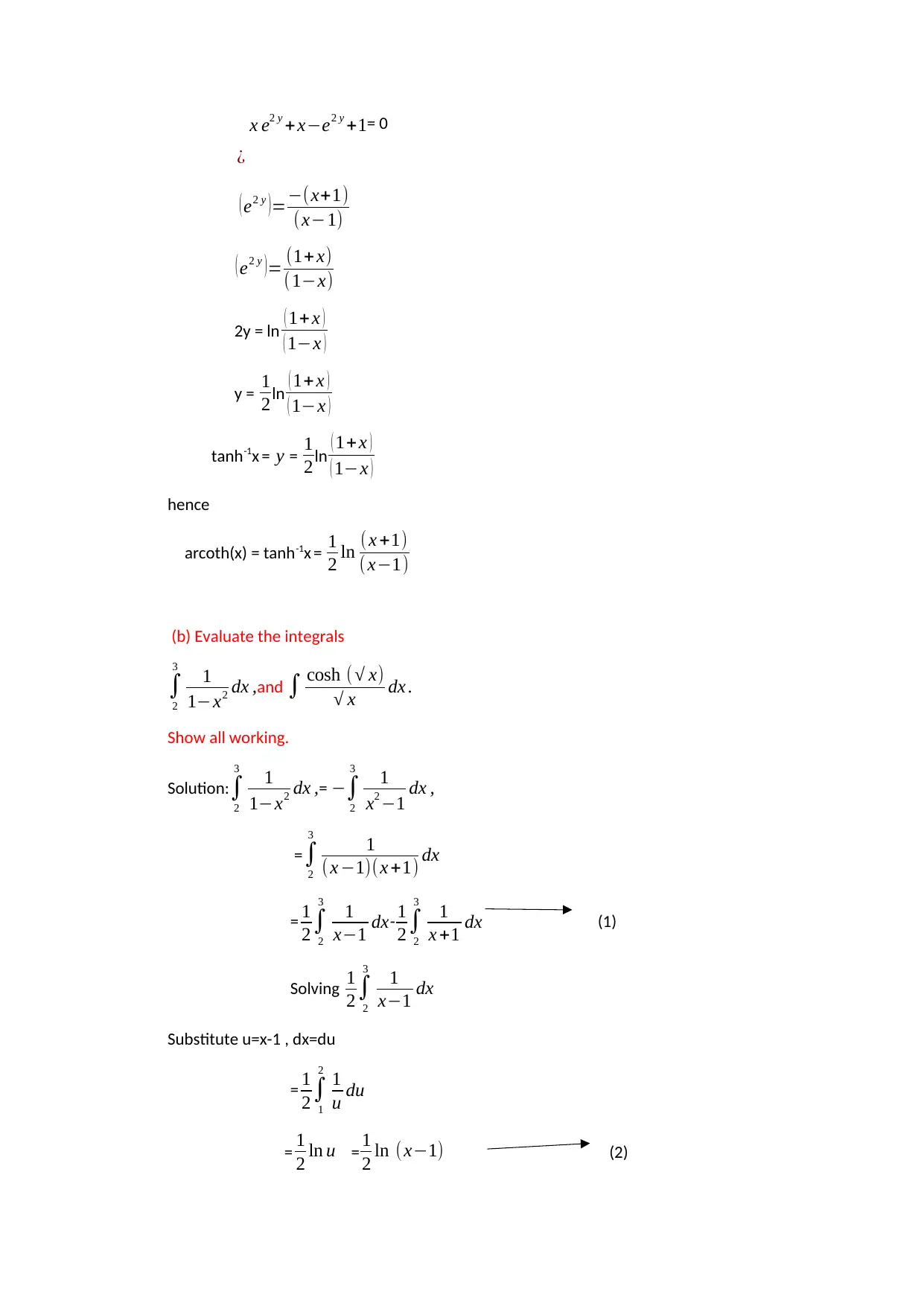
x e2 y + x−e2 y +1= 0
¿
( e2 y )=−(x+1)
(x−1)
( e2 y )= (1+ x)
(1−x)
2y = ln ( 1+x )
( 1−x )
y = 1
2ln ( 1+ x )
( 1−x )
tanh-1x = y = 1
2ln ( 1+ x )
( 1−x )
hence
arcoth(x) = tanh-1x = 1
2 ln ( x +1)
( x−1)
(b) Evaluate the integrals
∫
2
3
1
1−x2 dx ,and ∫ cosh ( √ x)
√ x dx .
Show all working.
Solution:∫
2
3
1
1−x2 dx ,= −∫
2
3
1
x2 −1 dx ,
=∫
2
3
1
( x −1)(x +1) dx
= 1
2∫
2
3
1
x−1 dx- 1
2∫
2
3
1
x +1 dx (1)
Solving 1
2∫
2
3
1
x−1 dx
Substitute u=x-1 , dx=du
= 1
2∫
1
2
1
u du
= 1
2 ln u = 1
2 ln ( x−1) (2)

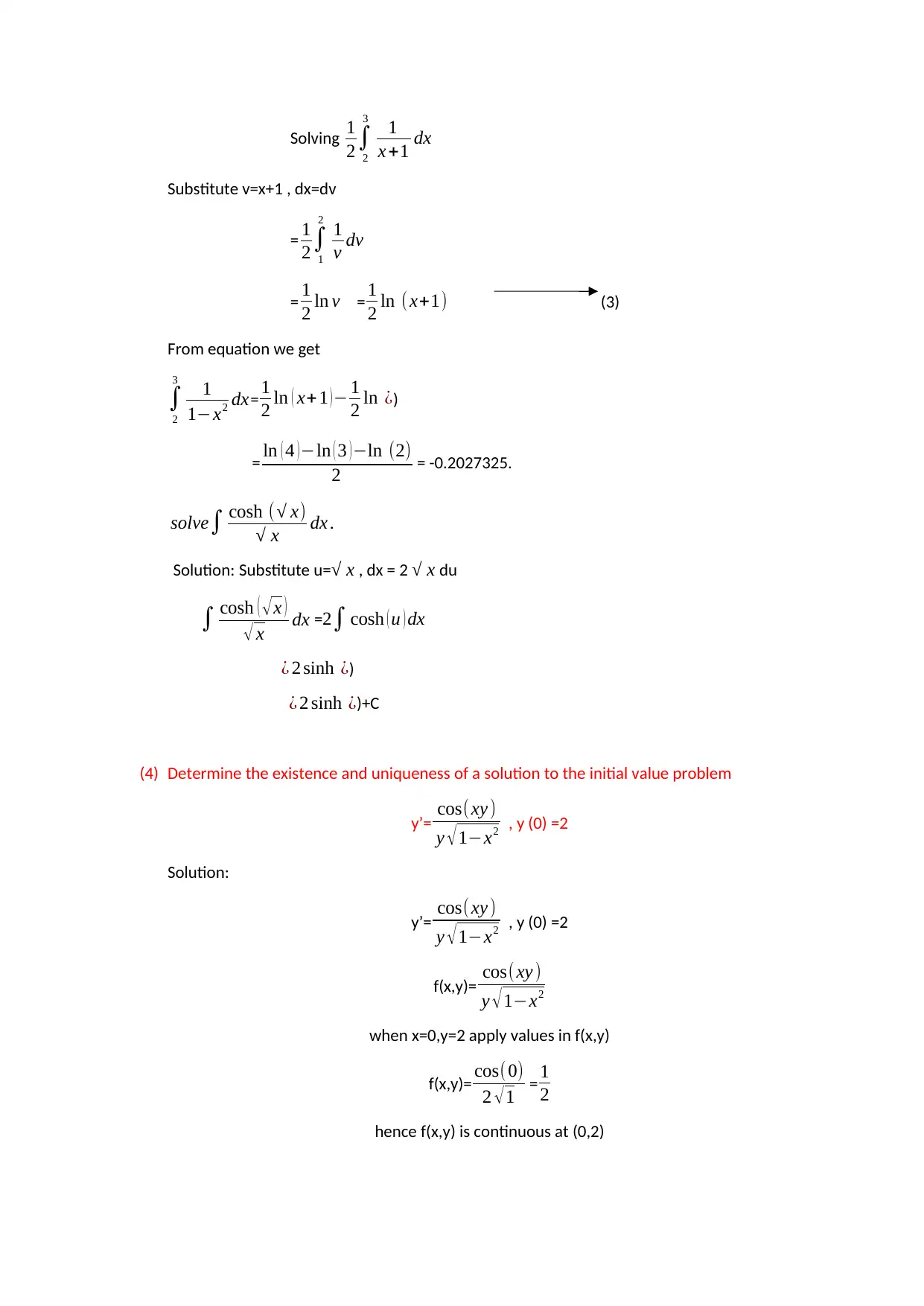
Solving 1
2∫
2
3
1
x +1 dx
Substitute v=x+1 , dx=dv
= 1
2∫
1
2
1
v dv
= 1
2 ln v = 1
2 ln ( x+1) (3)
From equation we get
∫
2
3
1
1−x2 dx= 1
2 ln ( x+ 1 )− 1
2 ln ¿)
= ln ( 4 )−ln ( 3 )−ln (2)
2 = -0.2027325.
solve∫ cosh (√ x)
√ x dx .
Solution: Substitute u= √ x , dx = 2 √ x du
∫ cosh ( √x )
√ x dx =2∫ cosh ( u ) dx
¿ 2 sinh ¿)
¿ 2 sinh ¿)+C
(4) Determine the existence and uniqueness of a solution to the initial value problem
y’= cos(xy )
y √ 1−x2 , y (0) =2
Solution:
y’= cos(xy )
y √ 1−x2 , y (0) =2
f(x,y)= cos( xy )
y √ 1−x2
when x=0,y=2 apply values in f(x,y)
f(x,y)= cos( 0)
2 √ 1 = 1
2
hence f(x,y) is continuous at (0,2)

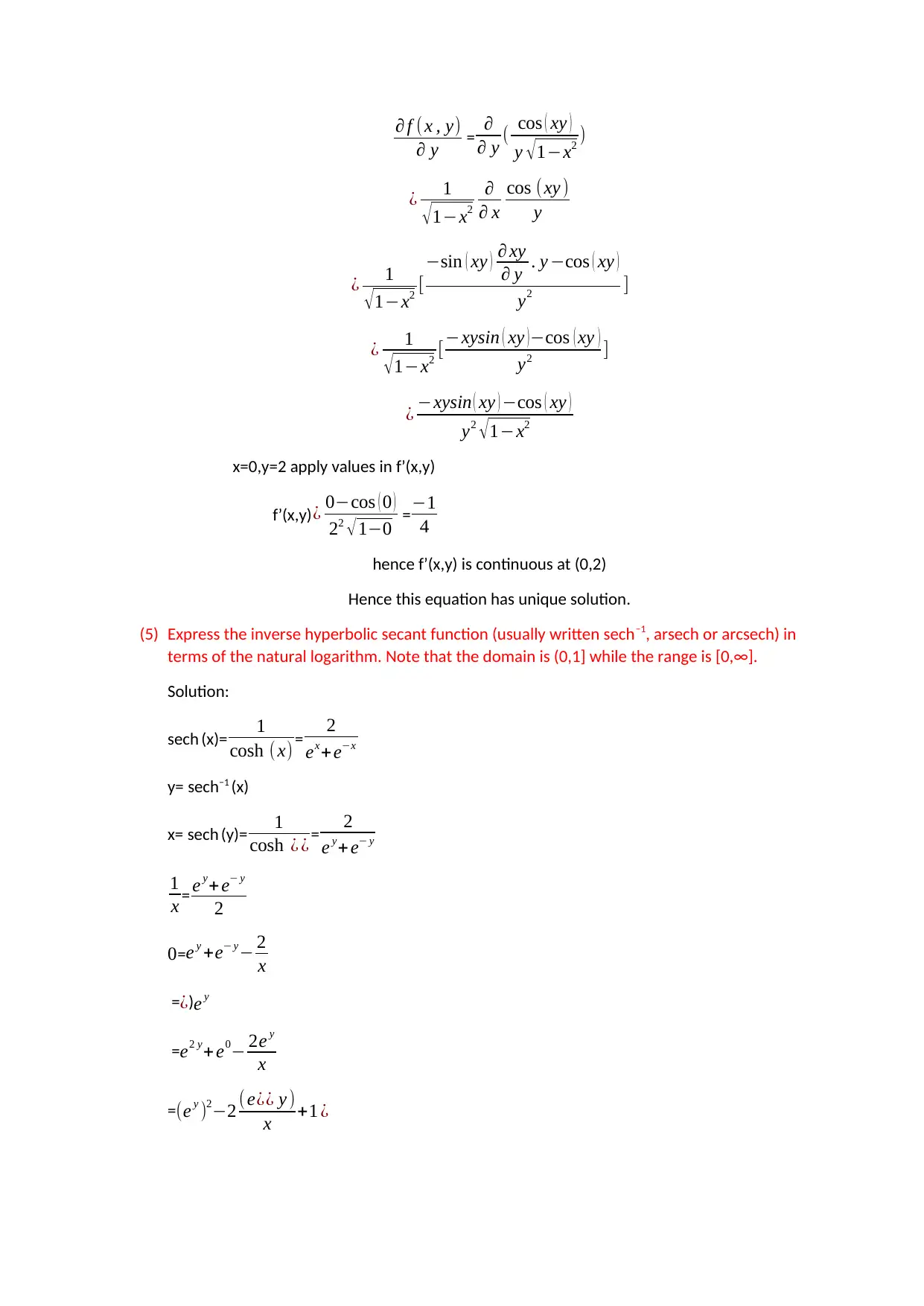
∂ f (x , y)
∂ y = ∂
∂ y ( cos ( xy )
y √ 1−x2 )
¿ 1
√1−x2
∂
∂ x
cos (xy )
y
¿ 1
√1−x2 [
−sin ( xy ) ∂ xy
∂ y . y −cos ( xy )
y2 ]
¿ 1
√ 1−x2 [−xysin ( xy ) −cos ( xy )
y2 ]
¿ −xysin ( xy ) −cos ( xy )
y2 √ 1−x2
x=0,y=2 apply values in f’(x,y)
f’(x,y) ¿ 0−cos ( 0 )
22 √ 1−0 =−1
4
hence f’(x,y) is continuous at (0,2)
Hence this equation has unique solution.
(5) Express the inverse hyperbolic secant function (usually written sech−1, arsech or arcsech) in
terms of the natural logarithm. Note that the domain is (0,1] while the range is [0,∞].
Solution:
sech (x)= 1
cosh ( x) = 2
ex+ e−x
y= sech−1 (x)
x= sech (y)= 1
cosh ¿ ¿= 2
e y+ e− y
1
x = e y+ e− y
2
0=e y +e− y− 2
x
=¿)e y
=e2 y+ e0− 2e y
x
=(e y )2−2 ( e¿¿ y )
x +1 ¿

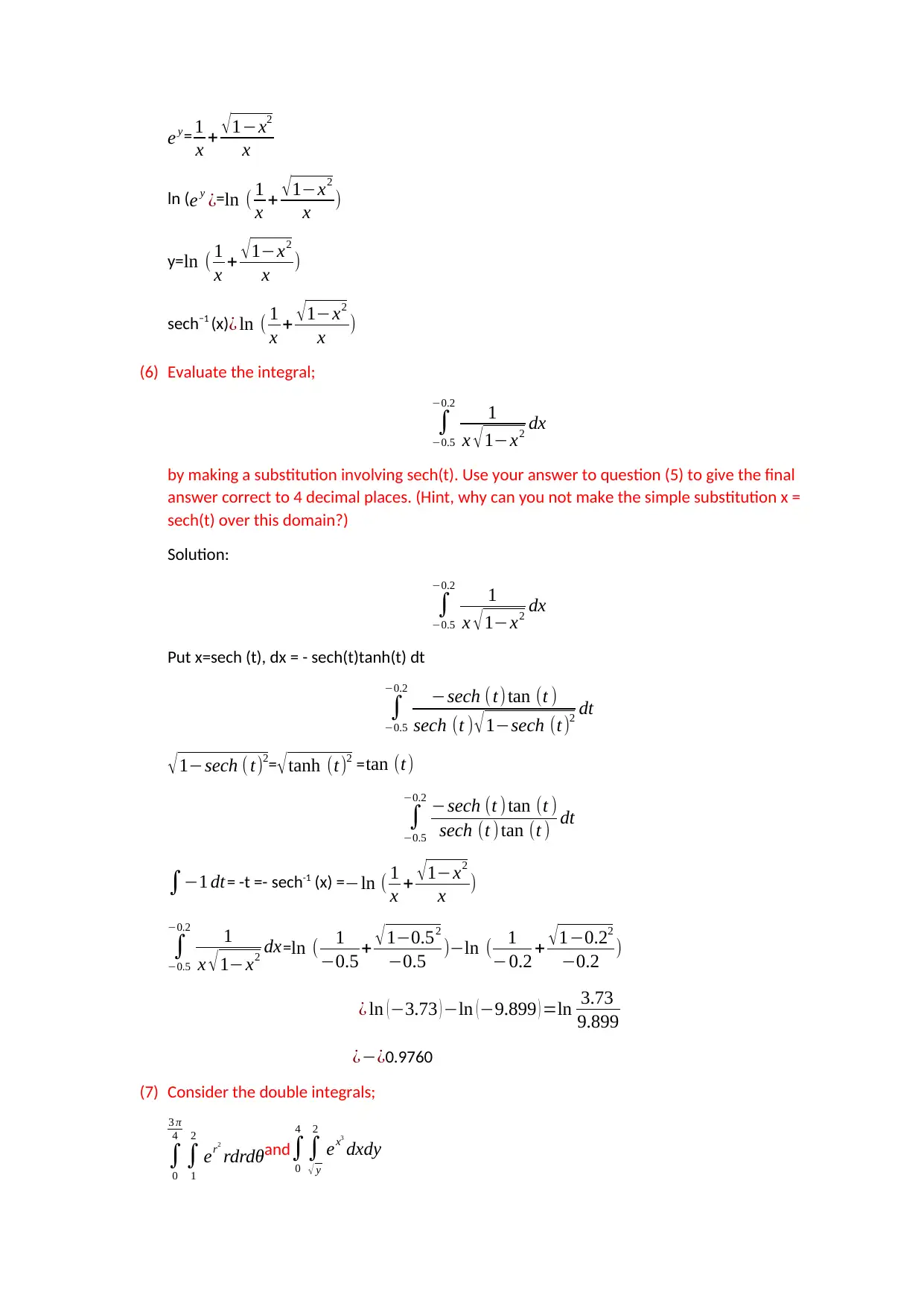
e y= 1
x + √1−x2
x
ln (e y ¿=ln ( 1
x + √1−x2
x )
y=ln ( 1
x + √1−x2
x )
sech−1 (x)¿ ln ( 1
x + √1−x2
x )
(6) Evaluate the integral;
∫
−0.5
−0.2
1
x √ 1−x2 dx
by making a substitution involving sech(t). Use your answer to question (5) to give the final
answer correct to 4 decimal places. (Hint, why can you not make the simple substitution x =
sech(t) over this domain?)
Solution:
∫
−0.5
−0.2
1
x √ 1−x2 dx
Put x=sech (t), dx = - sech(t)tanh(t) dt
∫
−0.5
−0.2 −sech ( t) tan (t )
sech (t ) √1−sech (t)2 dt
√1−sech ( t)2=√ tanh (t)2 =tan (t )
∫
−0.5
−0.2 −sech (t )tan (t )
sech (t ) tan (t ) dt
∫−1 dt= -t =- sech-1 (x) =−ln ( 1
x + √1−x2
x )
∫
−0.5
−0.2
1
x √ 1−x2 dx=ln ( 1
−0.5 + √1−0.52
−0.5 )−ln ( 1
−0.2 + √1−0.22
−0.2 )
¿ ln (−3.73 ) −ln (−9.899 ) =ln 3.73
9.899
¿−¿0.9760
(7) Consider the double integrals;
∫
0
3 π
4
∫
1
2
er2
rdrdθand∫
0
4
∫
√ y
2
ex3
dxdy

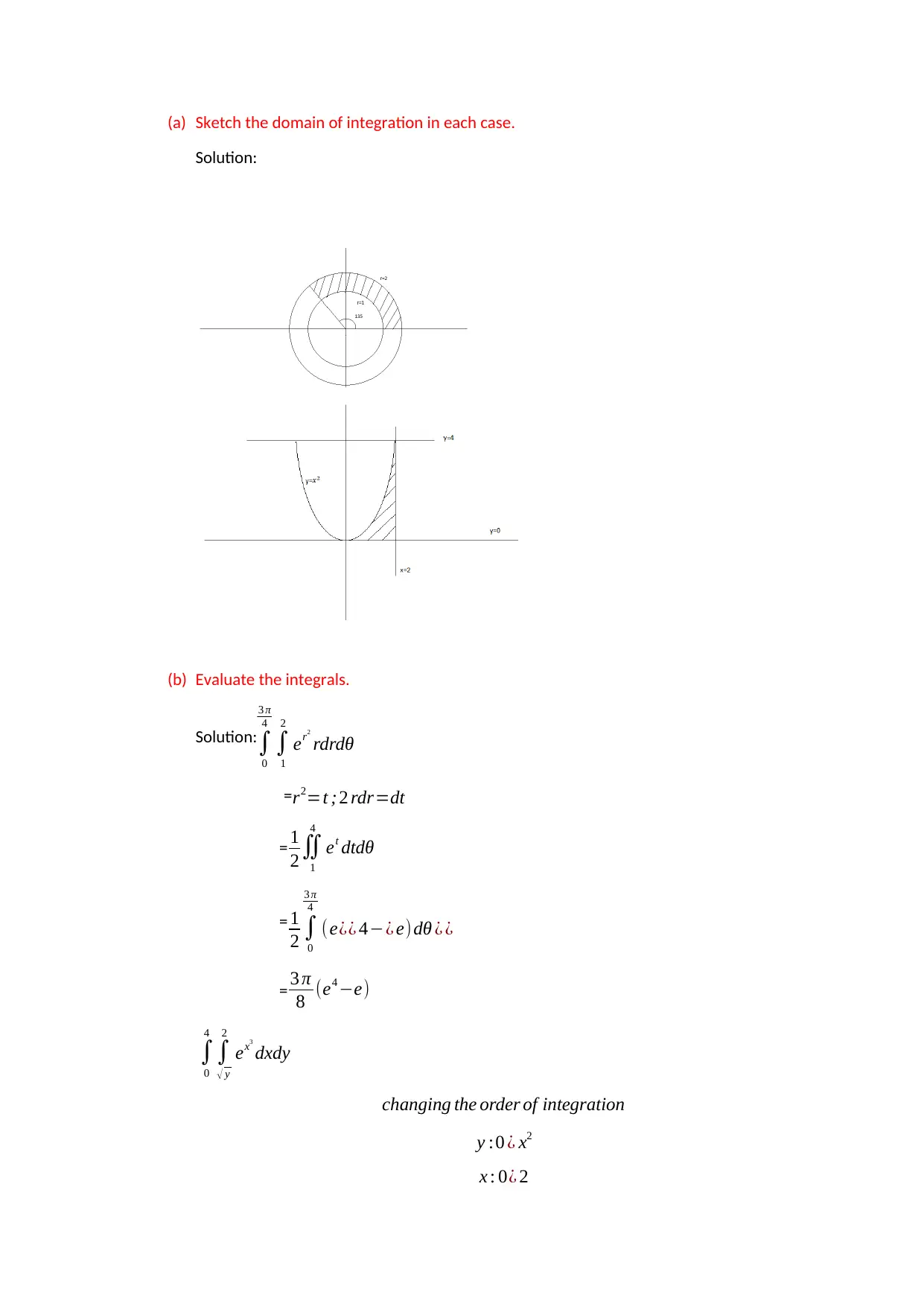
(a) Sketch the domain of integration in each case.
Solution:
(b) Evaluate the integrals.
Solution:
∫
0
3 π
4
∫
1
2
er2
rdrdθ
= r2=t ; 2 rdr=dt
= 1
2∬
1
4
et dtdθ
= 1
2 ∫
0
3 π
4
(e¿¿ 4−¿ e) dθ ¿ ¿
= 3 π
8 (e4 −e)
∫
0
4
∫
√ y
2
ex3
dxdy
changing the order of integration
y :0 ¿ x2
x : 0¿ 2

=∫
0
2
∫
0
x2
ex3
dydx
¿∫
0
2
ex3
y , 0 , x2 dx
¿∫
0
2
ex3
x2 dx; put x3=t ,3 x2 dx =dt
¿ 1
3 ∫
0
8
et dt
= 1
3 ( e8−1)
(8) Consider the expression
I= ∫
0
1
∫
0
1− z2
∫
0
z
dxdydz +∫
0
1
∫
1− z2
1
∫
0
√ 1− y
dxdydz
(a) Determine the value of I by evaluating the iterated integrals given.
Solution:
I= ∫
0
1
∫
0
1− z2
∫
0
z
dxdydz +∫
0
1
∫
1− z2
1
∫
0
√ 1− y
dxdydz
∫
0
1
∫
0
1− z2
∫
0
z
dxdydz= ∫
0
1
∫
0
1− z2
z dydz
=∫
0
1
z (1−z2 ¿) dz ¿
=∫
0
1
z2−z3 dz
= 1
2 − 1
4 = 1
4
∫
0
1
∫
1− z2
1
∫
0
√ 1− y
dxdydz=∫
0
1
∫
1− z2
1
√1− y dydz
Put 1-y =u
∫ √1− y dy =−∫ √u du
2u
3
2
3
= 2(1− y )
3
2
3

∫
0
1
∫
1− z2
1
∫
0
√ 1− y
dxdydz= 2
3 ∫
0
1
z3 dz
= 1
6
I= ∫
0
1
∫
0
1− z2
∫
0
z
dxdydz +∫
0
1
∫
1− z2
1
∫
0
√ 1− y
dxdydz = 5
12
(b) Rewrite I using the order of integration dy dx dz and evaluate the new expression.
Solution:
I= ∫
0
1
∫
0
z
∫
0
1− z2
dydxdz +∫
0
1
∫
0
√ 1− y
∫
1− z2
1
dydxdz
∫
0
1
∫
0
z
∫
0
1− z2
dydxdz = ∫
0
1
∫
0
z
(¿¿1−z2 ) dxdz ¿ ¿
= ∫
0
1
(1−z2 )z dz
=∫
0
1
z2−z3 dz
= 1
2 − 1
4 = 1
4
∫
0
1
∫
0
√ 1− y
∫
1− z2
1
dydxdz=∫
0
1
∫
0
√ 1− y
z2 dxdz
= ∫
0
1
z2 ( √ 1− y ) dz
= 1
3 √1− y
I= ∫
0
1
∫
0
z
∫
0
1− z2
dydxdz +∫
0
1
∫
0
√ 1− y
∫
1− z2
1
dydxdz = 1
4 + 1
3 √1− y
(9) Find the volume of the solid enclosed by the paraboloid z − x2 − y2 = −1 and the hemisphere
z=√ 1−x2− y2
Solution:
z − x2 − y2 = −1
x2 + y2 = z +1…………. (1)
z=√ 1−x2− y2

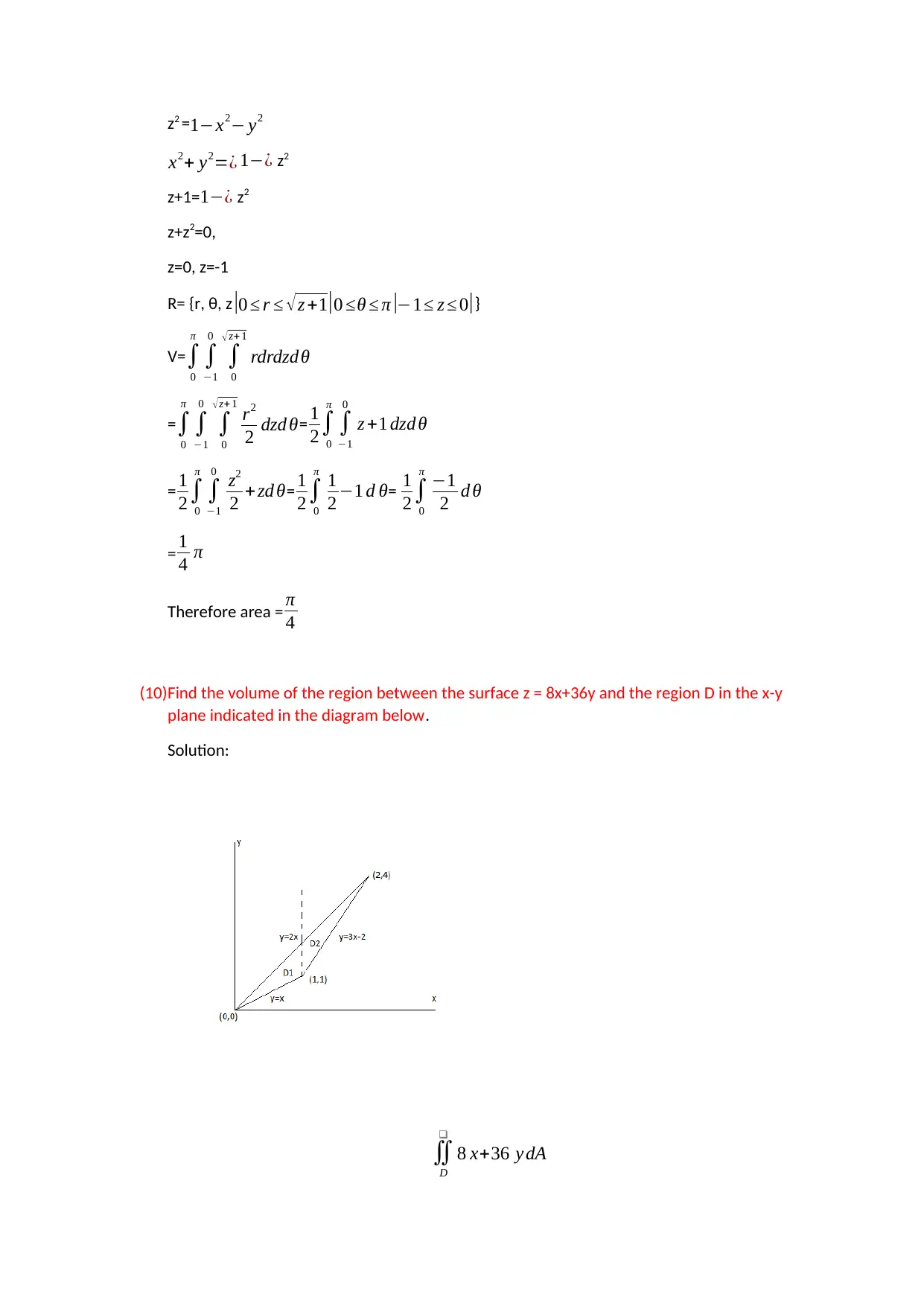
z2 =1−x2− y2
x2+ y2=¿ 1−¿ z2
z+1=1−¿ z2
z+z2=0,
z=0, z=-1
R= {r, θ, z |0 ≤ r ≤ √z +1|0 ≤θ ≤ π |−1≤ z ≤ 0|}
V=∫
0
π
∫
−1
0
∫
0
√z+ 1
rdrdzd θ
=∫
0
π
∫
−1
0
∫
0
√ z+ 1
r2
2 dzd θ= 1
2∫
0
π
∫
−1
0
z +1 dzd θ
= 1
2∫
0
π
∫
−1
0
z2
2 + zd θ= 1
2∫
0
π
1
2 −1 d θ= 1
2∫
0
π
−1
2 d θ
= 1
4 π
Therefore area = π
4
(10)Find the volume of the region between the surface z = 8x+36y and the region D in the x-y
plane indicated in the diagram below.
Solution:
∬
D
❑
8 x+36 y dA

inorder ¿ accomplishthis we have to cut section as shown in the figure
D=D1+D2
D1= {(x,y), |0 ≤ x ≤ 1|, x < y <2 x}
D2={(x,y), |1 ≤ x ≤2|, 3 x−2< y <2 x}
∬
D
❑
8 x+36 y dA=∫
0
1
∫
x
2 x
8 x +36 y dydx +∫
1
2
∫
3 x−2
2 x
8 x +36 y dydx
¿∫
0
1
8 xy +36 y2
2 dx +∫
1
2
8 xy +36 y2
2 dx
¿∫
0
1
16 x2−8 x2+36 4 x2
2 −36 x2
2 dx +∫
1
2
16 x2−8 x ( 3 x−2 ) + 36 4 x2
2 −36 (3 x−2)2
2 dx
= ∫
0
1
6 2 x2 dx +¿∫
1
2
16 x2−24 x2 +16 x+36 4 x2
2 −36 (9 x2−12 x+ 4)
2 dx ¿
= ∫
0
1
62 x3
3 +∫
1
2
−98 x2+232 x−72 dx
= 62
3 +∫
1
2
−98 x3
3 + 232 x2
2 −72 x
= 62
3 +−784
3 + 928
2 −144+ 98
3 − 232
2 + 72 =-208+420
=212 cubic unit
(11)Evaluate the integral ∭ ydV , where V is the solid lying below the plane x+y+z = 8 and
above the region in the x−y plane bounded by the curves y = 1, x = 0 and x = √y.
Solution:
Region R in x-y plane

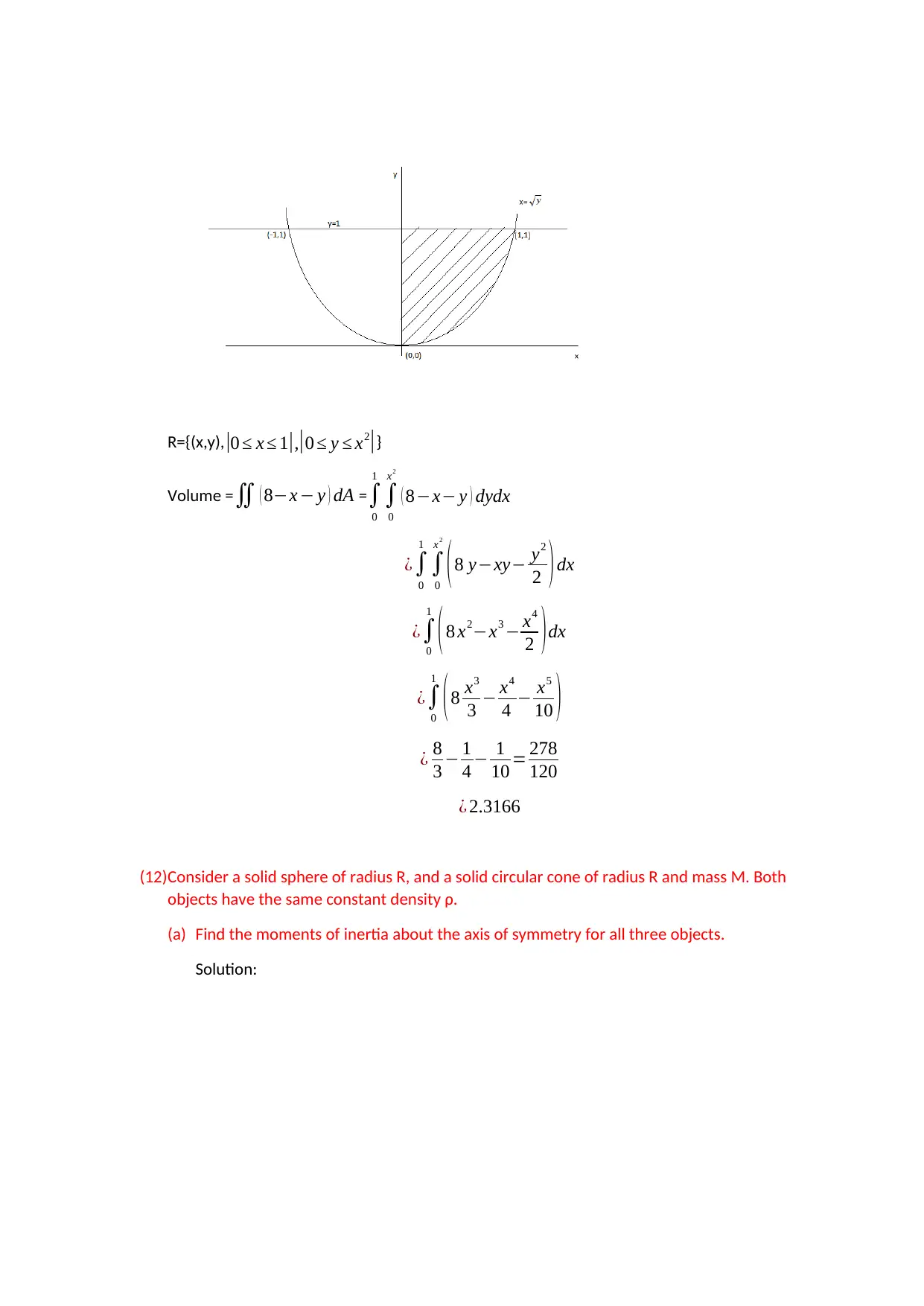
R={(x,y), |0 ≤ x ≤ 1|,|0 ≤ y ≤ x2
|}
Volume =∬ ( 8−x − y ) dA =∫
0
1
∫
0
x2
( 8−x− y ) dydx
¿∫
0
1
∫
0
x2
( 8 y−xy− y2
2 ) dx
¿∫
0
1
(8 x2−x3 − x4
2 )dx
¿∫
0
1
(8 x3
3 − x4
4 − x5
10 )
¿ 8
3 − 1
4 − 1
10 = 278
120
¿ 2.3166
(12)Consider a solid sphere of radius R, and a solid circular cone of radius R and mass M. Both
objects have the same constant density ρ.
(a) Find the moments of inertia about the axis of symmetry for all three objects.
Solution:

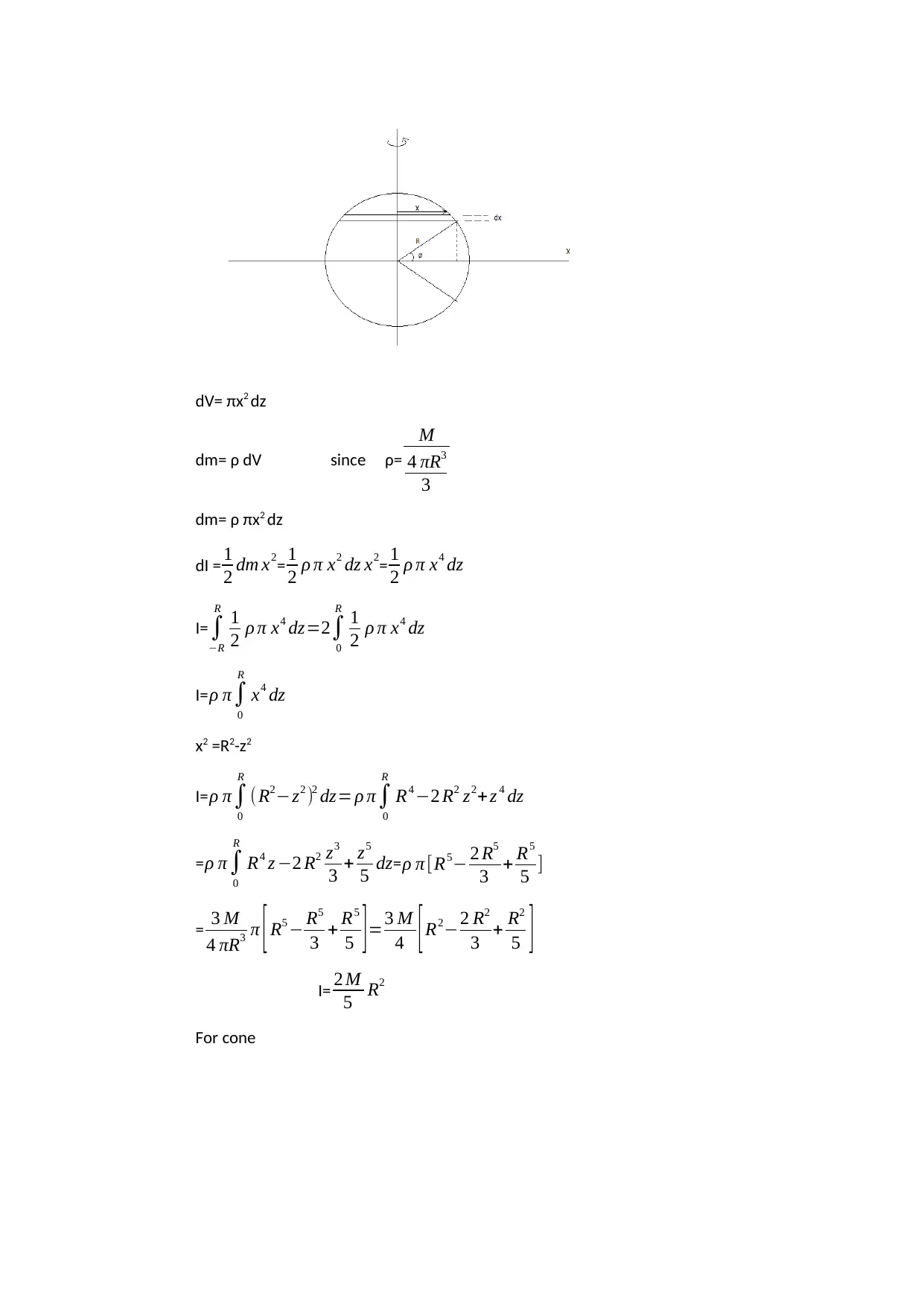
dV= πx2 dz
dm= ρ dV since ρ=
M
4 πR3
3
dm= ρ πx2 dz
dI = 1
2 dm x2= 1
2 ρ π x2 dz x2= 1
2 ρ π x4 dz
I= ∫
−R
R
1
2 ρ π x4 dz=2∫
0
R
1
2 ρ π x4 dz
I=ρ π∫
0
R
x4 dz
x2 =R2-z2
I=ρ π∫
0
R
( R2−z2 )2 dz= ρ π∫
0
R
R4 −2 R2 z2+ z 4 dz
=ρ π∫
0
R
R4 z −2 R2 z3
3 + z5
5 dz=ρ π [R5− 2 R5
3 + R5
5 ]
= 3 M
4 πR3 π [ R5 − R5
3 + R5
5 ] =3 M
4 [ R2−2 R2
3 + R2
5 ]
I= 2 M
5 R2
For cone

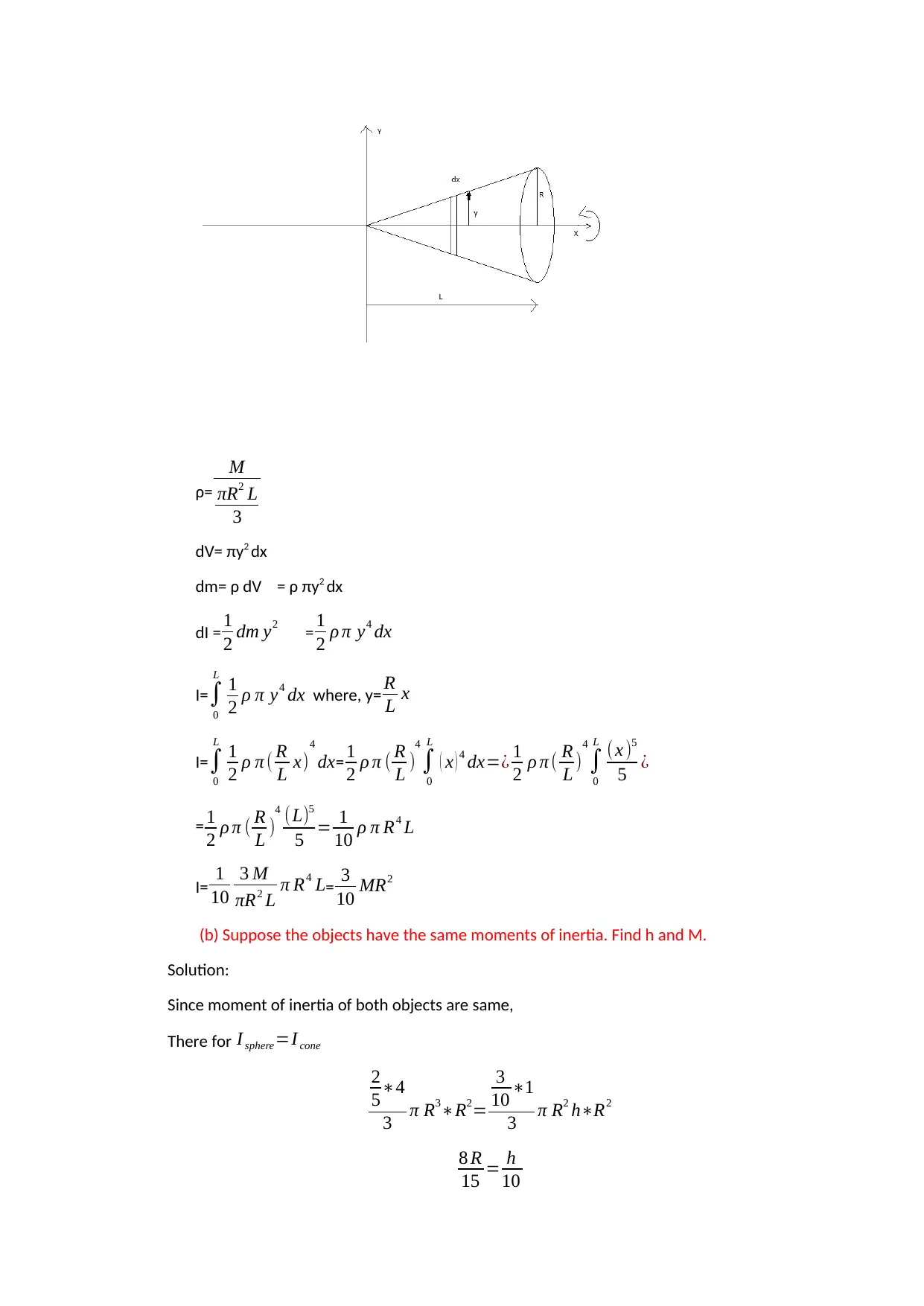
ρ=
M
πR2 L
3
dV= πy2 dx
dm= ρ dV = ρ πy2 dx
dI = 1
2 dm y2 = 1
2 ρ π y4 dx
I= ∫
0
L
1
2 ρ π y4 dx where, y= R
L x
I= ∫
0
L
1
2 ρ π ( R
L x)
4
dx= 1
2 ρ π ( R
L )
4
∫
0
L
( x ) 4 dx=¿ 1
2 ρ π ( R
L )
4
∫
0
L ( x )5
5 ¿
= 1
2 ρ π ( R
L )
4 (L)5
5 = 1
10 ρ π R4 L
I= 1
10
3 M
πR2 L π R4 L= 3
10 MR2
(b) Suppose the objects have the same moments of inertia. Find h and M.
Solution:
Since moment of inertia of both objects are same,
There for I sphere=I cone
2
5∗4
3 π R3∗R2=
3
10 ∗1
3 π R2 h∗R2
8 R
15 = h
10

h=16 R
3
(c) Given the assumptions in part (b), which is heavier, the cone or the sphere?
Solution: Sphere is heavier since it has high M.I
(13) Consider a torus whose equation in terms of spherical coordinates (r,θ,φ) is r = 2sinφ for 0 ≤
φ ≤ π and 0 ≤ θ ≤ 2π. Determine the mass of the region bounded by the torus if the density is
given by ρ = φ.
Solution: v= 2 π ∫2 √ (r2 ¿− ( x−R ) 2 )dx ¿
=4π ∫
R−r
r + R
2 √( r2− ( x−R )2 )dx
Pu u= x-R
=4π ∫
−r
r
(u+ R) √r2 −u2 du
=4π ∫
−r
r
u √r2 −u2 du+4π ∫
−r
r
R √r2 −u2 du
=0+4π ∫
−r
r
R √r2 −u2 du =4π R π r 2
2
v= 2 R π2 r2=2 R π2 (2 sin φ)2
ρ = φ= mass / volume
mass=density× volume =8 R π 2 (sin φ)2× φ
(14) Using spherical coordinates, evaluate the integral
∫
0
1
∫
− √1− y2
√1− y2
∫
√x2+ y2
√ 2− x2− y2
yzdzdxdy
Solution:
z= √ 2−x2− y2
z= √ x2+ y2
0 ≤ r ≤ √2
√ x2+ y2 =a=r cos ⏀
r cos ⏀=rsin⏀
tan⏀=1
=⏀ π
4

The limits are,
0≤x≤1
-√1− y2≤x≤√1− y2
π
2 ≤θ≤ 3 π
2
In spherical limits,
0≤ ≤⏀ π
4
π
2 ≤θ≤ 3 π
2
0≤r≤√ 2
∫
0
1
∫
− √1− y2
√ 1− y2
∫
√ x2+ y2
√ 2− x2− y2
yzdzdxdy=∫
0
π
4
∫
π
2
3 π
2
∫
0
√2
(rsin⏀ sinθrcos ⏀)∗rsin drdθd⏀ ⏀
=∫
0
π
4
∫
π
2
3 π
2
∫
0
√2
r3 sin2 ⏀cos ⏀ sinθdrdθd ⏀
¿∫
0
π
4
∫
π
2
3 π
2
r 4
4 sin2 ⏀ cos ⏀ sinθ dθd ⏀ , 0 , √2
¿∫
0
π
4
∫
π
2
3 π
2
sin2 ⏀cos ⏀ sinθdθd ⏀
¿−∫
0
π
4
sin2 ⏀ cos ⏀ cosθ , π
2 , 3 π
2 ,d ⏀
=−∫
0
π
4
sin2 ⏀ cos ⏀0 d ⏀
=0