Static Engineering System Assignment

32 Pages3416 Words392 Views

Added on 2019-12-18

Static Engineering System Assignment

Added on 2019-12-18

BookmarkRelated Documents

STATICENGINEERINGSYSTEM

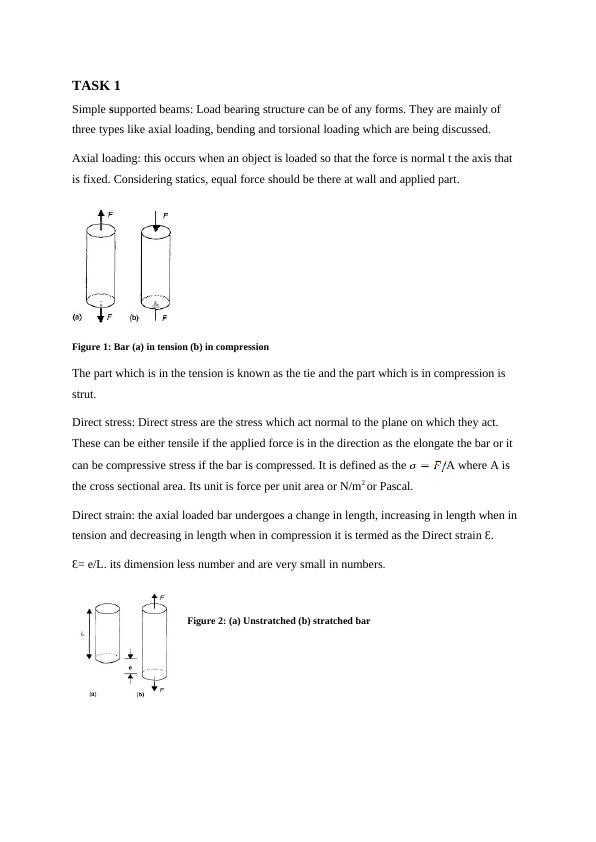
TASK 1Simple supported beams: Load bearing structure can be of any forms. They are mainly of three types like axial loading, bending and torsional loading which are being discussed. Axial loading: this occurs when an object is loaded so that the force is normal t the axis that is fixed. Considering statics, equal force should be there at wall and applied part. Figure 1: Bar (a) in tension (b) in compressionThe part which is in the tension is known as the tie and the part which is in compression is strut.Direct stress: Direct stress are the stress which act normal to the plane on which they act. These can be either tensile if the applied force is in the direction as the elongate the bar or it can be compressive stress if the bar is compressed. It is defined as the A where A is the cross sectional area. Its unit is force per unit area or N/m2 or Pascal. Direct strain: the axial loaded bar undergoes a change in length, increasing in length when in tension and decreasing in length when in compression it is termed as the Direct strain Ɛ.Ɛ= e/L. its dimension less number and are very small in numbers. Figure 2: (a) Unstratched (b) stratched bar

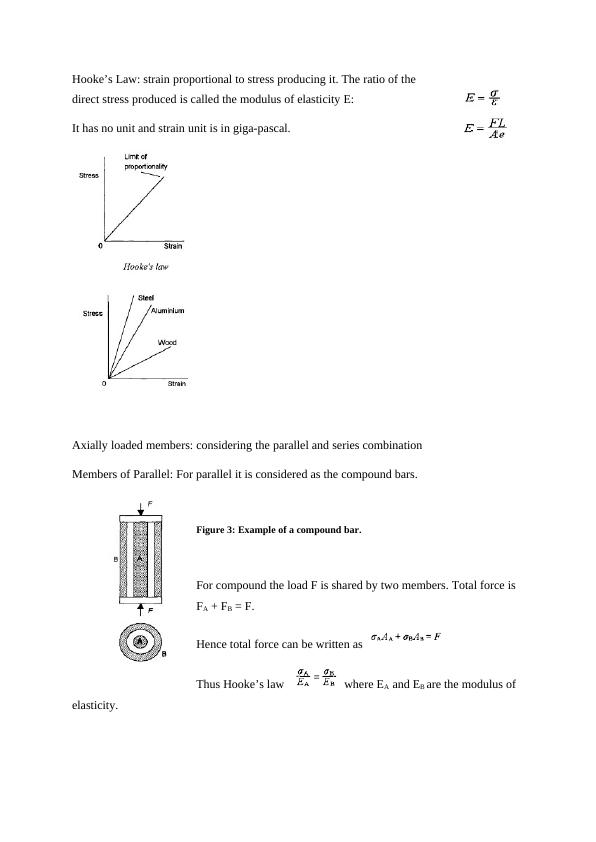
Hooke’s Law: strain proportional to stress producing it. The ratio of thedirect stress produced is called the modulus of elasticity E:It has no unit and strain unit is in giga-pascal. Axially loaded members: considering the parallel and series combinationMembers of Parallel: For parallel it is considered as the compound bars. Figure 3: Example of a compound bar.For compound the load F is shared by two members. Total force is FA + FB = F. Hence total force can be written as Thus Hooke’s law where EA and EB are the modulus of elasticity.

Members of Series: suppose two materials of different material and cross-section. It will be inEquilibrium condition and the force of stretching the A and B are same to C. Figure 4: Members of series.Poisson’s ratio: when material is longitudinal stretched it contracts in a transverse direction. The ration of the transverse strain to the longitudinal strain is called Poisson’s ratio.Figure 5 transverse contraction as a result of longitudinal stretchingthe negative sign as one strains is tensile and other is compressive. For most engineering metal its value is 0.3. Types of columns: Depending on the mode of failure, columns can be categorised in the following ways(a) a short column: a column which will fail in true compression(b) a long column: a column which buckles before full compressive strength is reachedTypes of beams: Beams can be classified according to the manner in which they supported

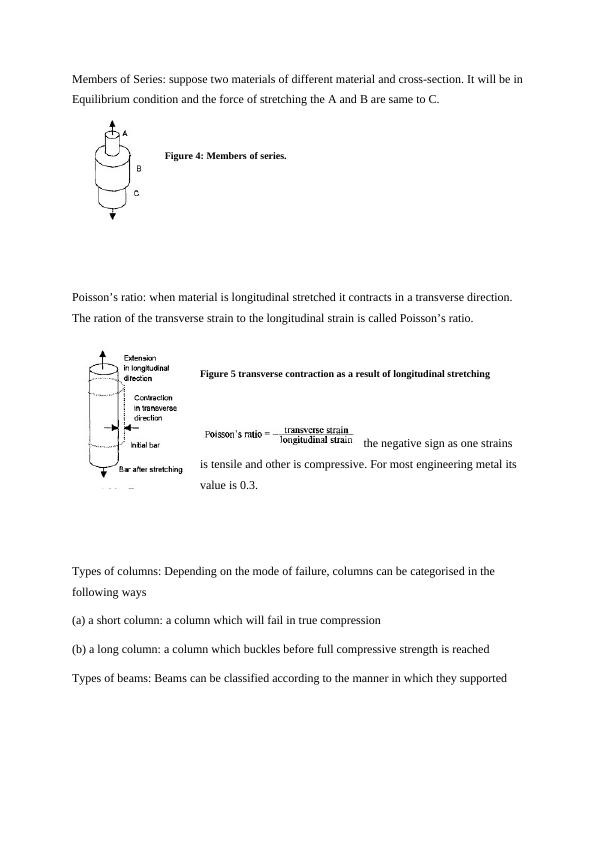
Figure 6: Various types of BeamsBeams used in building and bridges-Girders: Usually the most important beams, which are frequently ag wide spacing-Joists: Usually less important beams, which are closely spaced, frequently with truss-type webs. -Stringers: Longitudinal bridge beams spanning between floor beams. -Purlins: Roof beams spanning between trusses. -Girts: Horizontal wall beams to wind on the side of and industrial building-Lintels: Members supporting a wall over window or door openings. Loads: Loads can be applied on the beans in one of the following. Figure 7: types of load

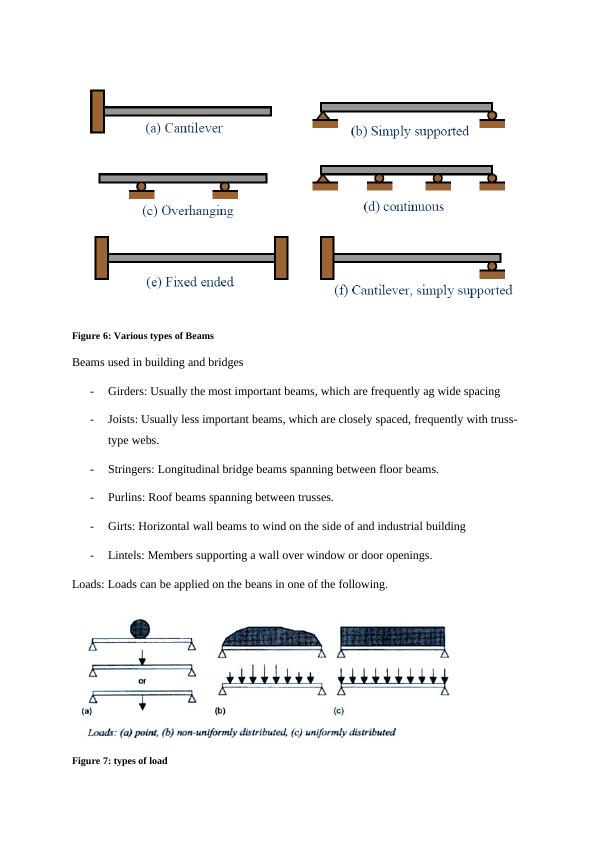
Sheer force and bending moments: •When a beam is loaded by forces or couples, internalstresses and strains are created. Consider a cantileverarrangement•It is convenient to reduce the resultant to a shear force, V,and a bending moment, M. Sign Convention:•Positive shear forces always deform right hand face downward with respect to the left hand face. Positive shear stress acts clockwise while negative shear stress acts counter-clockwise•Positive bending moments always elongate the lower section of the beam. Positive moment compresses upper (sagging moments) whereas negative moment compresses lower (hogging moments)Relationships for continuous loads: Consider the following beam segment with a uniformly distributed load with load intensity q. Note that distributed loads are positive when acting downward and negative when acting upward.•Summing forces vertically•Summing moments and discarding products of differentials because they are negligible compared to other terms

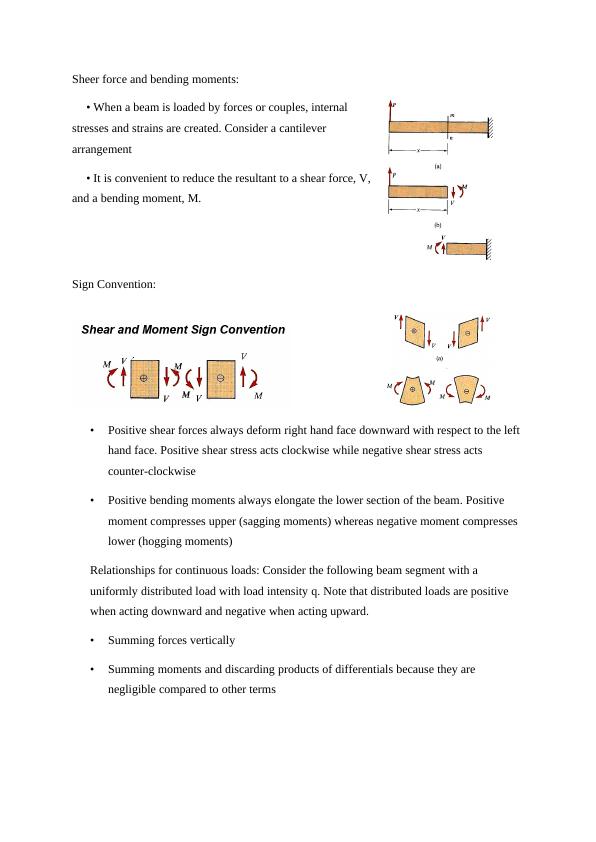
•Relationships for concentrated loads: consider the following beam segment with a concentrated load, P. Again, concentrated loads are positive when acting downward and negative when acting upward. •Summing forces vertically•An abrupt change occurs in the shear force at a point where a concentrated load acts.As one moves from left to right through a point of load application, the shear forcedecreases by an amount equal to the magnitude of the downward load. •Summing the moments

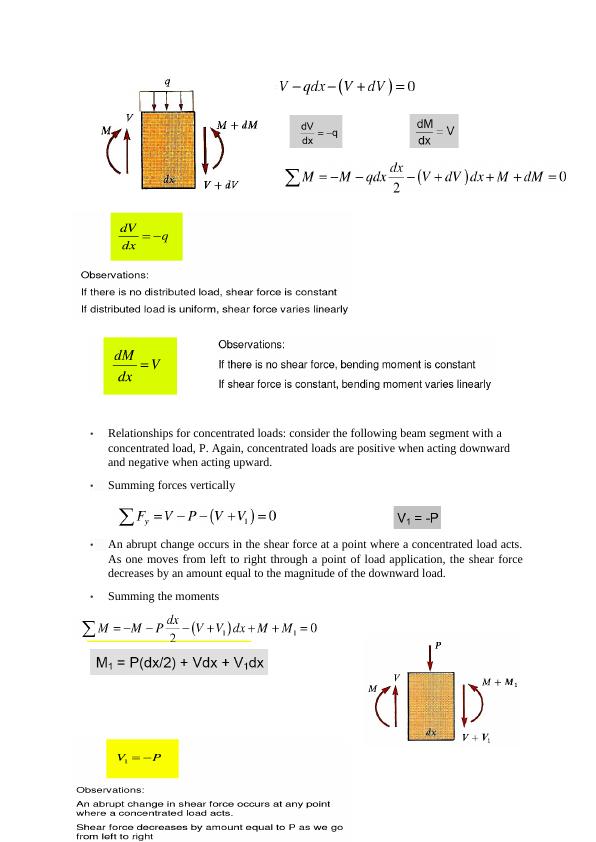
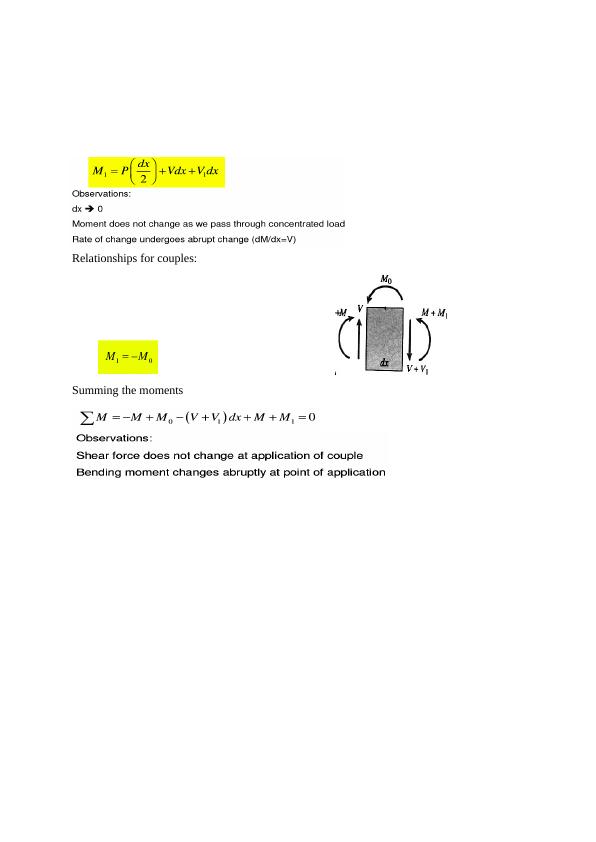
Relationships for couples: Summing the moments

End of preview

Want to access all the pages? Upload your documents or become a member.

Fracture Mechanics 1

|1662

|137

Design Continuous Simply Supported Prestressed Concreate Beam

|567

|299

Mechanical Properties of Materials and Testing Methods

|16

|2522

|323

Three Point Bending and Shaft Design

|17

|2029

|41

Static Engineering System Assignment