Designing a Statically Determinate Frame for Solids and Structures 1 Coursework

This coursework focuses on designing a statically determinate frame for Solids and Structures 1 course. It covers the concepts of determinacy, indeterminacy, and stability. It explains how to identify determinate, indeterminate, and stable structures. It also provides insights on selecting the most appropriate member type for an economical design.

Running head: COURSEWORK ASSIGNMENT 1
Solids and Structures 1
First Name MI Last Name
Queen’s University, Belfast

Running head: COURSEWORK ASSIGNMENT 2
A structure can either be defined as determinate or indeterminate based on the condition of
loading in a structure. It gives significant bearing on stability of such a structure as well. In
others, equilibrium equations and free- body diagrams can be used to determine all the internal
forces and reactions while in some this is hardly the case. Specifically, a statically determinate
structure is that kind of a structure whereby determinations of internal reactions and member
forces is solely achieved or derived from the three equations of equilibrium and from the free
body diagram.
This assignment focuses to design a statically determinate frame based on conditions namely:-
I. The frame should be able to support a vertical load of 8kN and a horizontal load of 5kN
in either direction
II. A number of individual member types are available
III. Frame should be statically determinate.
a) Selected Frame layout.
The selected frame layout is to be as shown:
The fixed end is designated A and intermediate one B.
b) Check that the proposed layout is statically determinate;
To check for determinacy; total number of unknown= total number of available
equations at joins hence the equation below is used-
r =3 n
Where,
j= number of joints in the entire structure.
i= total number of links in the entire structure.

Running head: COURSEWORK ASSIGNMENT 3
r= minimum number of reaction requisite for external stability/ determinacy.
When stability is to be entailed, the equation i+r=2 jwould be applied but we are to
check on determinacy alone. A structure is stable and internally determinate when
i+r=2 j while if i+r >2 jthe structure is defined to be stable and internally
determinate. In cases where i+r <2 jthe structure is known to be unstable.
From the selected frame;
Total number of joints j=3
Total number of links i=1
Minimum number of reaction requisite for external stability/ determinacy r=3
By substitution therefore in equation r=3n, 3= 3(1) hence the structure is statically
determinate.
c) Analysis to identify the force on each of the members, noting whether they are in
tension or compression.
d) Method of joints
Part c and D
External reactions:
summation of Fx =0
= Ax+5=0 , → Ax=−5 kN
−summation of F y=0
= A y+ By−¿8−¿8=0, → A y=By=0
summation of moments about A M A =0 ,
=(−8 × 3 )− ( 6 ×5 )+ ( B y × 3 )=0
→ B y=−2 kN
Joint A
Summation Fx=0 , AB=−5 kN ( Compression )

Running head: COURSEWORK ASSIGNMENT 4
summation F y=0 ,− Ay −10→ Ay =−10 kN
joint B
Summation Fx=0 , AB=0
summation F y=0 , By=−4 kN (compresssion)
e) Select ion of the most appropriate member type noting that In order to produce an
economical design the member type should as low a cost as possible.
The most an appropriate member should therefore have a compression capacity of not
less than 10 KN and length greater than 3m. Member type III would thereby be
appropriate and economically viable for the design.
Notes
Prior to starting to dissect a structure, it is critical to comprehend what sort of
structure it is. Diverse kinds of structures may should be dissected utilizing distinctive
strategies. For instance, structures that are determinate might be totally dissected
utilizing just static harmony, though uncertain structures require the utilization of
both static balance and similarity connections to locate the interior powers. Likewise,
genuine structures must be steady. This implies the structure can recoup static balance
after an unsettling influence. There is no point dissecting a structure that isn't steady.
This area will clarify the ideas of determinacy, indeterminacy and steadiness and
demonstrate to distinguish determinate, uncertain and stable structures.
Vital Terms
Stable/Unstable
A steady structure is one that won't crumple when aggravated. Steadiness may
likewise be characterized as "The ability to recoup equilibrium."[1] when all is said in
done, there are many ways that a structure may wind up temperamental, including
clasping of pressure individuals, yielding/burst of individuals, or nonlinear geometric
impacts like P-Delta; in any case, for straight basic examination, the fundamental

Running head: COURSEWORK ASSIGNMENT 5
concern is shakiness caused by deficient response focuses or poor format of basic
individuals.
Inside Stable
In inside stable structure is one that would keep up its shape if every one of the
responses bolsters were evacuated. A structure that is inside precarious may even now
be steady on the off chance that it has adequate outside help responses. A precedent is
appeared in Figure 1
Outer Determinacy
The capacity to compute the majority of the outer response segment powers utilizing
just static equilibrium. A structure that fulfills this necessity is remotely statically
determinate. A structure for which the outside responses segment powers can't be
computed utilizing just harmony is remotely statically indeterminacy.
Inward Determinacy
The capacity to ascertain the majority of the outside response part powers and inward
powers utilizing just static equilibrium. A structure that fulfills this necessity is inside
statically determinate. A structure for which the inner powers can't be ascertained
utilizing just harmony is inside statically vague. Ordinarily in the event that one
discusses 'determinacy', it is inward determinacy that is implied.
Repetitive

Running head: COURSEWORK ASSIGNMENT 6
Uncertain structures adequately have a larger number of questions than can be
fathomed utilizing the three harmony conditions (or six balance conditions in 3D).
The additional questions are called redundant.
Level of Indeterminacy
The level of indeterminacy is equivalent to the quantity of redundant. An uncertain
structure with 2 redundant might be said to be statically vague to the second degree or
"2º S.I."
On the off chance that a structure is remotely determinate, at that point the majority of
the responses might be ascertained utilizing harmony alone. To ascertain outside
determinacy, the accompanying conditions are utilized;
Where r is the quantity of response parts, and ec is the quantity of conditions of
condition. Both of these are depicted in detail beneath.
The level of indeterminacy is given by the accompanying equation:
i.e. =r− (3+ec) ~Eq. 4

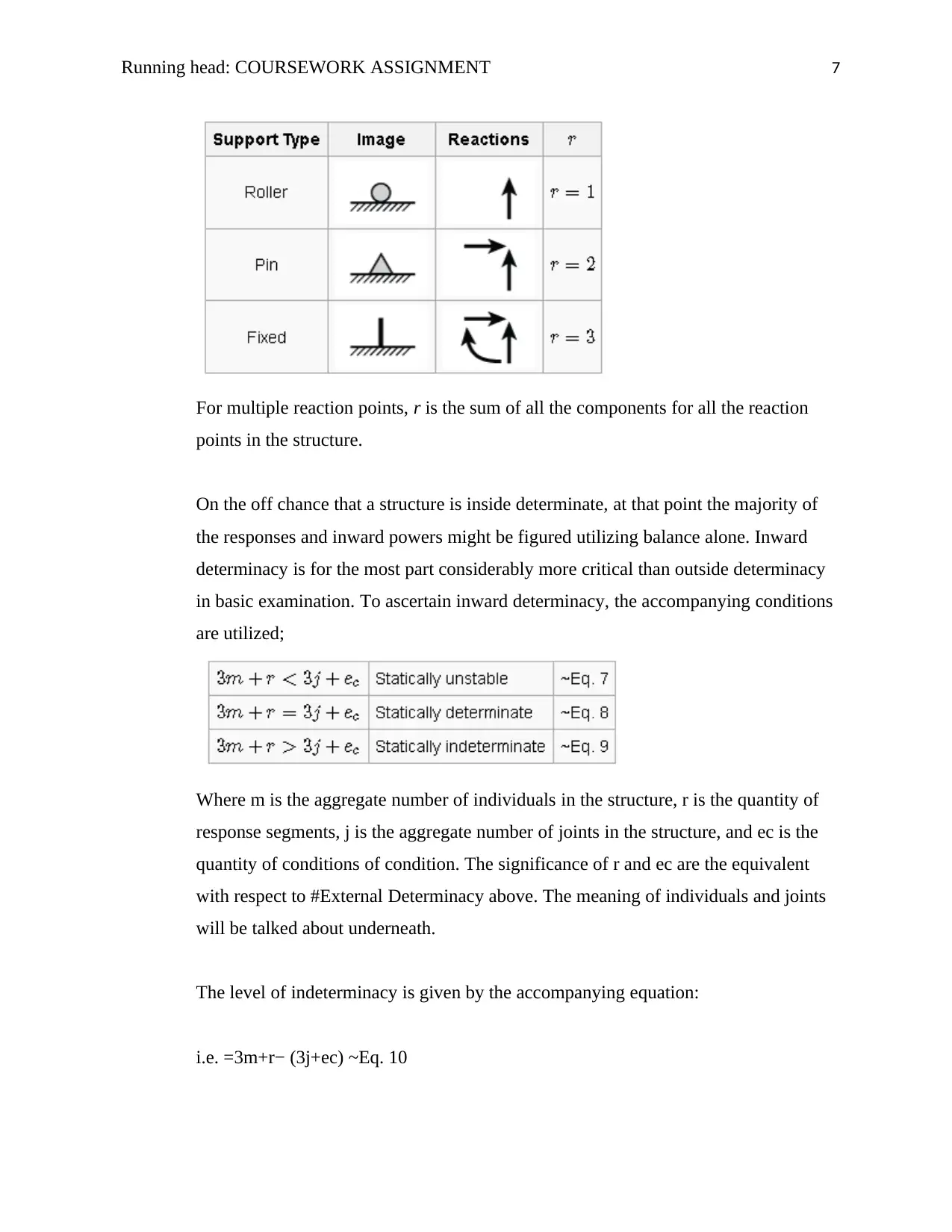
Running head: COURSEWORK ASSIGNMENT 7
For multiple reaction points, r is the sum of all the components for all the reaction
points in the structure.
On the off chance that a structure is inside determinate, at that point the majority of
the responses and inward powers might be figured utilizing balance alone. Inward
determinacy is for the most part considerably more critical than outside determinacy
in basic examination. To ascertain inward determinacy, the accompanying conditions
are utilized;
Where m is the aggregate number of individuals in the structure, r is the quantity of
response segments, j is the aggregate number of joints in the structure, and ec is the
quantity of conditions of condition. The significance of r and ec are the equivalent
with respect to #External Determinacy above. The meaning of individuals and joints
will be talked about underneath.
The level of indeterminacy is given by the accompanying equation:
i.e. =3m+r− (3j+ec) ~Eq. 10

Running head: COURSEWORK ASSIGNMENT 8
Individuals and Joints
Determination of the Number of Members and Joints
There is no particular way that a structure must be part into individuals and joints for
the motivations behind the determinacy examination. Any division of the structure is
alright as long as the individuals and joints are reliable with one another; be that as it
may, joints ought to be set at any rate at the accompanying areas:
Free closures
Responses
Crossing points of at least three components
Strength
A precarious structure for the most part can't be dissected. In this way, it is valuable
to know whether a structure is steady or precarious before a basic examination is led.
There are four principle ways that a structure might be geometrically insecure. These
apply just too straight geometric security and not to shakiness caused by clasping,
part yielding or nonlinear geometry.
There are insufficient reactions: This will for the most part be obvious from a use of
the determinacy conditions
The responses are parallel: All of the response segments point a similar way. In this
precedent, the level balance ∑Fx=0 can't be settled and there will be a net flat power
on the framework with no obstruction.
The responses are concurrent: All of the response parts meet at a point. A case of
such a circumstance is appeared in Figure 6. Successfully, the framework is allowed
to turn as an inflexible body around the point that the response parts meet at.
There is an inner crumple mechanism: This is any circumstance in which there is an
inside component in the framework that will make it twist between the backings. In
some such circumstances, this will be obvious from the utilization of the determinacy
conditions, yet in others, it may not. In every single such case, however, the

Running head: COURSEWORK ASSIGNMENT 9
flimsiness will turn out to be clear amid the auxiliary examination since it will be
difficult to explain for the majority of the inward powers. A precedent inward
crumple instrument

Running head: COURSEWORK ASSIGNMENT 10
References
Khalfallah, S. (2018, July 10). Structural Analysis 1: Statically Determinate Structures. Retrieved
November 2, 2018, from https://www.wiley.com/en-ad/Structural Analysis 1: Statically
Determinate Structures-p-9781786303387
Theory of Structures. (n.d.). Retrieved November 2, 2018, from
http://ocw.ump.edu.my/course/view.php?id=13