Statistical Analysis of Weight, Test Scores, Training Methods, and Road Traffic Data
VerifiedAdded on 2023/06/09
|19
|3035
|215
AI Summary
This report presents statistical analysis of weight, test scores, training methods, and road traffic data. The analysis includes hypotheses testing, computation of test statistics, determination of appropriate probability for committing type I error, and interpretation of results.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

Student Name
Course Name
Institution Affiliation
Course Name
Institution Affiliation
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
Question One
a. Stating the hypotheses to determine if there is a significant difference between the
weights of the contestants before and after the first week of the programme.
The hypotheses are
H0 : μ0=μ1
H1=μ0 ≠ μ1
b. Computation of test statistic to test the hypotheses
Here the t statistic (paired test) will be applied
t= ∑ D
√ n ∑ D2− ( ∑ D )
2
n−1
w h ere t h e D=difference between X∧Y , n=sample ¿ 12
In this case, the degree of freedom will be given by n−1=12−1=11
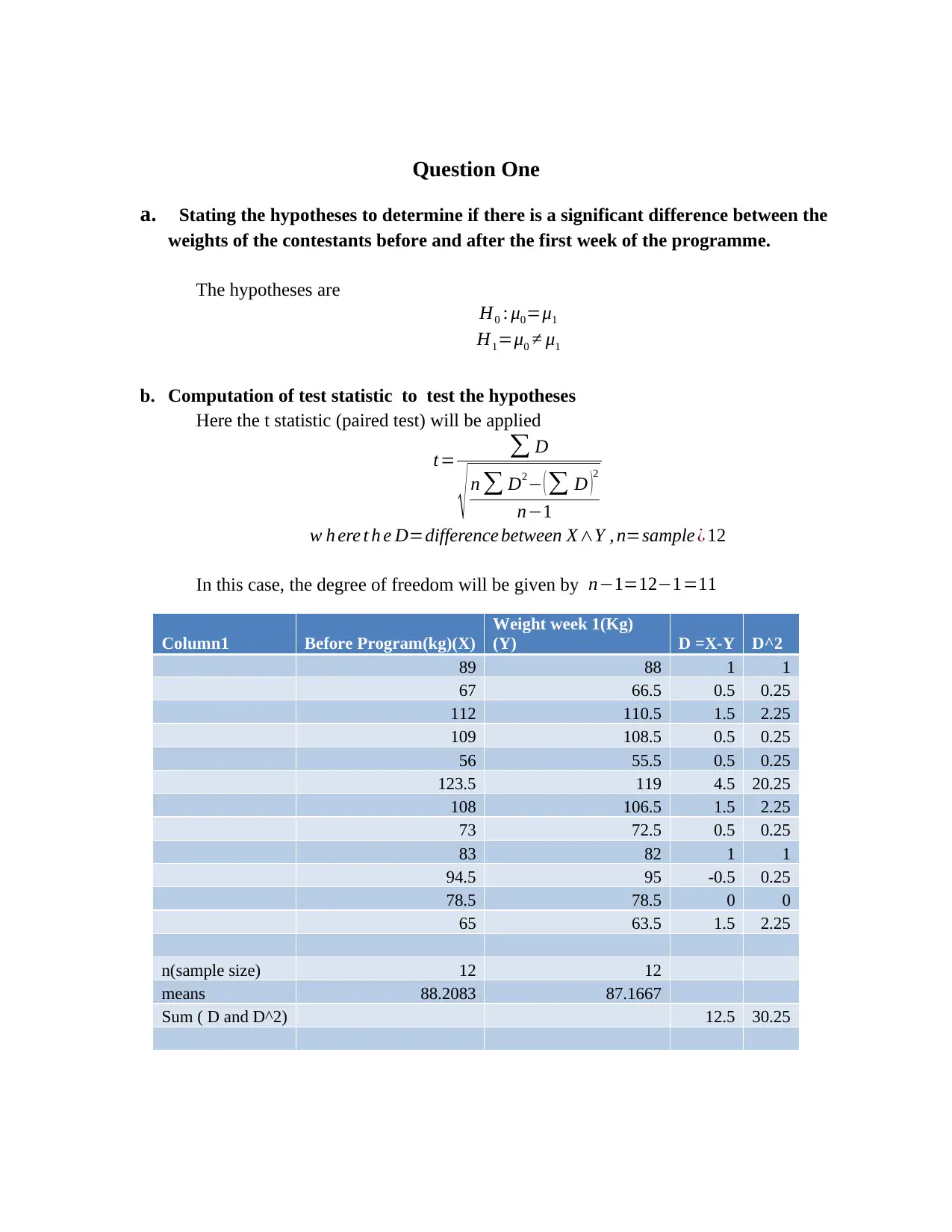
Column1 Before Program(kg)(X)
Weight week 1(Kg)
(Y) D =X-Y D^2
89 88 1 1
67 66.5 0.5 0.25
112 110.5 1.5 2.25
109 108.5 0.5 0.25
56 55.5 0.5 0.25
123.5 119 4.5 20.25
108 106.5 1.5 2.25
73 72.5 0.5 0.25
83 82 1 1
94.5 95 -0.5 0.25
78.5 78.5 0 0
65 63.5 1.5 2.25
n(sample size) 12 12
means 88.2083 87.1667
Sum ( D and D^2) 12.5 30.25
a. Stating the hypotheses to determine if there is a significant difference between the
weights of the contestants before and after the first week of the programme.
The hypotheses are
H0 : μ0=μ1
H1=μ0 ≠ μ1
b. Computation of test statistic to test the hypotheses
Here the t statistic (paired test) will be applied
t= ∑ D
√ n ∑ D2− ( ∑ D )
2
n−1
w h ere t h e D=difference between X∧Y , n=sample ¿ 12
In this case, the degree of freedom will be given by n−1=12−1=11
Column1 Before Program(kg)(X)
Weight week 1(Kg)
(Y) D =X-Y D^2
89 88 1 1
67 66.5 0.5 0.25
112 110.5 1.5 2.25
109 108.5 0.5 0.25
56 55.5 0.5 0.25
123.5 119 4.5 20.25
108 106.5 1.5 2.25
73 72.5 0.5 0.25
83 82 1 1
94.5 95 -0.5 0.25
78.5 78.5 0 0
65 63.5 1.5 2.25
n(sample size) 12 12
means 88.2083 87.1667
Sum ( D and D^2) 12.5 30.25

Therefore,
t= 12.5
√ 12 ( 30.5 )−12.52
12−1
= 12.5
√ 363−156.25
11
¿ 12.5
√ 206.75
11
= 12.5
4.3354
¿ 2.8833
Hence, tcomputed=2.8833
c. Specification and justification of an appropriate probability for committing
Type I error (α)
The probability of committing type I error will be 5%, which is the probability of
incorrectly rejecting the null hypothesis. This will be attained at the significance level
of 95%, which is the probability of rejecting or accepting the null hypothesis
accurately.
To make the decision, in this case, the critical value of need to determine from
t-tables
t0.05 ( 11 df ) ( twotailed ) =1.796
d. Reporting of the decision and clearly explain your result.
The tcomputed=2.8833 ¿ tα =1.796 , therefore, null hypothesis, H0 t h e t h e t h e : μ0=μ1
is rejected. This suggest that there is significant difference between the weights
of the contestants before and after the first week of the programme .
t= 12.5
√ 12 ( 30.5 )−12.52
12−1
= 12.5
√ 363−156.25
11
¿ 12.5
√ 206.75
11
= 12.5
4.3354
¿ 2.8833
Hence, tcomputed=2.8833
c. Specification and justification of an appropriate probability for committing
Type I error (α)
The probability of committing type I error will be 5%, which is the probability of
incorrectly rejecting the null hypothesis. This will be attained at the significance level
of 95%, which is the probability of rejecting or accepting the null hypothesis
accurately.
To make the decision, in this case, the critical value of need to determine from
t-tables
t0.05 ( 11 df ) ( twotailed ) =1.796
d. Reporting of the decision and clearly explain your result.
The tcomputed=2.8833 ¿ tα =1.796 , therefore, null hypothesis, H0 t h e t h e t h e : μ0=μ1
is rejected. This suggest that there is significant difference between the weights
of the contestants before and after the first week of the programme .

In this case, alternative hypothesis H1=μ0 ≠ μ1 is accepted. In conclusion the
weight of the contestant is not the same before and after the programme, the t-
test conducted reveals this.
Question Two
a. Stating the hypotheses determine if there is a significant difference between the test
scores of both classes
The hypotheses to be determined are
H0 : μ0=μ1
H1=μ0 ≠ μ1
b. Calculation of test statistic to test the hypotheses
Due to an unequal sample size of the two variables, t-statistics (unpaired test), equal
variance, will be conducted.
t= ( X −Y )
√ ( sx
2
n1
+ sy
2
n2 )
where , sx
2∧s y
2 are the variance of X ∧Y respectively , X∧Y are the means of X
Y are the means of X∧Y respectively , n1∧n2 are the sample ¿ X a nd Y
weight of the contestant is not the same before and after the programme, the t-
test conducted reveals this.
Question Two
a. Stating the hypotheses determine if there is a significant difference between the test
scores of both classes
The hypotheses to be determined are
H0 : μ0=μ1
H1=μ0 ≠ μ1
b. Calculation of test statistic to test the hypotheses
Due to an unequal sample size of the two variables, t-statistics (unpaired test), equal
variance, will be conducted.
t= ( X −Y )
√ ( sx
2
n1
+ sy
2
n2 )
where , sx
2∧s y
2 are the variance of X ∧Y respectively , X∧Y are the means of X
Y are the means of X∧Y respectively , n1∧n2 are the sample ¿ X a nd Y
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
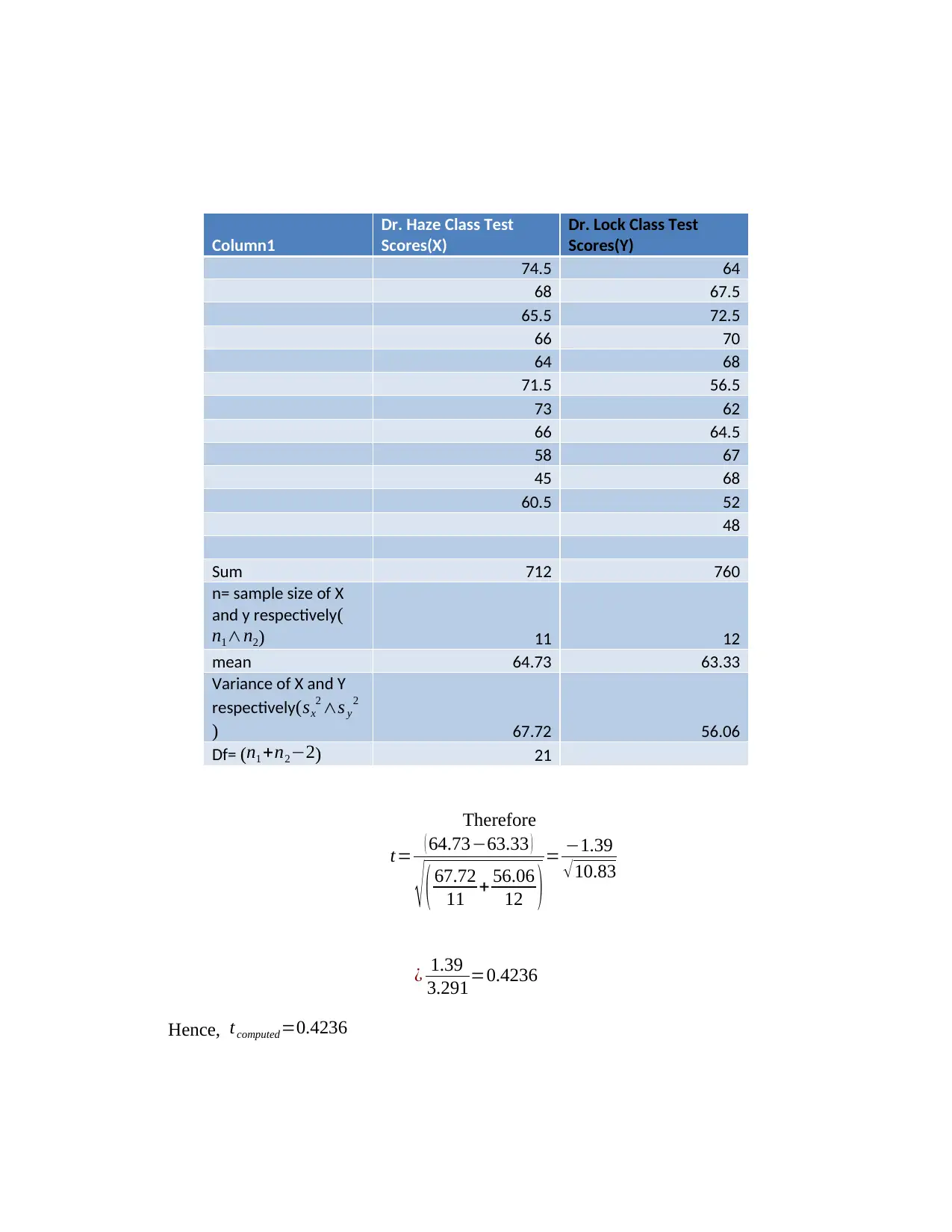
Column1
Dr. Haze Class Test
Scores(X)
Dr. Lock Class Test
Scores(Y)
74.5 64
68 67.5
65.5 72.5
66 70
64 68
71.5 56.5
73 62
66 64.5
58 67
45 68
60.5 52
48
Sum 712 760
n= sample size of X
and y respectively(
n1∧n2) 11 12
mean 64.73 63.33
Variance of X and Y
respectively( sx
2 ∧s y
2
) 67.72 56.06
Df= (n1 +n2−2) 21
Therefore
t= ( 64.73−63.33 )
√ ( 67.72
11 + 56.06
12 )= −1.39
√10.83
¿ 1.39
3.291=0.4236
Hence, tcomputed=0.4236
Dr. Haze Class Test
Scores(X)
Dr. Lock Class Test
Scores(Y)
74.5 64
68 67.5
65.5 72.5
66 70
64 68
71.5 56.5
73 62
66 64.5
58 67
45 68
60.5 52
48
Sum 712 760
n= sample size of X
and y respectively(
n1∧n2) 11 12
mean 64.73 63.33
Variance of X and Y
respectively( sx
2 ∧s y
2
) 67.72 56.06
Df= (n1 +n2−2) 21
Therefore
t= ( 64.73−63.33 )
√ ( 67.72
11 + 56.06
12 )= −1.39
√10.83
¿ 1.39
3.291=0.4236
Hence, tcomputed=0.4236

c. Specifying and justifying an appropriate probability of committing a
Type I error (α).
To make the decision critical value of t at 95% significance level from t-table is needed.
This will set the probability of incorrectly rejecting the null hypothesis at 5%, which is
the chance of committing type I error. This is a reasonable level at which the end results
of the statistical test are reasonable.
For the above case, the critical value of t at 95% significance level will be,
t0.05 (21 df ) ( twotailed )=1.721
d. Decision and clear explanation of the result
Since, tcomputed=0.4236 < critical value 1.721, null hypothesis H0 is accepted. This
indicates that there is no significant difference between the test scores of both classes, Dr
Haze Class Scores and Dr Lock Class Scores. This suggest that the student taking the
same statistical test from the two classes are likely to get similar results, the class
does not matter as revealed by the t-s statistic test results.
Type I error (α).
To make the decision critical value of t at 95% significance level from t-table is needed.
This will set the probability of incorrectly rejecting the null hypothesis at 5%, which is
the chance of committing type I error. This is a reasonable level at which the end results
of the statistical test are reasonable.
For the above case, the critical value of t at 95% significance level will be,
t0.05 (21 df ) ( twotailed )=1.721
d. Decision and clear explanation of the result
Since, tcomputed=0.4236 < critical value 1.721, null hypothesis H0 is accepted. This
indicates that there is no significant difference between the test scores of both classes, Dr
Haze Class Scores and Dr Lock Class Scores. This suggest that the student taking the
same statistical test from the two classes are likely to get similar results, the class
does not matter as revealed by the t-s statistic test results.

Question Three
a. Stating the hypotheses to determine if there is a significant difference between
the different methods of training
The hypotheses will be,
H0 : μ0=μ1 =μ2
H0 : μ0 ≠ μ1∨μ0 ≠ μ2∨μ1=μ2
The concern will be whether there are differences among the means of three methods of
training.
b. Calculation of test statistic to test the hypotheses
For the case above, one-way ANOVA is required, where F-statistic will be determined,
because the sample has more than two groups, has three. This involves comparison of
the between the groups and within the groups.
F=
SSb
Df b
SSW
Df W
= Mean Squareb etween
Mean Squarewithin
Within-group Sum of Square ( SSW )
SSW =∑
j=1
p
∑
i=1
n
( Xi , j −X j ) 2
where , Xi , j are theobservations ∈groups∧X j average of group J
a. Stating the hypotheses to determine if there is a significant difference between
the different methods of training
The hypotheses will be,
H0 : μ0=μ1 =μ2
H0 : μ0 ≠ μ1∨μ0 ≠ μ2∨μ1=μ2
The concern will be whether there are differences among the means of three methods of
training.
b. Calculation of test statistic to test the hypotheses
For the case above, one-way ANOVA is required, where F-statistic will be determined,
because the sample has more than two groups, has three. This involves comparison of
the between the groups and within the groups.
F=
SSb
Df b
SSW
Df W
= Mean Squareb etween
Mean Squarewithin
Within-group Sum of Square ( SSW )
SSW =∑
j=1
p
∑
i=1
n
( Xi , j −X j ) 2
where , Xi , j are theobservations ∈groups∧X j average of group J
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
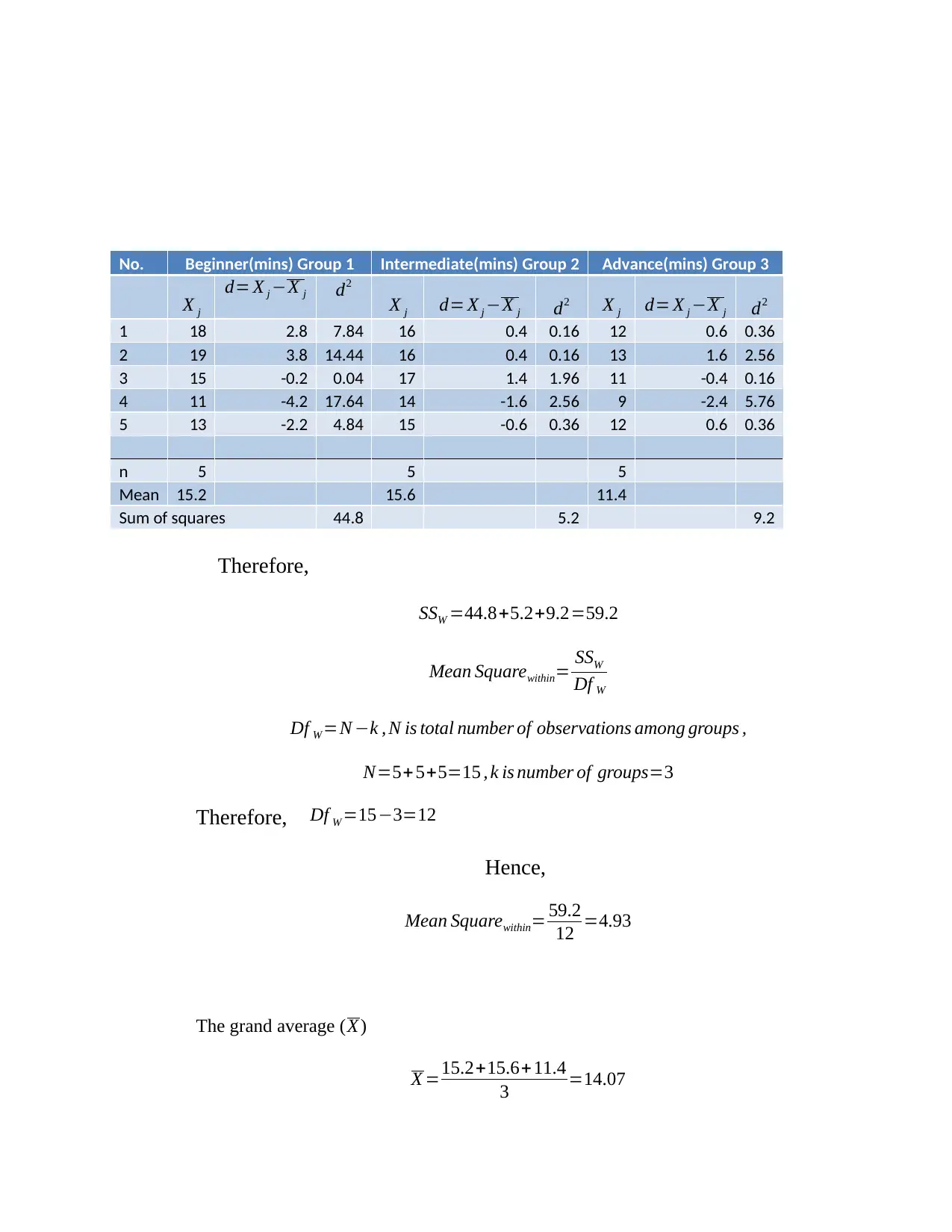
No. Beginner(mins) Group 1 Intermediate(mins) Group 2 Advance(mins) Group 3
X j
d= X j −X j d2
X j d= X j −X j d2 X j d= X j −X j d2
1 18 2.8 7.84 16 0.4 0.16 12 0.6 0.36
2 19 3.8 14.44 16 0.4 0.16 13 1.6 2.56
3 15 -0.2 0.04 17 1.4 1.96 11 -0.4 0.16
4 11 -4.2 17.64 14 -1.6 2.56 9 -2.4 5.76
5 13 -2.2 4.84 15 -0.6 0.36 12 0.6 0.36
n 5 5 5
Mean 15.2 15.6 11.4
Sum of squares 44.8 5.2 9.2
Therefore,
SSW =44.8+5.2+9.2=59.2
Mean Squarewithin= SSW
Df W
Df W =N −k , N is total number of observations among groups ,
N=5+ 5+5=15 , k is number of groups=3
Therefore, Df W =15−3=12
Hence,
Mean Squarewithin= 59.2
12 =4.93
The grand average ( X )
X =15.2+15.6+ 11.4
3 =14.07
X j
d= X j −X j d2
X j d= X j −X j d2 X j d= X j −X j d2
1 18 2.8 7.84 16 0.4 0.16 12 0.6 0.36
2 19 3.8 14.44 16 0.4 0.16 13 1.6 2.56
3 15 -0.2 0.04 17 1.4 1.96 11 -0.4 0.16
4 11 -4.2 17.64 14 -1.6 2.56 9 -2.4 5.76
5 13 -2.2 4.84 15 -0.6 0.36 12 0.6 0.36
n 5 5 5
Mean 15.2 15.6 11.4
Sum of squares 44.8 5.2 9.2
Therefore,
SSW =44.8+5.2+9.2=59.2
Mean Squarewithin= SSW
Df W
Df W =N −k , N is total number of observations among groups ,
N=5+ 5+5=15 , k is number of groups=3
Therefore, Df W =15−3=12
Hence,
Mean Squarewithin= 59.2
12 =4.93
The grand average ( X )
X =15.2+15.6+ 11.4
3 =14.07
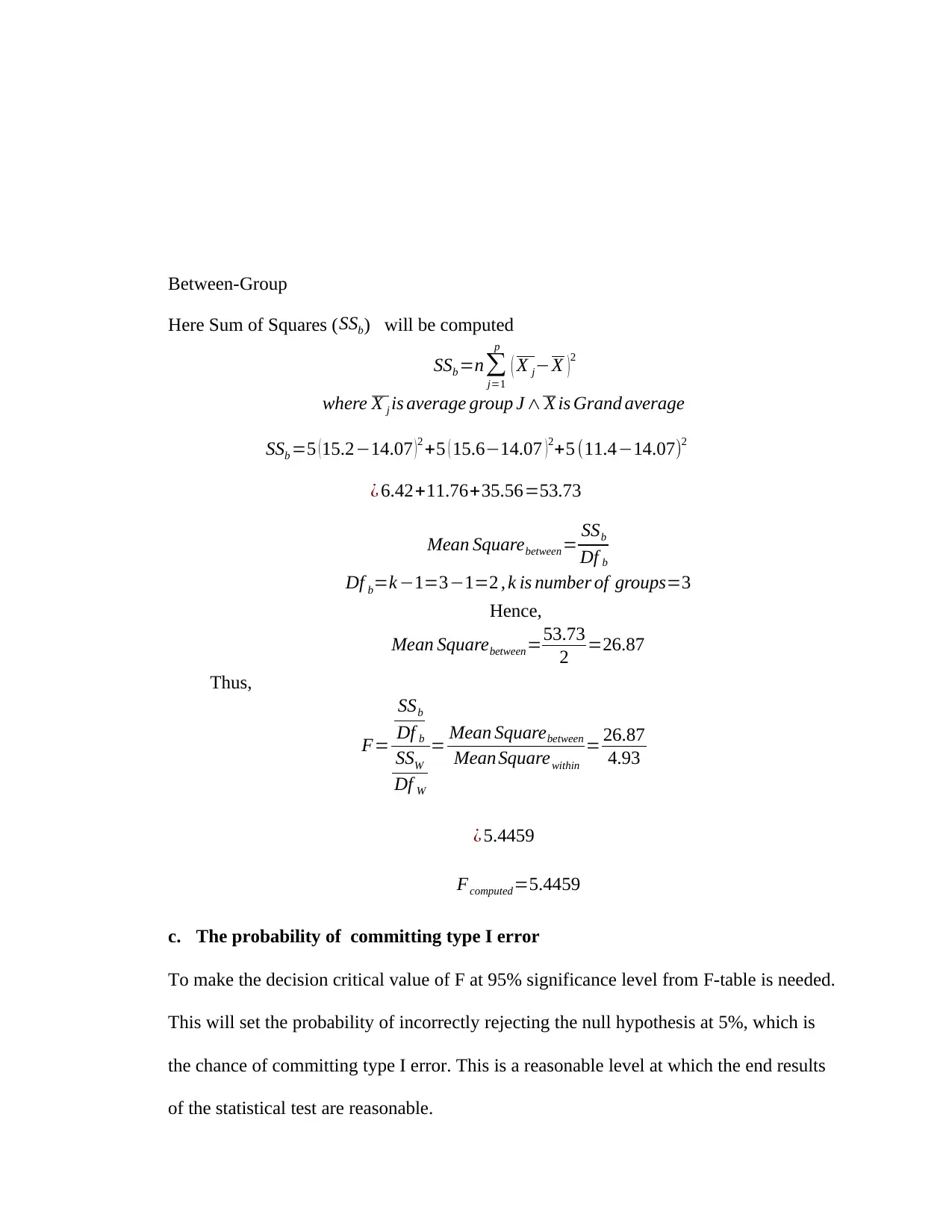
Between-Group
Here Sum of Squares ( SSb) will be computed
SSb =n∑
j=1
p
( X j−X )2
where X j is average group J ∧ X is Grand average
SSb =5 (15.2−14.07 )2 +5 ( 15.6−14.07 )2+5 (11.4−14.07)2
¿ 6.42+11.76+35.56=53.73
Mean Squarebetween= SSb
Df b
Df b=k −1=3−1=2 , k is number of groups=3
Hence,
Mean Squarebetween=53.73
2 =26.87
Thus,
F=
SSb
Df b
SSW
Df W
= Mean Squarebetween
Mean Squarewithin
= 26.87
4.93
¿ 5.4459
Fcomputed=5.4459
c. The probability of committing type I error
To make the decision critical value of F at 95% significance level from F-table is needed.
This will set the probability of incorrectly rejecting the null hypothesis at 5%, which is
the chance of committing type I error. This is a reasonable level at which the end results
of the statistical test are reasonable.
Here Sum of Squares ( SSb) will be computed
SSb =n∑
j=1
p
( X j−X )2
where X j is average group J ∧ X is Grand average
SSb =5 (15.2−14.07 )2 +5 ( 15.6−14.07 )2+5 (11.4−14.07)2
¿ 6.42+11.76+35.56=53.73
Mean Squarebetween= SSb
Df b
Df b=k −1=3−1=2 , k is number of groups=3
Hence,
Mean Squarebetween=53.73
2 =26.87
Thus,
F=
SSb
Df b
SSW
Df W
= Mean Squarebetween
Mean Squarewithin
= 26.87
4.93
¿ 5.4459
Fcomputed=5.4459
c. The probability of committing type I error
To make the decision critical value of F at 95% significance level from F-table is needed.
This will set the probability of incorrectly rejecting the null hypothesis at 5%, which is
the chance of committing type I error. This is a reasonable level at which the end results
of the statistical test are reasonable.

For the above case, the critical value of F at 95% significance level will be,
Fα ( 2 ,12 df ) =3.89
a. Decision
d. Since
Fcomputed=5.4459 ¿ Fα=3.89 , the null hypothesis H0 : μ0=μ1 =μ2 will be rejected.
In this case, the alternative hypothesis accepted. This suggests that there is a
significant difference between the different methods of training. This means that
employees from different level will not complete a task within the same period
of time, some will spend more time, while others will take less time.
In conclusion, the time takes to complete a task depends on the method of training
the employee has undergone. This revealed by the test statistic conducted on the data.
Question Four
a. Road traffic data from Road Safety Authority
i. Stating the hypotheses to test if there is a difference
The hypotheses will be:
H0: There’s no difference
This leading to the determination of Expected results, for our case, all the days of
the will be said to have equal number of accidents, 11 accidents. This establishes
the claim there is no difference
H1: There’s difference
Fα ( 2 ,12 df ) =3.89
a. Decision
d. Since
Fcomputed=5.4459 ¿ Fα=3.89 , the null hypothesis H0 : μ0=μ1 =μ2 will be rejected.
In this case, the alternative hypothesis accepted. This suggests that there is a
significant difference between the different methods of training. This means that
employees from different level will not complete a task within the same period
of time, some will spend more time, while others will take less time.
In conclusion, the time takes to complete a task depends on the method of training
the employee has undergone. This revealed by the test statistic conducted on the data.
Question Four
a. Road traffic data from Road Safety Authority
i. Stating the hypotheses to test if there is a difference
The hypotheses will be:
H0: There’s no difference
This leading to the determination of Expected results, for our case, all the days of
the will be said to have equal number of accidents, 11 accidents. This establishes
the claim there is no difference
H1: There’s difference
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
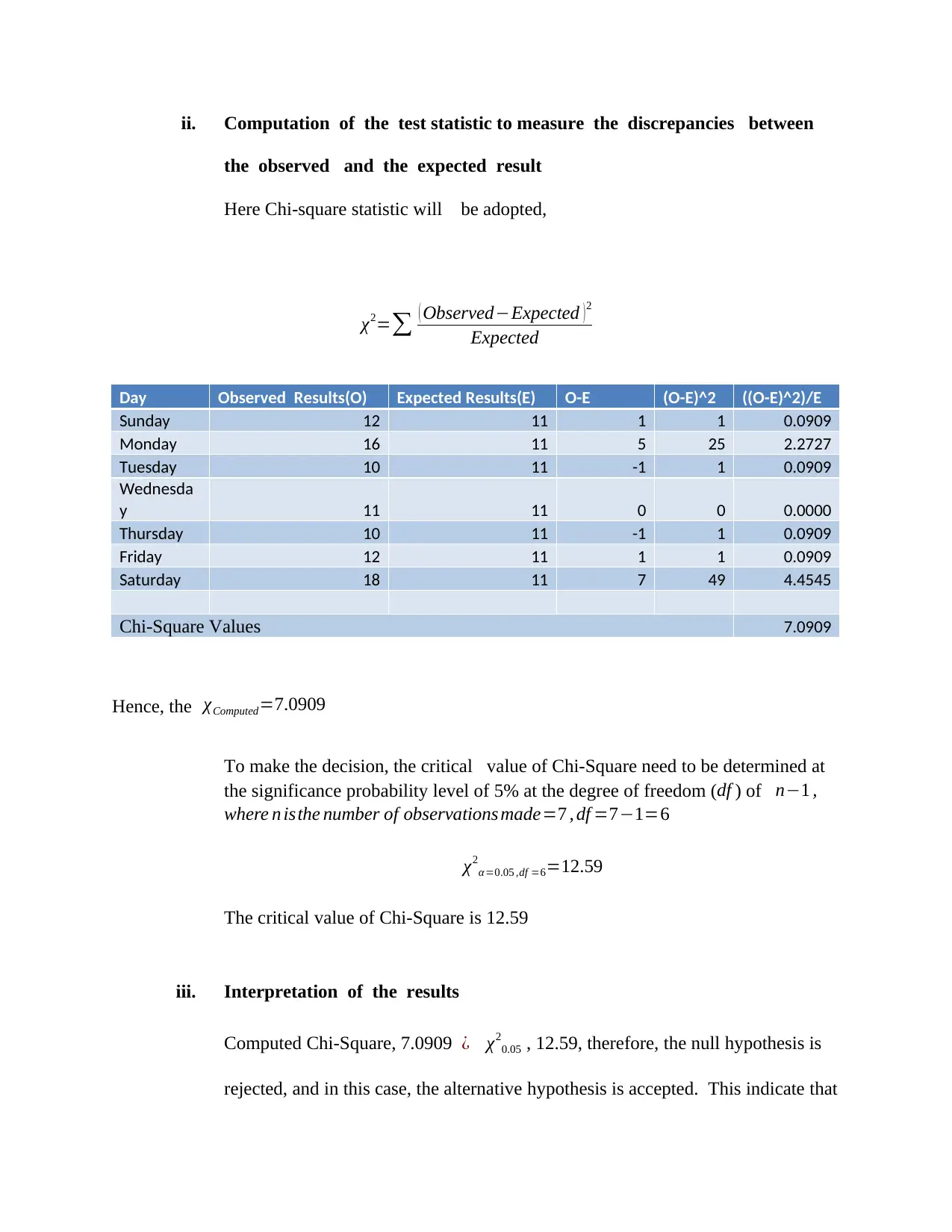
ii. Computation of the test statistic to measure the discrepancies between
the observed and the expected result
Here Chi-square statistic will be adopted,
χ2=∑ ( Observed−Expected )2
Expected
Day Observed Results(O) Expected Results(E) O-E (O-E)^2 ((O-E)^2)/E
Sunday 12 11 1 1 0.0909
Monday 16 11 5 25 2.2727
Tuesday 10 11 -1 1 0.0909
Wednesda
y 11 11 0 0 0.0000
Thursday 10 11 -1 1 0.0909
Friday 12 11 1 1 0.0909
Saturday 18 11 7 49 4.4545
Chi-Square Values 7.0909
Hence, the χComputed=7.0909
To make the decision, the critical value of Chi-Square need to be determined at
the significance probability level of 5% at the degree of freedom (df ) of n−1 ,
where n isthe number of observations made=7 , df =7−1=6
χ2
α =0.05 ,df =6=12.59
The critical value of Chi-Square is 12.59
iii. Interpretation of the results
Computed Chi-Square, 7.0909 ¿ χ2
0.05 , 12.59, therefore, the null hypothesis is
rejected, and in this case, the alternative hypothesis is accepted. This indicate that
the observed and the expected result
Here Chi-square statistic will be adopted,
χ2=∑ ( Observed−Expected )2
Expected
Day Observed Results(O) Expected Results(E) O-E (O-E)^2 ((O-E)^2)/E
Sunday 12 11 1 1 0.0909
Monday 16 11 5 25 2.2727
Tuesday 10 11 -1 1 0.0909
Wednesda
y 11 11 0 0 0.0000
Thursday 10 11 -1 1 0.0909
Friday 12 11 1 1 0.0909
Saturday 18 11 7 49 4.4545
Chi-Square Values 7.0909
Hence, the χComputed=7.0909
To make the decision, the critical value of Chi-Square need to be determined at
the significance probability level of 5% at the degree of freedom (df ) of n−1 ,
where n isthe number of observations made=7 , df =7−1=6
χ2
α =0.05 ,df =6=12.59
The critical value of Chi-Square is 12.59
iii. Interpretation of the results
Computed Chi-Square, 7.0909 ¿ χ2
0.05 , 12.59, therefore, the null hypothesis is
rejected, and in this case, the alternative hypothesis is accepted. This indicate that
there is a difference between the expected resulted and the observed results at
the significance probability level of 5%.
b. Closing Price of stock from 27Th October to 7Th November 2016
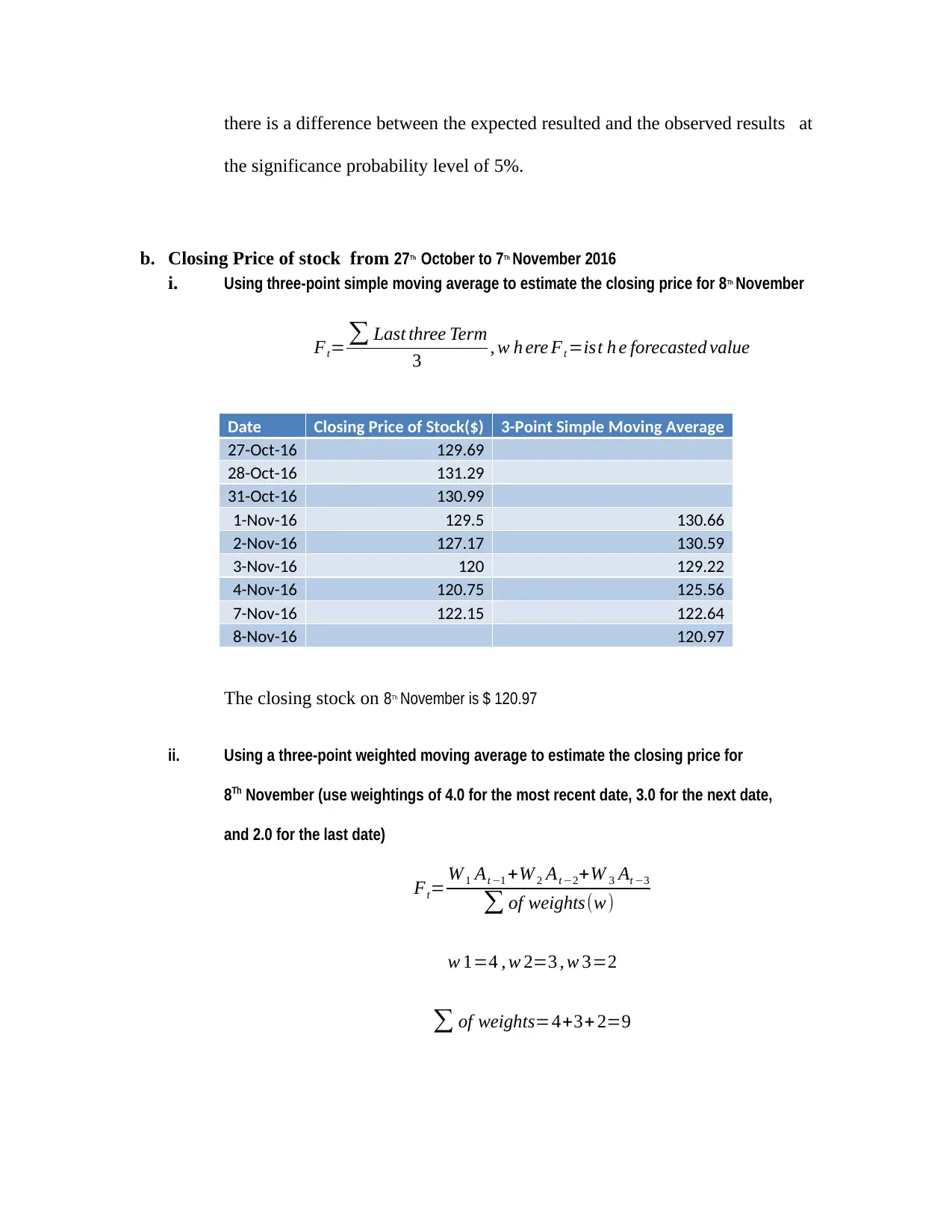
i. Using three-point simple moving average to estimate the closing price for 8Th November
Ft= ∑ Last three Term
3 , w h ere Ft =ist h e forecasted value
Date Closing Price of Stock($) 3-Point Simple Moving Average
27-Oct-16 129.69
28-Oct-16 131.29
31-Oct-16 130.99
1-Nov-16 129.5 130.66
2-Nov-16 127.17 130.59
3-Nov-16 120 129.22
4-Nov-16 120.75 125.56
7-Nov-16 122.15 122.64
8-Nov-16 120.97
The closing stock on 8Th November is $ 120.97
ii. Using a three-point weighted moving average to estimate the closing price for
8Th November (use weightings of 4.0 for the most recent date, 3.0 for the next date,
and 2.0 for the last date)
Ft= W 1 At −1 +W 2 At −2+W 3 At −3
∑ of weights(w)
w 1=4 , w 2=3 , w 3=2
∑ of weights=4+3+ 2=9
the significance probability level of 5%.
b. Closing Price of stock from 27Th October to 7Th November 2016
i. Using three-point simple moving average to estimate the closing price for 8Th November
Ft= ∑ Last three Term
3 , w h ere Ft =ist h e forecasted value
Date Closing Price of Stock($) 3-Point Simple Moving Average
27-Oct-16 129.69
28-Oct-16 131.29
31-Oct-16 130.99
1-Nov-16 129.5 130.66
2-Nov-16 127.17 130.59
3-Nov-16 120 129.22
4-Nov-16 120.75 125.56
7-Nov-16 122.15 122.64
8-Nov-16 120.97
The closing stock on 8Th November is $ 120.97
ii. Using a three-point weighted moving average to estimate the closing price for
8Th November (use weightings of 4.0 for the most recent date, 3.0 for the next date,
and 2.0 for the last date)
Ft= W 1 At −1 +W 2 At −2+W 3 At −3
∑ of weights(w)
w 1=4 , w 2=3 , w 3=2
∑ of weights=4+3+ 2=9
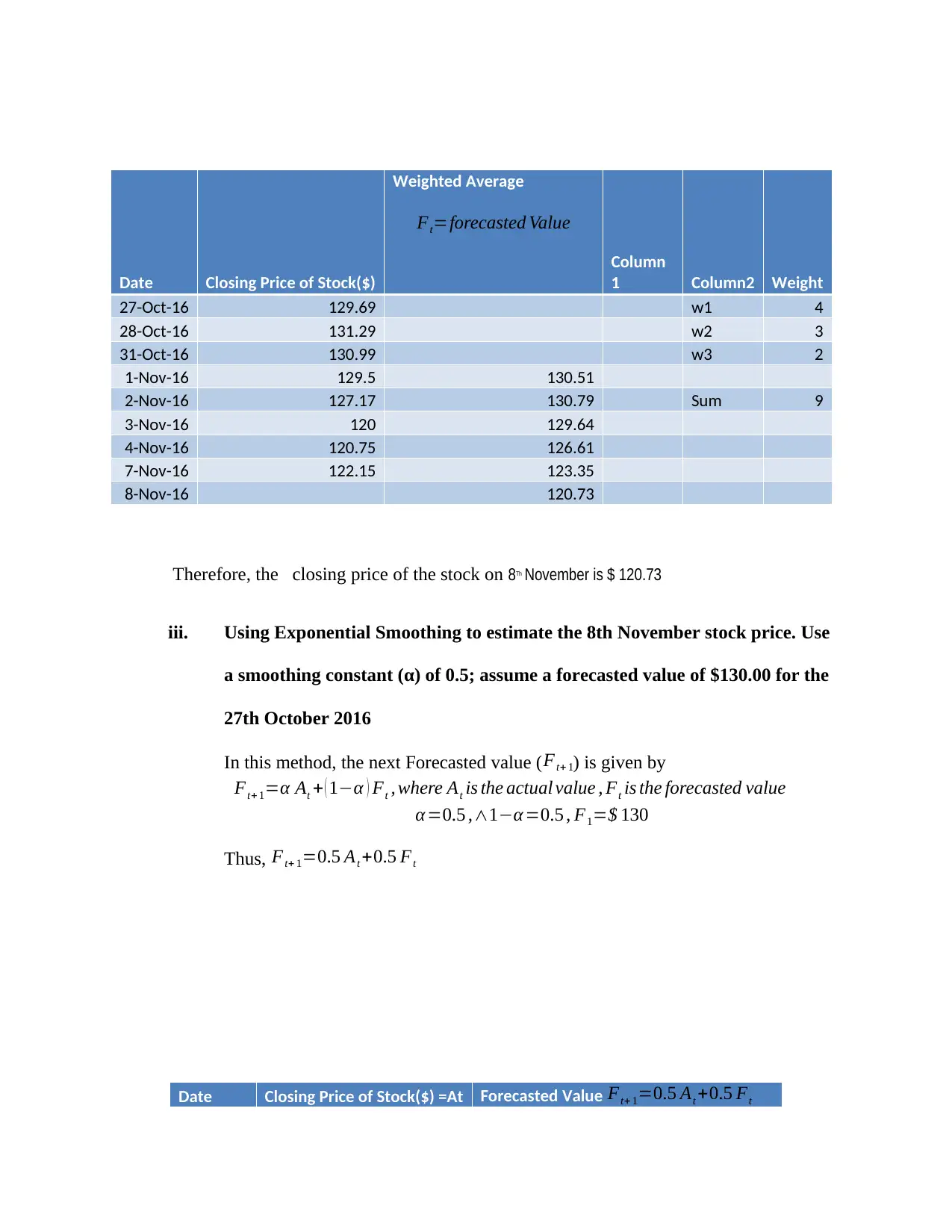
Date Closing Price of Stock($)
Weighted Average
Ft=forecasted Value
Column
1 Column2 Weight
27-Oct-16 129.69 w1 4
28-Oct-16 131.29 w2 3
31-Oct-16 130.99 w3 2
1-Nov-16 129.5 130.51
2-Nov-16 127.17 130.79 Sum 9
3-Nov-16 120 129.64
4-Nov-16 120.75 126.61
7-Nov-16 122.15 123.35
8-Nov-16 120.73
Therefore, the closing price of the stock on 8Th November is $ 120.73
iii. Using Exponential Smoothing to estimate the 8th November stock price. Use
a smoothing constant (α) of 0.5; assume a forecasted value of $130.00 for the
27th October 2016
In this method, the next Forecasted value ( Ft+ 1) is given by
Ft+ 1=α At + ( 1−α ) Ft , where At is the actual value , Ft is the forecasted value
α=0.5 ,∧1−α =0.5 , F1=$ 130
Thus, Ft+ 1=0.5 At +0.5 Ft
Date Closing Price of Stock($) =At Forecasted Value Ft+ 1=0.5 At +0.5 Ft
Weighted Average
Ft=forecasted Value
Column
1 Column2 Weight
27-Oct-16 129.69 w1 4
28-Oct-16 131.29 w2 3
31-Oct-16 130.99 w3 2
1-Nov-16 129.5 130.51
2-Nov-16 127.17 130.79 Sum 9
3-Nov-16 120 129.64
4-Nov-16 120.75 126.61
7-Nov-16 122.15 123.35
8-Nov-16 120.73
Therefore, the closing price of the stock on 8Th November is $ 120.73
iii. Using Exponential Smoothing to estimate the 8th November stock price. Use
a smoothing constant (α) of 0.5; assume a forecasted value of $130.00 for the
27th October 2016
In this method, the next Forecasted value ( Ft+ 1) is given by
Ft+ 1=α At + ( 1−α ) Ft , where At is the actual value , Ft is the forecasted value
α=0.5 ,∧1−α =0.5 , F1=$ 130
Thus, Ft+ 1=0.5 At +0.5 Ft
Date Closing Price of Stock($) =At Forecasted Value Ft+ 1=0.5 At +0.5 Ft
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
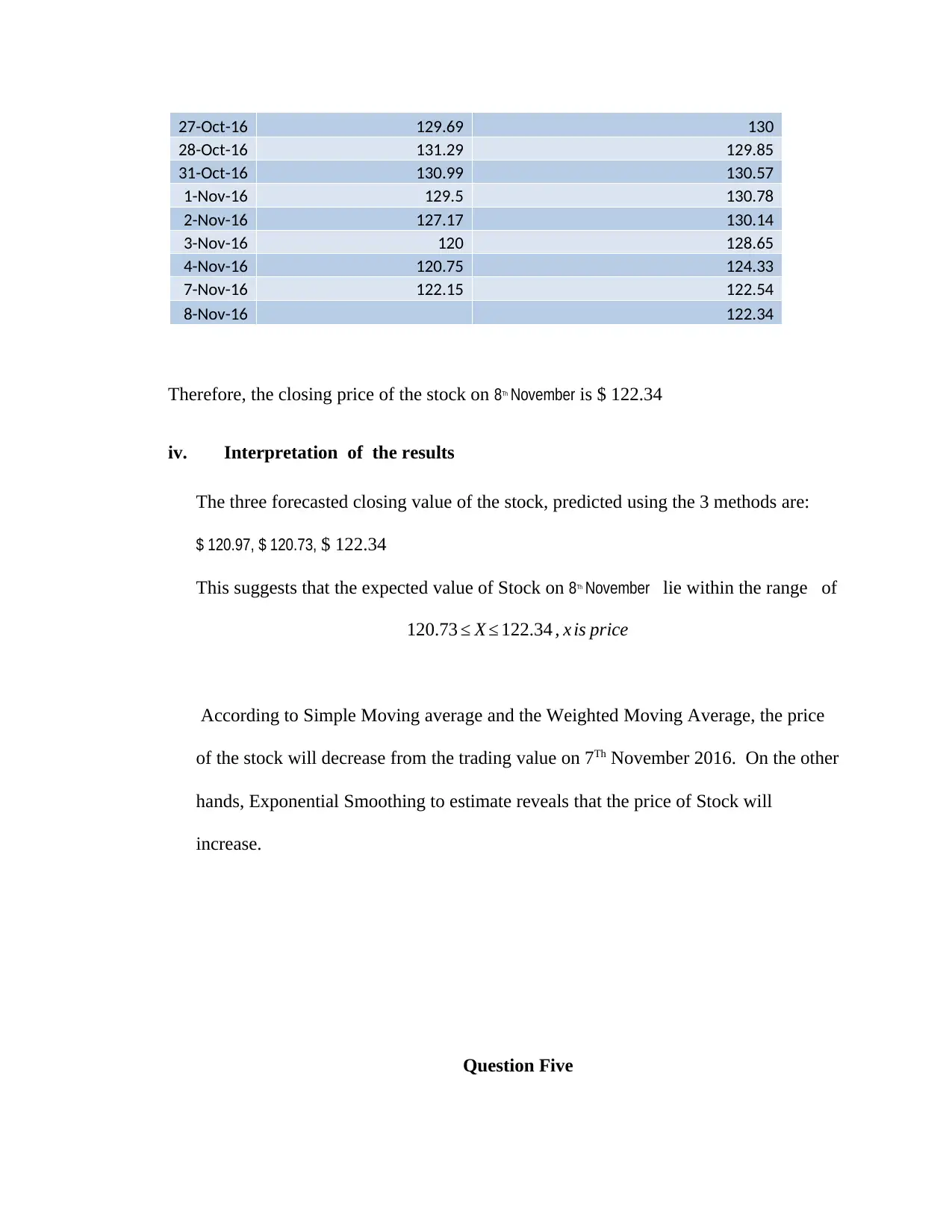
27-Oct-16 129.69 130
28-Oct-16 131.29 129.85
31-Oct-16 130.99 130.57
1-Nov-16 129.5 130.78
2-Nov-16 127.17 130.14
3-Nov-16 120 128.65
4-Nov-16 120.75 124.33
7-Nov-16 122.15 122.54
8-Nov-16 122.34
Therefore, the closing price of the stock on 8Th November is $ 122.34
iv. Interpretation of the results
The three forecasted closing value of the stock, predicted using the 3 methods are:
$ 120.97, $ 120.73, $ 122.34
This suggests that the expected value of Stock on 8Th November lie within the range of
120.73 ≤ X ≤ 122.34 , x is price
According to Simple Moving average and the Weighted Moving Average, the price
of the stock will decrease from the trading value on 7Th November 2016. On the other
hands, Exponential Smoothing to estimate reveals that the price of Stock will
increase.
Question Five
28-Oct-16 131.29 129.85
31-Oct-16 130.99 130.57
1-Nov-16 129.5 130.78
2-Nov-16 127.17 130.14
3-Nov-16 120 128.65
4-Nov-16 120.75 124.33
7-Nov-16 122.15 122.54
8-Nov-16 122.34
Therefore, the closing price of the stock on 8Th November is $ 122.34
iv. Interpretation of the results
The three forecasted closing value of the stock, predicted using the 3 methods are:
$ 120.97, $ 120.73, $ 122.34
This suggests that the expected value of Stock on 8Th November lie within the range of
120.73 ≤ X ≤ 122.34 , x is price
According to Simple Moving average and the Weighted Moving Average, the price
of the stock will decrease from the trading value on 7Th November 2016. On the other
hands, Exponential Smoothing to estimate reveals that the price of Stock will
increase.
Question Five
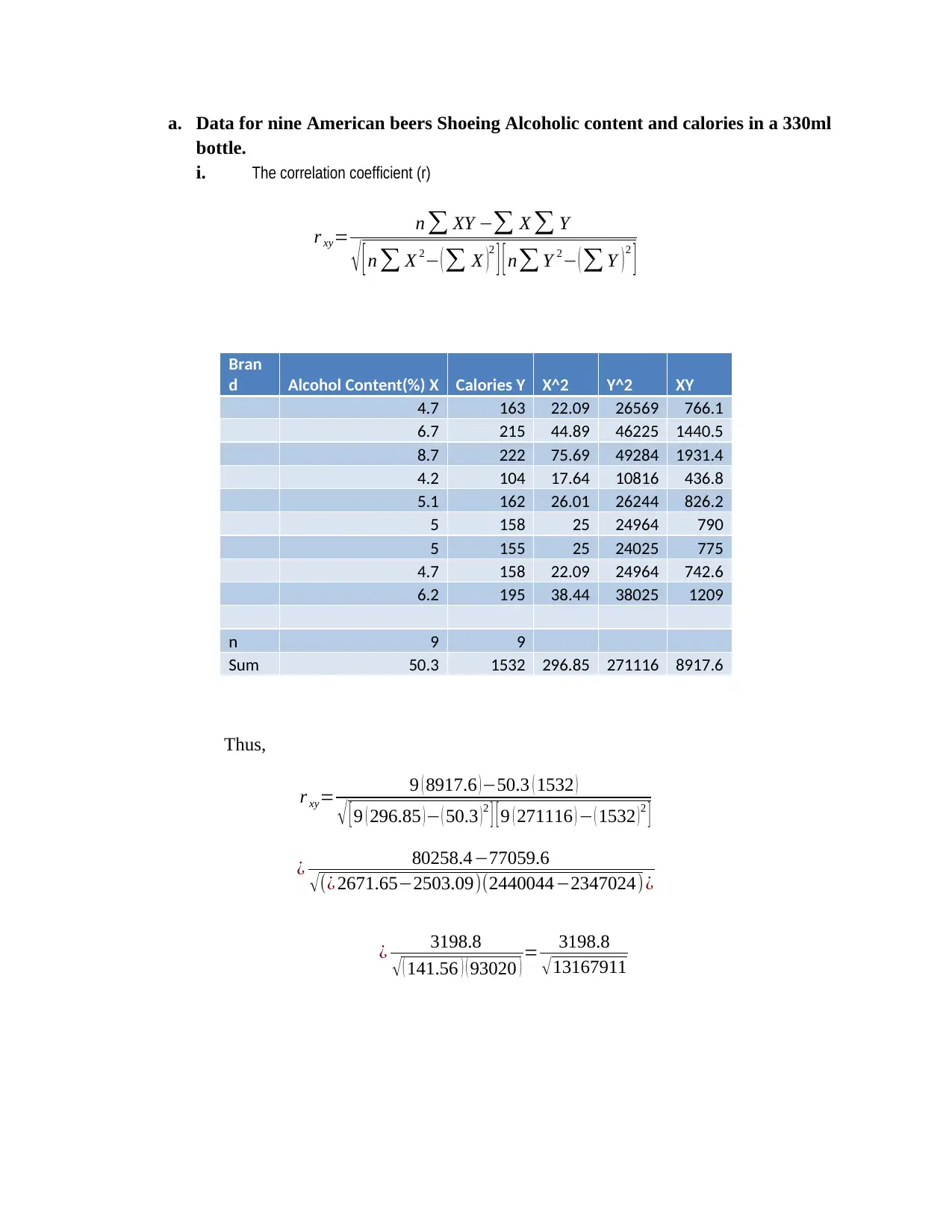
a. Data for nine American beers Shoeing Alcoholic content and calories in a 330ml
bottle.
i. The correlation coefficient (r)
r xy= n ∑ XY −∑ X ∑ Y
√ [ n ∑ X 2− (∑ X )2
] [ n∑ Y 2− (∑ Y )2
]
Bran
d Alcohol Content(%) X Calories Y X^2 Y^2 XY
4.7 163 22.09 26569 766.1
6.7 215 44.89 46225 1440.5
8.7 222 75.69 49284 1931.4
4.2 104 17.64 10816 436.8
5.1 162 26.01 26244 826.2
5 158 25 24964 790
5 155 25 24025 775
4.7 158 22.09 24964 742.6
6.2 195 38.44 38025 1209
n 9 9
Sum 50.3 1532 296.85 271116 8917.6
Thus,
r xy= 9 ( 8917.6 )−50.3 ( 1532 )
√ [ 9 ( 296.85 )− ( 50.3 )2 ] [ 9 ( 271116 ) − ( 1532 )2 ]
¿ 80258.4−77059.6
√(¿ 2671.65−2503.09)(2440044−2347024)¿
¿ 3198.8
√ ( 141.56 ) ( 93020 ) = 3198.8
√ 13167911
bottle.
i. The correlation coefficient (r)
r xy= n ∑ XY −∑ X ∑ Y
√ [ n ∑ X 2− (∑ X )2
] [ n∑ Y 2− (∑ Y )2
]
Bran
d Alcohol Content(%) X Calories Y X^2 Y^2 XY
4.7 163 22.09 26569 766.1
6.7 215 44.89 46225 1440.5
8.7 222 75.69 49284 1931.4
4.2 104 17.64 10816 436.8
5.1 162 26.01 26244 826.2
5 158 25 24964 790
5 155 25 24025 775
4.7 158 22.09 24964 742.6
6.2 195 38.44 38025 1209
n 9 9
Sum 50.3 1532 296.85 271116 8917.6
Thus,
r xy= 9 ( 8917.6 )−50.3 ( 1532 )
√ [ 9 ( 296.85 )− ( 50.3 )2 ] [ 9 ( 271116 ) − ( 1532 )2 ]
¿ 80258.4−77059.6
√(¿ 2671.65−2503.09)(2440044−2347024)¿
¿ 3198.8
√ ( 141.56 ) ( 93020 ) = 3198.8
√ 13167911
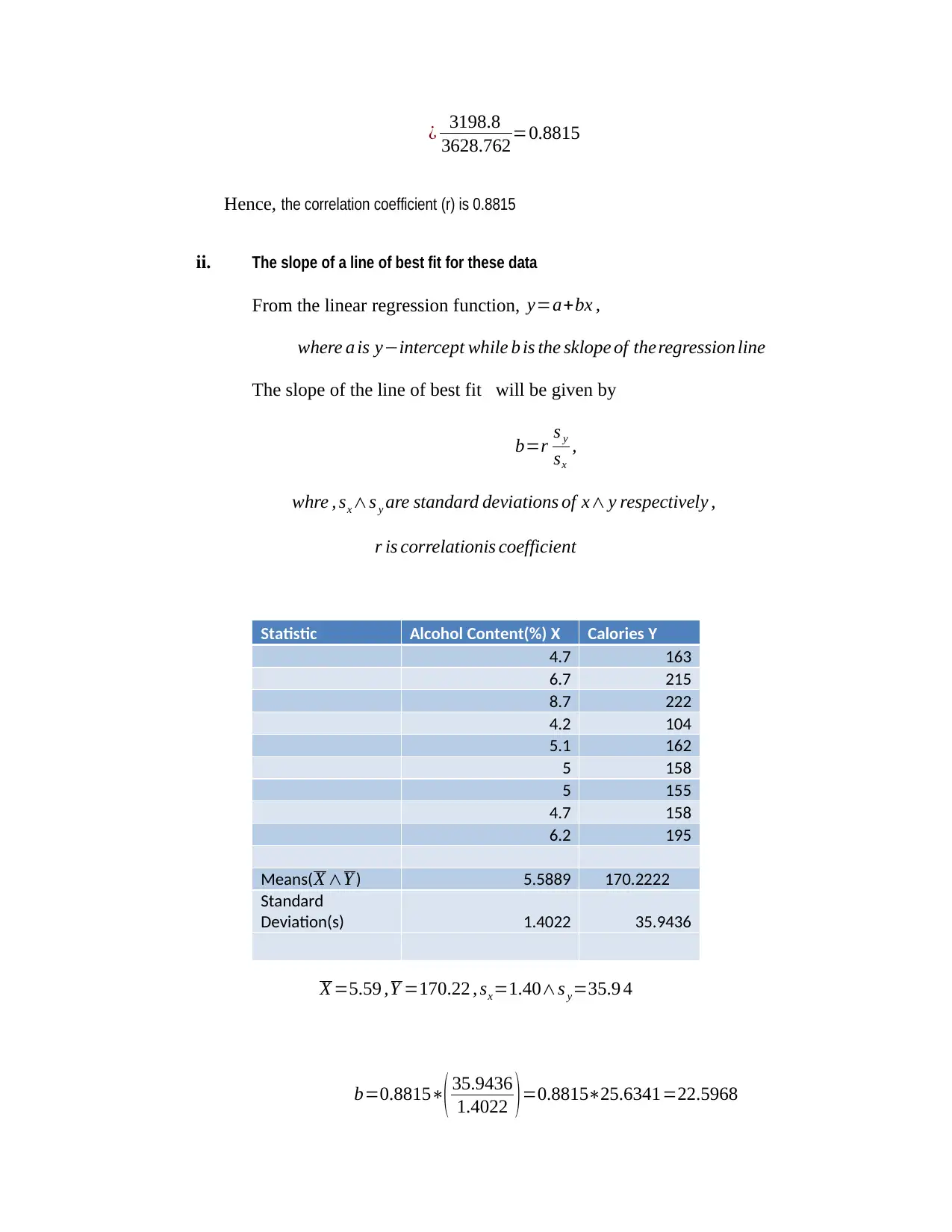
¿ 3198.8
3628.762=0.8815
Hence, the correlation coefficient (r) is 0.8815
ii. The slope of a line of best fit for these data
From the linear regression function, y=a+bx ,
where a is y−intercept while b is the sklope of the regression line
The slope of the line of best fit will be given by
b=r s y
sx
,
whre , sx∧s y are standard deviations of x∧ y respectively ,
r is correlationis coefficient
X =5.59 ,Y =170.22 , sx=1.40∧s y=35.9 4
b=0.8815∗( 35.9436
1.4022 ) =0.8815∗25.6341=22.5968
Statistic Alcohol Content(%) X Calories Y
4.7 163
6.7 215
8.7 222
4.2 104
5.1 162
5 158
5 155
4.7 158
6.2 195
Means(X ∧Y ) 5.5889 170.2222
Standard
Deviation(s) 1.4022 35.9436
3628.762=0.8815
Hence, the correlation coefficient (r) is 0.8815
ii. The slope of a line of best fit for these data
From the linear regression function, y=a+bx ,
where a is y−intercept while b is the sklope of the regression line
The slope of the line of best fit will be given by
b=r s y
sx
,
whre , sx∧s y are standard deviations of x∧ y respectively ,
r is correlationis coefficient
X =5.59 ,Y =170.22 , sx=1.40∧s y=35.9 4
b=0.8815∗( 35.9436
1.4022 ) =0.8815∗25.6341=22.5968
Statistic Alcohol Content(%) X Calories Y
4.7 163
6.7 215
8.7 222
4.2 104
5.1 162
5 158
5 155
4.7 158
6.2 195
Means(X ∧Y ) 5.5889 170.2222
Standard
Deviation(s) 1.4022 35.9436
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Therefore, the slope of the line of best fit will be 22.5968
iii. The Y-intercept, a
a=Y −b X
¿ 170.22−22.5968 ( 5.59 )=43.9313
Hence, the Y-intercept is 43.9313
iv. The value of Y (calories) given a value of X = 5.5% alcohol
From the ii and iii above the linear regression will be given by
The equation of the line of best fit will be given by
y=43.9313+22.5968 x
The value of y at X=5.5%
y=43.9313+22.5968 ( 5.5 )
¿ 168.2136
b. The simple liner regression equation for these data is y = 1.6656x + 68.999 and
the correlation coefficient is r = 0.17.
iii. The Y-intercept, a
a=Y −b X
¿ 170.22−22.5968 ( 5.59 )=43.9313
Hence, the Y-intercept is 43.9313
iv. The value of Y (calories) given a value of X = 5.5% alcohol
From the ii and iii above the linear regression will be given by
The equation of the line of best fit will be given by
y=43.9313+22.5968 x
The value of y at X=5.5%
y=43.9313+22.5968 ( 5.5 )
¿ 168.2136
b. The simple liner regression equation for these data is y = 1.6656x + 68.999 and
the correlation coefficient is r = 0.17.

Description of the relation between the two variables
The two variables are linearly related since the correlation of coefficient (0.17) is greater
than 0. Moreover, since the correlation coefficient (0.17) and the gradient of the linear
regression (1.6656) are positive, the two variables are said to have a positive linear
relationship. Finally, the positive gradient (1.6656) indicates that the line of two
variables has a positive slope, thus left-right upward slope on the chart.
In conclusion, the two variables, Health Expenditure and Prenatal Care have a
positive linear relationship due to their positive correlation coefficient (0.17), and
positive slope (1.6656). This suggests that two variables are directly related, thus a
change in one, leads to change in the other as demonstrated by the upward slope of the
linear chart in the question.
References
The two variables are linearly related since the correlation of coefficient (0.17) is greater
than 0. Moreover, since the correlation coefficient (0.17) and the gradient of the linear
regression (1.6656) are positive, the two variables are said to have a positive linear
relationship. Finally, the positive gradient (1.6656) indicates that the line of two
variables has a positive slope, thus left-right upward slope on the chart.
In conclusion, the two variables, Health Expenditure and Prenatal Care have a
positive linear relationship due to their positive correlation coefficient (0.17), and
positive slope (1.6656). This suggests that two variables are directly related, thus a
change in one, leads to change in the other as demonstrated by the upward slope of the
linear chart in the question.
References

1. Berenson, M., Levine, D., Szabat, K.A. and Krehbiel, T.C., 2012. Basic business
statistics: Concepts and applications. Pearson higher education AU
2. Francis, A., 2004. Business mathematics and statistics. Cengage Learning EMEA.
3. Hassett, M.J., and Stewart, D., 2006. The probability for risk management. Actex
Publications.
4. Montgomery, D.C. and Runger, G.C., 2010. Applied statistics and probability for
engineers. John Wiley & Sons.
5. Tallarida, R.J., and Murray, R.B., 1987. Chi-square test. In Manual of Pharmacologic
Calculations (pp. 140-142). Springer, New York, NY.
6. Wald, A. and Wolfowitz, J., 1940. On a test whether two samples are from the same
population. The Annals of Mathematical Statistics, 11(2), pp.147-162.
statistics: Concepts and applications. Pearson higher education AU
2. Francis, A., 2004. Business mathematics and statistics. Cengage Learning EMEA.
3. Hassett, M.J., and Stewart, D., 2006. The probability for risk management. Actex
Publications.
4. Montgomery, D.C. and Runger, G.C., 2010. Applied statistics and probability for
engineers. John Wiley & Sons.
5. Tallarida, R.J., and Murray, R.B., 1987. Chi-square test. In Manual of Pharmacologic
Calculations (pp. 140-142). Springer, New York, NY.
6. Wald, A. and Wolfowitz, J., 1940. On a test whether two samples are from the same
population. The Annals of Mathematical Statistics, 11(2), pp.147-162.
1 out of 19
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.




