Statistics - Analysis of Regression Models and ANOVA Tables

This article provides an in-depth analysis of regression models and ANOVA tables in statistics. It covers topics such as frequency distribution, linear regression, correlation, ANOVA tables, and multiple regression models.

Running Head: STATISTICS
Statistics
Name of the student:
Name of the university:
Course ID:

1STATISTICS
Table of Contents
Ans 1................................................................................................................................................3
Ans 1. a).......................................................................................................................................3
Ans 1. b).......................................................................................................................................3
Ans 2................................................................................................................................................4
Ans 2. a).......................................................................................................................................4
Ans 2. b).......................................................................................................................................4
Ans 2. c)........................................................................................................................................4
Ans 2. d).......................................................................................................................................5
Ans 2. e)........................................................................................................................................5
Ans 3................................................................................................................................................5
Ans 3. a).......................................................................................................................................5
Ans 3. b).......................................................................................................................................6
Ans 4................................................................................................................................................6
Ans 4. a).......................................................................................................................................7
Ans 4. b).......................................................................................................................................7
Ans 4. c)........................................................................................................................................7
Ans 4. d).......................................................................................................................................8
Ans 4. e)........................................................................................................................................8

2STATISTICS
Table of tables
Table 1: The frequency distribution of examination scores............................................................3
Table 2: Simple linear regression model of ‘Unit Price’ and ‘Supply’...........................................4
Table 3: One-way ANOVA table of four Programs........................................................................5
Table 4: Multiple regression model of ‘Sales’ as well as ‘Price’ and ‘Advertising’.......................6
Table 5: Simple linear regression model of ‘Sales’ and ‘Price’......................................................8
Table of Figures
Figure 1: Histogram of Examination Scores...................................................................................3

3STATISTICS
Ans 1.
Ans 1. a)
Table 1: The frequency distribution of examination scores
Ans 1. b)
Figure 1: Histogram of Examination Scores
The shape of the distribution of Examination scores indicates that-

4STATISTICS
 Most of the students has scored in the interval 90-99. Only 25% students have scored less
than 70.
 The distribution of examination score is left-skewed as the right tail of the distribution is
more elongated than its left tail.
 For this distribution, the value of mode is greater than median while mean has the least
value.
Ans 2.
Table 2: Simple linear regression model of ‘Unit Price’ and ‘Supply’
Ans 2. a)
The total frequency of the sample is 41 (=40+1).
Ans 2. b)
The level of significance of the regression model is assumed to be 5%. The outcome of
the simple linear regression model shows that the p-value of ‘Unit Price (X)’ (0.175) is greater
than the level of significance.

5STATISTICS
Therefore, the independent and dependent variables are insignificantly linearly associated
to each other with 95% probability. No strong evidence for significant association between
independent and dependent variables are found in this regression model.
Ans 2. c)
The statistic ‘R-square’ is also known as ‘Co-efficient of determination’. In this model,
the statistic R2 is found to be 0.048. It indicates that the predictor variable ‘Unit Price (X)’ can
only explain 4.8% variability of ‘Supply (Y)’.
Ans 2. d)
Pearson’s co-efficient of correlation (r) is 0.21908 that refers a weak positive correlation
between ‘Unit price’ and ‘Supply’ as (r is in-between 0.0 to 0.3). Therefore, these two variables
are not significantly linked.
The Pearson’s correlation coefficient (r) in the range of 0.0 to 0.3 refers a weak but
positive correlation. Such kind of a weak and positive link or correlation is observed between
‘Unit price’ and ‘Supply’ as per the calculated co-efficient of correlation (r = 0.21908).
Ans 2. e)
The linear regression equation is estimated in the following,
‘Supply (Y)’ = 54.076 + 0.029* ‘Unit price (X)’ …………… (1)
Given that the Unit price = $50,000, therefore, Supply = (54.076 + (0.029*50000)) =
1504.076.
Hence, the estimated supply is found to be 1504.076 units.
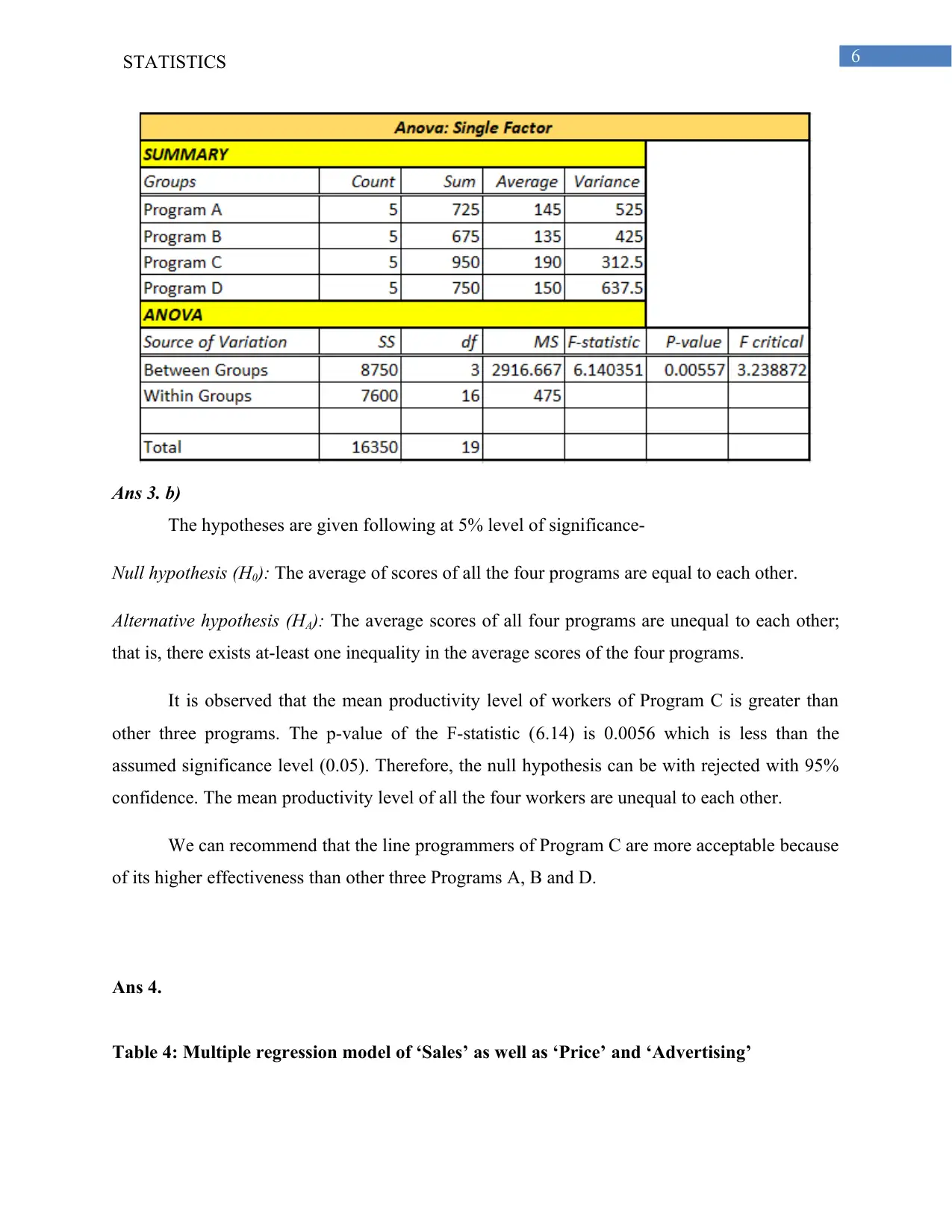
Ans 3.
Ans 3. a)
Table 3: One-way ANOVA table of four Programs

6STATISTICS
Ans 3. b)
The hypotheses are given following at 5% level of significance-
Null hypothesis (H0): The average of scores of all the four programs are equal to each other.
Alternative hypothesis (HA): The average scores of all four programs are unequal to each other;
that is, there exists at-least one inequality in the average scores of the four programs.
It is observed that the mean productivity level of workers of Program C is greater than
other three programs. The p-value of the F-statistic (6.14) is 0.0056 which is less than the
assumed significance level (0.05). Therefore, the null hypothesis can be with rejected with 95%
confidence. The mean productivity level of all the four workers are unequal to each other.
We can recommend that the line programmers of Program C are more acceptable because
of its higher effectiveness than other three Programs A, B and D.
Ans 4.
Table 4: Multiple regression model of ‘Sales’ as well as ‘Price’ and ‘Advertising’

7STATISTICS
Ans 4. a)
The estimated equation of the multiple regression model is-
‘Sales’ (y) = 3.597615086 + 41.32002219 * ‘Price’ (x1) + 0.013241819 * ‘Advertising’ (x2)
………. (2)
Ans 4. b)
The level of significance of the F-test in the multiple regression model is assumed to be
0.1. The regression model assumes ‘Weekly sales of products’ (y) as response factor whereas
‘Unit price of the competitor’s product’ (x1) and ‘Advertising expenditures’ (x2) as predictor
factors. The calculated p-value of the multiple regression model is 0.052643614 that is less than
0.1. Therefore, the null hypothesis can be rejected with 90% confidence. As per the logic of
hypothesis testing, the multiple regression model is found to be statistically significant at 10%
significance level.
Ans 4. c)
The calculated p-values of the independent variables are respectively-
 ‘Price’ (0.036289) and
 ‘Advertising’ (0.969694).

8STATISTICS
The calculated p-value of predictor ‘Price’ is less than 0.05 and ‘Advertising’ is more than
0.05. Therefore, ‘Advertising’ is the insignificant variable and ‘Price’ is the significant variable.
Table 5: Simple linear regression model of ‘Sales’ and ‘Price’
Ans 4. d)
‘Advertising’ is proved to be an insignificant factor; hence, it is omitted from the multiple
regression model.
As per the current regression model, ‘Sales’ is regarded as response factor and ‘Price’ is
regarded as predictive factor. The estimated equation as per the new simple linear regression
model-
‘Sales’ (Y) = 3.58178844 + 41.6030534 * ‘Price’ (X1) …………………………. (3)
Ans 4. e)
The value of slope (β) of the simple linear regression model is found to be 41.6030534. It
shows a positive association between the predictor factor ‘Price’ and the response factor ‘Sales’.
The positive relationship between dependent and independent variable is established as per the
regression model. Also, the predictor variable ‘Sales’ increases by 41.6 units while price
increases by 1 unit. Equally, ‘Sales’ decreases by 41.6 units while price decreases by 1 unit.