Comprehensive Statistical Analysis of Financial Earnings and Data
VerifiedAdded on 2021/02/22
|28
|4065
|51
Report
AI Summary
This report presents a comprehensive analysis of financial statistics, focusing on earnings comparisons between men and women in both public and private sectors. The report utilizes t-tests to determine the significance of earnings differences, time charts to visualize annual income trends, and calcul...

Statistics Financial
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Table of Contents
INTRODUCTION...........................................................................................................................3
MAIN BODY...................................................................................................................................3
TASK 1............................................................................................................................................3
A) Comparison of the earnings of women and men in public sector...........................................3
B) Comparison of the earnings of women and men in private sector..........................................4
C) Presentation of earnings of different sector by Time chart method........................................5
D) Determination of annual earning growth rate.........................................................................6
TASK 2............................................................................................................................................7
A) ................................................................................................................................................7
1) Estimation of median hourly earning and quartiles by ogive method.....................................7
2) Calculation for mean and standard deviation..........................................................................9
B) Comparison of the earnings..................................................................................................10
TASK 3 .........................................................................................................................................11
Section A....................................................................................................................................11
Section B....................................................................................................................................11
TASK 4..........................................................................................................................................12
4.1...............................................................................................................................................12
1. Bar chart.................................................................................................................................12
2. Pie chart.................................................................................................................................15
....................................................................................................................................................17
4.2 Relationship between the number of bedrooms and the house price of bedrooms in all of
the three streets..........................................................................................................................18
CONCLUSION..............................................................................................................................24
REFERENCES..............................................................................................................................25
INTRODUCTION...........................................................................................................................3
MAIN BODY...................................................................................................................................3
TASK 1............................................................................................................................................3
A) Comparison of the earnings of women and men in public sector...........................................3
B) Comparison of the earnings of women and men in private sector..........................................4
C) Presentation of earnings of different sector by Time chart method........................................5
D) Determination of annual earning growth rate.........................................................................6
TASK 2............................................................................................................................................7
A) ................................................................................................................................................7
1) Estimation of median hourly earning and quartiles by ogive method.....................................7
2) Calculation for mean and standard deviation..........................................................................9
B) Comparison of the earnings..................................................................................................10
TASK 3 .........................................................................................................................................11
Section A....................................................................................................................................11
Section B....................................................................................................................................11
TASK 4..........................................................................................................................................12
4.1...............................................................................................................................................12
1. Bar chart.................................................................................................................................12
2. Pie chart.................................................................................................................................15
....................................................................................................................................................17
4.2 Relationship between the number of bedrooms and the house price of bedrooms in all of
the three streets..........................................................................................................................18
CONCLUSION..............................................................................................................................24
REFERENCES..............................................................................................................................25
INTRODUCTION
The term financial statistics is related with all the numerical figures, data and values
which helps in summarizing relevant as well as appropriate information. Data as gathered with
the help of different statistical means is required to be evaluated with the help of the best tools
and methods for deriving proper interpretation from it. The present report is related with the use
of different statistical tools for assessing results out of it. This report will help in assessing
earnings of both the men and women of public & private sector with the help of hypothesis
made. Also, various charts and graphs will be presented for supporting such table made.
Furthermore, analysis of hourly pay rate in different region of UK will be provided. Also,
explanation related to economic order quantity along with requisite calculations made. At last the
report will also shed light on designing of both the bar and pie chart for supporting data of
different streets.
MAIN BODY
TASK 1
A) Comparison of the earnings of women and men in public sector
Null hypothesis H0 : There is no significance difference in the earning of both women and men
who work in public sector.
Alternative Hypothesis H1 : There is significance difference in the earnings of both women and
men who work in public sector.
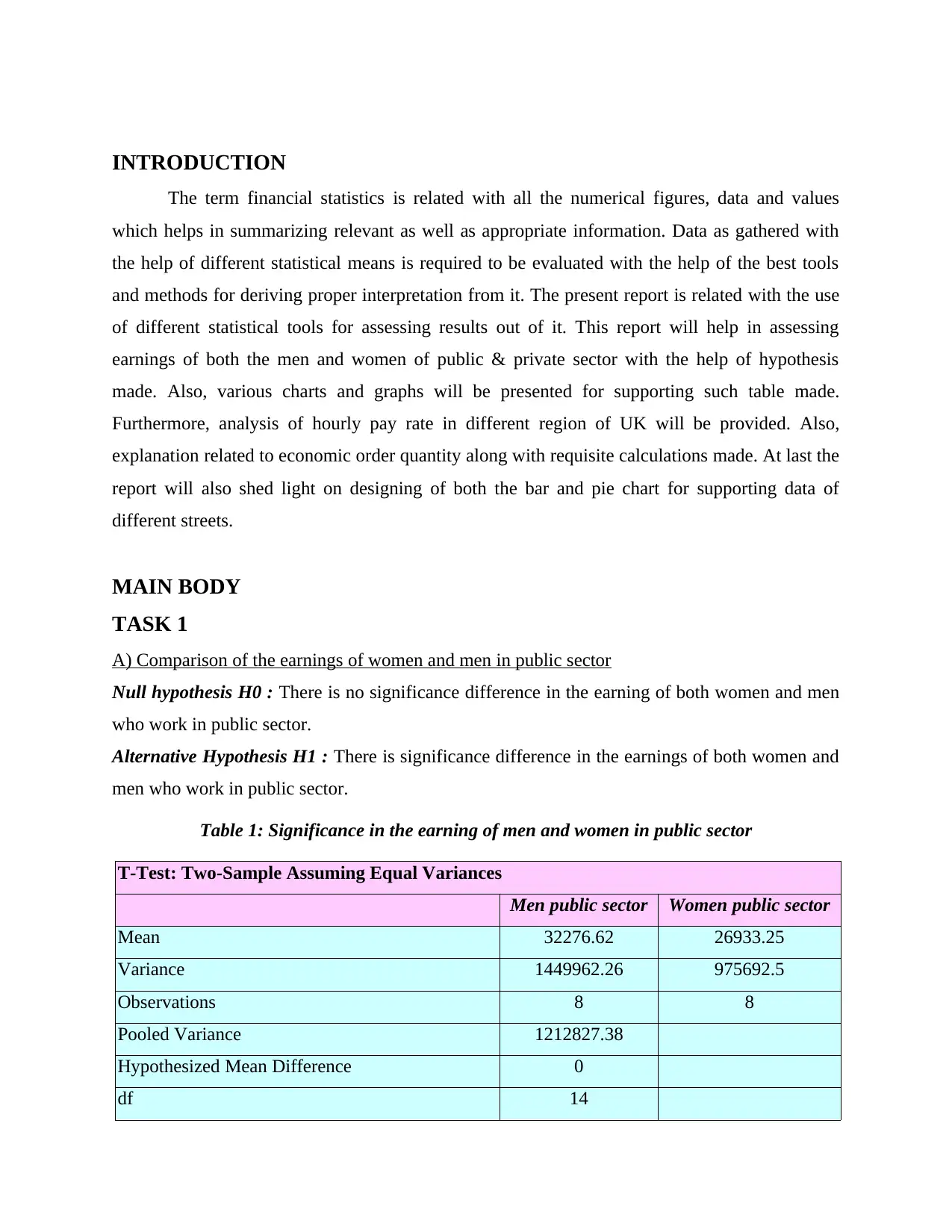
Table 1: Significance in the earning of men and women in public sector
T-Test: Two-Sample Assuming Equal Variances
Men public sector Women public sector
Mean 32276.62 26933.25
Variance 1449962.26 975692.5
Observations 8 8
Pooled Variance 1212827.38
Hypothesized Mean Difference 0
df 14
The term financial statistics is related with all the numerical figures, data and values
which helps in summarizing relevant as well as appropriate information. Data as gathered with
the help of different statistical means is required to be evaluated with the help of the best tools
and methods for deriving proper interpretation from it. The present report is related with the use
of different statistical tools for assessing results out of it. This report will help in assessing
earnings of both the men and women of public & private sector with the help of hypothesis
made. Also, various charts and graphs will be presented for supporting such table made.
Furthermore, analysis of hourly pay rate in different region of UK will be provided. Also,
explanation related to economic order quantity along with requisite calculations made. At last the
report will also shed light on designing of both the bar and pie chart for supporting data of
different streets.
MAIN BODY
TASK 1
A) Comparison of the earnings of women and men in public sector
Null hypothesis H0 : There is no significance difference in the earning of both women and men
who work in public sector.
Alternative Hypothesis H1 : There is significance difference in the earnings of both women and
men who work in public sector.
Table 1: Significance in the earning of men and women in public sector
T-Test: Two-Sample Assuming Equal Variances
Men public sector Women public sector
Mean 32276.62 26933.25
Variance 1449962.26 975692.5
Observations 8 8
Pooled Variance 1212827.38
Hypothesized Mean Difference 0
df 14
You're viewing a preview
Unlock full access by subscribing today!
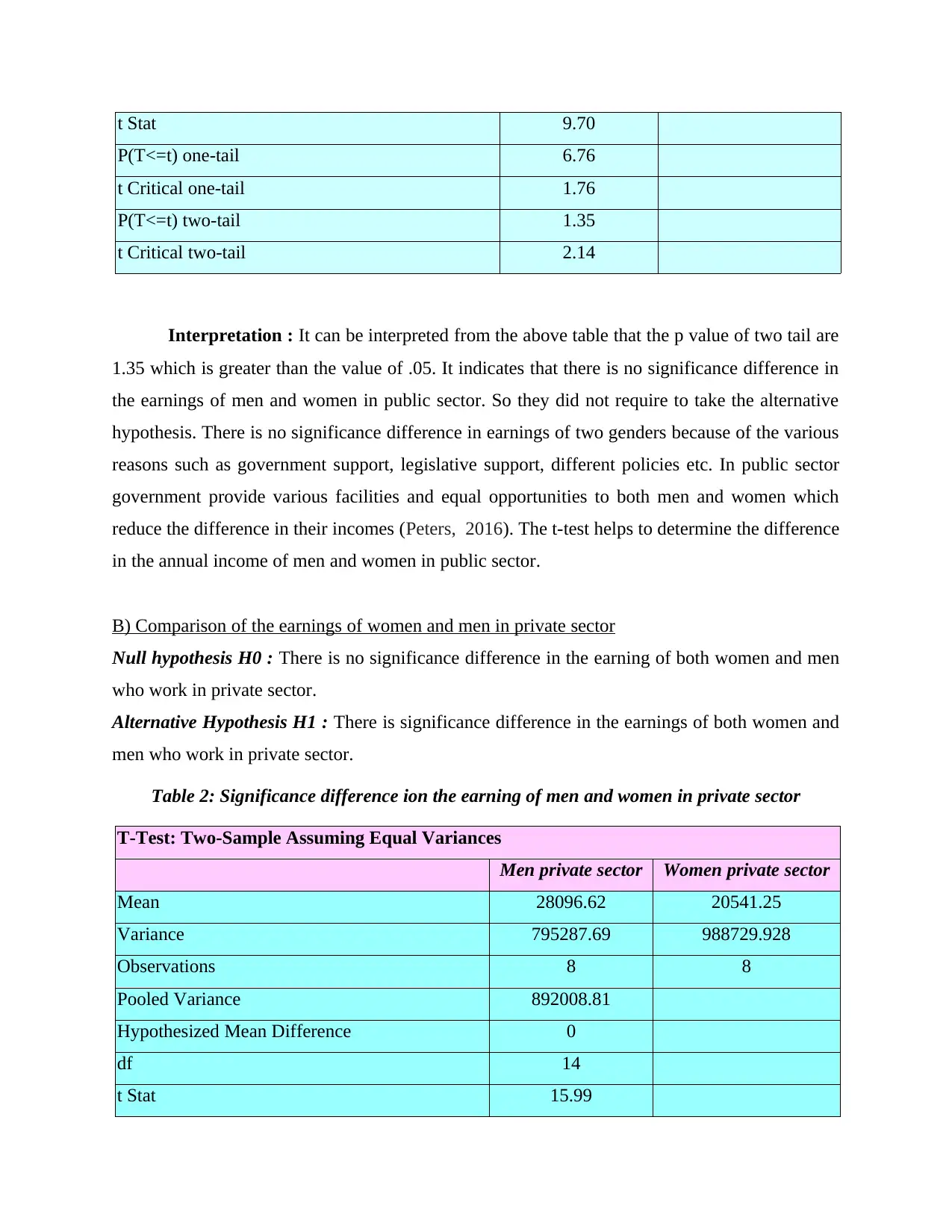
t Stat 9.70
P(T<=t) one-tail 6.76
t Critical one-tail 1.76
P(T<=t) two-tail 1.35
t Critical two-tail 2.14
Interpretation : It can be interpreted from the above table that the p value of two tail are
1.35 which is greater than the value of .05. It indicates that there is no significance difference in
the earnings of men and women in public sector. So they did not require to take the alternative
hypothesis. There is no significance difference in earnings of two genders because of the various
reasons such as government support, legislative support, different policies etc. In public sector
government provide various facilities and equal opportunities to both men and women which
reduce the difference in their incomes (Peters, 2016). The t-test helps to determine the difference
in the annual income of men and women in public sector.
B) Comparison of the earnings of women and men in private sector
Null hypothesis H0 : There is no significance difference in the earning of both women and men
who work in private sector.
Alternative Hypothesis H1 : There is significance difference in the earnings of both women and
men who work in private sector.
Table 2: Significance difference ion the earning of men and women in private sector
T-Test: Two-Sample Assuming Equal Variances
Men private sector Women private sector
Mean 28096.62 20541.25
Variance 795287.69 988729.928
Observations 8 8
Pooled Variance 892008.81
Hypothesized Mean Difference 0
df 14
t Stat 15.99
P(T<=t) one-tail 6.76
t Critical one-tail 1.76
P(T<=t) two-tail 1.35
t Critical two-tail 2.14
Interpretation : It can be interpreted from the above table that the p value of two tail are
1.35 which is greater than the value of .05. It indicates that there is no significance difference in
the earnings of men and women in public sector. So they did not require to take the alternative
hypothesis. There is no significance difference in earnings of two genders because of the various
reasons such as government support, legislative support, different policies etc. In public sector
government provide various facilities and equal opportunities to both men and women which
reduce the difference in their incomes (Peters, 2016). The t-test helps to determine the difference
in the annual income of men and women in public sector.
B) Comparison of the earnings of women and men in private sector
Null hypothesis H0 : There is no significance difference in the earning of both women and men
who work in private sector.
Alternative Hypothesis H1 : There is significance difference in the earnings of both women and
men who work in private sector.
Table 2: Significance difference ion the earning of men and women in private sector
T-Test: Two-Sample Assuming Equal Variances
Men private sector Women private sector
Mean 28096.62 20541.25
Variance 795287.69 988729.928
Observations 8 8
Pooled Variance 892008.81
Hypothesized Mean Difference 0
df 14
t Stat 15.99
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
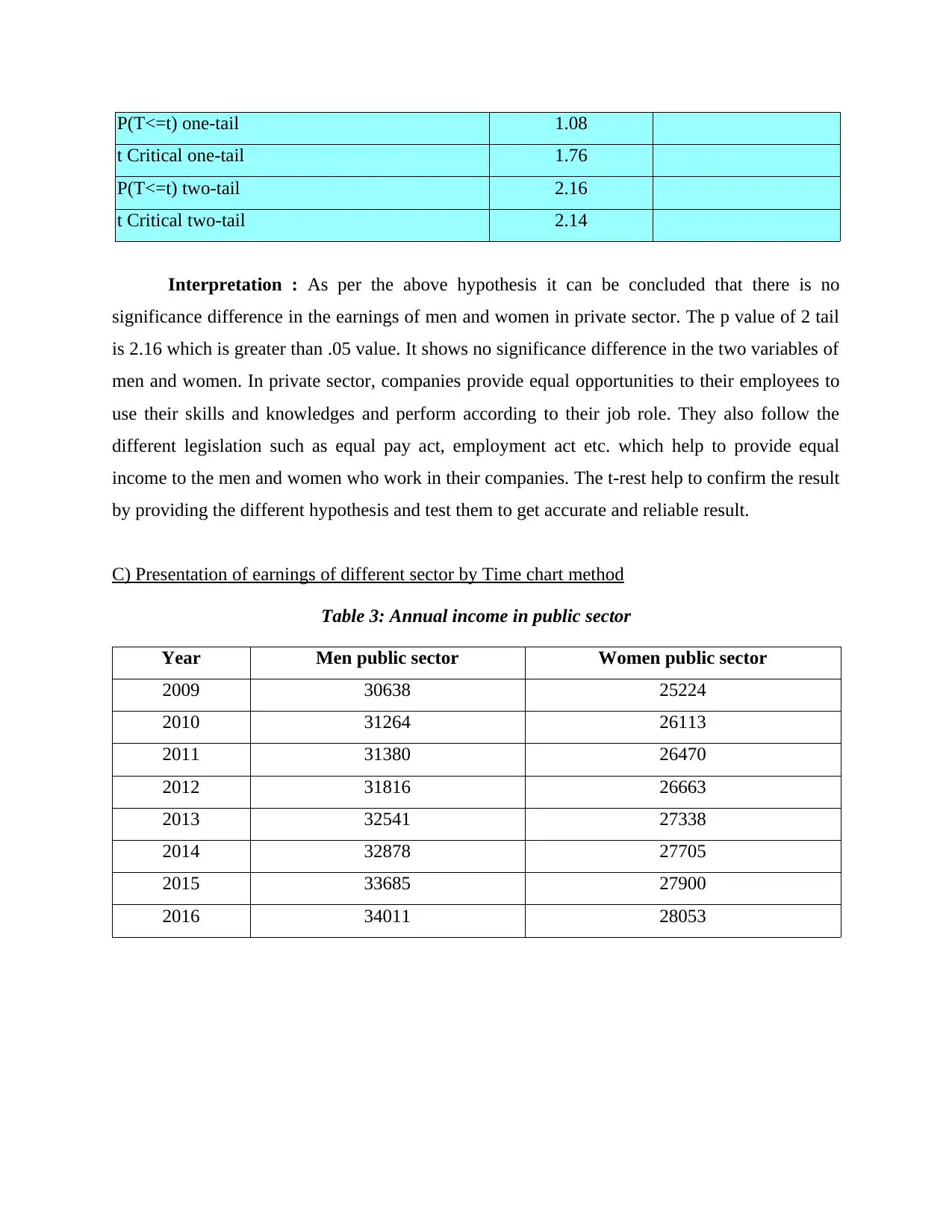
P(T<=t) one-tail 1.08
t Critical one-tail 1.76
P(T<=t) two-tail 2.16
t Critical two-tail 2.14
Interpretation : As per the above hypothesis it can be concluded that there is no
significance difference in the earnings of men and women in private sector. The p value of 2 tail
is 2.16 which is greater than .05 value. It shows no significance difference in the two variables of
men and women. In private sector, companies provide equal opportunities to their employees to
use their skills and knowledges and perform according to their job role. They also follow the
different legislation such as equal pay act, employment act etc. which help to provide equal
income to the men and women who work in their companies. The t-rest help to confirm the result
by providing the different hypothesis and test them to get accurate and reliable result.
C) Presentation of earnings of different sector by Time chart method
Table 3: Annual income in public sector
Year Men public sector Women public sector
2009 30638 25224
2010 31264 26113
2011 31380 26470
2012 31816 26663
2013 32541 27338
2014 32878 27705
2015 33685 27900
2016 34011 28053
t Critical one-tail 1.76
P(T<=t) two-tail 2.16
t Critical two-tail 2.14
Interpretation : As per the above hypothesis it can be concluded that there is no
significance difference in the earnings of men and women in private sector. The p value of 2 tail
is 2.16 which is greater than .05 value. It shows no significance difference in the two variables of
men and women. In private sector, companies provide equal opportunities to their employees to
use their skills and knowledges and perform according to their job role. They also follow the
different legislation such as equal pay act, employment act etc. which help to provide equal
income to the men and women who work in their companies. The t-rest help to confirm the result
by providing the different hypothesis and test them to get accurate and reliable result.
C) Presentation of earnings of different sector by Time chart method
Table 3: Annual income in public sector
Year Men public sector Women public sector
2009 30638 25224
2010 31264 26113
2011 31380 26470
2012 31816 26663
2013 32541 27338
2014 32878 27705
2015 33685 27900
2016 34011 28053
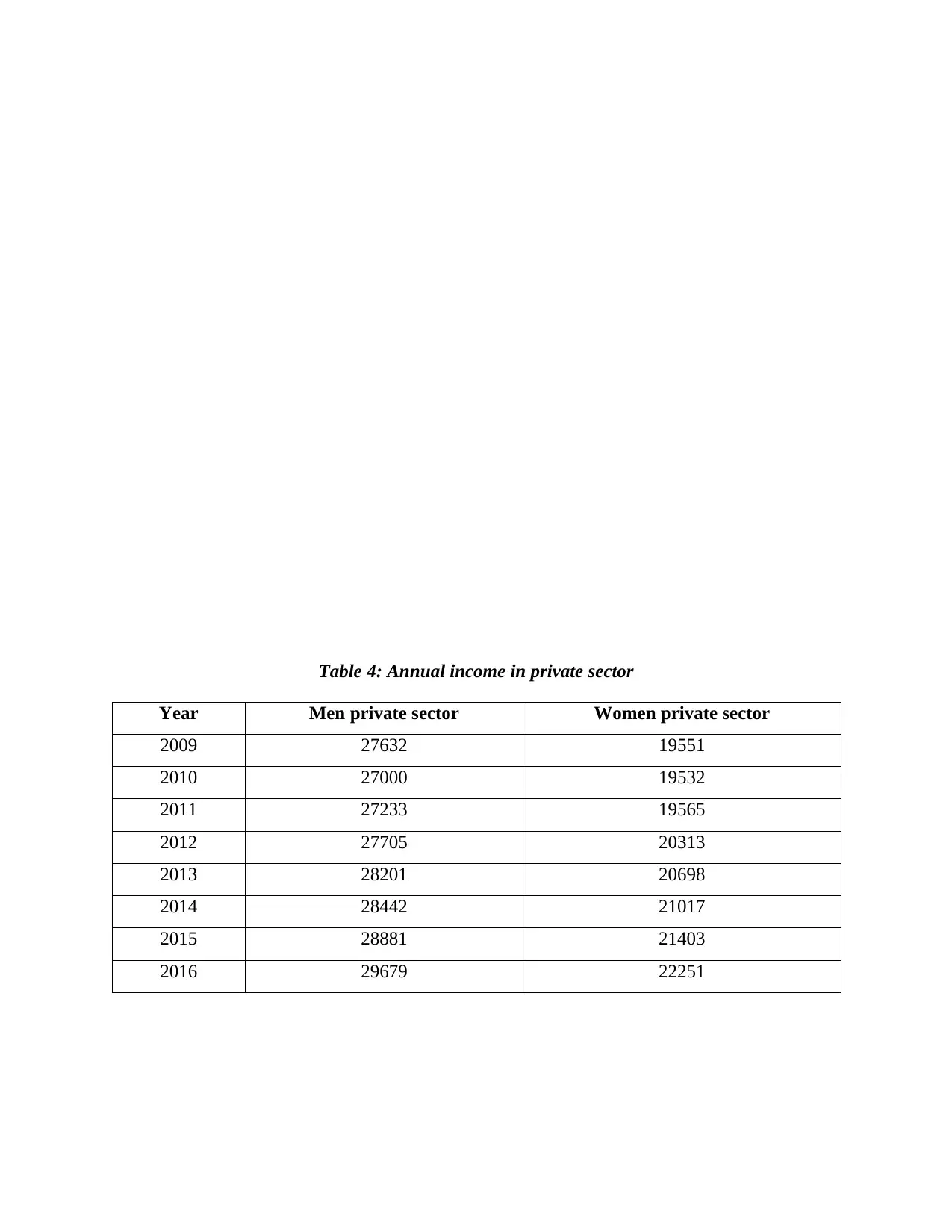
Table 4: Annual income in private sector
Year Men private sector Women private sector
2009 27632 19551
2010 27000 19532
2011 27233 19565
2012 27705 20313
2013 28201 20698
2014 28442 21017
2015 28881 21403
2016 29679 22251
Year Men private sector Women private sector
2009 27632 19551
2010 27000 19532
2011 27233 19565
2012 27705 20313
2013 28201 20698
2014 28442 21017
2015 28881 21403
2016 29679 22251
You're viewing a preview
Unlock full access by subscribing today!
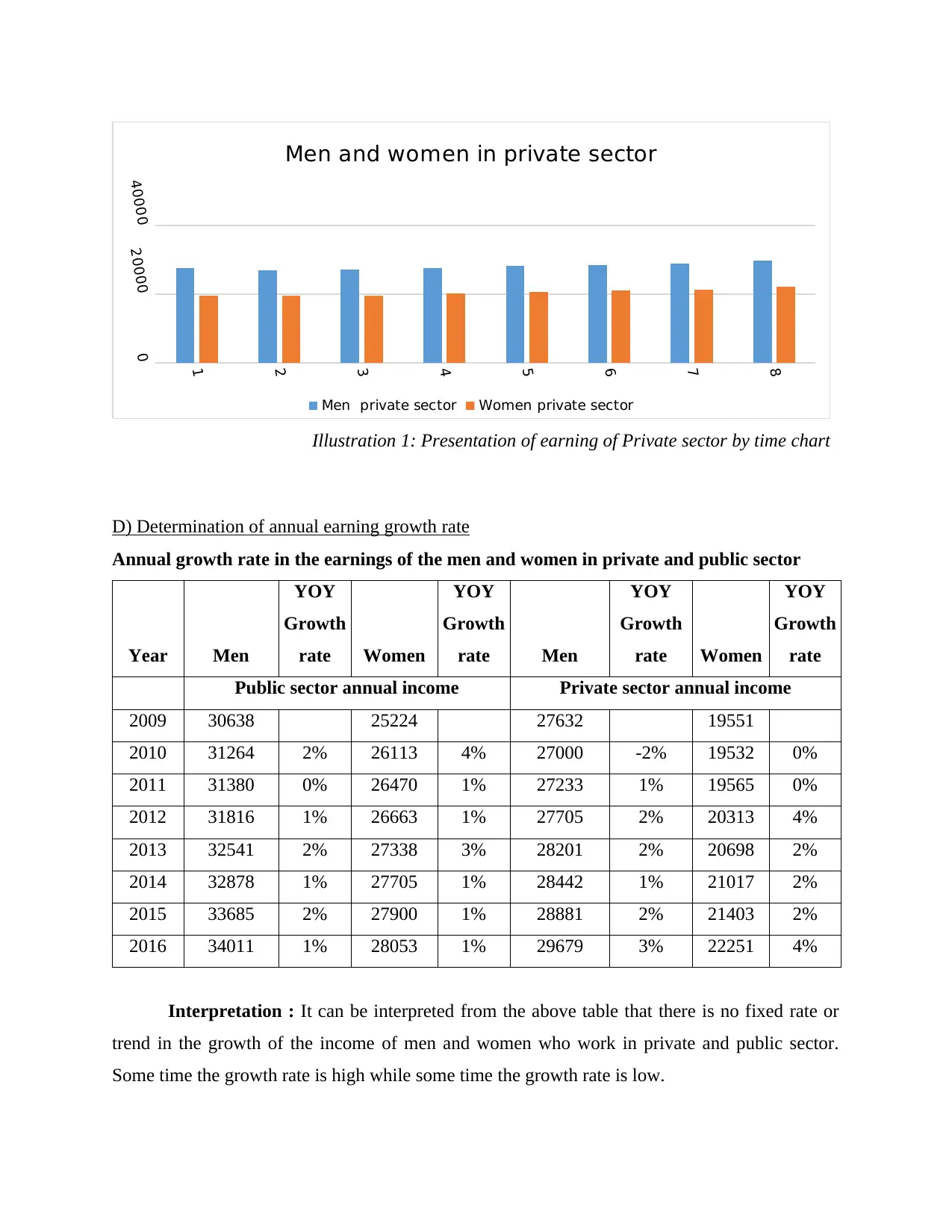
1
2
3
4
5
6
7
8
0
20000
40000
Men and women in private sector
Men private sector Women private sector
Illustration 1: Presentation of earning of Private sector by time chart
D) Determination of annual earning growth rate
Annual growth rate in the earnings of the men and women in private and public sector
Year Men
YOY
Growth
rate Women
YOY
Growth
rate Men
YOY
Growth
rate Women
YOY
Growth
rate
Public sector annual income Private sector annual income
2009 30638 25224 27632 19551
2010 31264 2% 26113 4% 27000 -2% 19532 0%
2011 31380 0% 26470 1% 27233 1% 19565 0%
2012 31816 1% 26663 1% 27705 2% 20313 4%
2013 32541 2% 27338 3% 28201 2% 20698 2%
2014 32878 1% 27705 1% 28442 1% 21017 2%
2015 33685 2% 27900 1% 28881 2% 21403 2%
2016 34011 1% 28053 1% 29679 3% 22251 4%
Interpretation : It can be interpreted from the above table that there is no fixed rate or
trend in the growth of the income of men and women who work in private and public sector.
Some time the growth rate is high while some time the growth rate is low.
2
3
4
5
6
7
8
0
20000
40000
Men and women in private sector
Men private sector Women private sector
Illustration 1: Presentation of earning of Private sector by time chart
D) Determination of annual earning growth rate
Annual growth rate in the earnings of the men and women in private and public sector
Year Men
YOY
Growth
rate Women
YOY
Growth
rate Men
YOY
Growth
rate Women
YOY
Growth
rate
Public sector annual income Private sector annual income
2009 30638 25224 27632 19551
2010 31264 2% 26113 4% 27000 -2% 19532 0%
2011 31380 0% 26470 1% 27233 1% 19565 0%
2012 31816 1% 26663 1% 27705 2% 20313 4%
2013 32541 2% 27338 3% 28201 2% 20698 2%
2014 32878 1% 27705 1% 28442 1% 21017 2%
2015 33685 2% 27900 1% 28881 2% 21403 2%
2016 34011 1% 28053 1% 29679 3% 22251 4%
Interpretation : It can be interpreted from the above table that there is no fixed rate or
trend in the growth of the income of men and women who work in private and public sector.
Some time the growth rate is high while some time the growth rate is low.
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
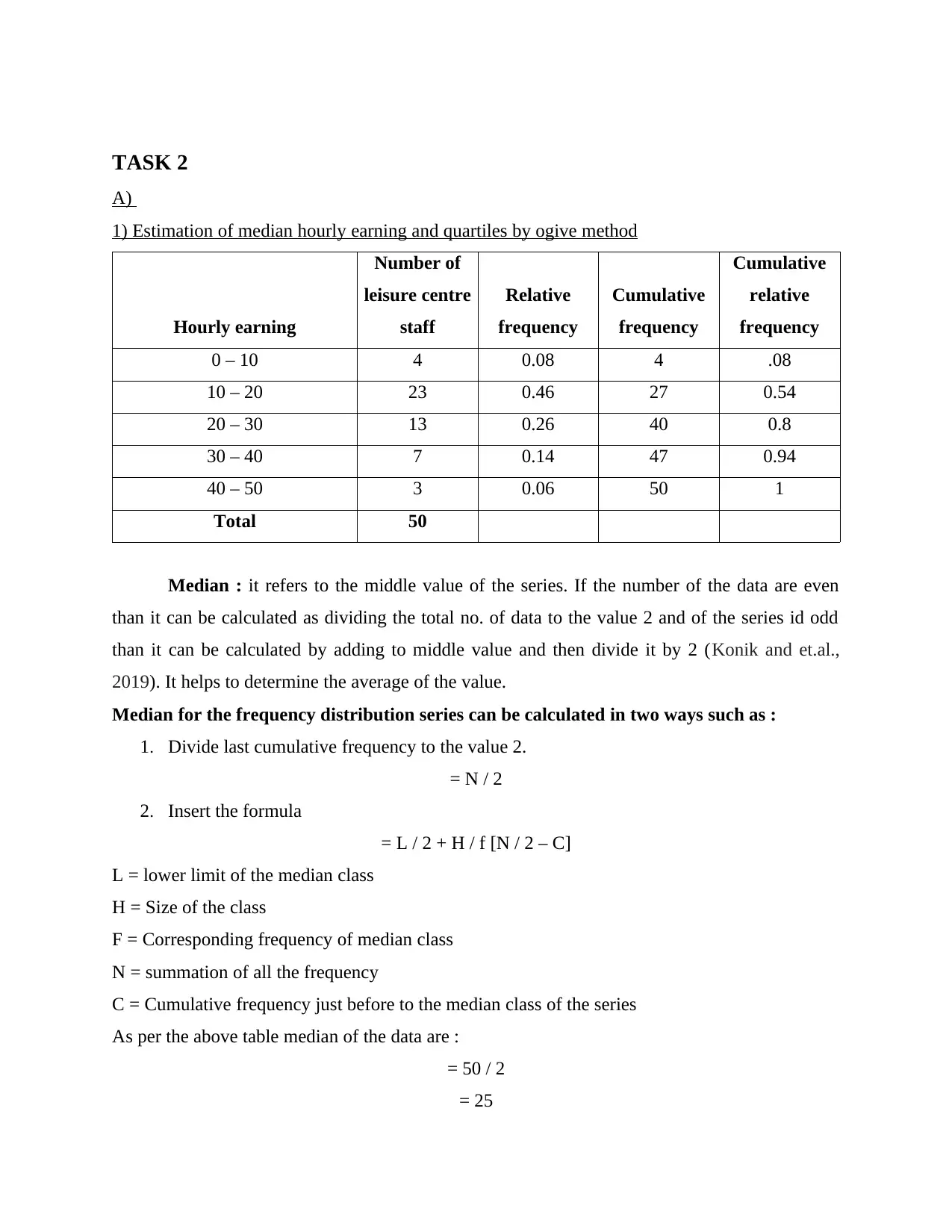
TASK 2
A)
1) Estimation of median hourly earning and quartiles by ogive method
Hourly earning
Number of
leisure centre
staff
Relative
frequency
Cumulative
frequency
Cumulative
relative
frequency
0 – 10 4 0.08 4 .08
10 – 20 23 0.46 27 0.54
20 – 30 13 0.26 40 0.8
30 – 40 7 0.14 47 0.94
40 – 50 3 0.06 50 1
Total 50
Median : it refers to the middle value of the series. If the number of the data are even
than it can be calculated as dividing the total no. of data to the value 2 and of the series id odd
than it can be calculated by adding to middle value and then divide it by 2 (Konik and et.al.,
2019). It helps to determine the average of the value.
Median for the frequency distribution series can be calculated in two ways such as :
1. Divide last cumulative frequency to the value 2.
= N / 2
2. Insert the formula
= L / 2 + H / f [N / 2 – C]
L = lower limit of the median class
H = Size of the class
F = Corresponding frequency of median class
N = summation of all the frequency
C = Cumulative frequency just before to the median class of the series
As per the above table median of the data are :
= 50 / 2
= 25
A)
1) Estimation of median hourly earning and quartiles by ogive method
Hourly earning
Number of
leisure centre
staff
Relative
frequency
Cumulative
frequency
Cumulative
relative
frequency
0 – 10 4 0.08 4 .08
10 – 20 23 0.46 27 0.54
20 – 30 13 0.26 40 0.8
30 – 40 7 0.14 47 0.94
40 – 50 3 0.06 50 1
Total 50
Median : it refers to the middle value of the series. If the number of the data are even
than it can be calculated as dividing the total no. of data to the value 2 and of the series id odd
than it can be calculated by adding to middle value and then divide it by 2 (Konik and et.al.,
2019). It helps to determine the average of the value.
Median for the frequency distribution series can be calculated in two ways such as :
1. Divide last cumulative frequency to the value 2.
= N / 2
2. Insert the formula
= L / 2 + H / f [N / 2 – C]
L = lower limit of the median class
H = Size of the class
F = Corresponding frequency of median class
N = summation of all the frequency
C = Cumulative frequency just before to the median class of the series
As per the above table median of the data are :
= 50 / 2
= 25
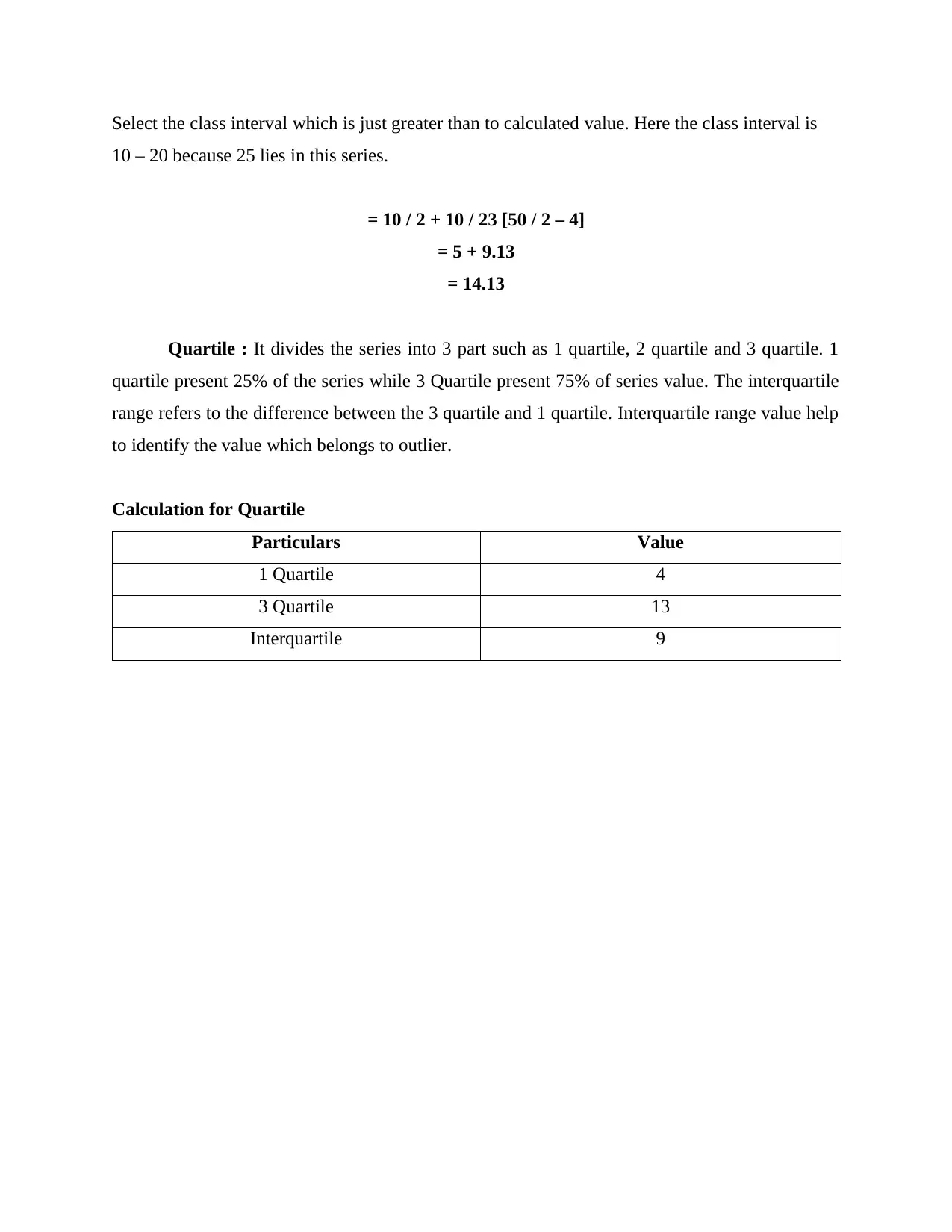
Select the class interval which is just greater than to calculated value. Here the class interval is
10 – 20 because 25 lies in this series.
= 10 / 2 + 10 / 23 [50 / 2 – 4]
= 5 + 9.13
= 14.13
Quartile : It divides the series into 3 part such as 1 quartile, 2 quartile and 3 quartile. 1
quartile present 25% of the series while 3 Quartile present 75% of series value. The interquartile
range refers to the difference between the 3 quartile and 1 quartile. Interquartile range value help
to identify the value which belongs to outlier.
Calculation for Quartile
Particulars Value
1 Quartile 4
3 Quartile 13
Interquartile 9
10 – 20 because 25 lies in this series.
= 10 / 2 + 10 / 23 [50 / 2 – 4]
= 5 + 9.13
= 14.13
Quartile : It divides the series into 3 part such as 1 quartile, 2 quartile and 3 quartile. 1
quartile present 25% of the series while 3 Quartile present 75% of series value. The interquartile
range refers to the difference between the 3 quartile and 1 quartile. Interquartile range value help
to identify the value which belongs to outlier.
Calculation for Quartile
Particulars Value
1 Quartile 4
3 Quartile 13
Interquartile 9
You're viewing a preview
Unlock full access by subscribing today!
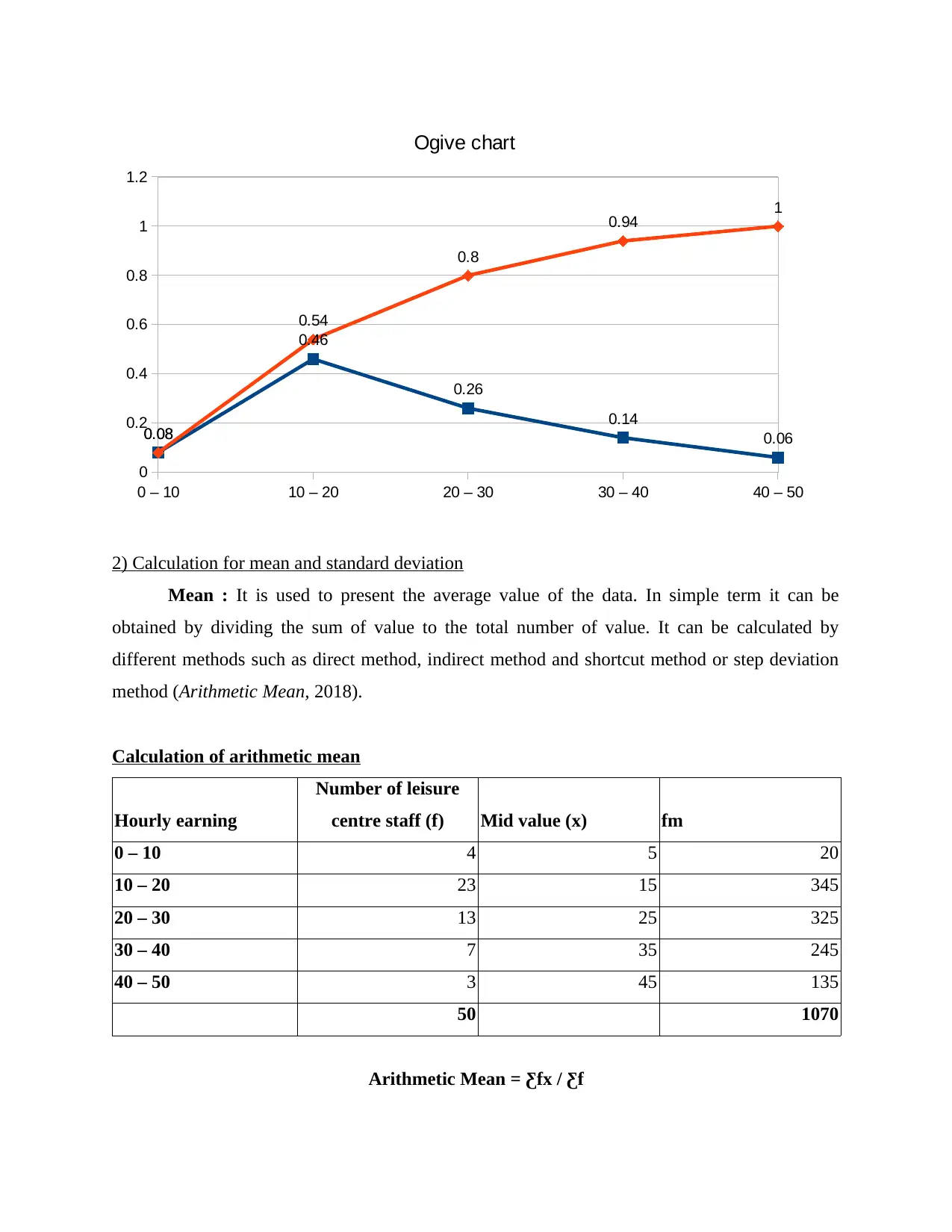
0 – 10 10 – 20 20 – 30 30 – 40 40 – 50
0
0.2
0.4
0.6
0.8
1
1.2
0.08
0.46
0.26
0.14
0.060.08
0.54
0.8
0.94 1
Ogive chart
2) Calculation for mean and standard deviation
Mean : It is used to present the average value of the data. In simple term it can be
obtained by dividing the sum of value to the total number of value. It can be calculated by
different methods such as direct method, indirect method and shortcut method or step deviation
method (Arithmetic Mean, 2018).
Calculation of arithmetic mean
Hourly earning
Number of leisure
centre staff (f) Mid value (x) fm
0 – 10 4 5 20
10 – 20 23 15 345
20 – 30 13 25 325
30 – 40 7 35 245
40 – 50 3 45 135
50 1070
Arithmetic Mean = Ƹfx / Ƹf
0
0.2
0.4
0.6
0.8
1
1.2
0.08
0.46
0.26
0.14
0.060.08
0.54
0.8
0.94 1
Ogive chart
2) Calculation for mean and standard deviation
Mean : It is used to present the average value of the data. In simple term it can be
obtained by dividing the sum of value to the total number of value. It can be calculated by
different methods such as direct method, indirect method and shortcut method or step deviation
method (Arithmetic Mean, 2018).
Calculation of arithmetic mean
Hourly earning
Number of leisure
centre staff (f) Mid value (x) fm
0 – 10 4 5 20
10 – 20 23 15 345
20 – 30 13 25 325
30 – 40 7 35 245
40 – 50 3 45 135
50 1070
Arithmetic Mean = Ƹfx / Ƹf
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
= 1070 / 50
= 21.4
Calculation of standard deviation
Standard deviation : It is used to interpret the relation with the average or arithmetic
number. In simple term it present the deviation of value from the average. If the standard
deviation is low than the spread from the mean is minimum but if the standard deviation is
higher than the deviation or spread from the average is high (Statistics - Standard Deviation of
Discrete Data Series, 2019).
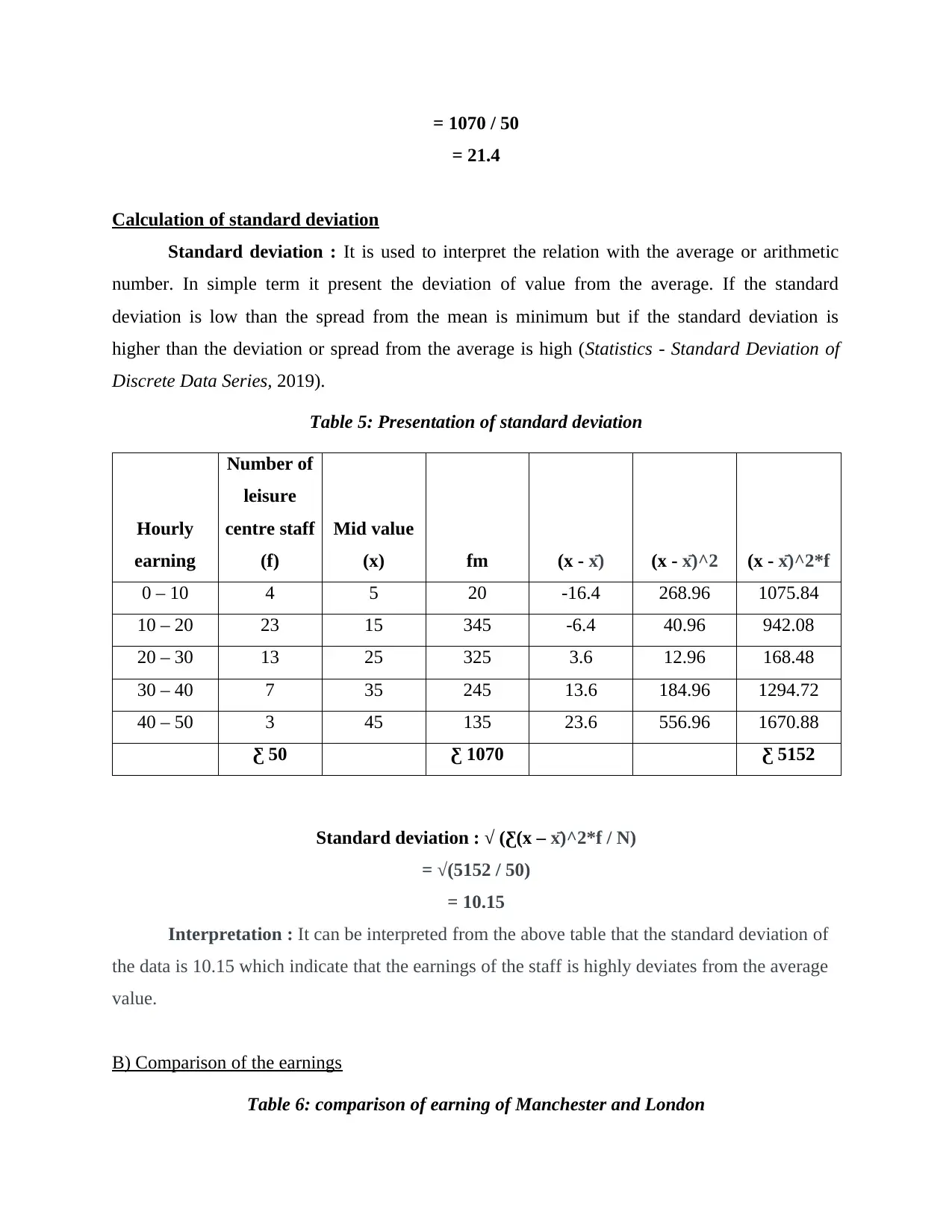
Table 5: Presentation of standard deviation
Hourly
earning
Number of
leisure
centre staff
(f)
Mid value
(x) fm (x - x̄) (x - x̄)^2 (x - x̄)^2*f
0 – 10 4 5 20 -16.4 268.96 1075.84
10 – 20 23 15 345 -6.4 40.96 942.08
20 – 30 13 25 325 3.6 12.96 168.48
30 – 40 7 35 245 13.6 184.96 1294.72
40 – 50 3 45 135 23.6 556.96 1670.88
Ƹ 50 Ƹ 1070 Ƹ 5152
Standard deviation : √ (Ƹ(x – x̄)^2*f / N)
= √(5152 / 50)
= 10.15
Interpretation : It can be interpreted from the above table that the standard deviation of
the data is 10.15 which indicate that the earnings of the staff is highly deviates from the average
value.
B) Comparison of the earnings
Table 6: comparison of earning of Manchester and London
= 21.4
Calculation of standard deviation
Standard deviation : It is used to interpret the relation with the average or arithmetic
number. In simple term it present the deviation of value from the average. If the standard
deviation is low than the spread from the mean is minimum but if the standard deviation is
higher than the deviation or spread from the average is high (Statistics - Standard Deviation of
Discrete Data Series, 2019).
Table 5: Presentation of standard deviation
Hourly
earning
Number of
leisure
centre staff
(f)
Mid value
(x) fm (x - x̄) (x - x̄)^2 (x - x̄)^2*f
0 – 10 4 5 20 -16.4 268.96 1075.84
10 – 20 23 15 345 -6.4 40.96 942.08
20 – 30 13 25 325 3.6 12.96 168.48
30 – 40 7 35 245 13.6 184.96 1294.72
40 – 50 3 45 135 23.6 556.96 1670.88
Ƹ 50 Ƹ 1070 Ƹ 5152
Standard deviation : √ (Ƹ(x – x̄)^2*f / N)
= √(5152 / 50)
= 10.15
Interpretation : It can be interpreted from the above table that the standard deviation of
the data is 10.15 which indicate that the earnings of the staff is highly deviates from the average
value.
B) Comparison of the earnings
Table 6: comparison of earning of Manchester and London
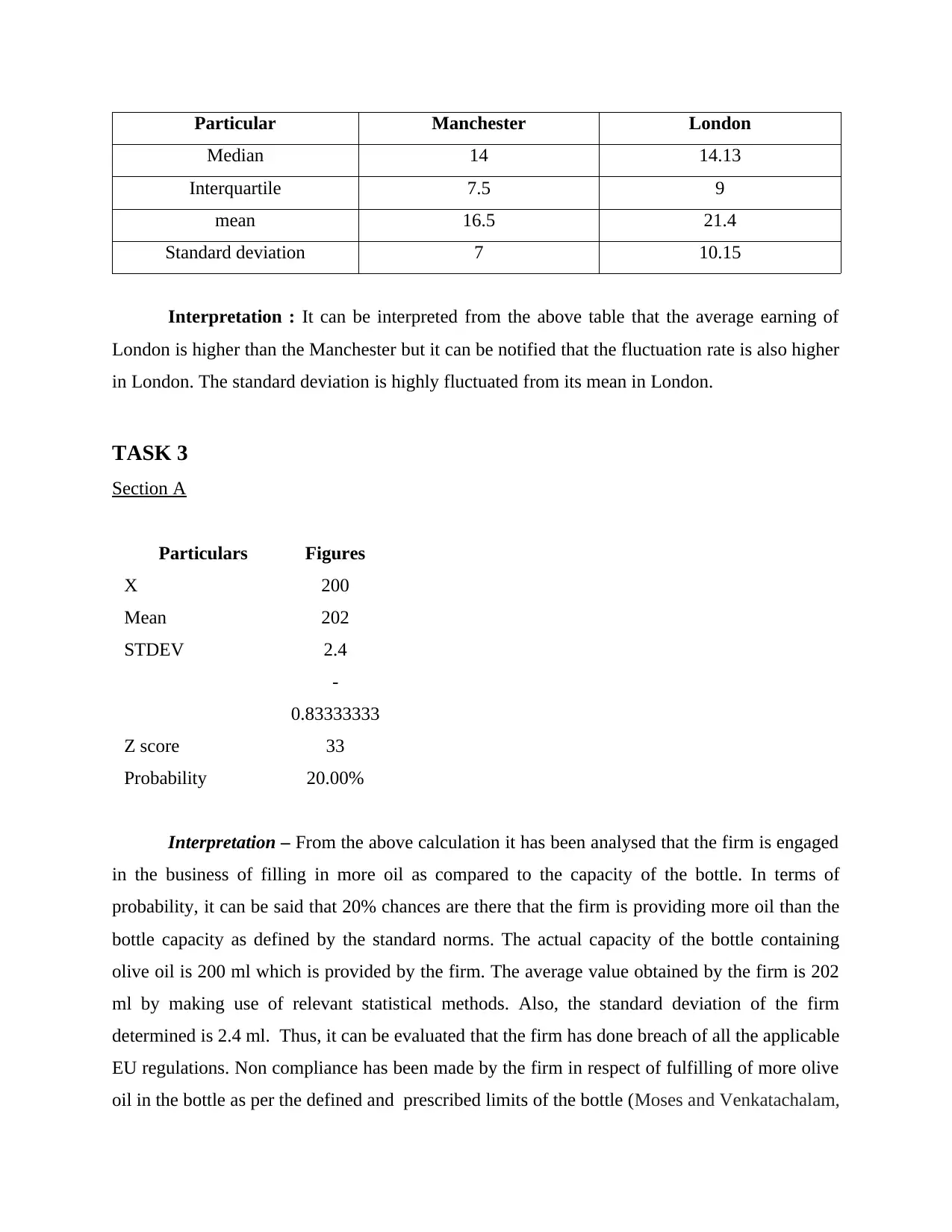
Particular Manchester London
Median 14 14.13
Interquartile 7.5 9
mean 16.5 21.4
Standard deviation 7 10.15
Interpretation : It can be interpreted from the above table that the average earning of
London is higher than the Manchester but it can be notified that the fluctuation rate is also higher
in London. The standard deviation is highly fluctuated from its mean in London.
TASK 3
Section A
Particulars Figures
X 200
Mean 202
STDEV 2.4
Z score
-
0.83333333
33
Probability 20.00%
Interpretation – From the above calculation it has been analysed that the firm is engaged
in the business of filling in more oil as compared to the capacity of the bottle. In terms of
probability, it can be said that 20% chances are there that the firm is providing more oil than the
bottle capacity as defined by the standard norms. The actual capacity of the bottle containing
olive oil is 200 ml which is provided by the firm. The average value obtained by the firm is 202
ml by making use of relevant statistical methods. Also, the standard deviation of the firm
determined is 2.4 ml. Thus, it can be evaluated that the firm has done breach of all the applicable
EU regulations. Non compliance has been made by the firm in respect of fulfilling of more olive
oil in the bottle as per the defined and prescribed limits of the bottle (Moses and Venkatachalam,
Median 14 14.13
Interquartile 7.5 9
mean 16.5 21.4
Standard deviation 7 10.15
Interpretation : It can be interpreted from the above table that the average earning of
London is higher than the Manchester but it can be notified that the fluctuation rate is also higher
in London. The standard deviation is highly fluctuated from its mean in London.
TASK 3
Section A
Particulars Figures
X 200
Mean 202
STDEV 2.4
Z score
-
0.83333333
33
Probability 20.00%
Interpretation – From the above calculation it has been analysed that the firm is engaged
in the business of filling in more oil as compared to the capacity of the bottle. In terms of
probability, it can be said that 20% chances are there that the firm is providing more oil than the
bottle capacity as defined by the standard norms. The actual capacity of the bottle containing
olive oil is 200 ml which is provided by the firm. The average value obtained by the firm is 202
ml by making use of relevant statistical methods. Also, the standard deviation of the firm
determined is 2.4 ml. Thus, it can be evaluated that the firm has done breach of all the applicable
EU regulations. Non compliance has been made by the firm in respect of fulfilling of more olive
oil in the bottle as per the defined and prescribed limits of the bottle (Moses and Venkatachalam,
You're viewing a preview
Unlock full access by subscribing today!
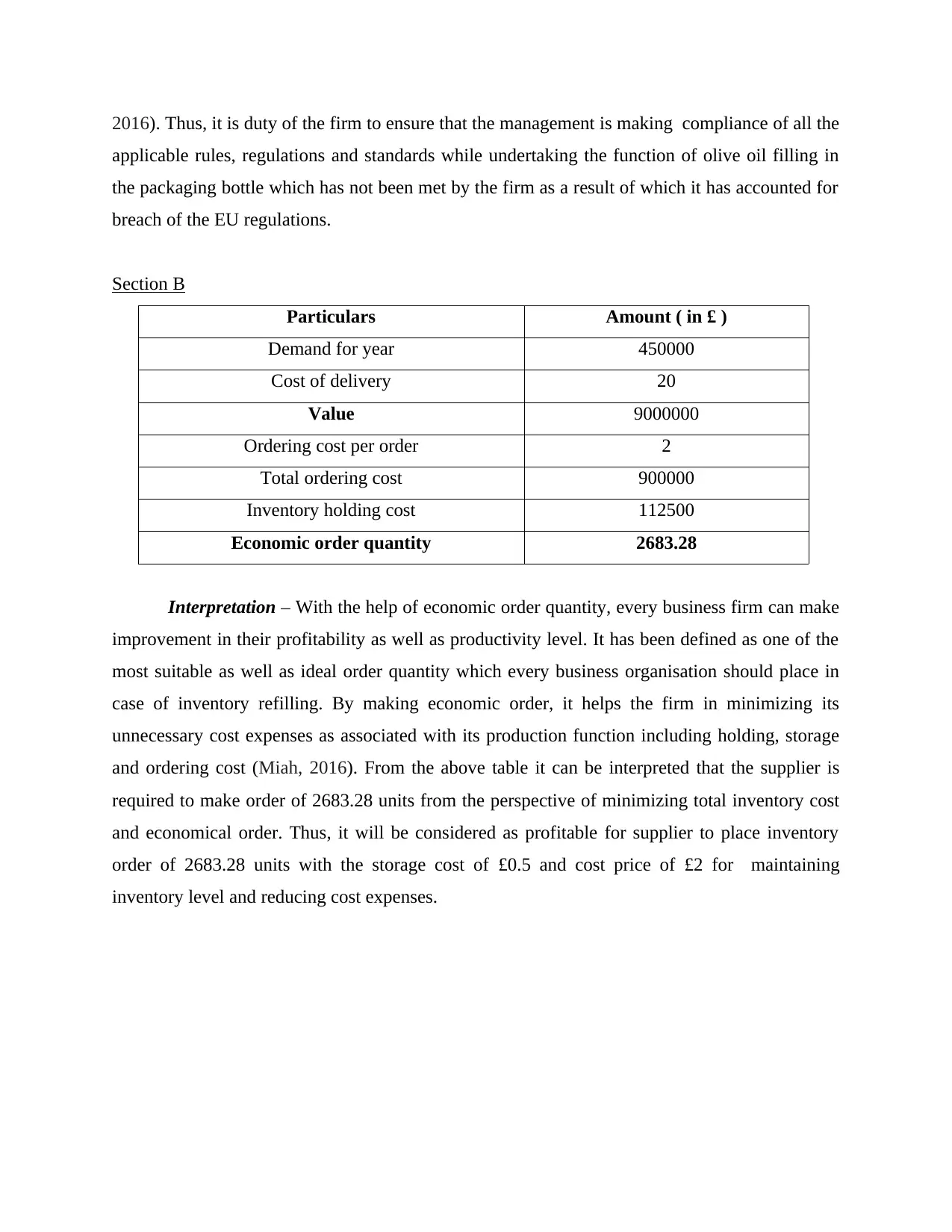
2016). Thus, it is duty of the firm to ensure that the management is making compliance of all the
applicable rules, regulations and standards while undertaking the function of olive oil filling in
the packaging bottle which has not been met by the firm as a result of which it has accounted for
breach of the EU regulations.
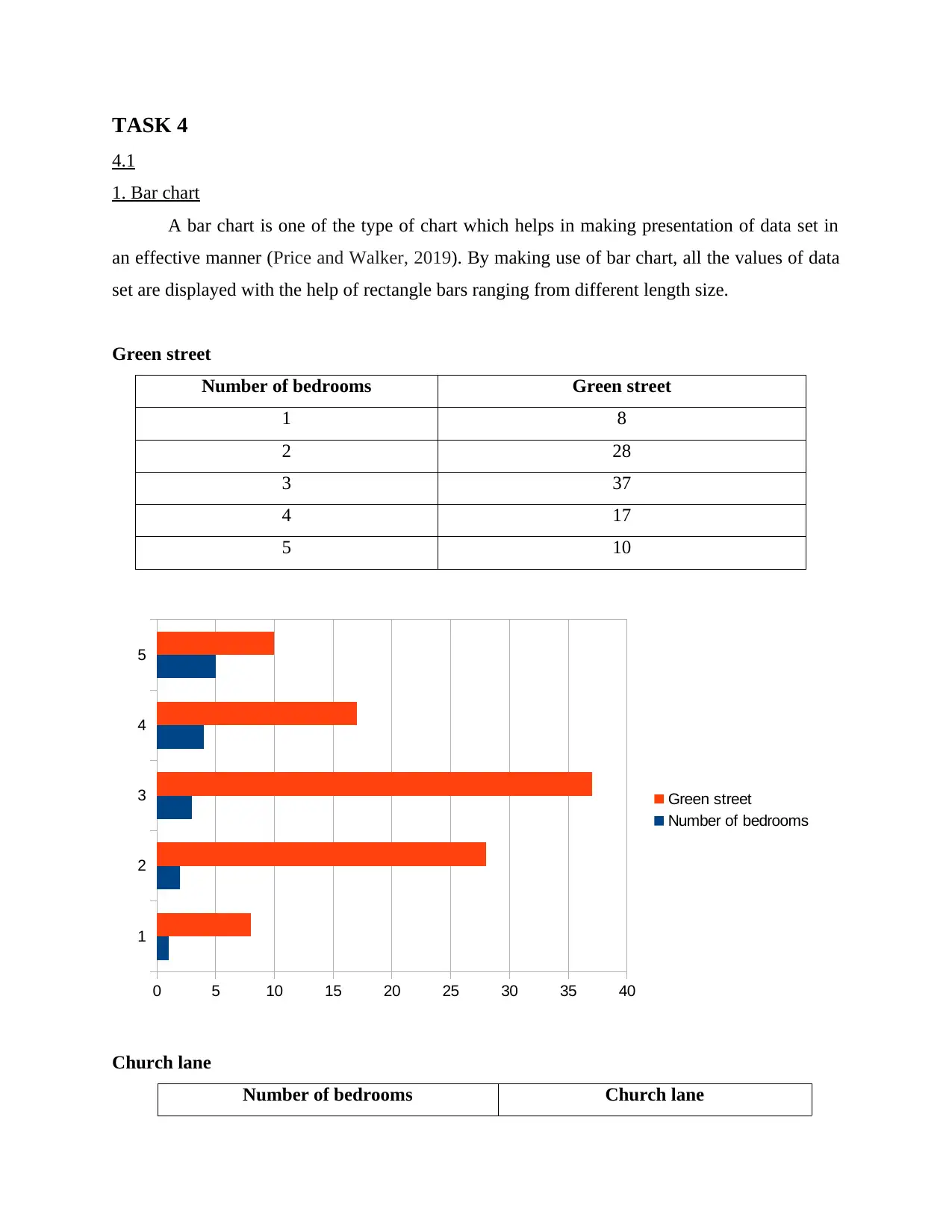
Section B
Particulars Amount ( in £ )
Demand for year 450000
Cost of delivery 20
Value 9000000
Ordering cost per order 2
Total ordering cost 900000
Inventory holding cost 112500
Economic order quantity 2683.28
Interpretation – With the help of economic order quantity, every business firm can make
improvement in their profitability as well as productivity level. It has been defined as one of the
most suitable as well as ideal order quantity which every business organisation should place in
case of inventory refilling. By making economic order, it helps the firm in minimizing its
unnecessary cost expenses as associated with its production function including holding, storage
and ordering cost (Miah, 2016). From the above table it can be interpreted that the supplier is
required to make order of 2683.28 units from the perspective of minimizing total inventory cost
and economical order. Thus, it will be considered as profitable for supplier to place inventory
order of 2683.28 units with the storage cost of £0.5 and cost price of £2 for maintaining
inventory level and reducing cost expenses.
applicable rules, regulations and standards while undertaking the function of olive oil filling in
the packaging bottle which has not been met by the firm as a result of which it has accounted for
breach of the EU regulations.
Section B
Particulars Amount ( in £ )
Demand for year 450000
Cost of delivery 20
Value 9000000
Ordering cost per order 2
Total ordering cost 900000
Inventory holding cost 112500
Economic order quantity 2683.28
Interpretation – With the help of economic order quantity, every business firm can make
improvement in their profitability as well as productivity level. It has been defined as one of the
most suitable as well as ideal order quantity which every business organisation should place in
case of inventory refilling. By making economic order, it helps the firm in minimizing its
unnecessary cost expenses as associated with its production function including holding, storage
and ordering cost (Miah, 2016). From the above table it can be interpreted that the supplier is
required to make order of 2683.28 units from the perspective of minimizing total inventory cost
and economical order. Thus, it will be considered as profitable for supplier to place inventory
order of 2683.28 units with the storage cost of £0.5 and cost price of £2 for maintaining
inventory level and reducing cost expenses.
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
TASK 4
4.1
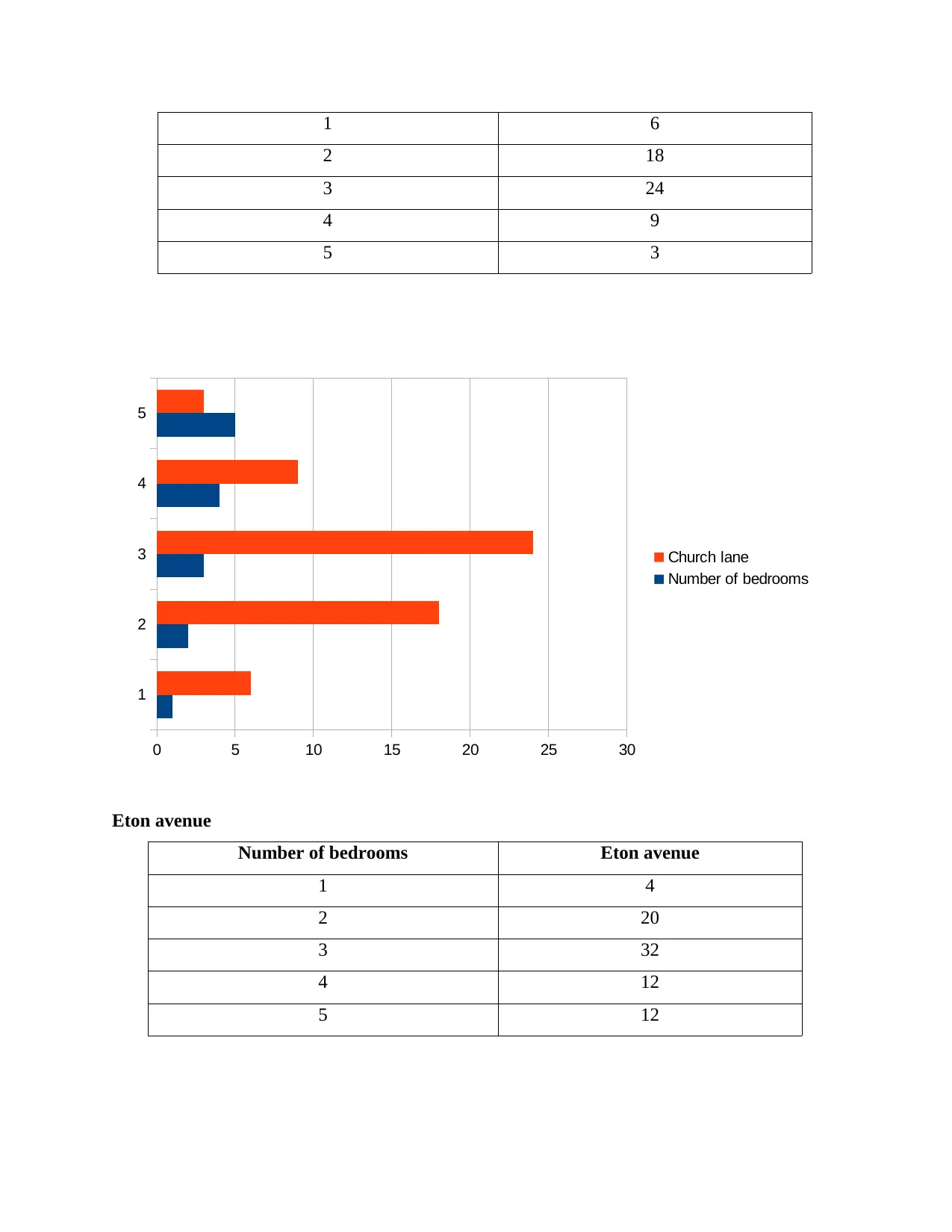
1. Bar chart
A bar chart is one of the type of chart which helps in making presentation of data set in
an effective manner (Price and Walker, 2019). By making use of bar chart, all the values of data
set are displayed with the help of rectangle bars ranging from different length size.
Green street
Number of bedrooms Green street
1 8
2 28
3 37
4 17
5 10
Church lane
Number of bedrooms Church lane
1
2
3
4
5
0 5 10 15 20 25 30 35 40
Green street
Number of bedrooms
4.1
1. Bar chart
A bar chart is one of the type of chart which helps in making presentation of data set in
an effective manner (Price and Walker, 2019). By making use of bar chart, all the values of data
set are displayed with the help of rectangle bars ranging from different length size.
Green street
Number of bedrooms Green street
1 8
2 28
3 37
4 17
5 10
Church lane
Number of bedrooms Church lane
1
2
3
4
5
0 5 10 15 20 25 30 35 40
Green street
Number of bedrooms
1 6
2 18
3 24
4 9
5 3
Eton avenue
Number of bedrooms Eton avenue
1 4
2 20
3 32
4 12
5 12
1
2
3
4
5
0 5 10 15 20 25 30
Church lane
Number of bedrooms
2 18
3 24
4 9
5 3
Eton avenue
Number of bedrooms Eton avenue
1 4
2 20
3 32
4 12
5 12
1
2
3
4
5
0 5 10 15 20 25 30
Church lane
Number of bedrooms
You're viewing a preview
Unlock full access by subscribing today!
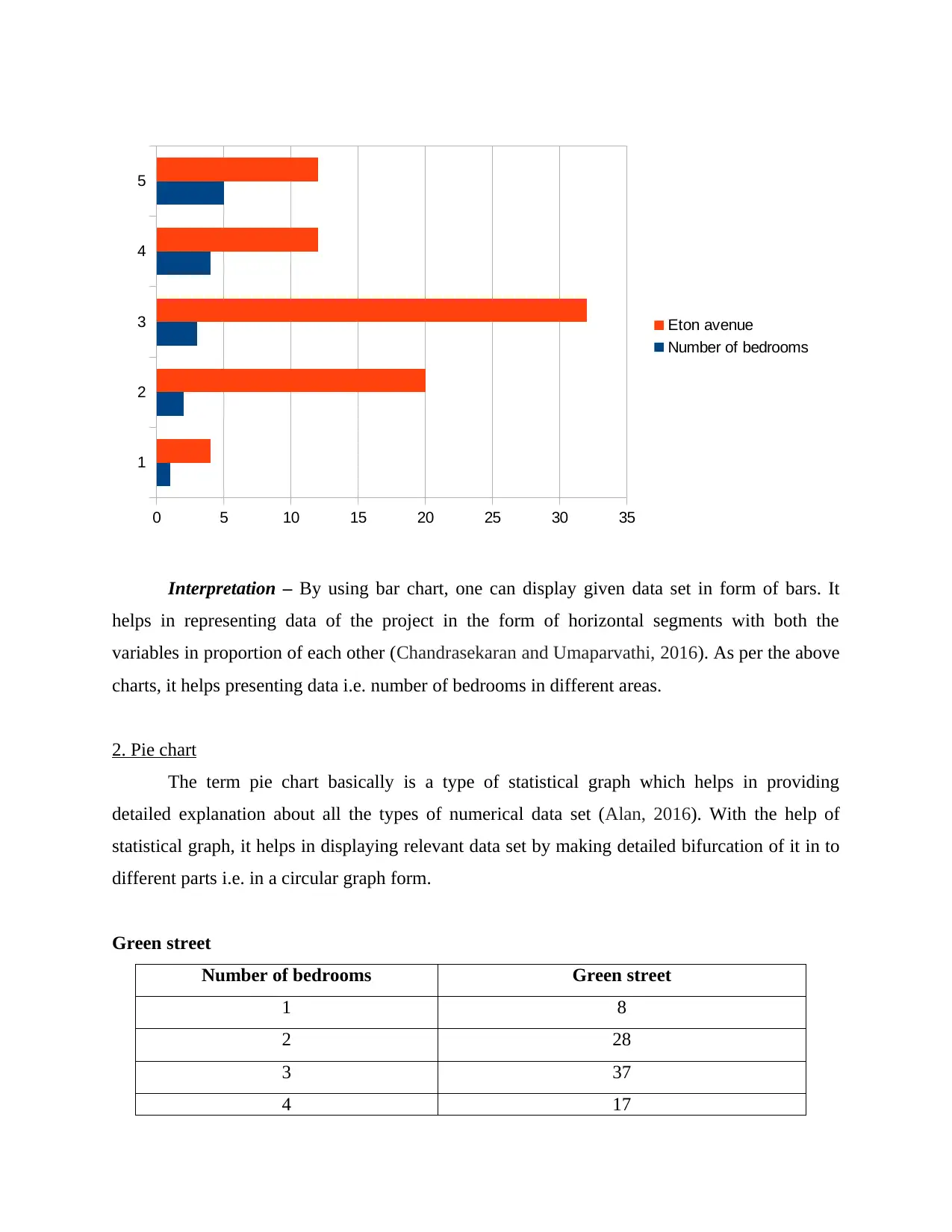
Interpretation – By using bar chart, one can display given data set in form of bars. It
helps in representing data of the project in the form of horizontal segments with both the
variables in proportion of each other (Chandrasekaran and Umaparvathi, 2016). As per the above
charts, it helps presenting data i.e. number of bedrooms in different areas.
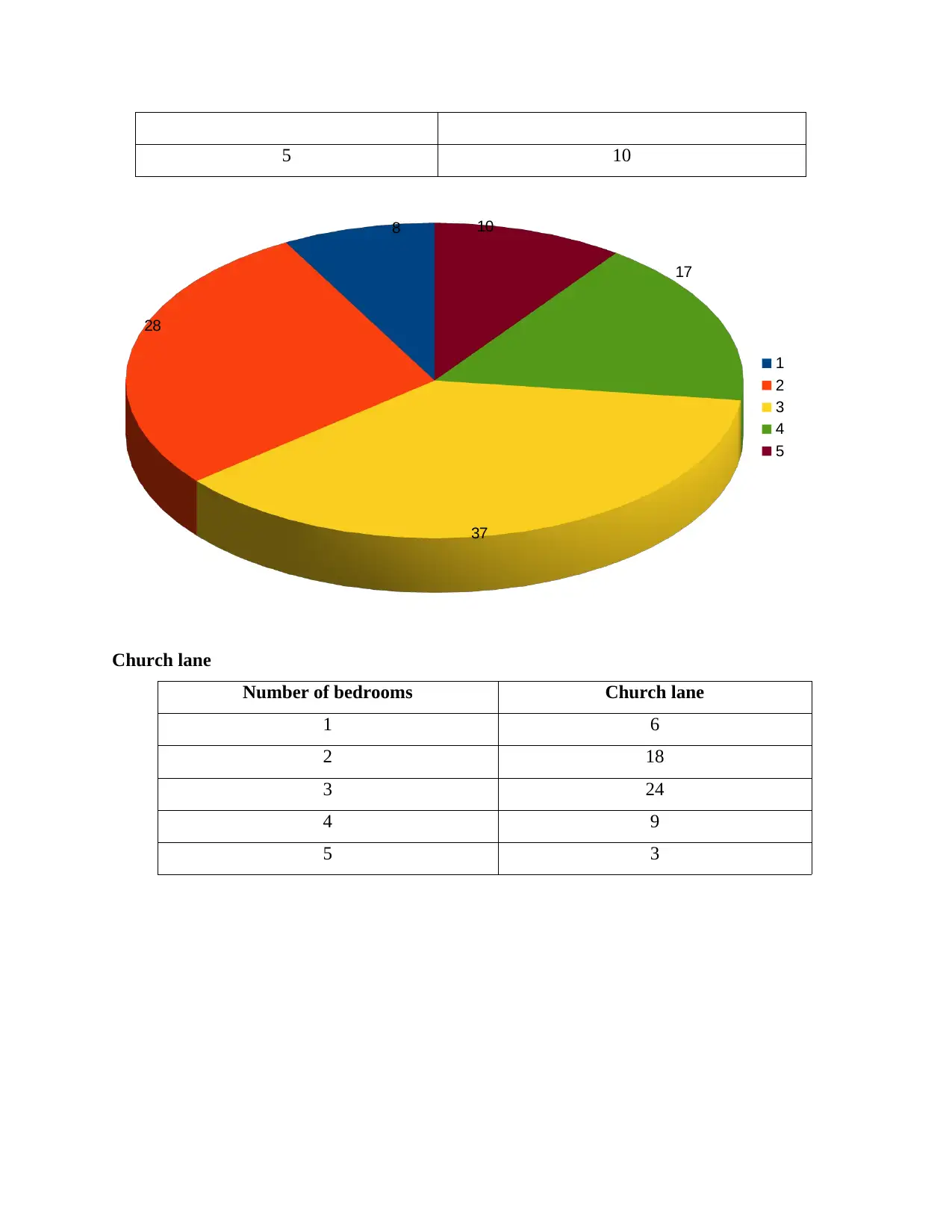
2. Pie chart
The term pie chart basically is a type of statistical graph which helps in providing
detailed explanation about all the types of numerical data set (Alan, 2016). With the help of
statistical graph, it helps in displaying relevant data set by making detailed bifurcation of it in to
different parts i.e. in a circular graph form.
Green street
Number of bedrooms Green street
1 8
2 28
3 37
4 17
1
2
3
4
5
0 5 10 15 20 25 30 35
Eton avenue
Number of bedrooms
helps in representing data of the project in the form of horizontal segments with both the
variables in proportion of each other (Chandrasekaran and Umaparvathi, 2016). As per the above
charts, it helps presenting data i.e. number of bedrooms in different areas.
2. Pie chart
The term pie chart basically is a type of statistical graph which helps in providing
detailed explanation about all the types of numerical data set (Alan, 2016). With the help of
statistical graph, it helps in displaying relevant data set by making detailed bifurcation of it in to
different parts i.e. in a circular graph form.
Green street
Number of bedrooms Green street
1 8
2 28
3 37
4 17
1
2
3
4
5
0 5 10 15 20 25 30 35
Eton avenue
Number of bedrooms
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
5 10
8
28
37
17
10
1
2
3
4
5
Church lane
Number of bedrooms Church lane
1 6
2 18
3 24
4 9
5 3
8
28
37
17
10
1
2
3
4
5
Church lane
Number of bedrooms Church lane
1 6
2 18
3 24
4 9
5 3
6
18
24
9
3
1
2
3
4
5
Eton avenue
Number of bedrooms Eton avenue
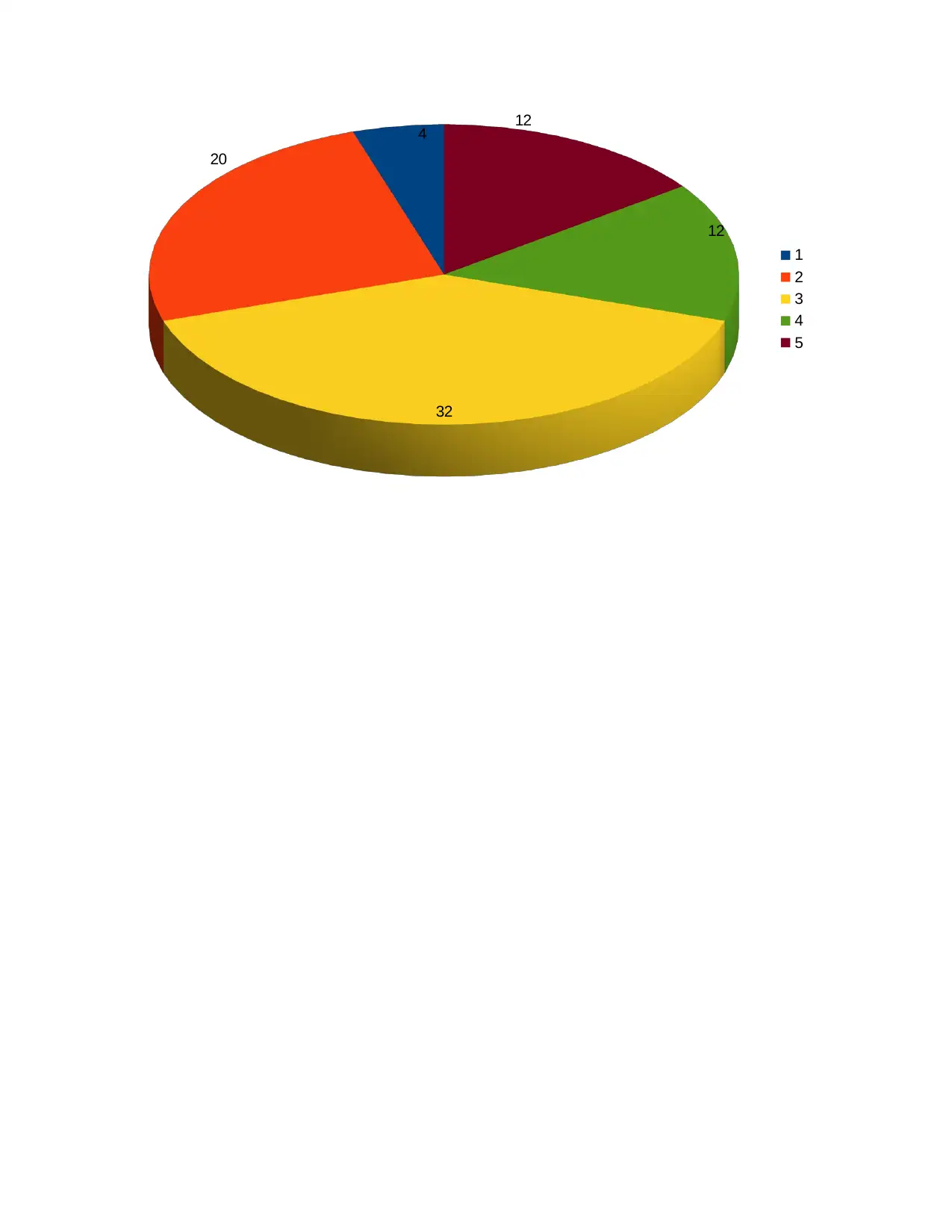
1 4
2 20
3 32
4 12
5 12
18
24
9
3
1
2
3
4
5
Eton avenue
Number of bedrooms Eton avenue
1 4
2 20
3 32
4 12
5 12
You're viewing a preview
Unlock full access by subscribing today!
4
20
32
12
12
1
2
3
4
5
20
32
12
12
1
2
3
4
5
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

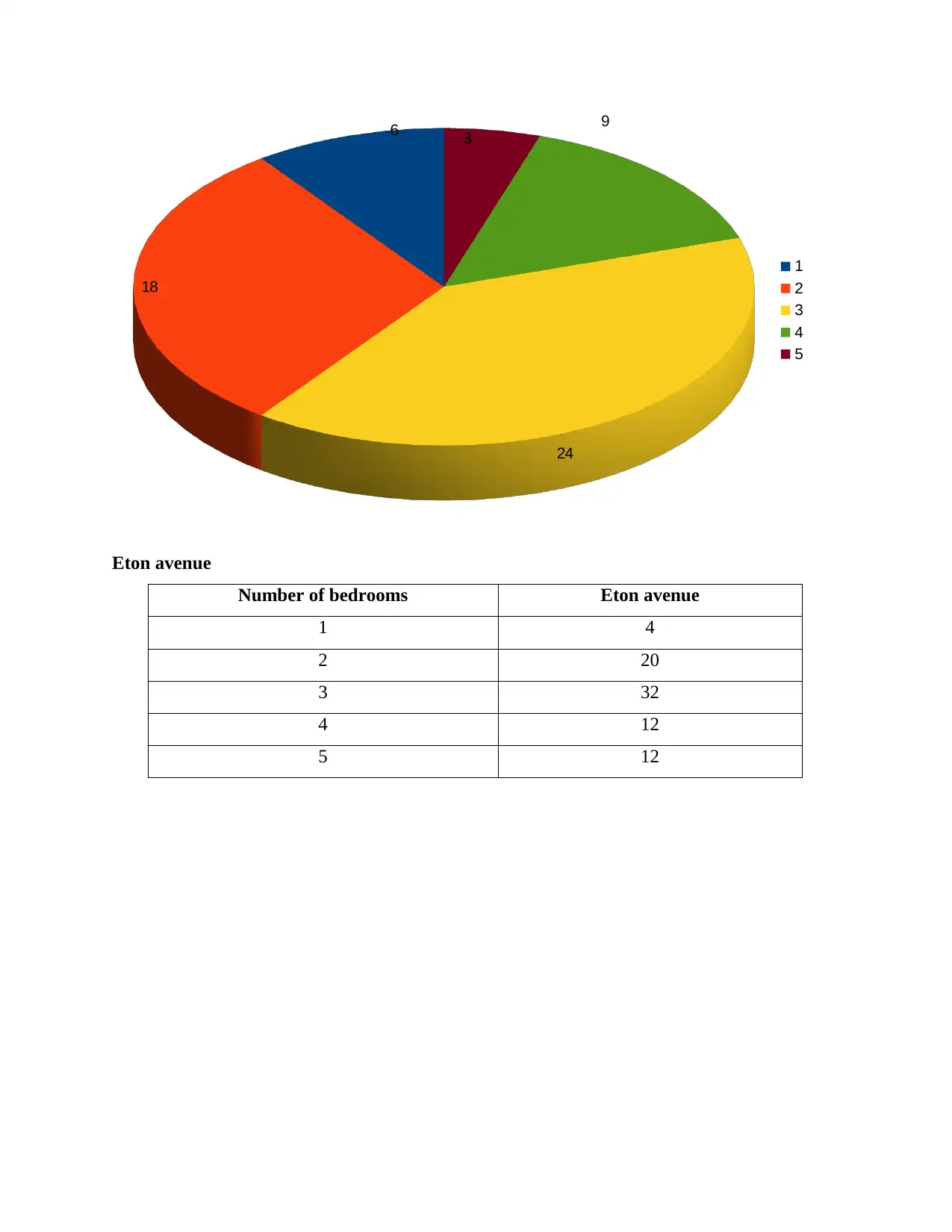
Interpretation – The above pie chart helps in understanding the statistical data in proper
manner. All the information has been divided in to number of different parts which further
depicts about numerical proportion as associated with each data set (Anderson and et.al., 2016).
With the help of pie chart, explanation related to the number of bedrooms as situated among the
different types of street areas has been provided.
4.2 Relationship between the number of bedrooms and the house price of bedrooms in all of the
three streets.
A. Relationship between 2 Number of bedrooms and house price in different streets.
1. Relationship between Price of 2 bedroom in the Green street and Church Lane
Number of
bedrooms
Green street
(price)
Church lane
(price)
2 600000 700000
Percentage change 16.67%
1.5 2 2.5 3 3.5 4
540000
560000
580000
600000
620000
640000
660000
680000
700000
720000
Green street
Church lane
manner. All the information has been divided in to number of different parts which further
depicts about numerical proportion as associated with each data set (Anderson and et.al., 2016).
With the help of pie chart, explanation related to the number of bedrooms as situated among the
different types of street areas has been provided.
4.2 Relationship between the number of bedrooms and the house price of bedrooms in all of the
three streets.
A. Relationship between 2 Number of bedrooms and house price in different streets.
1. Relationship between Price of 2 bedroom in the Green street and Church Lane
Number of
bedrooms
Green street
(price)
Church lane
(price)
2 600000 700000
Percentage change 16.67%
1.5 2 2.5 3 3.5 4
540000
560000
580000
600000
620000
640000
660000
680000
700000
720000
Green street
Church lane
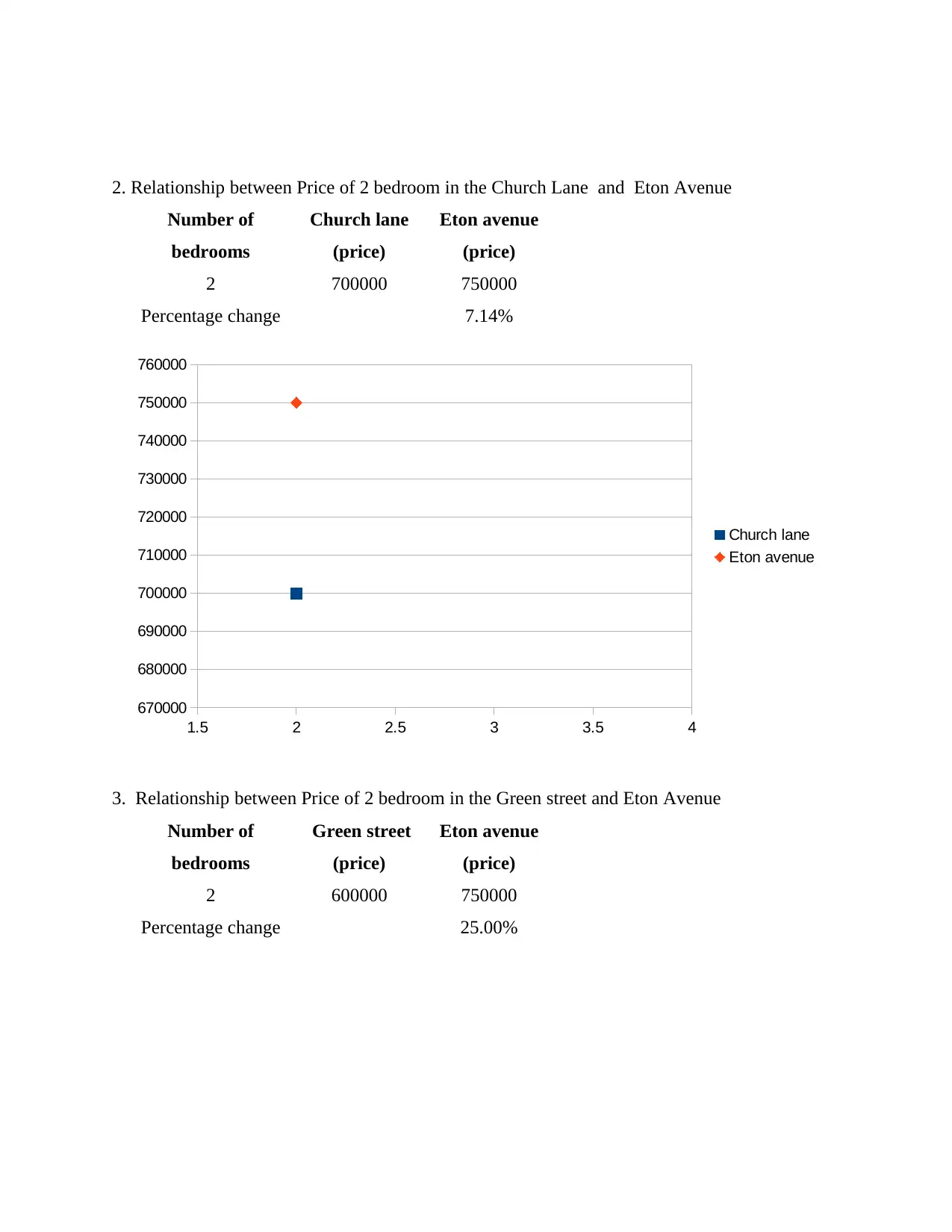
2. Relationship between Price of 2 bedroom in the Church Lane and Eton Avenue
Number of
bedrooms
Church lane
(price)
Eton avenue
(price)
2 700000 750000
Percentage change 7.14%
3. Relationship between Price of 2 bedroom in the Green street and Eton Avenue
Number of
bedrooms
Green street
(price)
Eton avenue
(price)
2 600000 750000
Percentage change 25.00%
1.5 2 2.5 3 3.5 4
670000
680000
690000
700000
710000
720000
730000
740000
750000
760000
Church lane
Eton avenue
Number of
bedrooms
Church lane
(price)
Eton avenue
(price)
2 700000 750000
Percentage change 7.14%
3. Relationship between Price of 2 bedroom in the Green street and Eton Avenue
Number of
bedrooms
Green street
(price)
Eton avenue
(price)
2 600000 750000
Percentage change 25.00%
1.5 2 2.5 3 3.5 4
670000
680000
690000
700000
710000
720000
730000
740000
750000
760000
Church lane
Eton avenue
You're viewing a preview
Unlock full access by subscribing today!
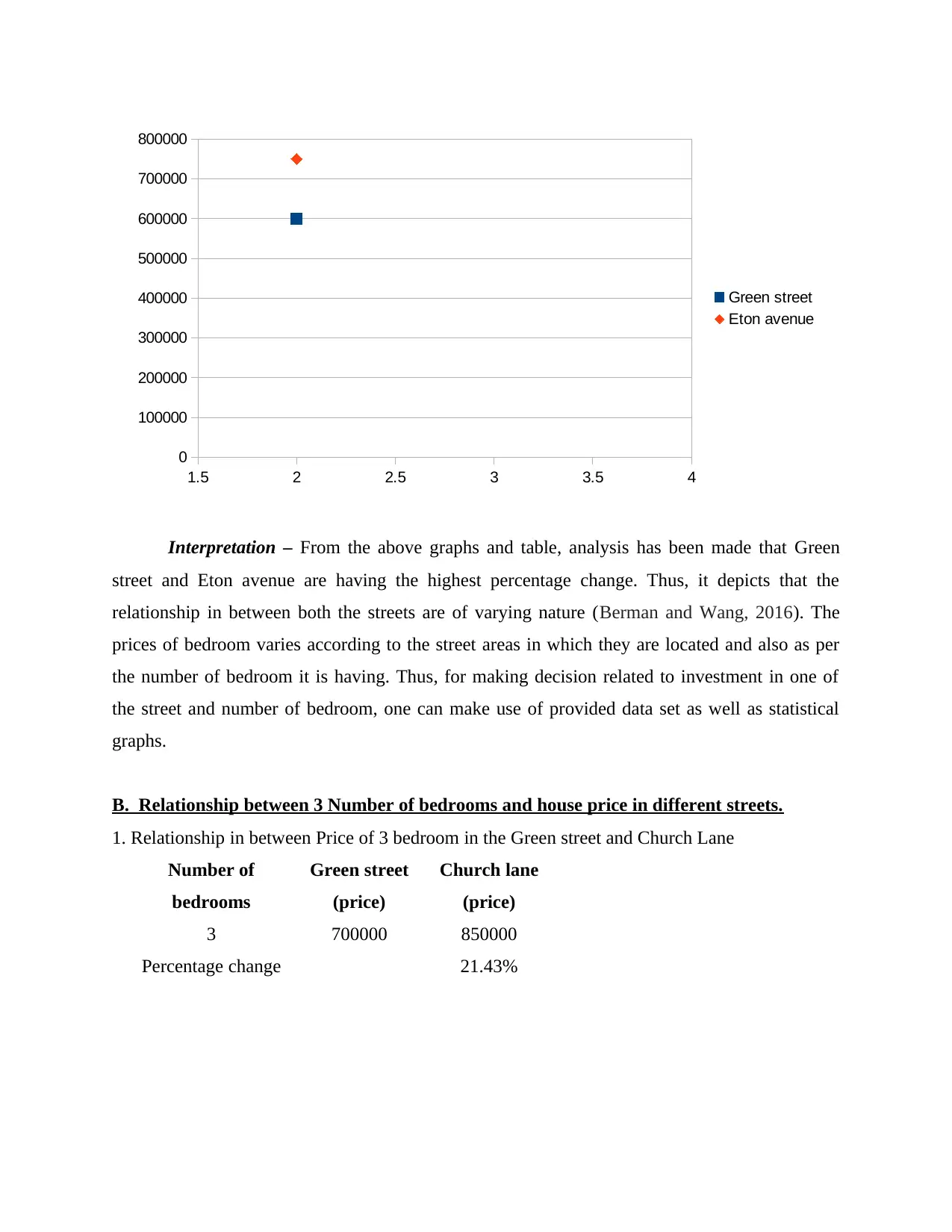
Interpretation – From the above graphs and table, analysis has been made that Green
street and Eton avenue are having the highest percentage change. Thus, it depicts that the
relationship in between both the streets are of varying nature (Berman and Wang, 2016). The
prices of bedroom varies according to the street areas in which they are located and also as per
the number of bedroom it is having. Thus, for making decision related to investment in one of
the street and number of bedroom, one can make use of provided data set as well as statistical
graphs.
B. Relationship between 3 Number of bedrooms and house price in different streets.
1. Relationship in between Price of 3 bedroom in the Green street and Church Lane
Number of
bedrooms
Green street
(price)
Church lane
(price)
3 700000 850000
Percentage change 21.43%
1.5 2 2.5 3 3.5 4
0
100000
200000
300000
400000
500000
600000
700000
800000
Green street
Eton avenue
street and Eton avenue are having the highest percentage change. Thus, it depicts that the
relationship in between both the streets are of varying nature (Berman and Wang, 2016). The
prices of bedroom varies according to the street areas in which they are located and also as per
the number of bedroom it is having. Thus, for making decision related to investment in one of
the street and number of bedroom, one can make use of provided data set as well as statistical
graphs.
B. Relationship between 3 Number of bedrooms and house price in different streets.
1. Relationship in between Price of 3 bedroom in the Green street and Church Lane
Number of
bedrooms
Green street
(price)
Church lane
(price)
3 700000 850000
Percentage change 21.43%
1.5 2 2.5 3 3.5 4
0
100000
200000
300000
400000
500000
600000
700000
800000
Green street
Eton avenue
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
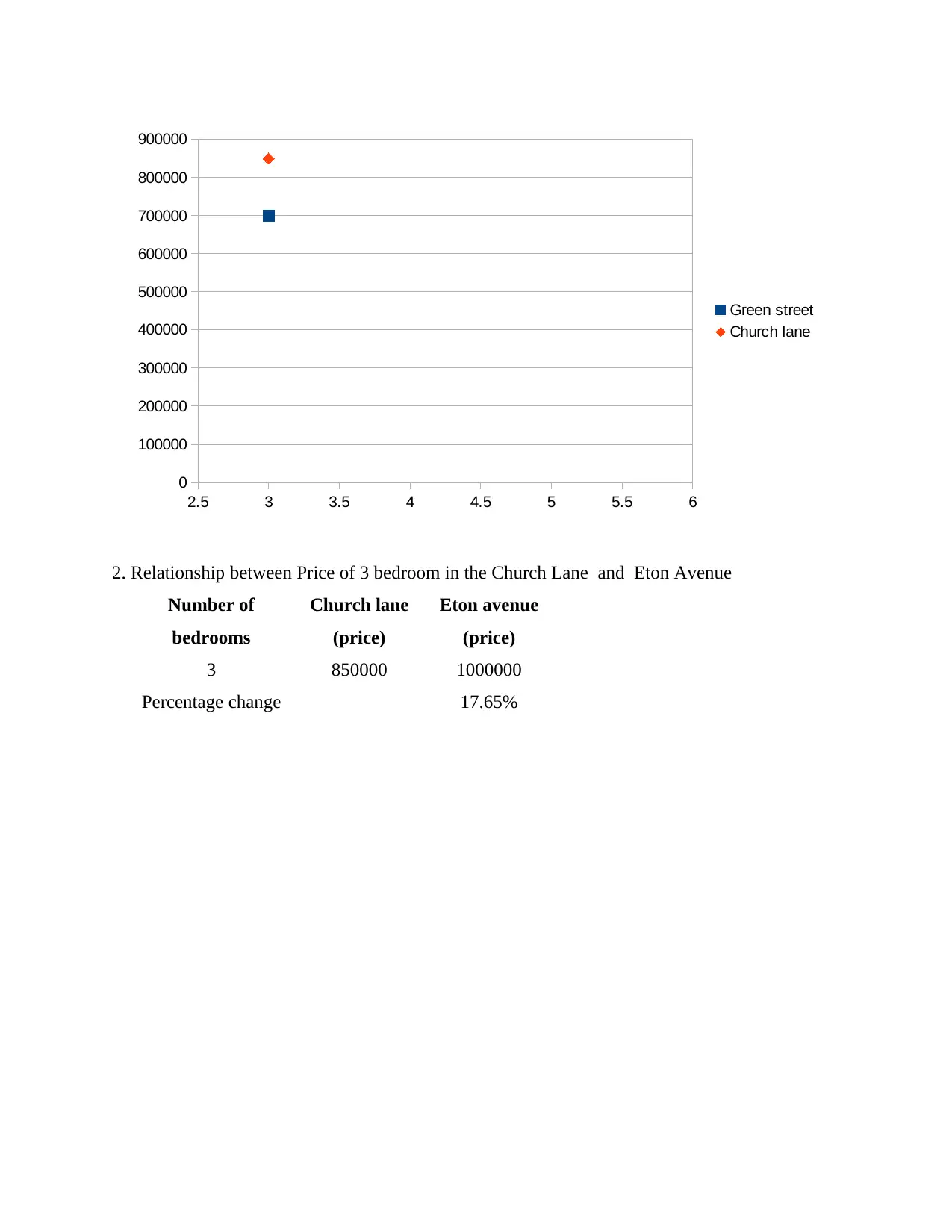
2. Relationship between Price of 3 bedroom in the Church Lane and Eton Avenue
Number of
bedrooms
Church lane
(price)
Eton avenue
(price)
3 850000 1000000
Percentage change 17.65%
2.5 3 3.5 4 4.5 5 5.5 6
0
100000
200000
300000
400000
500000
600000
700000
800000
900000
Green street
Church lane
Number of
bedrooms
Church lane
(price)
Eton avenue
(price)
3 850000 1000000
Percentage change 17.65%
2.5 3 3.5 4 4.5 5 5.5 6
0
100000
200000
300000
400000
500000
600000
700000
800000
900000
Green street
Church lane
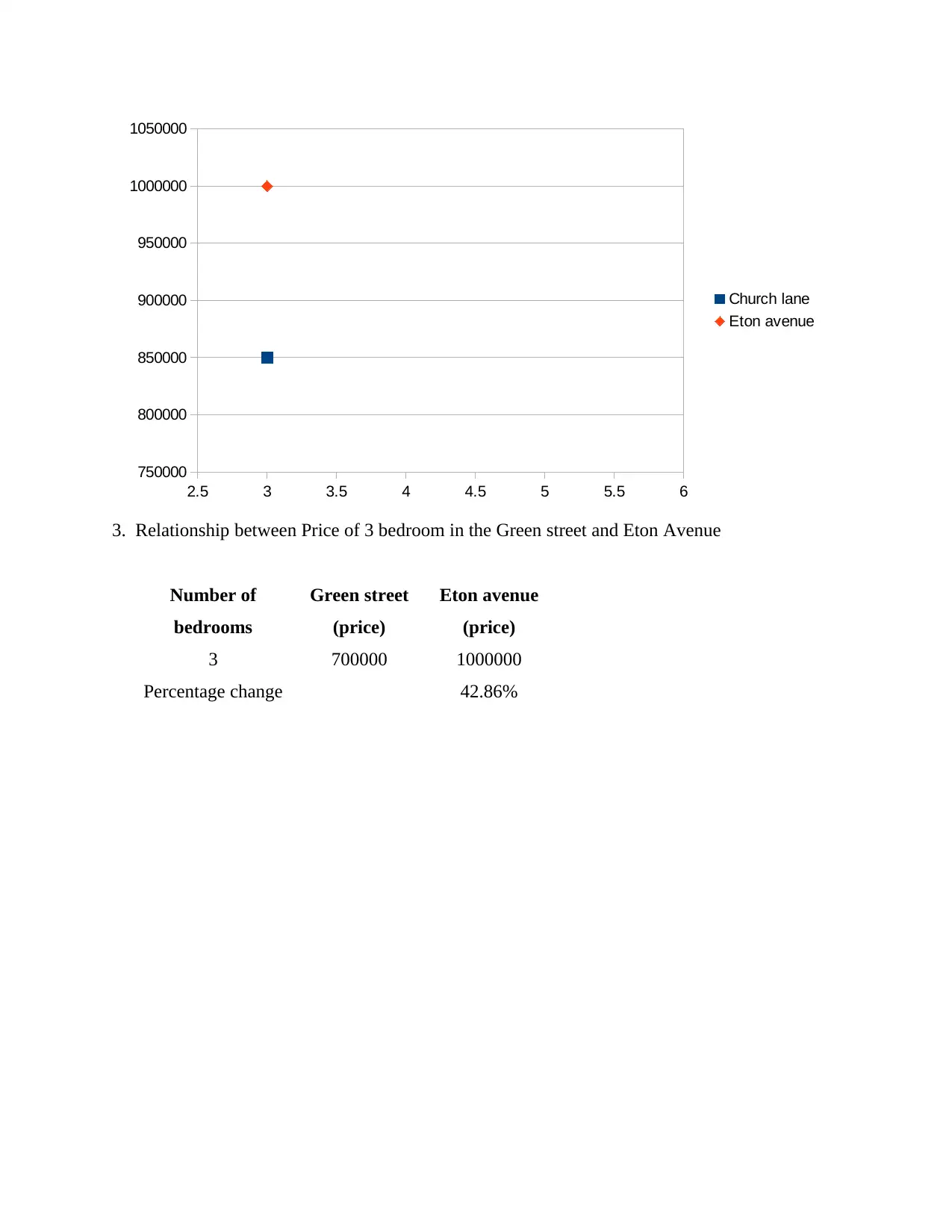
3. Relationship between Price of 3 bedroom in the Green street and Eton Avenue
Number of
bedrooms
Green street
(price)
Eton avenue
(price)
3 700000 1000000
Percentage change 42.86%
2.5 3 3.5 4 4.5 5 5.5 6
750000
800000
850000
900000
950000
1000000
1050000
Church lane
Eton avenue
Number of
bedrooms
Green street
(price)
Eton avenue
(price)
3 700000 1000000
Percentage change 42.86%
2.5 3 3.5 4 4.5 5 5.5 6
750000
800000
850000
900000
950000
1000000
1050000
Church lane
Eton avenue
You're viewing a preview
Unlock full access by subscribing today!
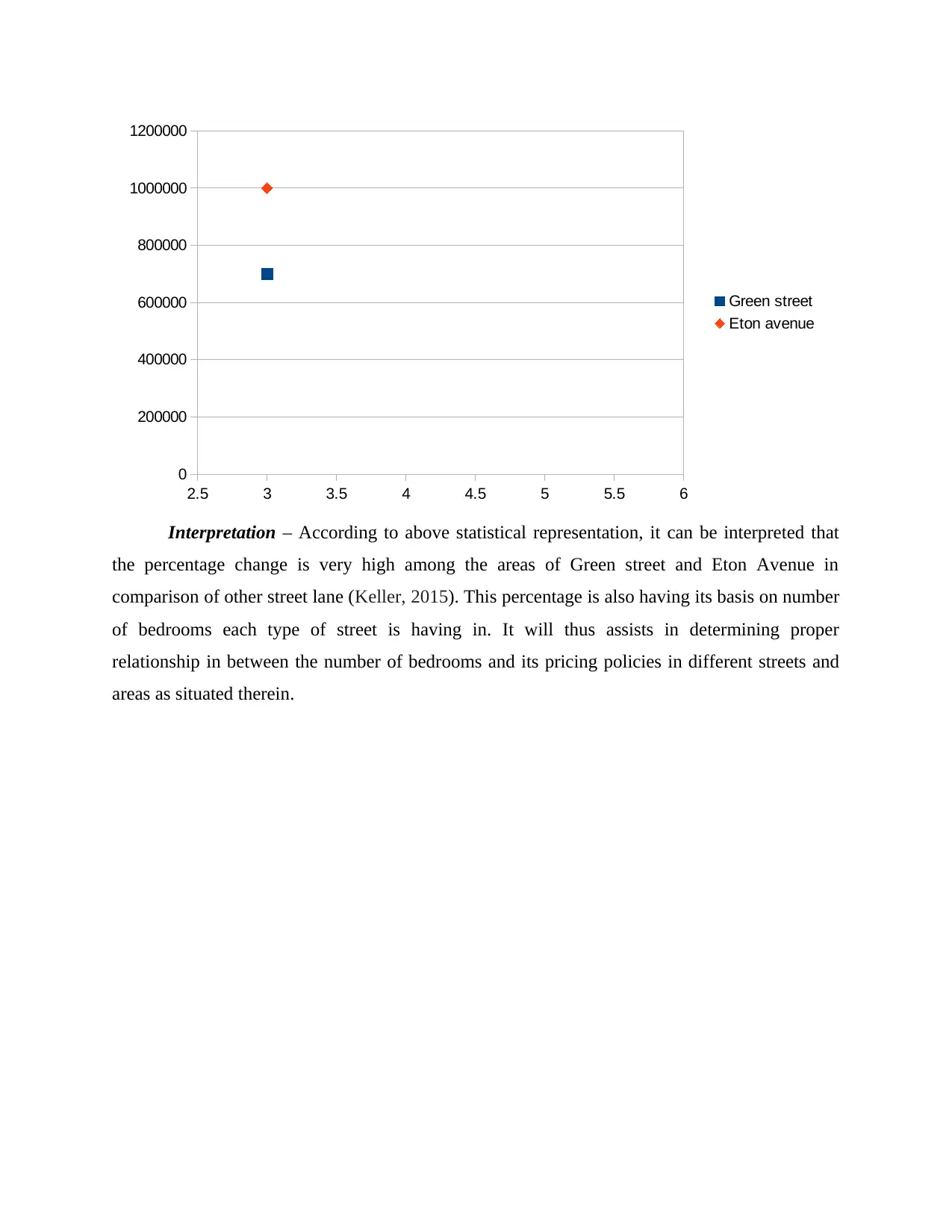
Interpretation – According to above statistical representation, it can be interpreted that
the percentage change is very high among the areas of Green street and Eton Avenue in
comparison of other street lane (Keller, 2015). This percentage is also having its basis on number
of bedrooms each type of street is having in. It will thus assists in determining proper
relationship in between the number of bedrooms and its pricing policies in different streets and
areas as situated therein.
2.5 3 3.5 4 4.5 5 5.5 6
0
200000
400000
600000
800000
1000000
1200000
Green street
Eton avenue
the percentage change is very high among the areas of Green street and Eton Avenue in
comparison of other street lane (Keller, 2015). This percentage is also having its basis on number
of bedrooms each type of street is having in. It will thus assists in determining proper
relationship in between the number of bedrooms and its pricing policies in different streets and
areas as situated therein.
2.5 3 3.5 4 4.5 5 5.5 6
0
200000
400000
600000
800000
1000000
1200000
Green street
Eton avenue
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

CONCLUSION
From the above report it can be concluded that for making statistical analysis it is very
much important to have proper knowledge about all the statistical methods and tolls. Also, for
making evaluation of statistical data in effective manner accurate as well as reliable information
is needed. By making of different types of statistical methods and graphs, it has assisted business
firms in their decision making processes related to business operations. Furthermore, it has been
evaluated that by making use of different types of graphs, charts, tables and excels data can be
analysed and interpreted in much effective and efficient manner which results in providing of
more better understanding about the subject matter to its users. For getting proper interpretation
and better understanding, it is required on the part of statistical users to make use of different
types of statistical as well as mathematical tools and methods. In this report, all the data gathered
has been interpreted with the help of bar chart, pie char and different types of statistical tables so
as to derive meaningful as well as useful information from it.
From the above report it can be concluded that for making statistical analysis it is very
much important to have proper knowledge about all the statistical methods and tolls. Also, for
making evaluation of statistical data in effective manner accurate as well as reliable information
is needed. By making of different types of statistical methods and graphs, it has assisted business
firms in their decision making processes related to business operations. Furthermore, it has been
evaluated that by making use of different types of graphs, charts, tables and excels data can be
analysed and interpreted in much effective and efficient manner which results in providing of
more better understanding about the subject matter to its users. For getting proper interpretation
and better understanding, it is required on the part of statistical users to make use of different
types of statistical as well as mathematical tools and methods. In this report, all the data gathered
has been interpreted with the help of bar chart, pie char and different types of statistical tables so
as to derive meaningful as well as useful information from it.

REFERENCES
Books and Journals
Alan, J., 2016. StatsNotes: Some Statistics for Management Problems. World Scientific.
Anderson, D. R. and et.al., 2016. Statistics for business & economics. Nelson Education.
Berman, E. and Wang, X., 2016. Essential statistics for public managers and policy analysts. Cq
Press.
Chandrasekaran, N. and Umaparvathi, M., 2016. Statistics for Management. PHI Learning Pvt.
Ltd..
Keller, G., 2015. Statistics for Management and Economics, Abbreviated. Cengage Learning.
Konik, R. P. and et.al., International Business Machines Corp, 2019. Dynamically adjusting
statistics collection time in a database management system. U.S. Patent Application
10/372,578.
Miah, A. Q., 2016. Applied statistics for social and management sciences. Springer.
Moses, D. and Venkatachalam, M., Intel Corp, 2016. Statistics for optimizing distributed
mobility anchoring for wireless networks. U.S. Patent 9,247,490.
Peters, B. G., 2016. Civil Registration and Vital Statistics as a Tool to Improve Public
Management. Inter-American Development Bank.
Price, C. and Walker, M., 2019. Improving the accessibility of foundation statistics for
undergraduate business and management students using a flipped classroom. Studies in
Higher Education, pp.1-13.
Online
Arithmetic Mean. 2018. [Online]. Available through :
<https://www.toppr.com/guides/maths/data-handling/arithmetic-mean/>.
Statistics - Standard Deviation of Discrete Data Series. 2019. [Online]. Available through :
<https://www.tutorialspoint.com/statistics/discrete_series_standard_deviation.htm>.
Books and Journals
Alan, J., 2016. StatsNotes: Some Statistics for Management Problems. World Scientific.
Anderson, D. R. and et.al., 2016. Statistics for business & economics. Nelson Education.
Berman, E. and Wang, X., 2016. Essential statistics for public managers and policy analysts. Cq
Press.
Chandrasekaran, N. and Umaparvathi, M., 2016. Statistics for Management. PHI Learning Pvt.
Ltd..
Keller, G., 2015. Statistics for Management and Economics, Abbreviated. Cengage Learning.
Konik, R. P. and et.al., International Business Machines Corp, 2019. Dynamically adjusting
statistics collection time in a database management system. U.S. Patent Application
10/372,578.
Miah, A. Q., 2016. Applied statistics for social and management sciences. Springer.
Moses, D. and Venkatachalam, M., Intel Corp, 2016. Statistics for optimizing distributed
mobility anchoring for wireless networks. U.S. Patent 9,247,490.
Peters, B. G., 2016. Civil Registration and Vital Statistics as a Tool to Improve Public
Management. Inter-American Development Bank.
Price, C. and Walker, M., 2019. Improving the accessibility of foundation statistics for
undergraduate business and management students using a flipped classroom. Studies in
Higher Education, pp.1-13.
Online
Arithmetic Mean. 2018. [Online]. Available through :
<https://www.toppr.com/guides/maths/data-handling/arithmetic-mean/>.
Statistics - Standard Deviation of Discrete Data Series. 2019. [Online]. Available through :
<https://www.tutorialspoint.com/statistics/discrete_series_standard_deviation.htm>.
You're viewing a preview
Unlock full access by subscribing today!

1 out of 28
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.





