Statistics for Business 200032 Assignment: Data Set 119 Solution

This assignment solution addresses a Statistics for Business problem set, specifically focusing on data set 119. The solution provides a detailed analysis of the data using statistical methods. Question 1 computes a 95% confidence interval for employee overtime, utilizing a t-value due to an unknown ...Read More

STATISTICS FOR BUSINESS
200032 DATA SET: 119
[DATE]

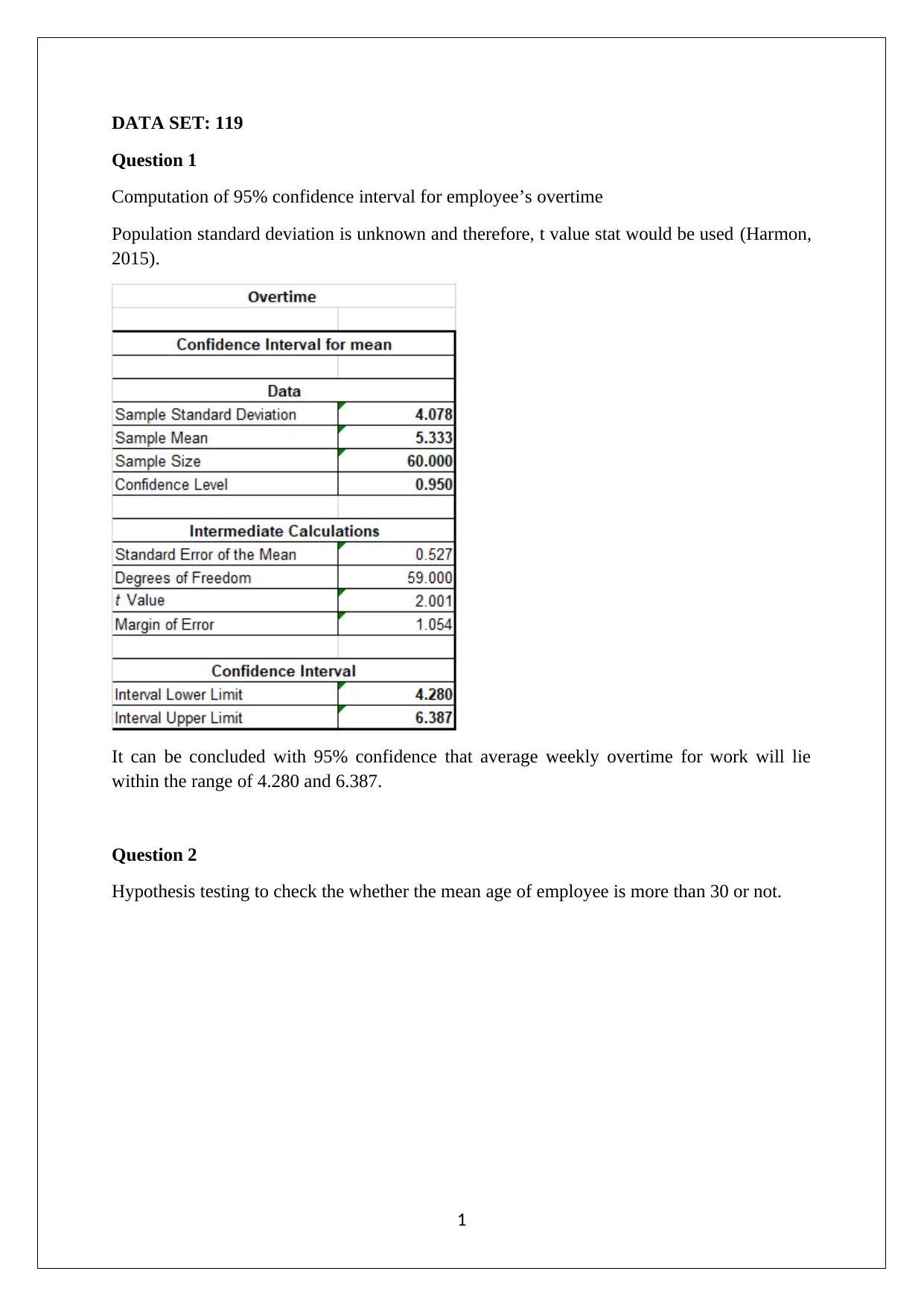
DATA SET: 119
Question 1
Computation of 95% confidence interval for employee’s overtime
Population standard deviation is unknown and therefore, t value stat would be used (Harmon,
2015).
It can be concluded with 95% confidence that average weekly overtime for work will lie
within the range of 4.280 and 6.387.
Question 2
Hypothesis testing to check the whether the mean age of employee is more than 30 or not.
1

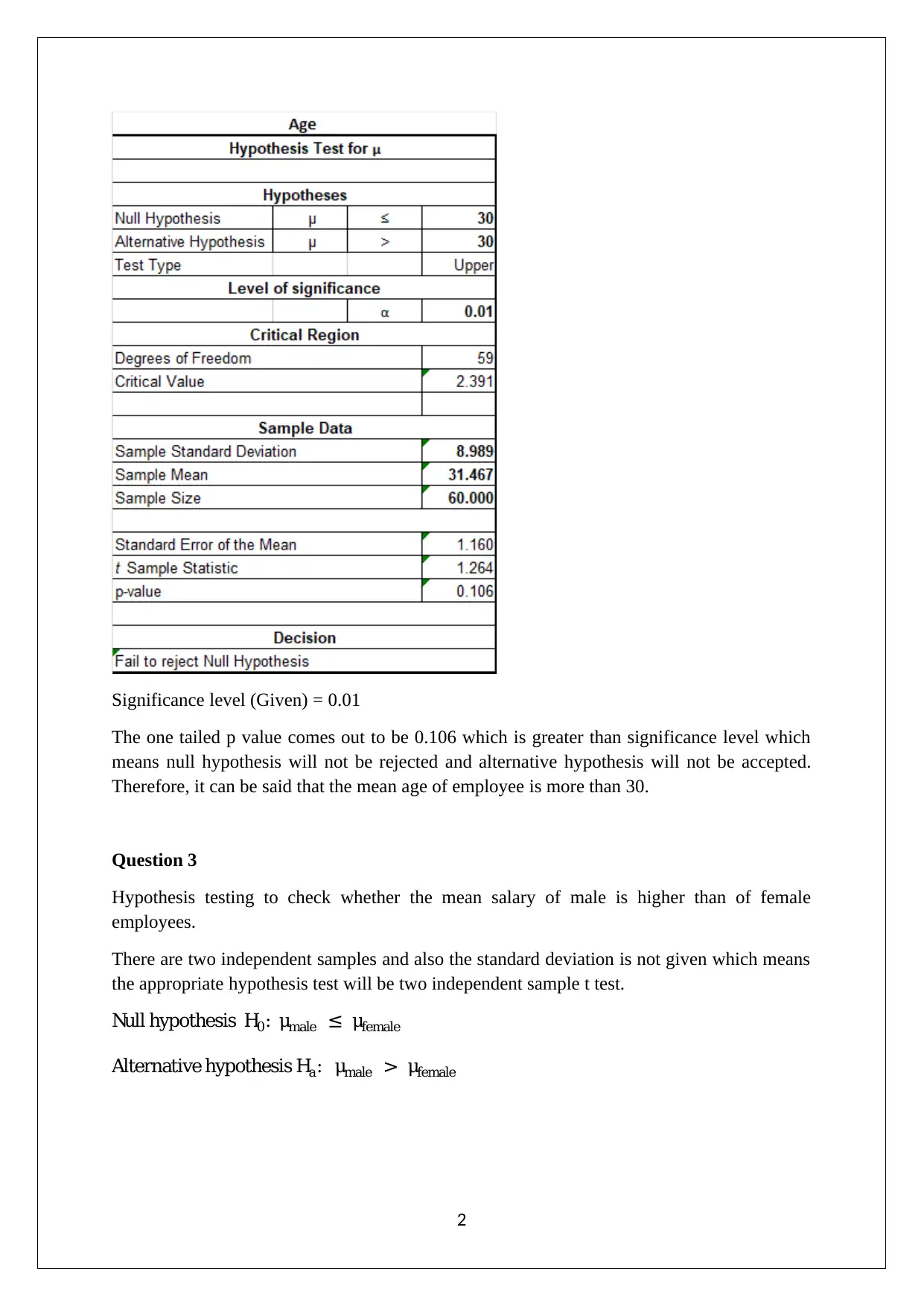
Significance level (Given) = 0.01
The one tailed p value comes out to be 0.106 which is greater than significance level which
means null hypothesis will not be rejected and alternative hypothesis will not be accepted.
Therefore, it can be said that the mean age of employee is more than 30.
Question 3
Hypothesis testing to check whether the mean salary of male is higher than of female
employees.
There are two independent samples and also the standard deviation is not given which means
the appropriate hypothesis test will be two independent sample t test.
Null hypothesis H0: μmale ≤ μfemale
Alternative hypothesis Ha: μmale > μfemale
2

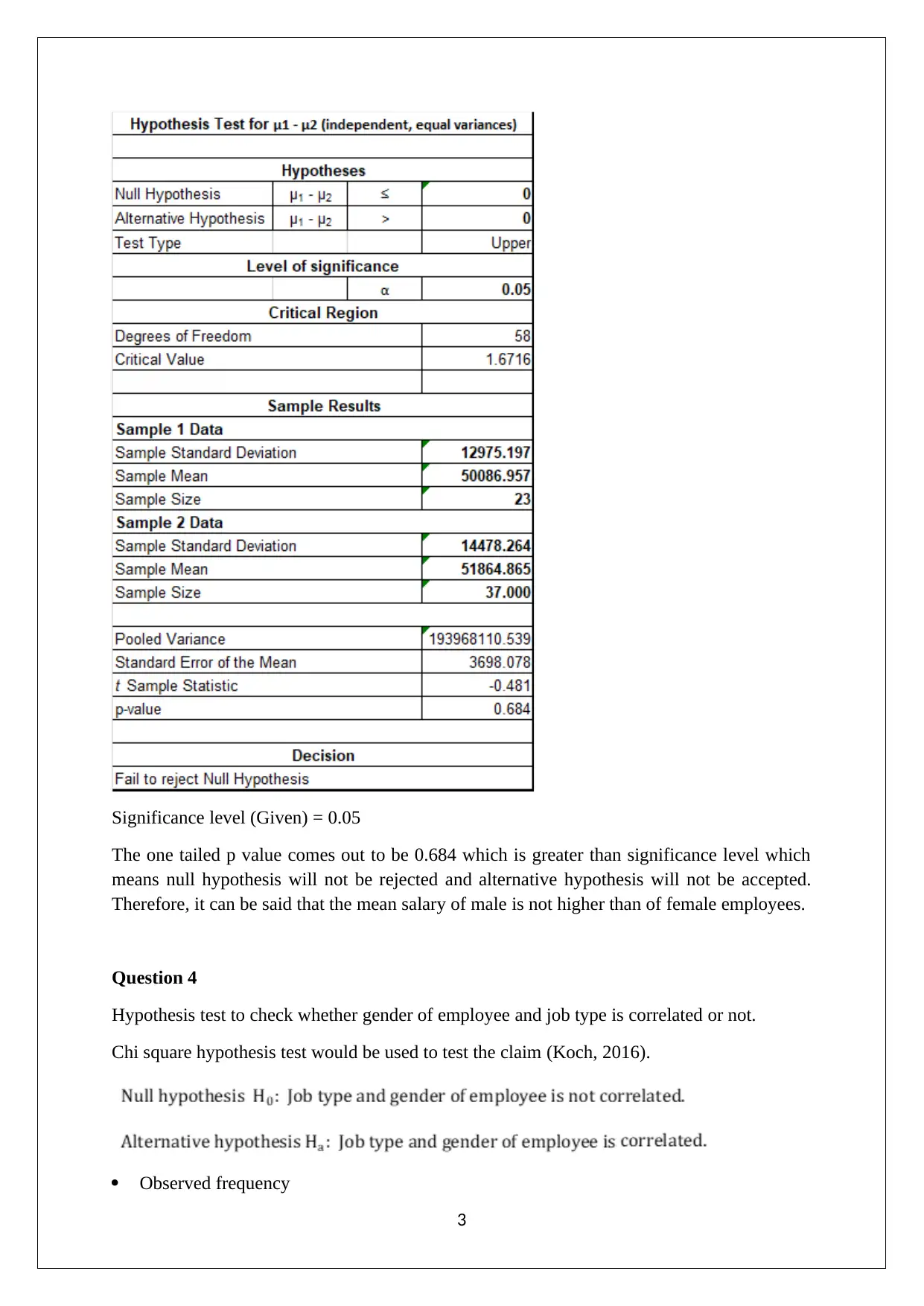
Significance level (Given) = 0.05
The one tailed p value comes out to be 0.684 which is greater than significance level which
means null hypothesis will not be rejected and alternative hypothesis will not be accepted.
Therefore, it can be said that the mean salary of male is not higher than of female employees.
Question 4
Hypothesis test to check whether gender of employee and job type is correlated or not.
Chi square hypothesis test would be used to test the claim (Koch, 2016).
 Observed frequency
3

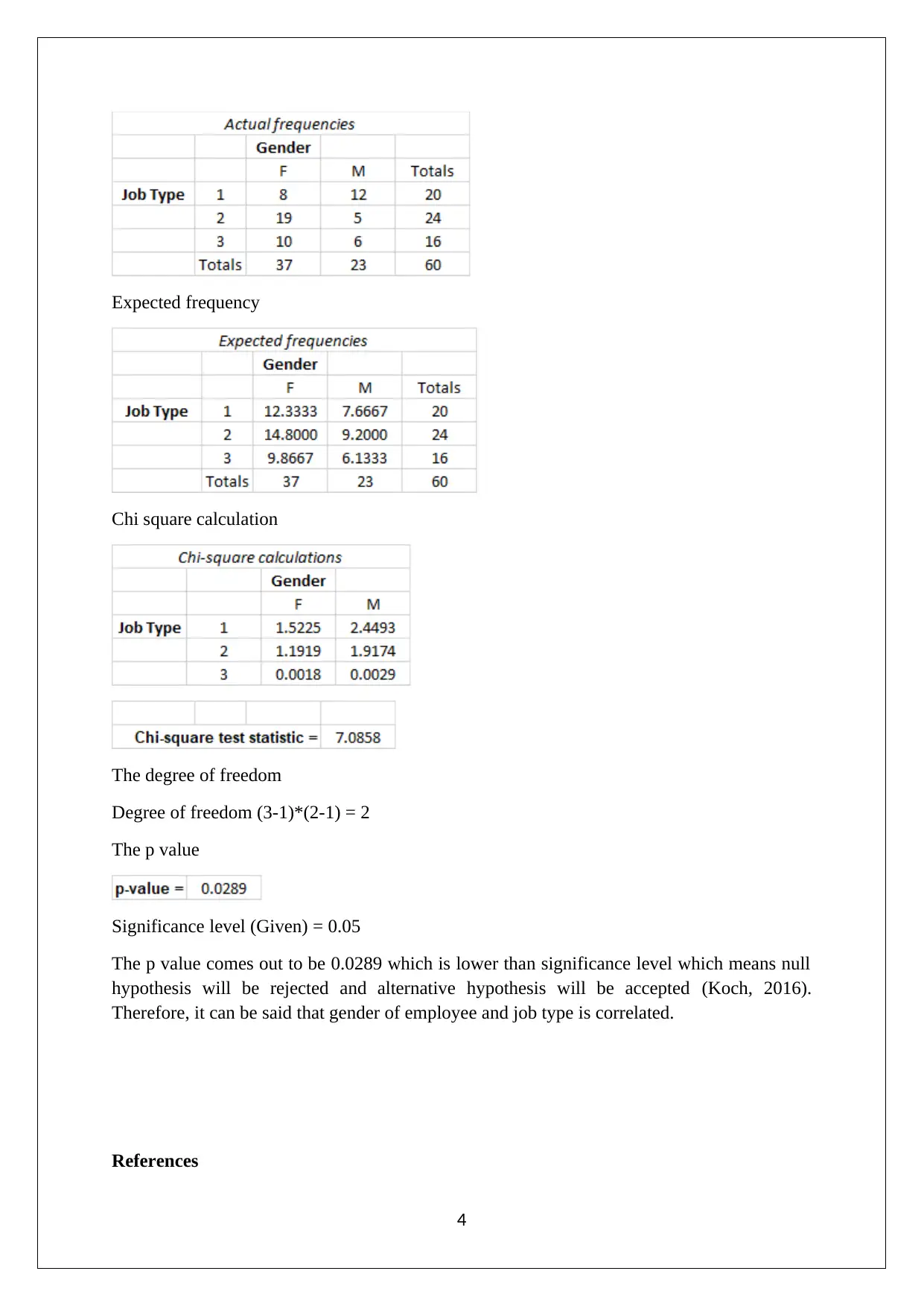
Expected frequency
Chi square calculation
The degree of freedom
Degree of freedom (3-1)*(2-1) = 2
The p value
Significance level (Given) = 0.05
The p value comes out to be 0.0289 which is lower than significance level which means null
hypothesis will be rejected and alternative hypothesis will be accepted (Koch, 2016).
Therefore, it can be said that gender of employee and job type is correlated.
References
4

Harmon, M. (2015). Hypothesis Testing in Excel - The Excel Statistical Master (7th ed.).
Florida: Mark Harmon.
Koch, K.R. (2016). Parameter Estimation and Hypothesis Testing in Linear Models (2nd ed.).
London: Springer Science & Business Media.
5