Quantitative Methods (BUSN1009) Assessment 3a: Short Answer

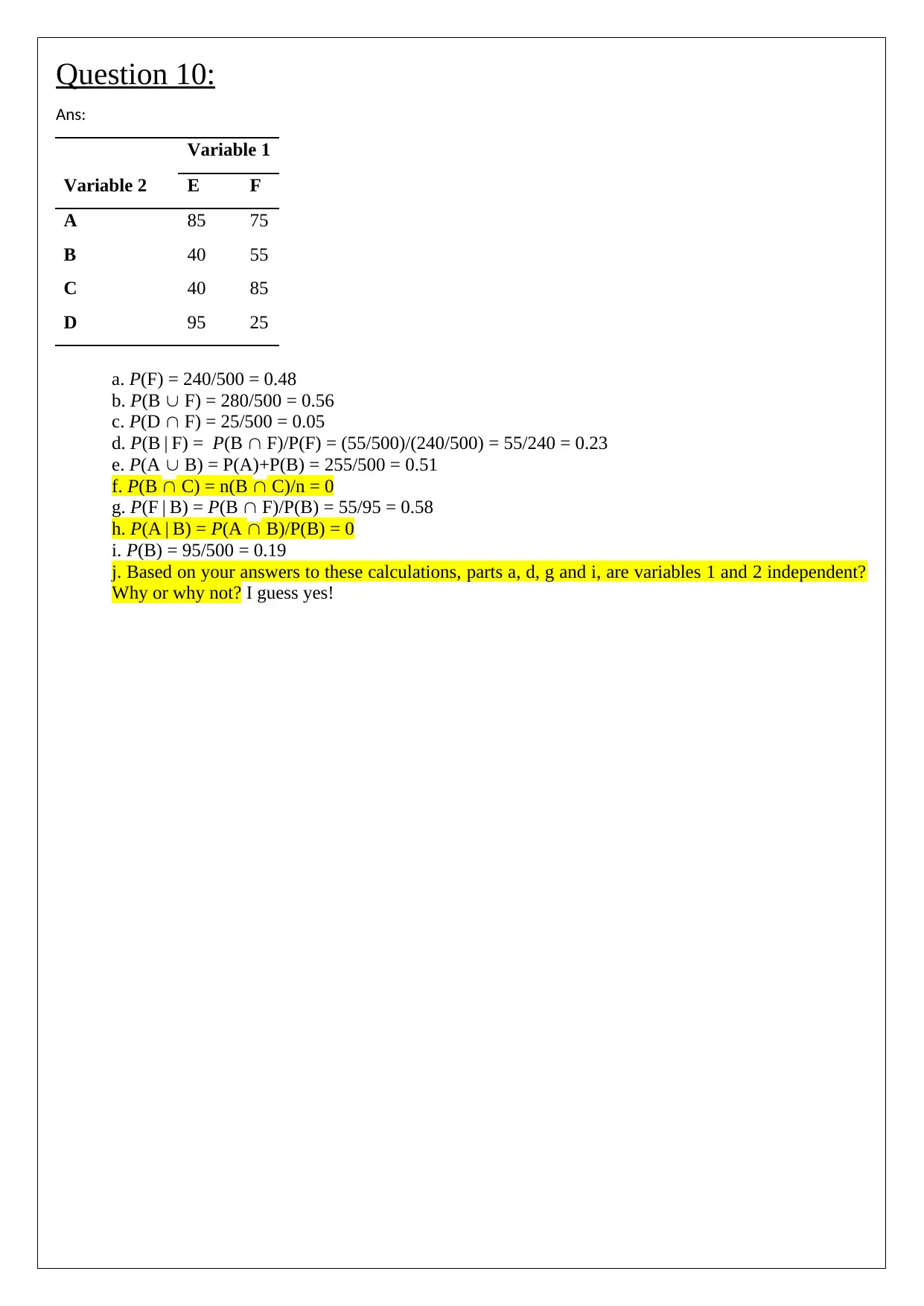
This document provides a detailed solution to a quantitative methods assignment, covering a range of statistical concepts. The assignment includes questions on sample size calculation, systematic sampling, percentiles, quartiles, variance, standard deviation, and the empirical rule. Probability questions involve calculating probabilities, conditional probabilities, and understanding independent events. The solution demonstrates step-by-step calculations and explanations for each question, offering a comprehensive guide to understanding and solving quantitative methods problems. This assignment is designed to help students grasp fundamental statistical concepts and apply them to real-world scenarios.

Question 1:
Sample size = 100000/200 = 500
Question 2:
k = (total sample)/(size of random sample) = 3500/175 = 20
sampling process will start between 1st and kth employee (including both).
Question3:
Sorted List =[146, 204, 280, 298, 320, 356, 445, 450, 470, 786, 800, 820, 849, 918, 957, 964]
20th Percentile(index) = 0.20 * 16 = 3.2(index). Therefore 20th Percentile = 280.
60th Percentile (index) = 0.60 * 16 = 9.6(index). Therefore 60th Percentile = 786.
Q1 is the median (the middle) of the lower half of the data, and Q3 is the median (the middle) of the upper
half of the data
Quartile Q1: 309
Quartile Q2: 460
Quartile Q3: 834.5
IQR = 834.5 – 309 = 525.5
Question 4:
a)
Sample variance (s2)= (xi-x)2(n-1)
xi = a number in data set
x = mean (average)
n = total number of samples in dataset
In the given example,
n = 8
x = (4+3+0+5+2+9+4+5)/8 = 4
Sample variance (s2) = (xi-x)2(n-1) = (0+1+16+1+4+25+0+1)/7 = 48/7 = 6.8571
b)
Sample standard deviation (Sx) = (xi-x)2(n-1) = 6.8571 = 2.6186
Question 5:
Using Emperical Rule,

For 68% of data fall:
Time Range 1=419 -27=392 Minutes.
Time Range 2 = 419+27 = 446 Minutes
For 95% of data fall:
Time Range 1=419 –(2*27)=365 Minutes.
Time Range 2 = 419+(2*27) = 473 Minutes
For 99.7% of data fall:
Time Range 1=419 –(3*27)=338 Minutes.
Time Range 2 = 419+(3*27) =500 Minutes
Question 6:
n = 300
a)
n(A) = 42+54+21 = 117
P(A) = n(A)/n = 117/300 = 0.39
b)
n(Z) = 21+39+12 = 72
P(Z) = 72/300 = 0.24
c)
P(A ∩ X) = 42/300 = 0.14
d)
P(B ∩ Z) = 39/300 = 0.13
e)
P(A ∪ C) = (n(A)+n(C))/n = (117+66)/300 = 0.61
f)
P(A ∩ B) = n(A ∩ B)/n = 0/300 = 0

Question 7:
D E
A 0.32 0.16
B 0.20 0.12
C 0.16 0.04
a. P(A) = 0.32+0.16 = 0.48
b. P(E) = 0.16+0.12+0.04 = 0.32
c. P(A ∩ E) = 0.16
d. P(A ⎟ E) = P(A ∩ E)/P(E) = 0.16/0.32 = 0.5
Question8:
Let A = the event that a woman randomly selected participate in labor force, and B = the event that a woman
randomly selected is married, and ~X = complement of X.
a. P(A or B) = P(A) + P(B) - P(A and B) = .75 + .78 - .61 = .92
b. P[(A and ~B) or (B and ~A)] = P(A and ~B) + P(B and ~A) = P(A) - P(A and B) + P(B) - P(B and A)
= .75 - .61 + .78 - .61 = .14 + .17 = .31
c. P(~A and ~B) = P(~(A or B)) = 1 - P(A or B) = 1 - .92 = .08
Question 9:
a)
P(B | A) = P(A ∩ B)/P(A)
P(A ∩ B) = P(A)*P(B | A) = (0.40)*(0.25) = 0.1
b)
P(A | C) = P(A ∩ C)/P(C)
P(A ∩ C) = P(C)*P(A | C) = (0.35)*(0.80) = 0.28
c)
If A and B are independent, P(A ∩ B) = 0
d) If A and C are independent, P(A ∩ C) = 0

Question 10:
Ans:
Variable 1
Variable 2 E F
A 85 75
B 40 55
C 40 85
D 95 25
a. P(F) = 240/500 = 0.48
b. P(B ∪ F) = 280/500 = 0.56
c. P(D ∩ F) = 25/500 = 0.05
d. P(B | F) = P(B ∩ F)/P(F) = (55/500)/(240/500) = 55/240 = 0.23
e. P(A ∪ B) = P(A)+P(B) = 255/500 = 0.51
f. P(B ∩ C) = n(B ∩ C)/n = 0
g. P(F | B) = P(B ∩ F)/P(B) = 55/95 = 0.58
h. P(A | B) = P(A ∩ B)/P(B) = 0
i. P(B) = 95/500 = 0.19
j. Based on your answers to these calculations, parts a, d, g and i, are variables 1 and 2 independent?
Why or why not? I guess yes!