Statistics and Probability - Desklib

This document contains solved assignments and study material related to Statistics and Probability. It covers topics such as mean, standard deviation, probability, linear regression and more. The content includes answers to questions related to days lost, defective components, normal distribution, and linear regression.

Running head: STATISTICS AND PROBABILITY
Statistics and Probability
Name of the Student:
Name of the University:
Author’s note:

1STATISTICS AND PROBABILITY
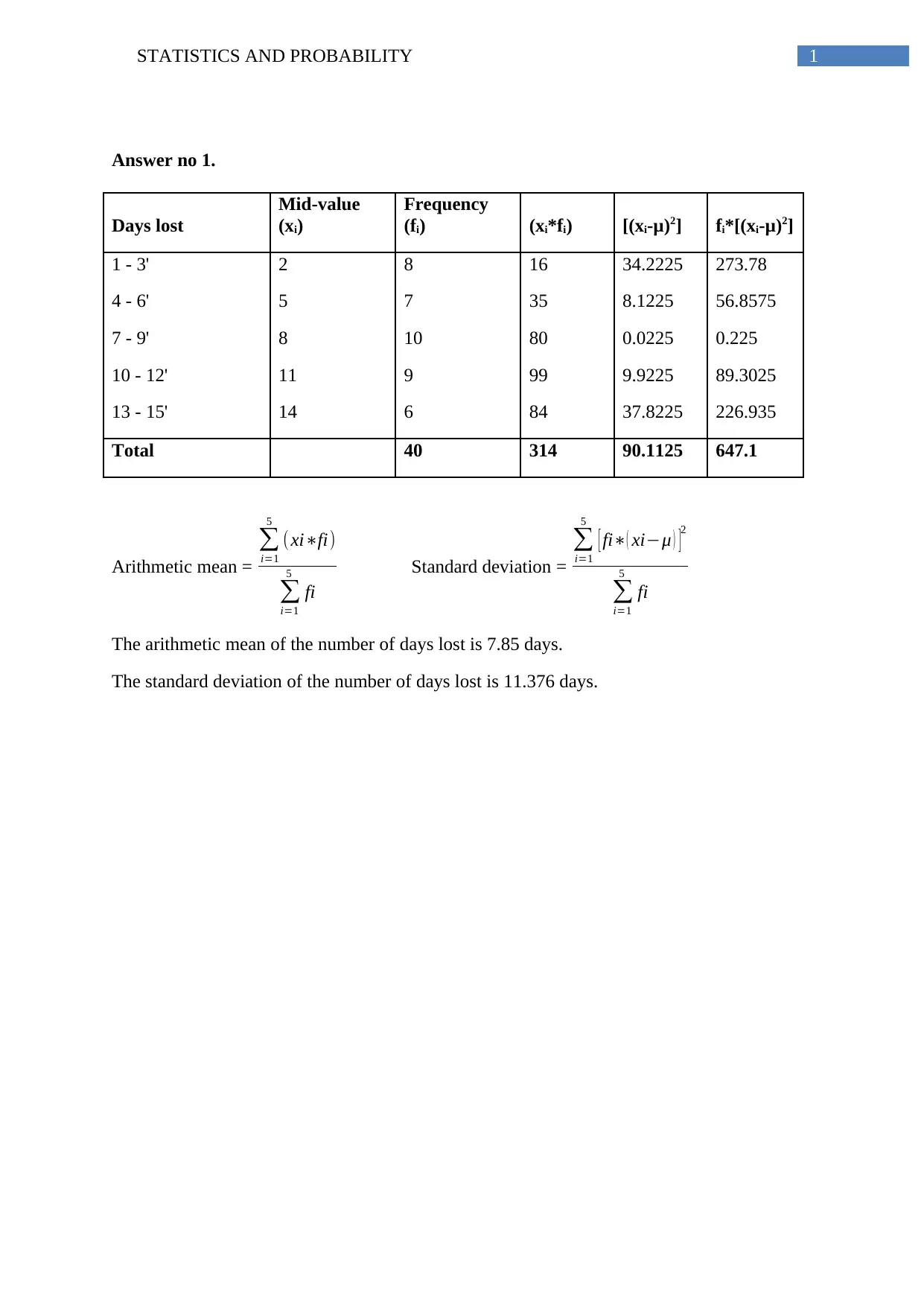
Answer no 1.
Days lost
Mid-value
(xi)
Frequency
(fi) (xi*fi) [(xi-μ)2] fi*[(xi-μ)2]
1 - 3' 2 8 16 34.2225 273.78
4 - 6' 5 7 35 8.1225 56.8575
7 - 9' 8 10 80 0.0225 0.225
10 - 12' 11 9 99 9.9225 89.3025
13 - 15' 14 6 84 37.8225 226.935
Total 40 314 90.1125 647.1
Arithmetic mean =
∑
i=1
5
(xi∗fi)
∑
i=1
5
fi
Standard deviation =
∑
i=1
5
[ fi∗( xi−μ ) ]2
∑
i=1
5
fi
The arithmetic mean of the number of days lost is 7.85 days.
The standard deviation of the number of days lost is 11.376 days.

2STATISTICS AND PROBABILITY
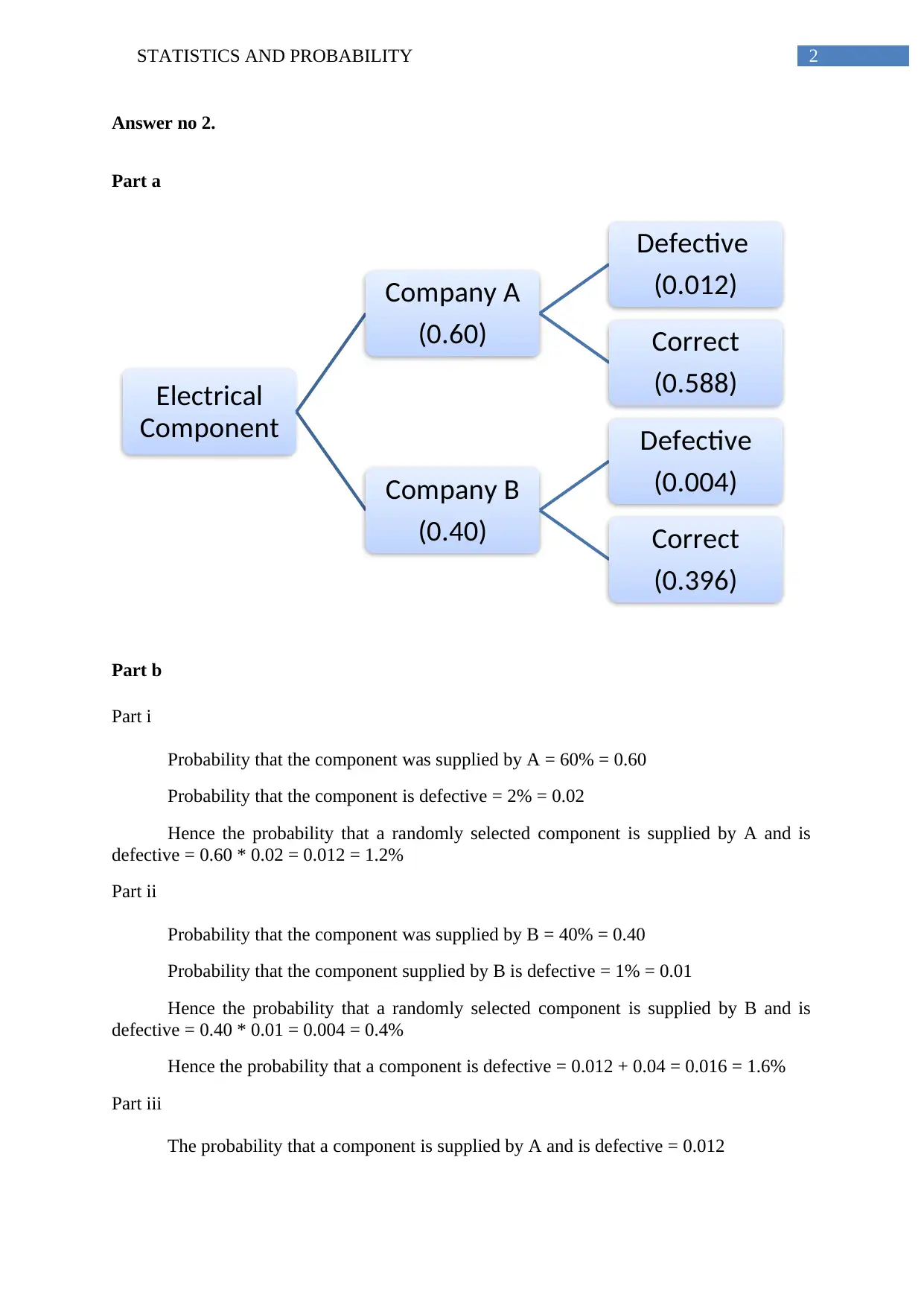
Answer no 2.
Part a
Part b
Part i
Probability that the component was supplied by A = 60% = 0.60
Probability that the component is defective = 2% = 0.02
Hence the probability that a randomly selected component is supplied by A and is
defective = 0.60 * 0.02 = 0.012 = 1.2%
Part ii
Probability that the component was supplied by B = 40% = 0.40
Probability that the component supplied by B is defective = 1% = 0.01
Hence the probability that a randomly selected component is supplied by B and is
defective = 0.40 * 0.01 = 0.004 = 0.4%
Hence the probability that a component is defective = 0.012 + 0.04 = 0.016 = 1.6%
Part iii
The probability that a component is supplied by A and is defective = 0.012
Electrical
Component
Company A
(0.60)
Defective
(0.012)
Correct
(0.588)
Company B
(0.40)
Defective
(0.004)
Correct
(0.396)

3STATISTICS AND PROBABILITY
Probability that a component is defective = 0.016
Hence, the probability that a component is defective and is supplied by Company A ¿ 0.012
0.016
= 75.0 or 75%
Answer no 3.
The mean length of metal strips produced by a machine is normally distributed. The mean
length is 150 cm and the standard deviation is 10 cm.
Part a
The probability that the length of a randomly selected strip is shorter than 165 cm. =
P (X<165) = P[ ( X−μ )
σ < ( 165−150 )
10 ] = P[ ( X −μ )
σ <1.5] = P[Z<1.5] = (1-0.06681) =
0.93319
Part b
The probability that the length of a randomly selected strip is longer than 170 cm. =
P (X>170) = P[ ( X−μ )
σ > ( 170−150 )
10 ] = P[ ( X −μ )
σ > 2] = P[Z>2] = 0.02275
Part c
The probability that the length of a randomly selected strip is with the range of 145 cm. to
155 cm. =
P (145<X<155) = P[ ( 145−150 )
10 < ( X−μ )
σ < ( 155−150 )
10 ] = P [−0.5< ( X−μ )
σ <0.5] = P [-
0.5<Z<0.5] = P[mod(Z)<0.5] = 0.3829.
Answer no 4.
Diameters (D mm) Torque (T Nm)
6 5.5
10 7
14 9.5
18 12.5
21 13.5

4STATISTICS AND PROBABILITY
25 16.5
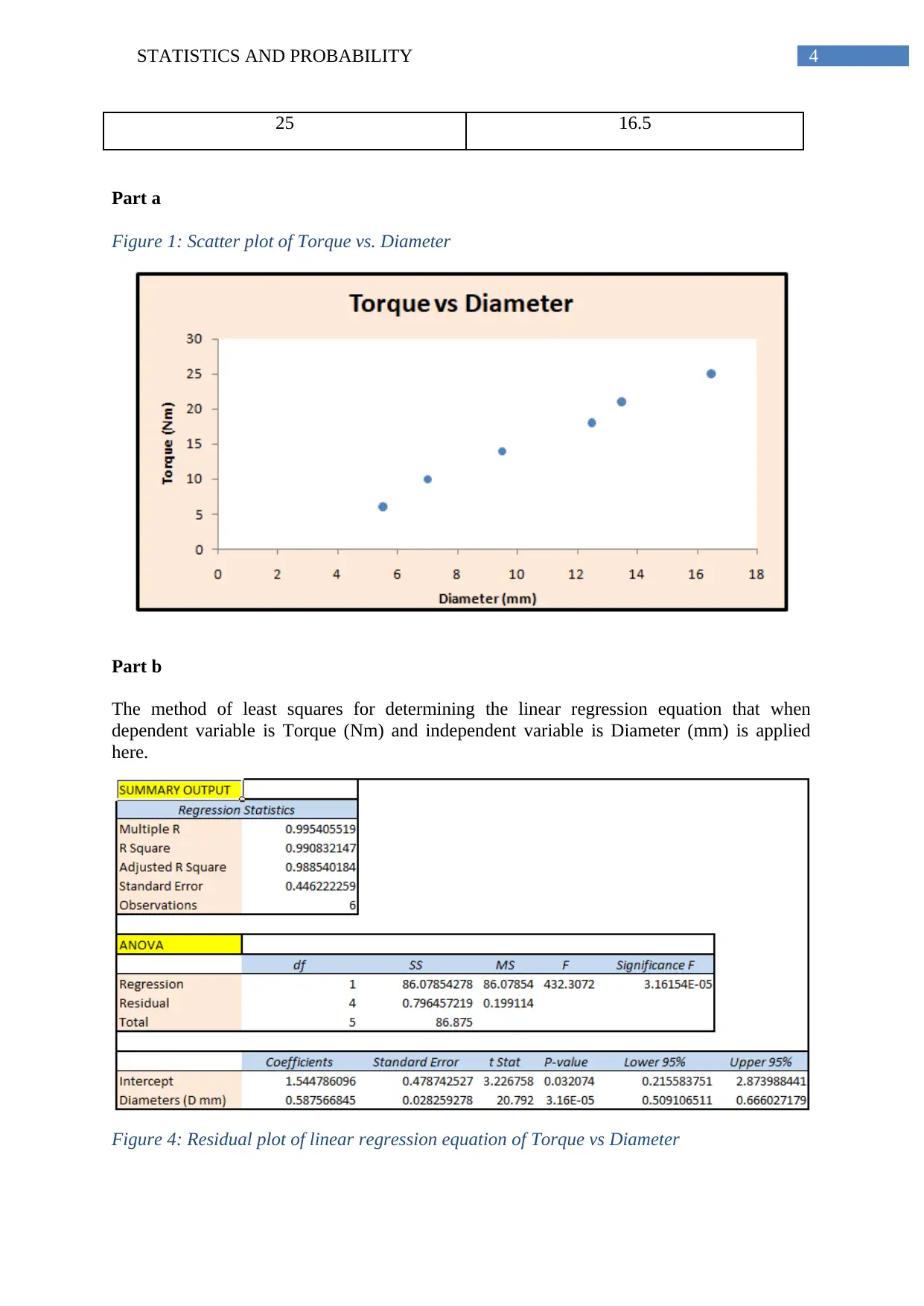
Part a
Figure 1: Scatter plot of Torque vs. Diameter
Part b
The method of least squares for determining the linear regression equation that when
dependent variable is Torque (Nm) and independent variable is Diameter (mm) is applied
here.
Figure 4: Residual plot of linear regression equation of Torque vs Diameter

5STATISTICS AND PROBABILITY
Part c
The linear regression model assuming Torque (Nm) as a dependent and Diameter (mm) as a
independent variable is calculated as-
Torque = (1.544786096) + 0.587566845 * Diameters
The value of torque when diameter is 16 mm, is found out to be 10.95 Nm.
Part d
The correlation coefficient ( r ) is calculated in this linear regression model as 0.995405519.
It refers a very strong link between dependent and independent variable.
The value of multiple R2 is found out to be 0.990832147. Therefore, the linear association
between dependent and independent variable is very strong. Diameter can explain more than
99% variability of the Torque. The value of slope (0.587566845) is positive. Therefore, the
with increment of diameter, Torque increases. With decrement of the diameter, Torque
decreases. Per unit change of diameter, the torque changes by 0.587566845 units.