Psychology Statistics Assignment: Regression, ANOVA, Hypothesis Tests

This assignment presents a comprehensive analysis of statistical methods applied to psychological research. The solution begins with a multiple regression analysis, examining the relationships between fear of death and several predictor variables (importance of religion, avoidance of death, meanin...Read More

1
Project 2
Name:
Institution

2
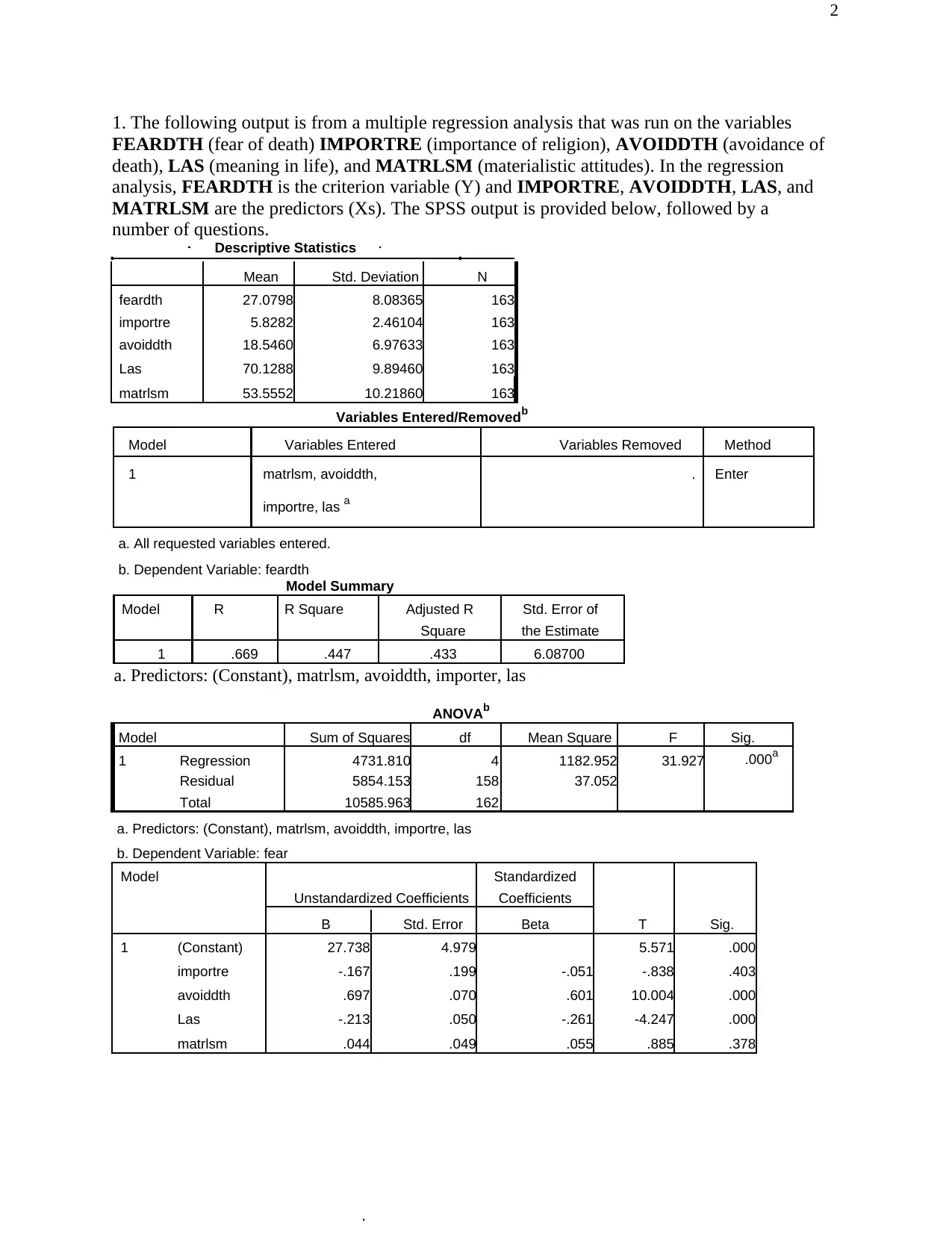
1. The following output is from a multiple regression analysis that was run on the variables
FEARDTH (fear of death) IMPORTRE (importance of religion), AVOIDDTH (avoidance of
death), LAS (meaning in life), and MATRLSM (materialistic attitudes). In the regression
analysis, FEARDTH is the criterion variable (Y) and IMPORTRE, AVOIDDTH, LAS, and
MATRLSM are the predictors (Xs). The SPSS output is provided below, followed by a
number of questions.
Descriptive Statistics
Mean Std. Deviation N
feardth 27.0798 8.08365 163
importre 5.8282 2.46104 163
avoiddth 18.5460 6.97633 163
Las 70.1288 9.89460 163
matrlsm 53.5552 10.21860 163
Variables Entered/Removedb
Model Variables Entered Variables Removed Method
1 matrlsm, avoiddth, . Enter
importre, las a
a. All requested variables entered.
b. Dependent Variable: feardth
Model Summary
Model R R Square Adjusted R Std. Error of
Square the Estimate
1 .669 .447 .433 6.08700
a. Predictors: (Constant), matrlsm, avoiddth, importer, las
ANOVAb
Model Sum of Squares df Mean Square F Sig.
1 Regression 4731.810 4 1182.952 31.927 .000a
Residual 5854.153 158 37.052
Total 10585.963 162
a. Predictors: (Constant), matrlsm, avoiddth, importre, las
b. Dependent Variable: fear
Model Standardized
Unstandardized Coefficients Coefficients
B Std. Error Beta T Sig.
1 (Constant) 27.738 4.979 5.571 .000
importre -.167 .199 -.051 -.838 .403
avoiddth .697 .070 .601 10.004 .000
Las -.213 .050 -.261 -4.247 .000
matrlsm .044 .049 .055 .885 .378

3
Coefficientsa
a. Dependent Variable: feardth
Answer the following questions for the regression procedure:
a. How many people were in the study? _______163______ (1 point)
b. What is R2 for the regression analysis? _______0.447____ (2 points)
c. Is R2 significant? Report the appropriate statistical criteria to support your answer (do not
just provide the p-value, but the APA formatted information as appropriate)
As evident, the model recorded a p-value of 0.000 which is less than the significance
level 0.01 or 0.05 thus, the R2 value is significant.
d. Which predictor(s), if any, are significant? Which predictor(s), if any, are not significant? Be
sure to (1) indicate whether each predictor is significant or not and (2) report the corresponding
t values and p-values for each of the predictors (whether significant or not) below.
At 0.05 significance level
There are 4 predictors, which include importance of religion, avoidance of death,
meaning in life, and materialistic attitudes. Notably, predictors that recorded p-values less than
0.05 are significant otherwise they are not significant. Therefore, avoidance of death and
meaning in life are significant whereas importance of religion and materialistic attitudes are not
significant.
e. Write the final equation for the regression model. (Consult your text as needed for details on
writing the regression equation.)
Regression equation
Feardth=27.738 – 0.167 Importre +0.697 Avoiddth – 0.213 Las+ 0.044 Matrlsm
However, by eliminating the non-significant predictors the final regression model becomes
Feardth=27.738+0.697 Avoiddth – 0.213 Las

4
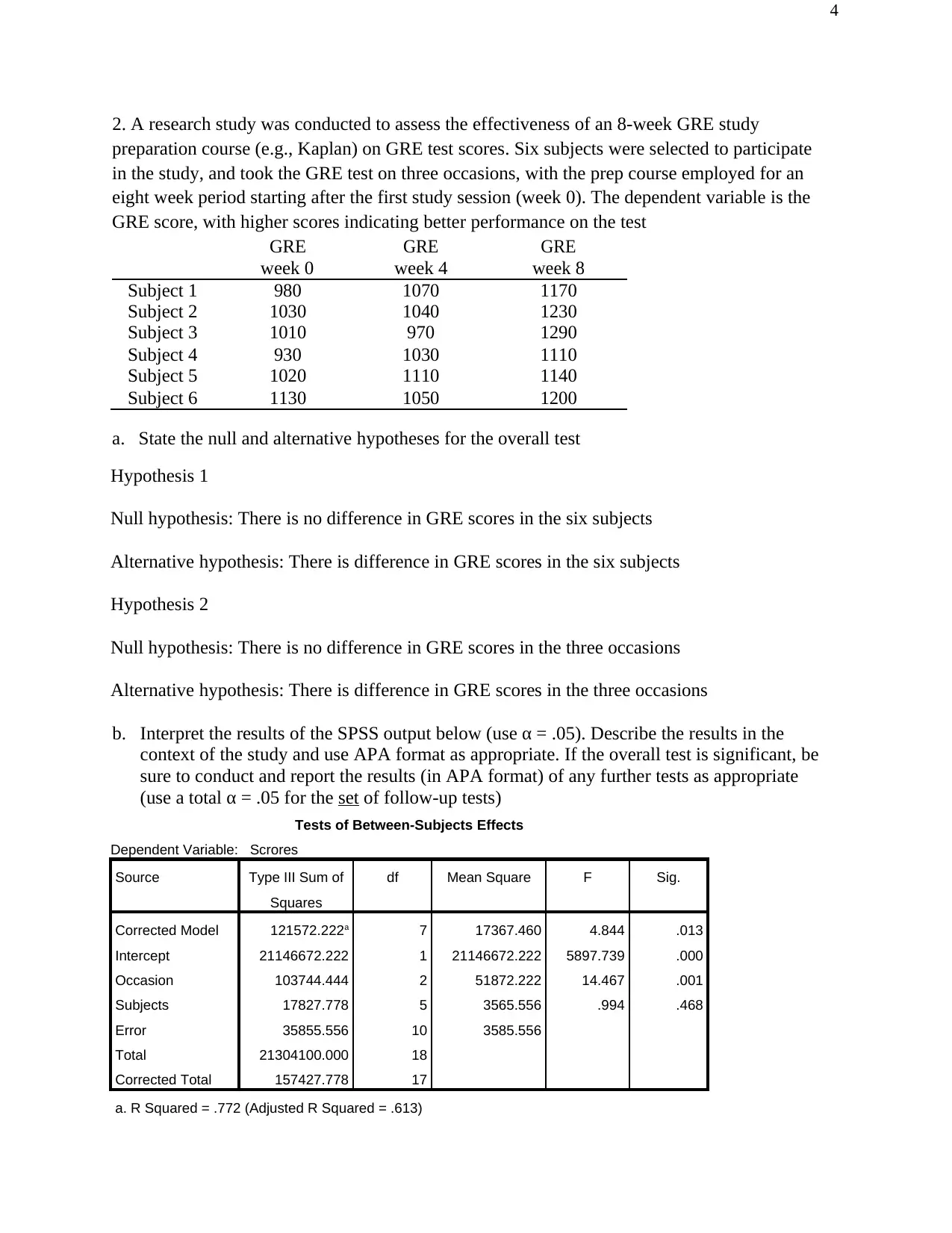
2. A research study was conducted to assess the effectiveness of an 8-week GRE study
preparation course (e.g., Kaplan) on GRE test scores. Six subjects were selected to participate
in the study, and took the GRE test on three occasions, with the prep course employed for an
eight week period starting after the first study session (week 0). The dependent variable is the
GRE score, with higher scores indicating better performance on the test
GRE GRE GRE
week 0 week 4 week 8
Subject 1 980 1070 1170
Subject 2 1030 1040 1230
Subject 3 1010 970 1290
Subject 4 930 1030 1110
Subject 5 1020 1110 1140
Subject 6 1130 1050 1200
a. State the null and alternative hypotheses for the overall test
Hypothesis 1
Null hypothesis: There is no difference in GRE scores in the six subjects
Alternative hypothesis: There is difference in GRE scores in the six subjects
Hypothesis 2
Null hypothesis: There is no difference in GRE scores in the three occasions
Alternative hypothesis: There is difference in GRE scores in the three occasions
b. Interpret the results of the SPSS output below (use α = .05). Describe the results in the
context of the study and use APA format as appropriate. If the overall test is significant, be
sure to conduct and report the results (in APA format) of any further tests as appropriate
(use a total α = .05 for the set of follow-up tests)
Tests of Between-Subjects Effects
Dependent Variable: Scrores
Source Type III Sum of
Squares
df Mean Square F Sig.
Corrected Model 121572.222a 7 17367.460 4.844 .013
Intercept 21146672.222 1 21146672.222 5897.739 .000
Occasion 103744.444 2 51872.222 14.467 .001
Subjects 17827.778 5 3565.556 .994 .468
Error 35855.556 10 3585.556
Total 21304100.000 18
Corrected Total 157427.778 17
a. R Squared = .772 (Adjusted R Squared = .613)

5
Hypothesis 1
It is shown the subjects had a p-value of 0.468 which is greater than the significance level 0.05
thus we fail to null hypothesis is rejected and conclude that there is no difference in GRE scores
in the six subjects
It is shown the occasion had a p-value of 0.001 which is less than the significance level 0.05 thus
the null hypothesis is rejected and conclude that there is difference in GRE scores in the three
occasions.

6
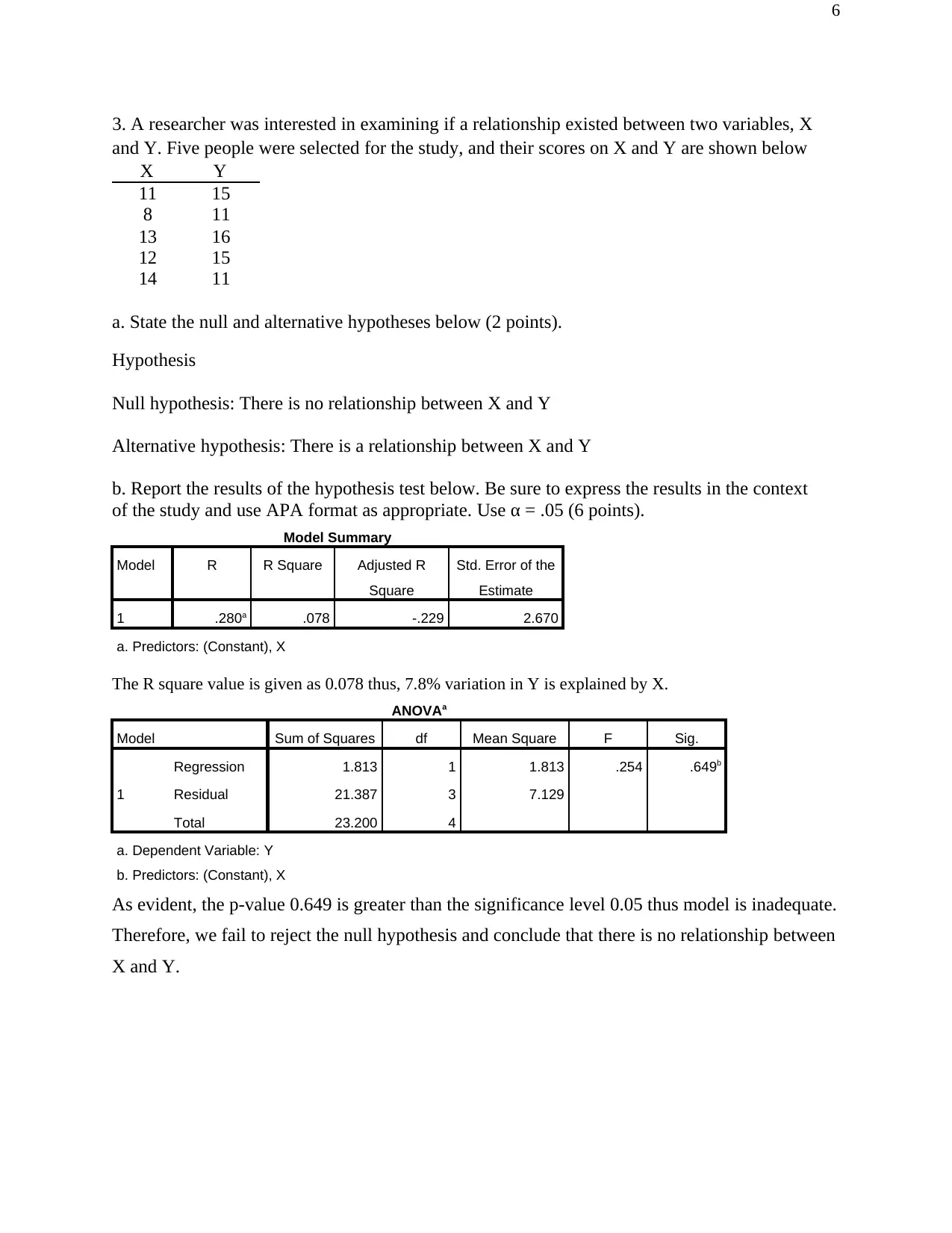
3. A researcher was interested in examining if a relationship existed between two variables, X
and Y. Five people were selected for the study, and their scores on X and Y are shown below
X Y
11 15
8 11
13 16
12 15
14 11
a. State the null and alternative hypotheses below (2 points).
Hypothesis
Null hypothesis: There is no relationship between X and Y
Alternative hypothesis: There is a relationship between X and Y
b. Report the results of the hypothesis test below. Be sure to express the results in the context
of the study and use APA format as appropriate. Use α = .05 (6 points).
Model Summary
Model R R Square Adjusted R
Square
Std. Error of the
Estimate
1 .280a .078 -.229 2.670
a. Predictors: (Constant), X
The R square value is given as 0.078 thus, 7.8% variation in Y is explained by X.
ANOVAa
Model Sum of Squares df Mean Square F Sig.
1
Regression 1.813 1 1.813 .254 .649b
Residual 21.387 3 7.129
Total 23.200 4
a. Dependent Variable: Y
b. Predictors: (Constant), X
As evident, the p-value 0.649 is greater than the significance level 0.05 thus model is inadequate.
Therefore, we fail to reject the null hypothesis and conclude that there is no relationship between
X and Y.

7
4. An experiment was conducted to examine the performance (as measured by the number of
errors made) on a motor skills task. Twenty-four subjects (12 young and 12 old) were assigned
to either an easy or hard motor skills task. There were two factors in the study: age (young/old)
and difficulty of the motor skills task (easy/hard). The number of errors made on the motor
skills task was recorded for each person and is presented in the table below
Difficulty
Easy Hard
3 6
4 5
Young 2 8
6 9
3 9
Age 6 7
5 10
7 11
Old 6 14
4 15
2 16
4 17
a. State the null and alternative hypotheses below
Hypothesis 1
H0: There is no interaction between age and difficulty of motor skills task
H1: There is an interaction between age and difficulty of motor skills tasks
Hypothesis 2
H0: There is no error mean difference between the two age groups (young and old).
H1: There is error mean difference between the two age groups (young and old).
Hypothesis 2
H0There is no error mean difference between the two difficult level (easy and hard).
H1: There is error mean difference between the two difficult level (easy and hard).
b. Report the results of the hypothesis tests in #a below. Be sure to express the results in the
context of the study and use APA format as appropriate. Sketch, label, and describe any
significant interaction(s). (For your sketch, it is fine to make it look like the plot shown
in SPSS.) Use α = .05 for each test

8
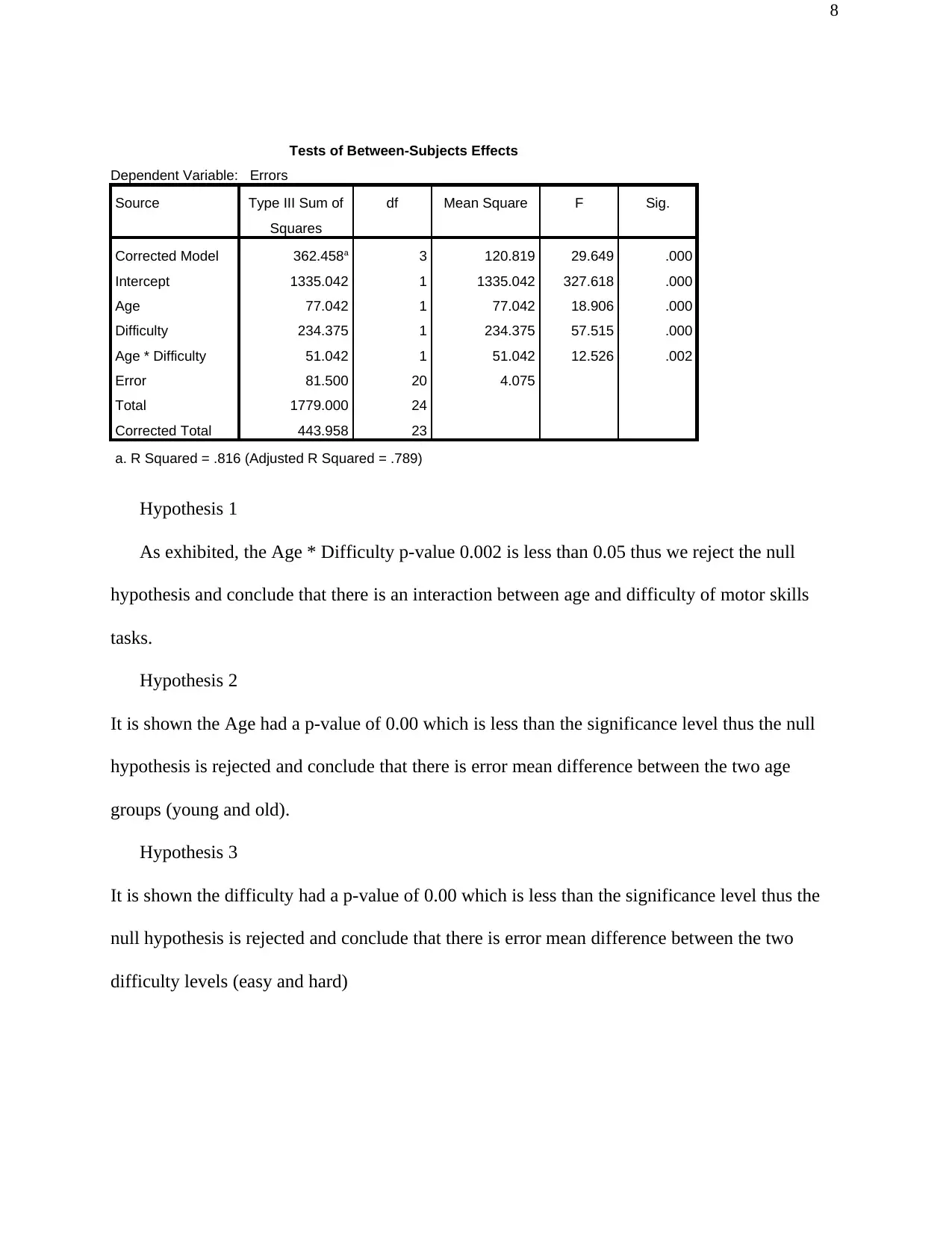
Tests of Between-Subjects Effects
Dependent Variable: Errors
Source Type III Sum of
Squares
df Mean Square F Sig.
Corrected Model 362.458a 3 120.819 29.649 .000
Intercept 1335.042 1 1335.042 327.618 .000
Age 77.042 1 77.042 18.906 .000
Difficulty 234.375 1 234.375 57.515 .000
Age * Difficulty 51.042 1 51.042 12.526 .002
Error 81.500 20 4.075
Total 1779.000 24
Corrected Total 443.958 23
a. R Squared = .816 (Adjusted R Squared = .789)
Hypothesis 1
As exhibited, the Age * Difficulty p-value 0.002 is less than 0.05 thus we reject the null
hypothesis and conclude that there is an interaction between age and difficulty of motor skills
tasks.
Hypothesis 2
It is shown the Age had a p-value of 0.00 which is less than the significance level thus the null
hypothesis is rejected and conclude that there is error mean difference between the two age
groups (young and old).
Hypothesis 3
It is shown the difficulty had a p-value of 0.00 which is less than the significance level thus the
null hypothesis is rejected and conclude that there is error mean difference between the two
difficulty levels (easy and hard)

9
Extra Credit. Provide an example (that has not been used in class or in your text) of a two-way
between subjects ANOVA. For full extra credit, state each IV (including all levels) and the DV
in sufficient detail below so that I am able to properly evaluate your example as being
appropriate for the two-way ANOVA. It is not necessary to provide values (i.e., data) with your
example.
A farmer seeks to examine if there is difference in the maize yield (dependent variable)
when he applies different level of nitrogen fertilizer; besides, the farmer wants to evaluate the
impact of different time of watering the plants on yield. There are four levels of nitrogen, which
include 10kg, 20kg, 30kg, and 40kg. Consequently, there are time levels, which include morning,
noon, and evening. Notably, nitrogen and time are independent variables

10