Statistics: Multiple Choice, Fill in Blank, and Answered Questions

This article covers multiple choice, fill in blank, and answered questions on statistics. It includes topics such as binomial probability, normal distribution, hypothesis testing, type 1 and type 2 errors, central limit theorem, distribution sample means, null and alternative hypothesis, significance level, and interval estimate.

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
University
Statistics
By
Your Name
Lecturers Name
Date
Page 1 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
Part A: Multiple Choice (1–10)
1. The cumulative probability distribution of a random variable X gives the probability
that X is less than or equal (Choice C) to x0, some specified value of X.
2. The probability of P (-1.4 < Z < 0.6) = 0.6449 (Choice B)
3. By using the binomial table, if the sample size is 20 and p equals to 0.70, the
value for P (X¿18) = 0.0279 (Choice A)
4. In a standard normal distribution, the area which lies between Z = -1.72 and
Z = 2.53 is 0.9516 (Choice C).
5. The value for 95% confidence interval for μ if s = 7.3, x = 84.2, and n = 40 is 81.937≤ μ ≤
86.463 (Choice A).
6. We say that sample results are significant when H0 is rejected (Choice B).
7. We commit a Type 1 error if we reject (Choice C) a true null hypothesis.
8. We say that sample results are significant when H0 is rejected (Choice B).
9. If random variable x has a normal distribution with μ=40 and σ = 6, the value of
P( X ≥ X0 )= 0.4 is 41.50 (Choice C).
10. The Z0 score for P( Z ≥ Z0)= 0.0708 is 1.47 (Choice A)
Page 2 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
Part B: Fill in Blank Question number 11-20
11. When the null hypothesis is true, the probability that the test statistic will fall in the
critical region is called significance level of the test.
12. There two statistical hypotheses. They are the null hypothesis and the alternative
hypothesis.
13. A type 1 error occurs when the investigator rejects the null hypothesis when it is
actually true.
14. Values of the test statistic that separate the acceptance region from the region of rejection
are called critical values.
15. The probability of obtaining a value of the test statistic as extreme as or more extreme
than actually obtained, given that the tested null hypothesis is true is called the p-value
for the hypothesis test.
16. When one is testing H0: μ= μ0 on the basis of data from a sample of size n from a
normally distributed population with a known variance of σ2, the test statistic is Z-
statistics.
17. The null hypothesis contains a statement of equality or no difference.
18. The statement μ ≥ 0 is an inappropriate statement for the alternative hypothesis.
19. The null hypothesis and the alternative hypothesis are compliments of each other.
20. For the normal distribution, the observation closer to the middle will occur with
increasing frequency.
Page 3 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
PART C: ANSWER THE FOLLOWING QUESTIONS (21-30)
21. The difference between discrete random variable and continuous random variable.
Discrete random variables can only take a finite number of values. Example is the
number of posts used in electrical installation. On the other hand, the continuous
random variable takes values within a given a range or interval. Example is the
height of an individual.
22. Description of the normal distribution.
This is a distribution that gives a bell-shaped curve while giving a description of the
spread of a characteristic throughout a population.
23. When the Z-test should be used and when t-test should be used.
The Z-test should be used when there is a large sample size i.e. n is greater than 30
or when the standard deviation is known for any sample size. On the other hand, t-
test should be used when the sample size is small. i.e. n<30 or when the value of the s
is given or has to be determined.
24. The Q’ method with regards to Binomial Probability.
The Q method in binomial probability involves defining the success for an
individual trial, then the probability of the success is determined. After that, the
probability of failure is determined. The number of trials is defined and finally the
successes number out of the trial (Bruce, 2015).
25. Difference between Type 1 error and Type II error.
A Type I error is an error committed in statistics when the null hypothesis is
rejected but it is true. It is also known as a false positive. On the other hand, a type
Page 4 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
11 error is committed when we fail to reject the null hypothesis when in real else it is
false. It is also known as a false negative (Hinton, 2014) .
26. The Central Limit Theorem.
According to the central limit theorem, the sampling distribution for any
distribution in statistics is normal or close to normal when the sample size large
enough is selected. The sample size is determined based on the accuracy needed and
the shape of the population under consideration.
27. Distribution sample means.
This refers to the theoretical distribution of values of a sample which the mean of
the sample takes in all the possible sample combinations of given sizes made from
the selected population.
28. The null and alternative hypothesis
The null hypothesis refers to the hypothesis that indicate that the population
parameter is equal to or there is no difference with a given specification. On the
other hand, the alternative hypothesis is the compliment of the null hypothesis and
it indicates non-equality or existence of difference with a given specification.
29. The significance level.
This refers to a value in statistical hypothesis test which indicates the boundary of
the region below which a conclusion can be drawn that a given p-value shows
significant evidence in favor of the alternative hypothesis or against the null
hypothesis.
30. What is Interval Estimate.
Page 5 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
This is where an interval is developed around the point estimate and the interval is
likely to cover the corresponding population parameters.
Part D: Answers to Question 31-44
31. The problem 5 of page 6-13 (a-e) following given instructions
a) Using Binomial Formula
P (x=1 | n =10, p = 0.40)
p ( x ) = n !
x ! ( n−x ) ! π x (1−π )n−x
p ( x ) = 10 !
1 ! ( 10−1 ) ! 0.401 (1−0.4)10−1
p ( x )=3628800
362880 0.41 (0.6)9
p ( x ) =0.0403
b) Using Binomial table
P (x=2 | n =10, p = 0.70)
Checking from the binomial table the answer is 0.002
c) Using Binomial table
P (x≤16 | n =50, p = 0.50)
p ( x ≤ 16 ) = p ( x=0 ) + p ( x=1 ) + p ( x=2 ) +… …+ P (x=16)
Checking the various probabilities from the
table and summing them gives the answer as
0.0767
d) Using Binomial table
P (x<5 | n =10, p = 0.60)
p ( x<5 ) = p ( x=0 ) + p ( x=1 ) + p ( x=2 ) +… …+ P( x=4)
Checking the probability and summing them from the
table gives the answer as 0.1662
e) Using Binomial table
Page 6 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
P (x≥ 8 | n =50, p = 0.80)
p ( x ≥ 8 )=(1− p ( x <8 ) )
p ( x ≥ 8 ) =[1− { p ( x=7 ) + p ( x=6 ) + … …+ P ( x =0 ) } ]
Checking the values from the table and carrying out the computation above gives the answer as 1.000
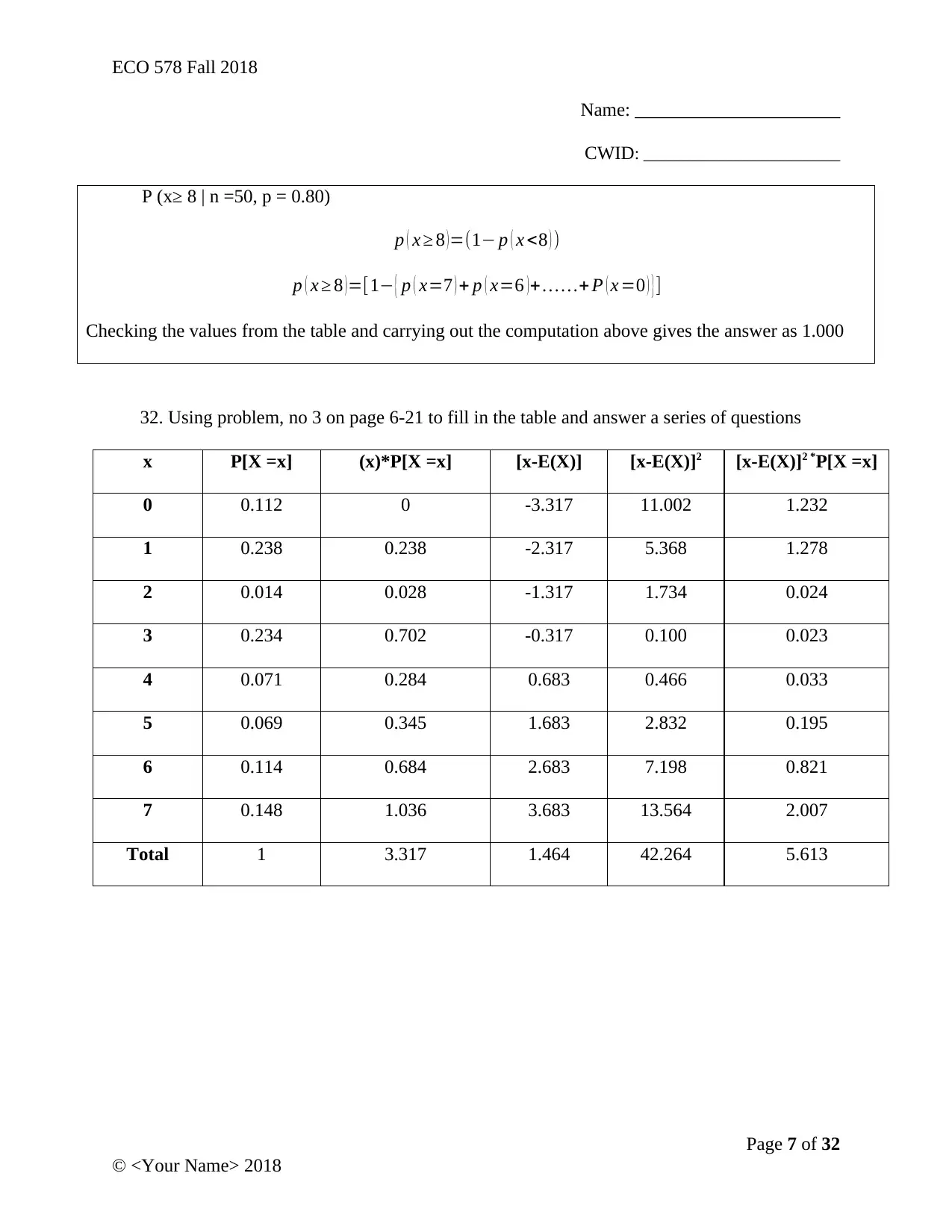
32. Using problem, no 3 on page 6-21 to fill in the table and answer a series of questions
x P[X =x] (x)*P[X =x] [x-E(X)] [x-E(X)]2 [x-E(X)]2 *P[X =x]
0 0.112 0 -3.317 11.002 1.232
1 0.238 0.238 -2.317 5.368 1.278
2 0.014 0.028 -1.317 1.734 0.024
3 0.234 0.702 -0.317 0.100 0.023
4 0.071 0.284 0.683 0.466 0.033
5 0.069 0.345 1.683 2.832 0.195
6 0.114 0.684 2.683 7.198 0.821
7 0.148 1.036 3.683 13.564 2.007
Total 1 3.317 1.464 42.264 5.613
Page 7 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
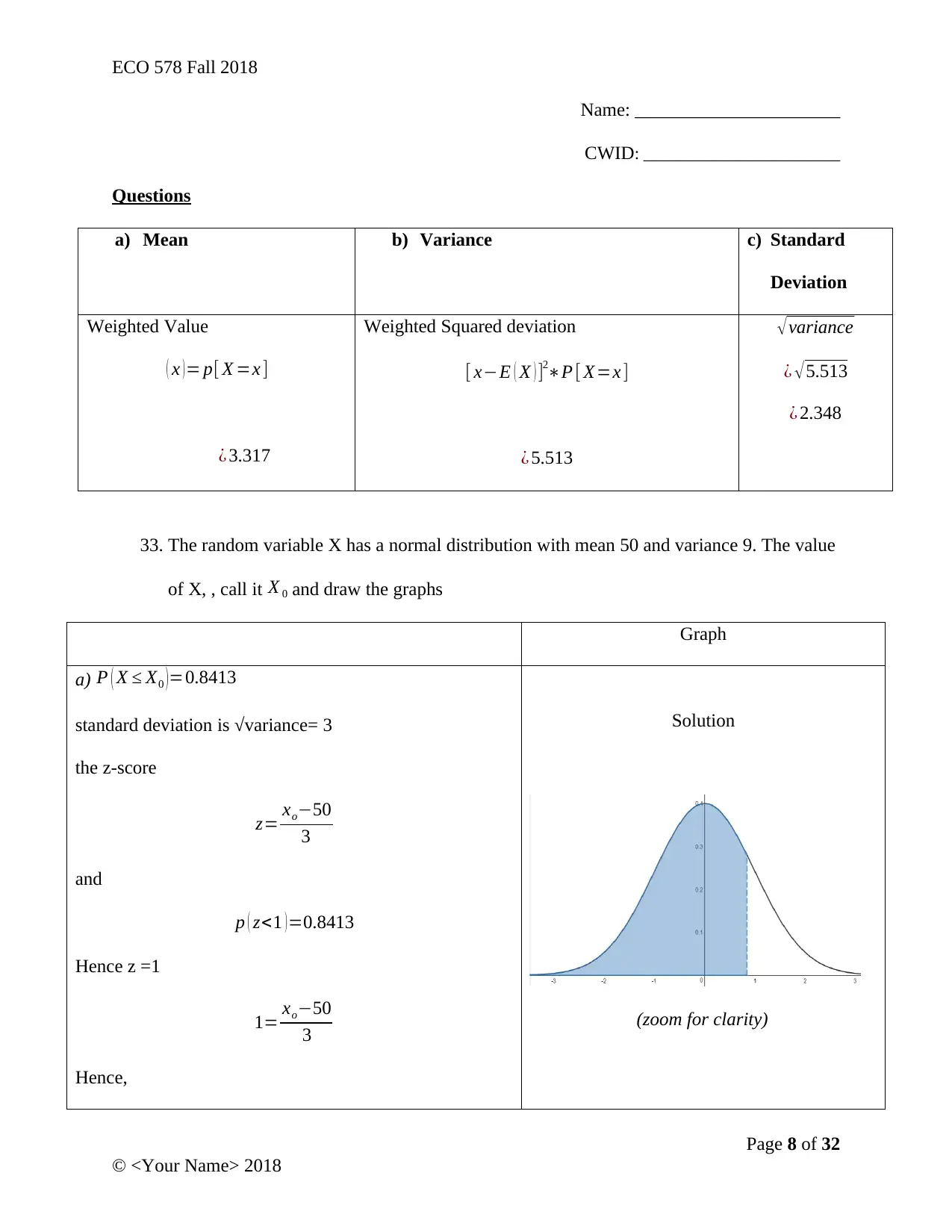
Questions
a) Mean b) Variance c) Standard
Deviation
Weighted Value
( x )= p[ X =x ]
¿ 3.317
Weighted Squared deviation
[ x−E ( X ) ]2∗P [ X=x ]
¿ 5.513
√variance
¿ √ 5.513
¿ 2.348
33. The random variable X has a normal distribution with mean 50 and variance 9. The value
of X, , call it X 0 and draw the graphs
Graph
a) P ( X ≤ X0 )=0.8413
standard deviation is √variance= 3
the z-score
z= xo−50
3
and
p ( z<1 ) =0.8413
Hence z =1
1= xo−50
3
Hence,
Solution
(zoom for clarity)
Page 8 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
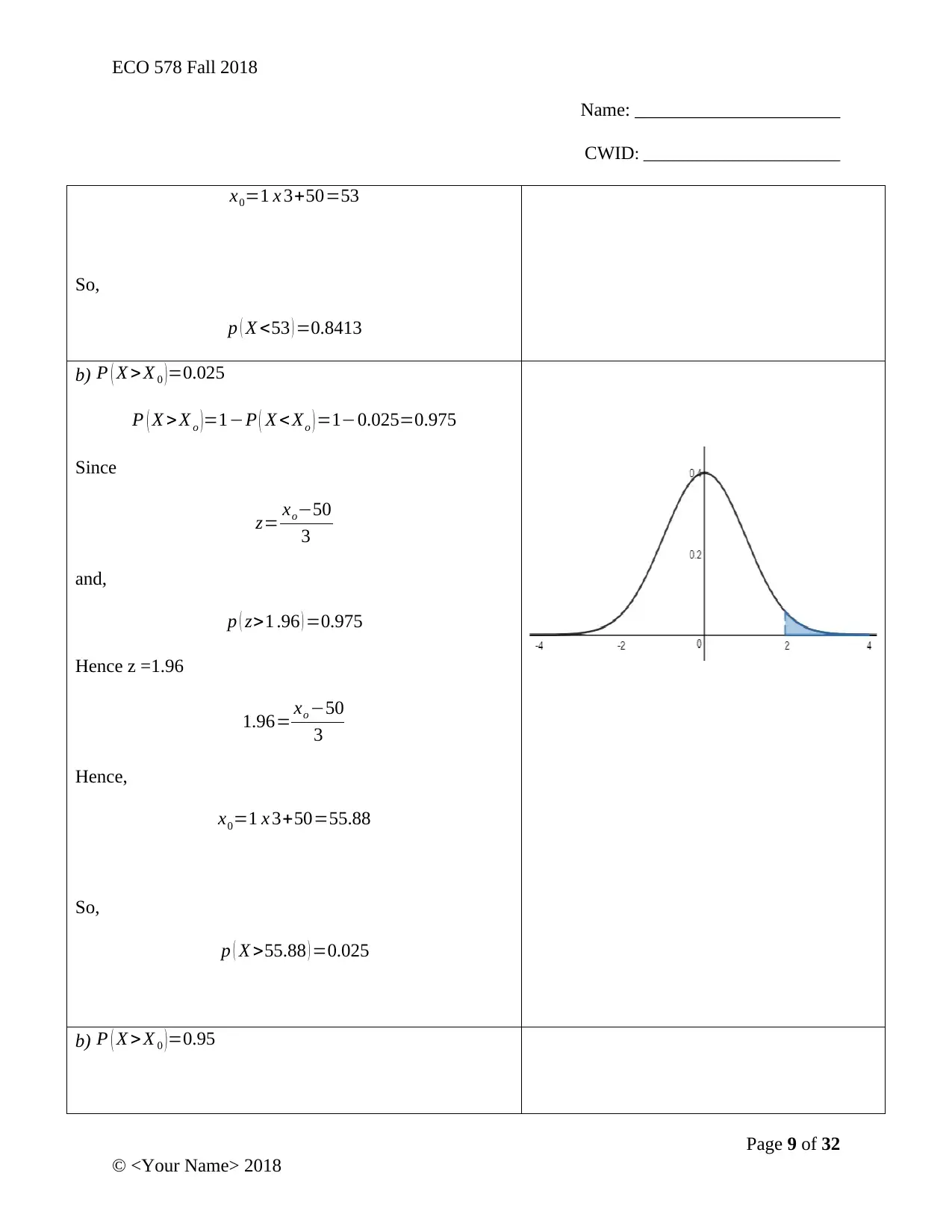
x0=1 x 3+50=53
So,
p ( X <53 ) =0.8413
b) P ( X > X 0 )=0.025
P ( X > X o )=1−P ( X < Xo ) =1−0.025=0.975
Since
z= xo−50
3
and,
p ( z>1 .96 ) =0.975
Hence z =1.96
1.96= xo −50
3
Hence,
x0=1 x 3+50=55.88
So,
p ( X >55.88 ) =0.025
b) P ( X > X 0 )=0.95
Page 9 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
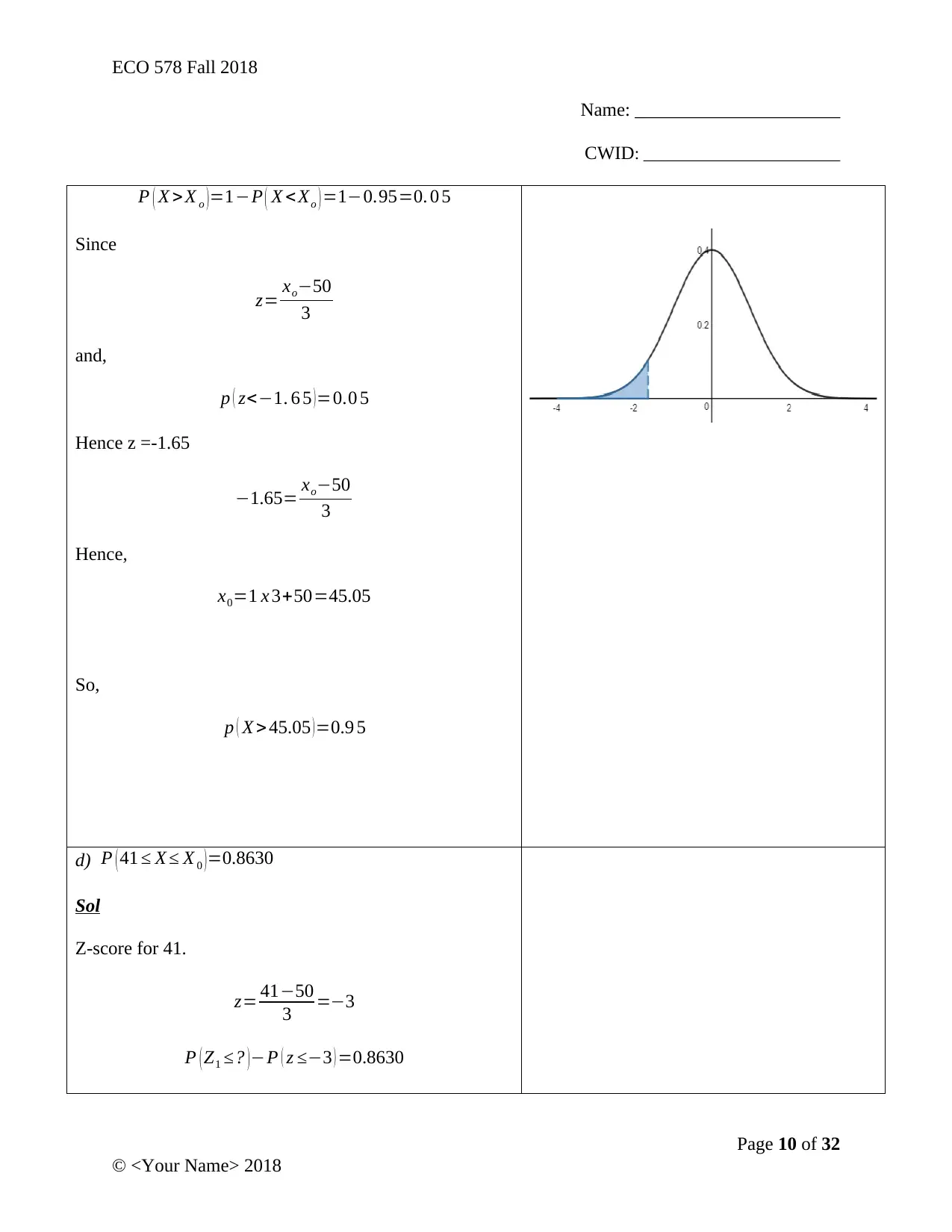
P ( X > X o ) =1−P ( X < Xo ) =1−0.95=0. 0 5
Since
z= xo−50
3
and,
p ( z<−1. 6 5 )=0.0 5
Hence z =-1.65
−1.65= xo−50
3
Hence,
x0=1 x 3+50=45.05
So,
p ( X > 45.05 )=0.9 5
d) P ( 41 ≤ X ≤ X 0 )=0.8630
Sol
Z-score for 41.
z= 41−50
3 =−3
P ( Z1 ≤? ) −P ( z ≤−3 ) =0.8630
Page 10 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
p ( z1 ≤ ? ) −0.0013=0.8680
p ( z1 ≤ ? ) =0.8680+0.0013=0.8643
From the z-table
p ( z1 ≤ 1.01 )=0.8643
Hence Z1=1.01
z= xo−50
3 =1.01
xo=53.03
34. The problem no 10 on page 7-63 and the resulting graphs
a. Graphs
b. a. P ( X > 15.95 | n=80, σ =1, μ =15.9)
Z= X−μ
σ
√ N
Z=15.95−15.9
1
√ 80
=0.447
P ( X > 15.95)= P ( Z> 0.447)=0.3274
Page 11 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
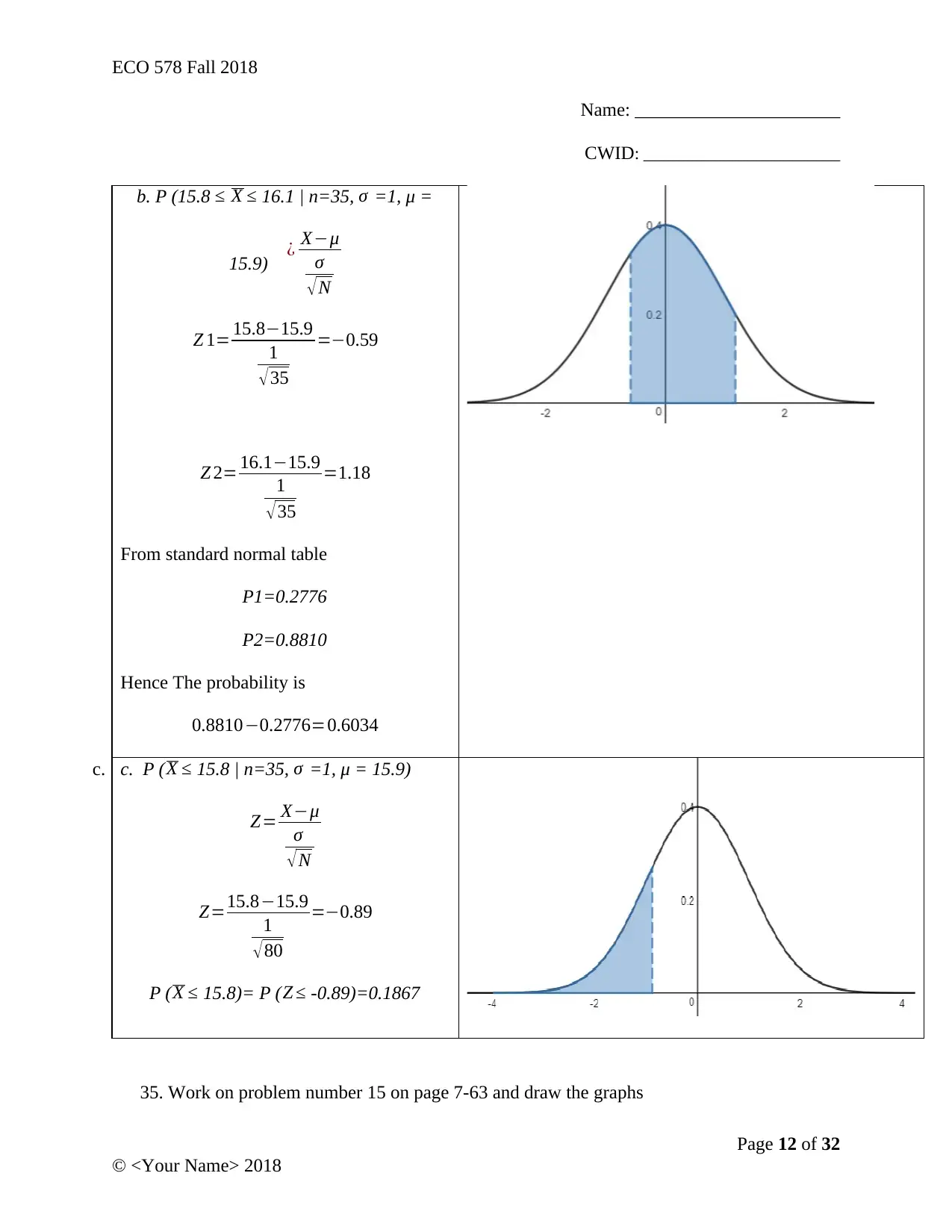
b. P (15.8 ≤ X ≤ 16.1 | n=35, σ =1, μ =
15.9) ¿ X−μ
σ
√N
Z 1= 15.8−15.9
1
√ 35
=−0.59
Z 2= 16.1−15.9
1
√ 35
=1.18
From standard normal table
P1=0.2776
P2=0.8810
Hence The probability is
0.8810−0.2776=0.6034
c. c. P ( X ≤ 15.8 | n=35, σ =1, μ = 15.9)
Z= X−μ
σ
√ N
Z=15.8−15.9
1
√ 80
=−0.89
P ( X ≤ 15.8)= P ( Z ≤ -0.89)=0.1867
35. Work on problem number 15 on page 7-63 and draw the graphs
Page 12 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
a. Graphs
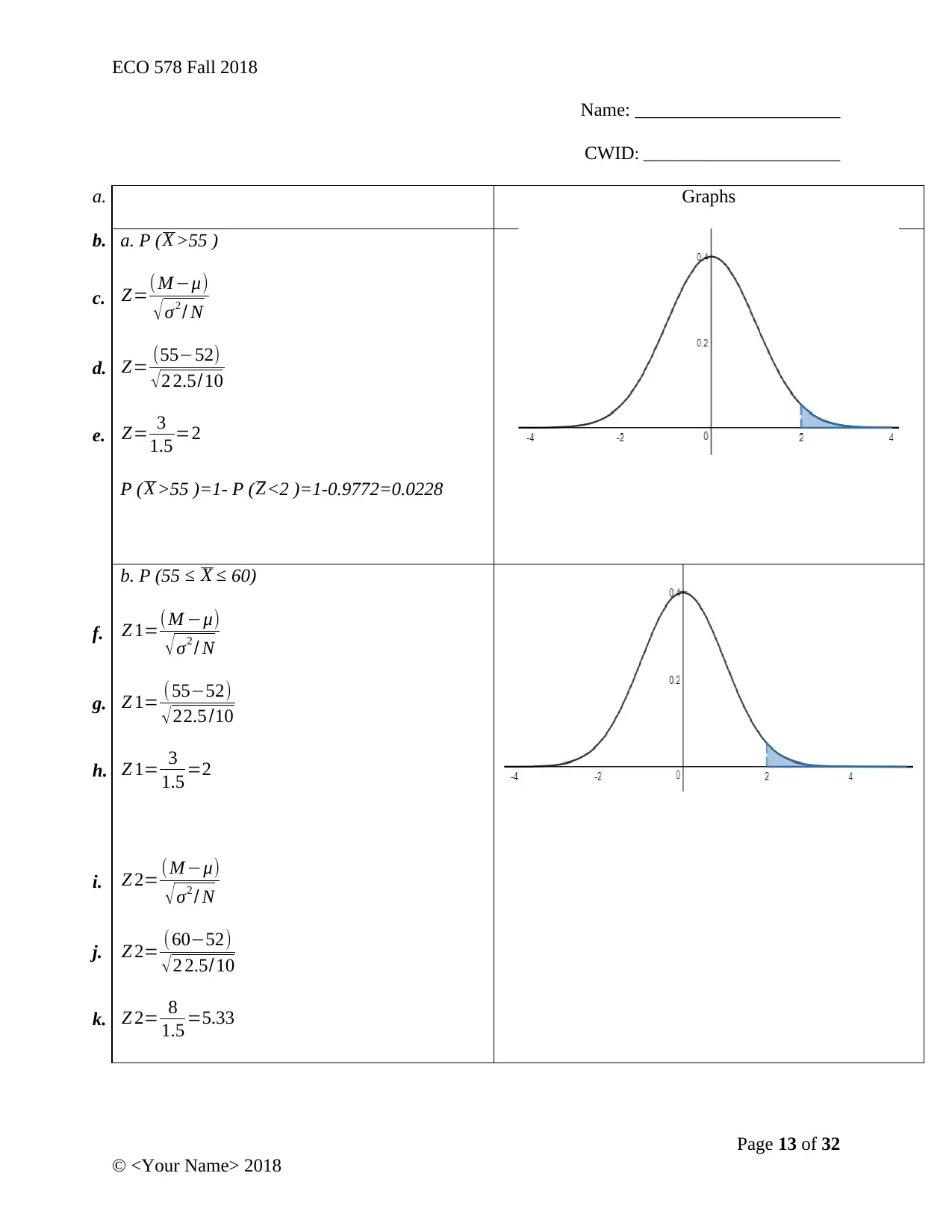
b. a. P (X >55 )
c. Z=(M−μ)
√ σ2 / N
d. Z= (55−52)
√ 2 2.5/10
e. Z= 3
1.5 =2
P ( X >55 )=1- P ( Z<2 )=1-0.9772=0.0228
b. P (55 ≤ X ≤ 60)
f. Z 1=( M −μ)
√σ2 / N
g. Z 1= (55−52)
√ 22.5 /10
h. Z 1= 3
1.5 =2
i. Z 2= (M −μ)
√σ2 / N
j. Z 2= (60−52)
√2 2.5/10
k. Z 2= 8
1.5 =5.33
Page 13 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
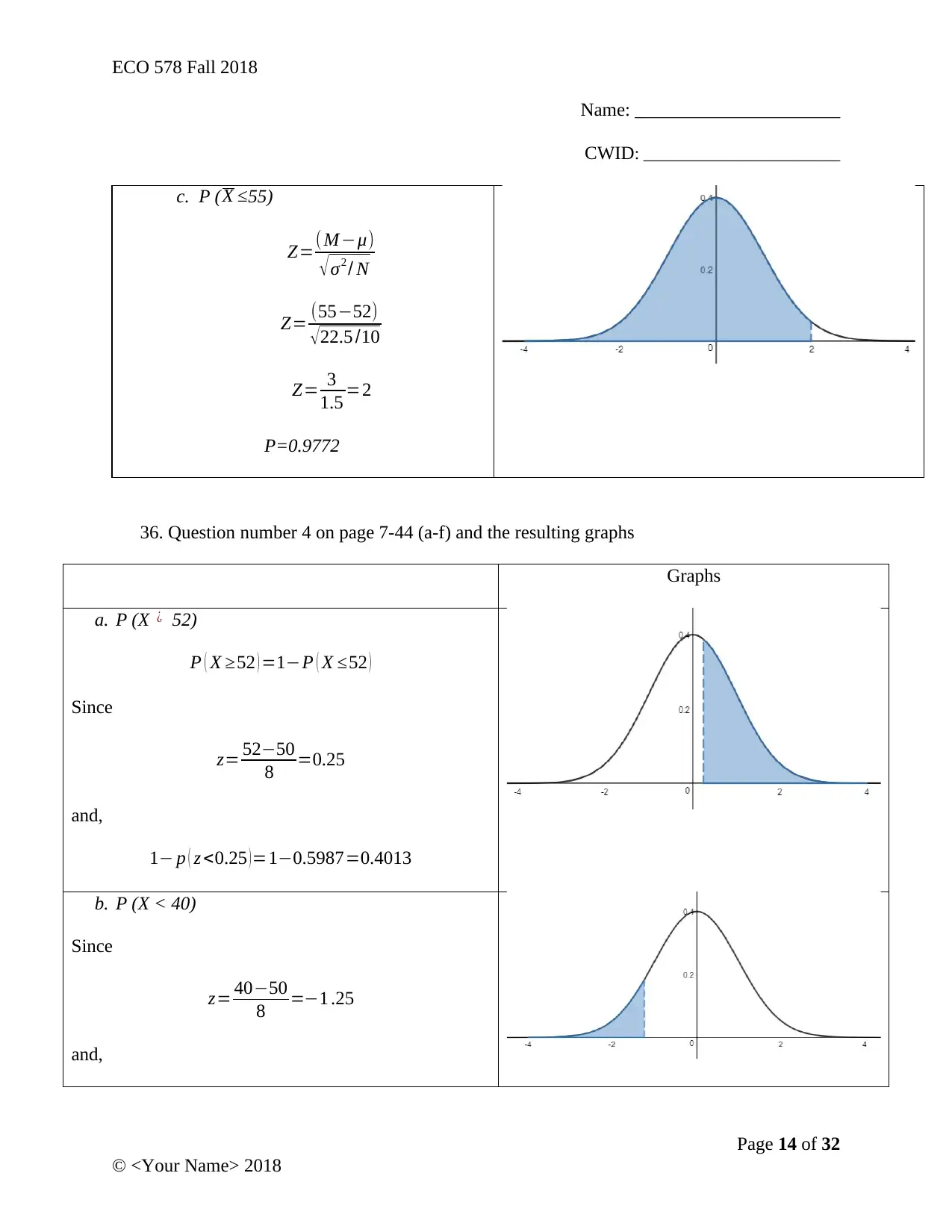
c. P ( X ≤55)
Z=( M−μ)
√ σ2 / N
Z= (55−52)
√22.5 /10
Z= 3
1.5 =2
P=0.9772
36. Question number 4 on page 7-44 (a-f) and the resulting graphs
Graphs
a. P (X ¿ 52)
P ( X ≥52 ) =1−P ( X ≤52 )
Since
z= 52−50
8 =0.25
and,
1− p ( z <0.25 )=1−0.5987=0.4013
b. P (X < 40)
Since
z= 40−50
8 =−1 .25
and,
Page 14 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
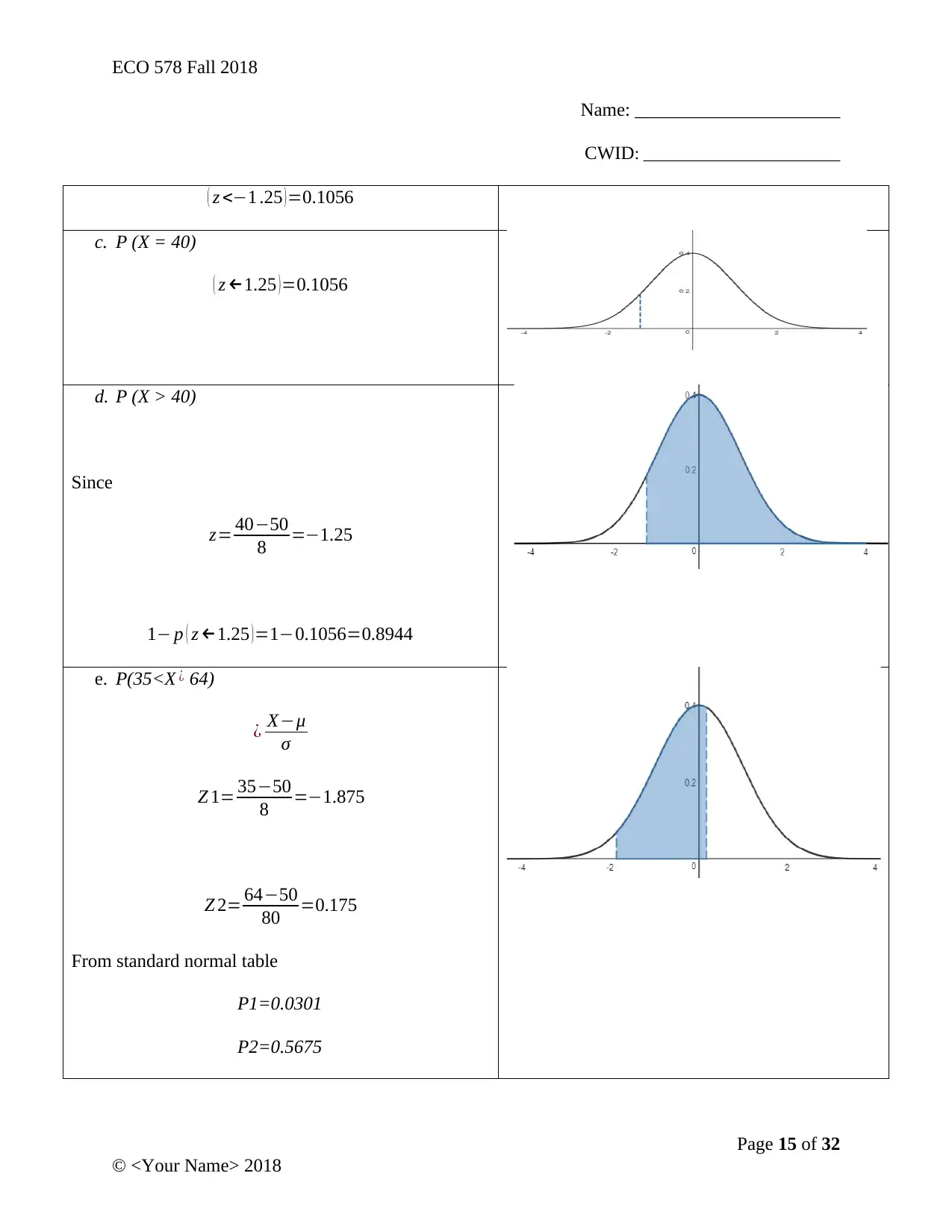
( z <−1 .25 )=0.1056
c. P (X = 40)
( z ←1.25 )=0.1056
d. P (X > 40)
Since
z= 40−50
8 =−1.25
1− p ( z ←1.25 )=1−0.1056=0.8944
e. P(35<X ¿ 64)
¿ X−μ
σ
Z 1= 35−50
8 =−1.875
Z 2= 64−50
80 =0.175
From standard normal table
P1=0.0301
P2=0.5675
Page 15 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
Hence The probability is
0.5675−0.0301=0.5374
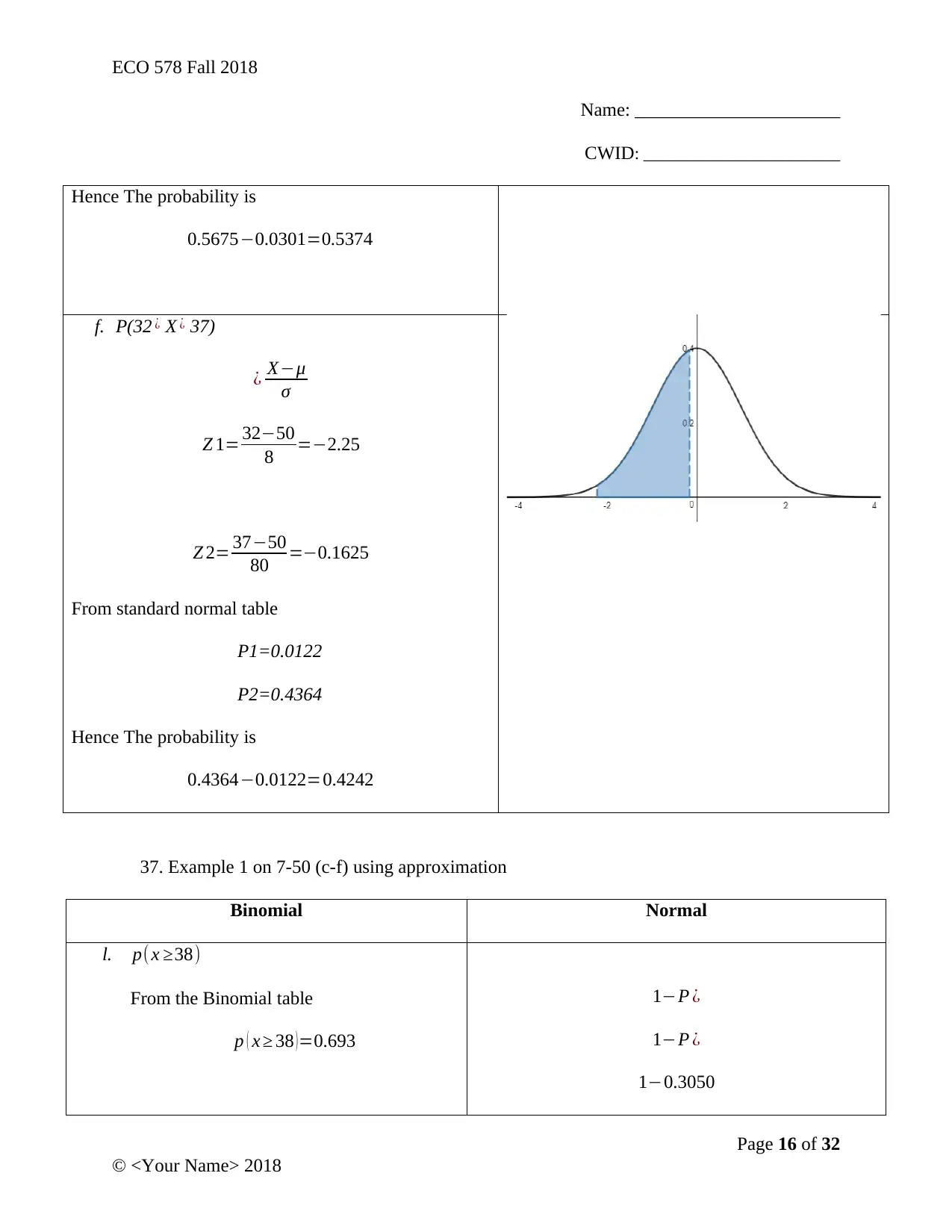
f. P(32 ¿ X ¿ 37)
¿ X−μ
σ
Z 1= 32−50
8 =−2.25
Z 2= 37−50
80 =−0.1625
From standard normal table
P1=0.0122
P2=0.4364
Hence The probability is
0.4364−0.0122=0.4242
37. Example 1 on 7-50 (c-f) using approximation
Binomial Normal
l. p(x ≥38)
From the Binomial table
p ( x ≥ 38 )=0.693
1−P ¿
1−P ¿
1−0.3050
Page 16 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
¿ 0.695
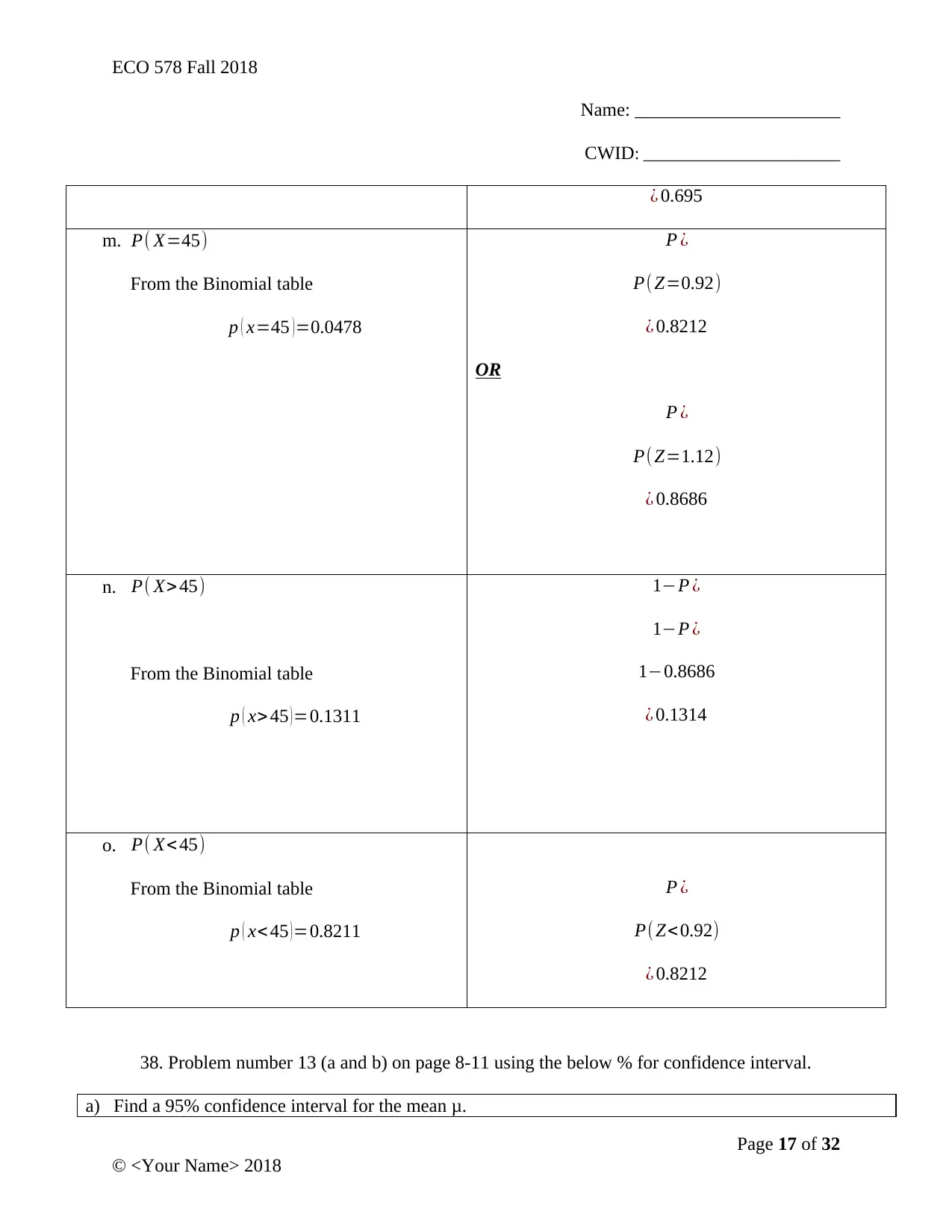
m. P( X=45)
From the Binomial table
p ( x=45 )=0.0478
P ¿
P( Z=0.92)
¿ 0.8212
OR
P ¿
P( Z=1.12)
¿ 0.8686
n. P( X>45)
From the Binomial table
p ( x> 45 )=0.1311
1−P ¿
1−P ¿
1−0.8686
¿ 0.1314
o. P( X<45)
From the Binomial table
p ( x< 45 )=0.8211
P ¿
P( Z<0.92)
¿ 0.8212
38. Problem number 13 (a and b) on page 8-11 using the below % for confidence interval.
a) Find a 95% confidence interval for the mean μ.
Page 17 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
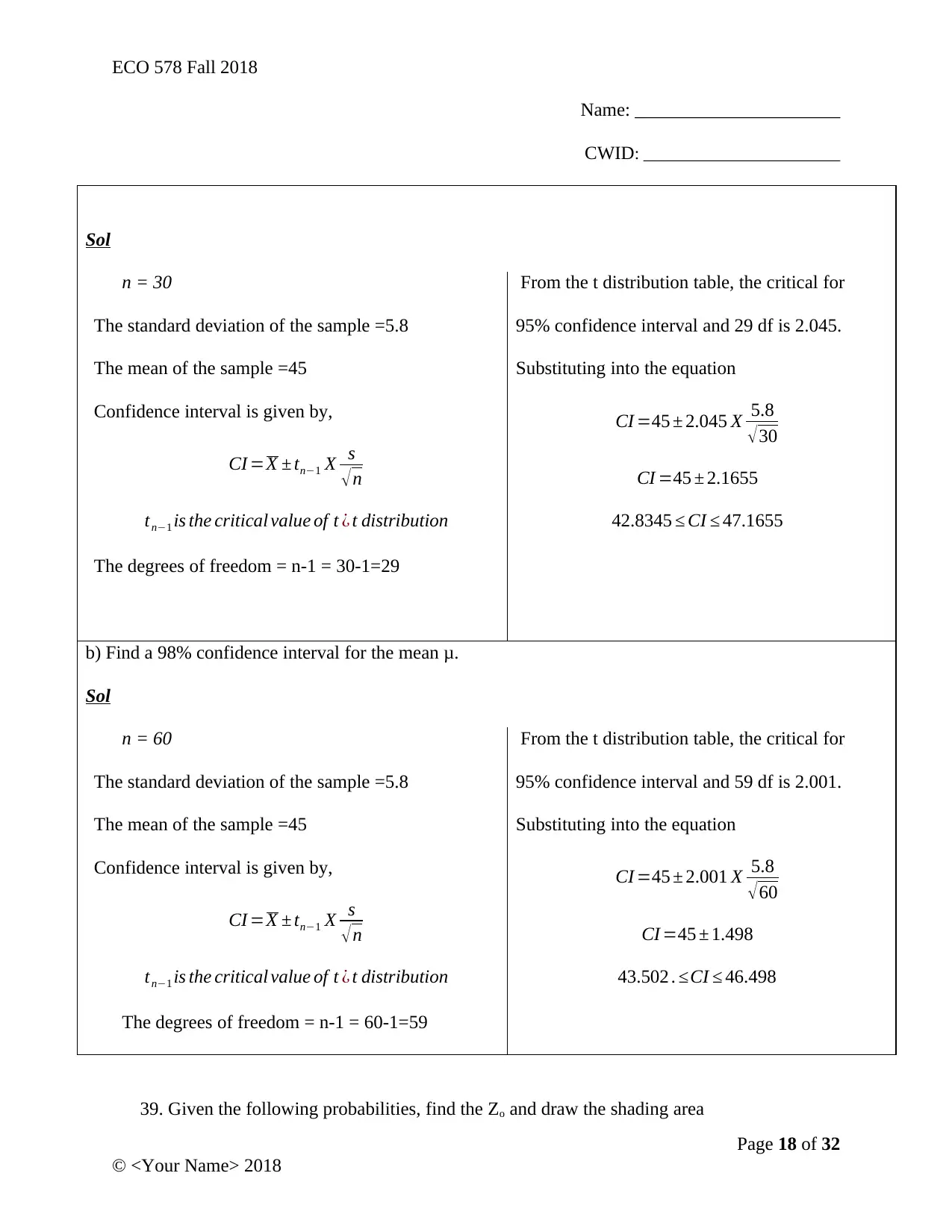
Sol
n = 30
The standard deviation of the sample =5.8
The mean of the sample =45
Confidence interval is given by,
CI =X ± tn−1 X s
√ n
tn−1 is the critical value of t ¿ t distribution
The degrees of freedom = n-1 = 30-1=29
From the t distribution table, the critical for
95% confidence interval and 29 df is 2.045.
Substituting into the equation
CI =45 ± 2.045 X 5.8
√30
CI =45 ± 2.1655
42.8345 ≤ CI ≤ 47.1655
b) Find a 98% confidence interval for the mean μ.
Sol
n = 60
The standard deviation of the sample =5.8
The mean of the sample =45
Confidence interval is given by,
CI =X ± tn−1 X s
√ n
tn−1 is the critical value of t ¿ t distribution
The degrees of freedom = n-1 = 60-1=59
From the t distribution table, the critical for
95% confidence interval and 59 df is 2.001.
Substituting into the equation
CI =45 ± 2.001 X 5.8
√ 60
CI =45 ± 1.498
43.502 . ≤CI ≤ 46.498
39. Given the following probabilities, find the Zo and draw the shading area
Page 18 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
Show your work graphs
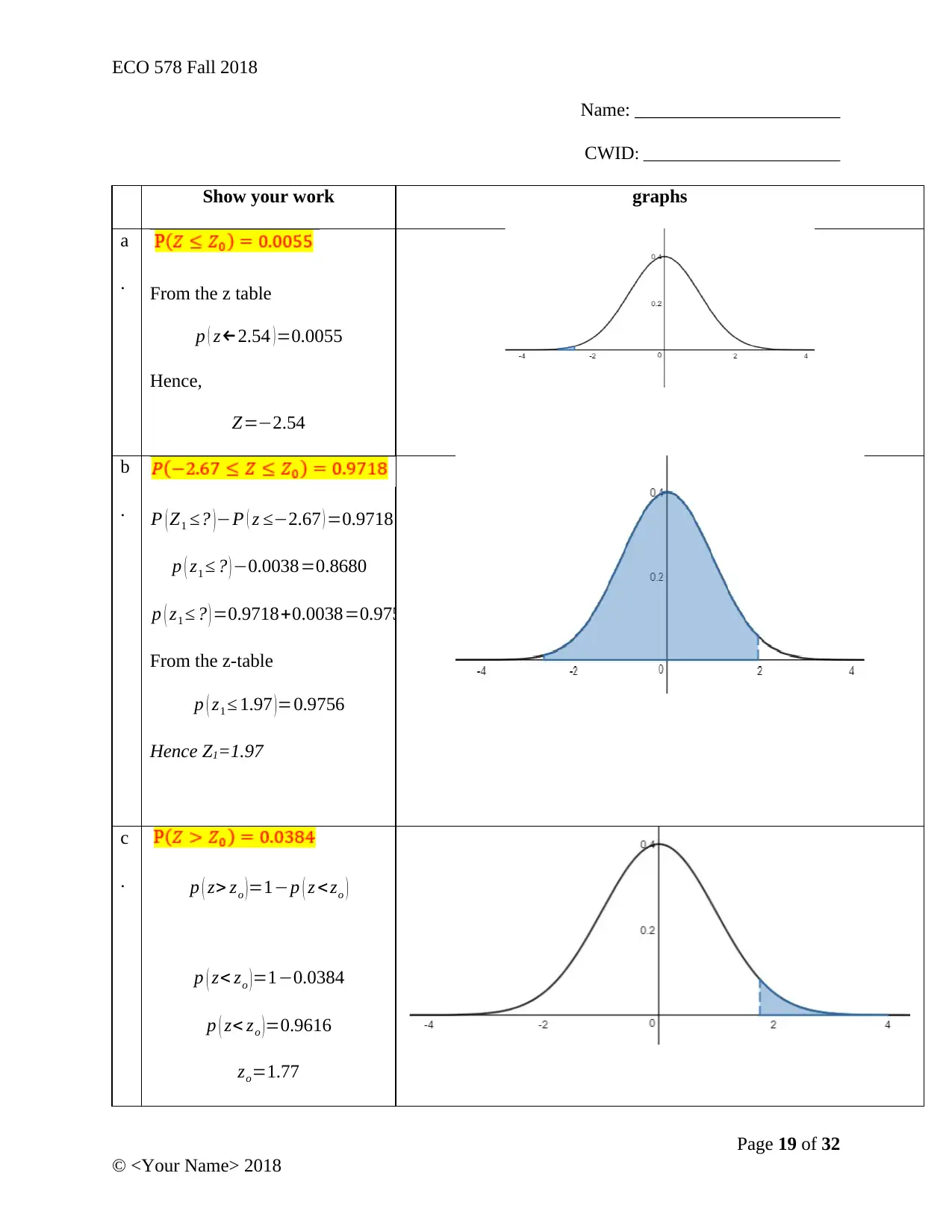
a
. From the z table
p ( z←2.54 )=0.0055
Hence,
Z=−2.54
b
. P ( Z1 ≤? )−P ( z ≤−2.67 ) =0.9718
p ( z1 ≤ ? ) −0.0038=0.8680
p ( z1 ≤ ? ) =0.9718+0.0038=0.9756
From the z-table
p ( z1 ≤ 1.97 )=0.9756
Hence Z1=1.97
c
. p ( z> zo ) =1−p ( z <zo )
p ( z< zo )=1−0.0384
p ( z< zo ) =0.9616
zo=1.77
Page 19 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
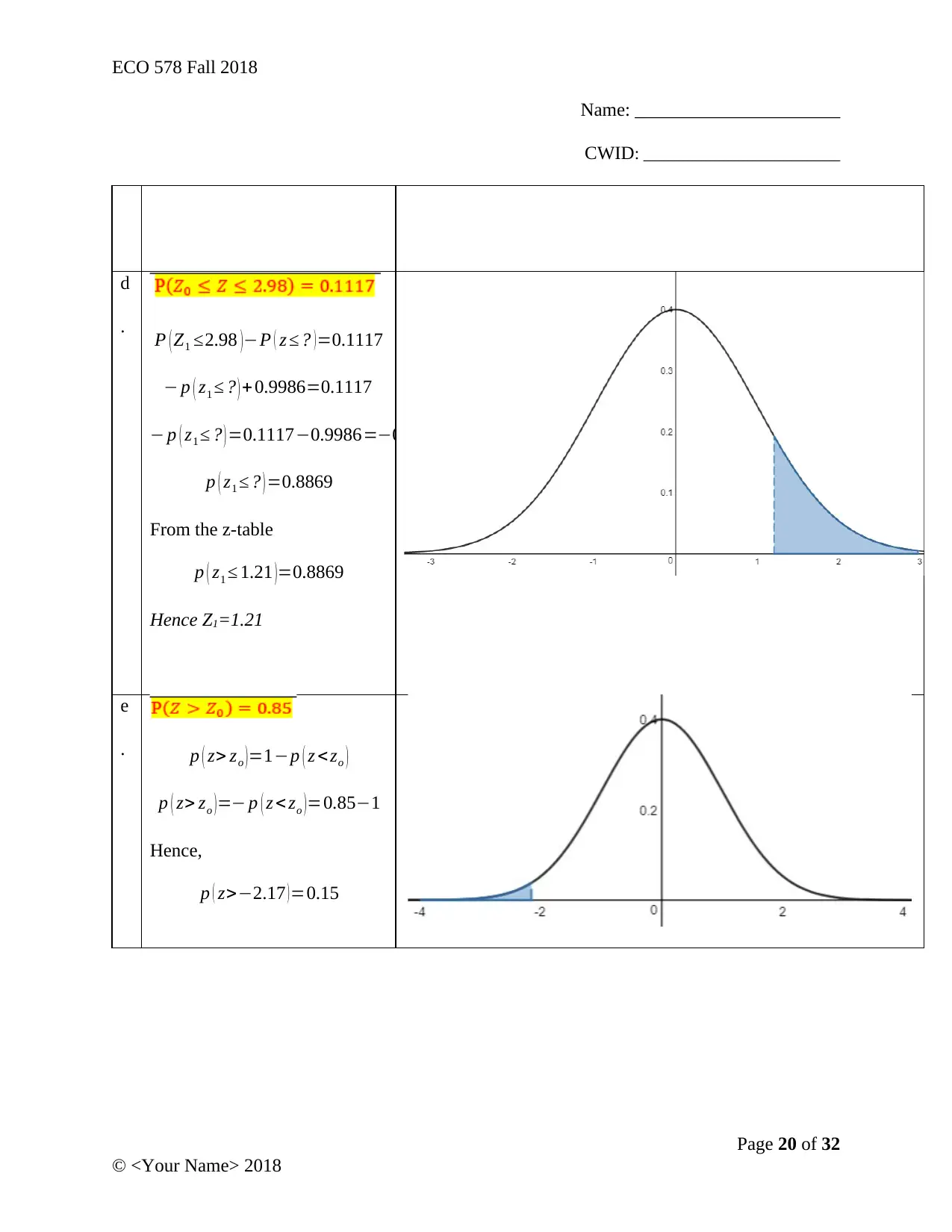
d
. P ( Z1 ≤2.98 )−P ( z ≤ ? )=0.1117
− p ( z1 ≤ ? ) +0.9986=0.1117
− p ( z1 ≤ ? ) =0.1117−0.9986=−0.8869
p ( z1 ≤ ? ) =0.8869
From the z-table
p ( z1 ≤ 1.21 )=0.8869
Hence Z1=1.21
e
. p ( z> zo )=1−p ( z <zo )
p ( z> zo ) =− p ( z < zo )=0.85−1
Hence,
p ( z>−2.17 )=0.15
Page 20 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
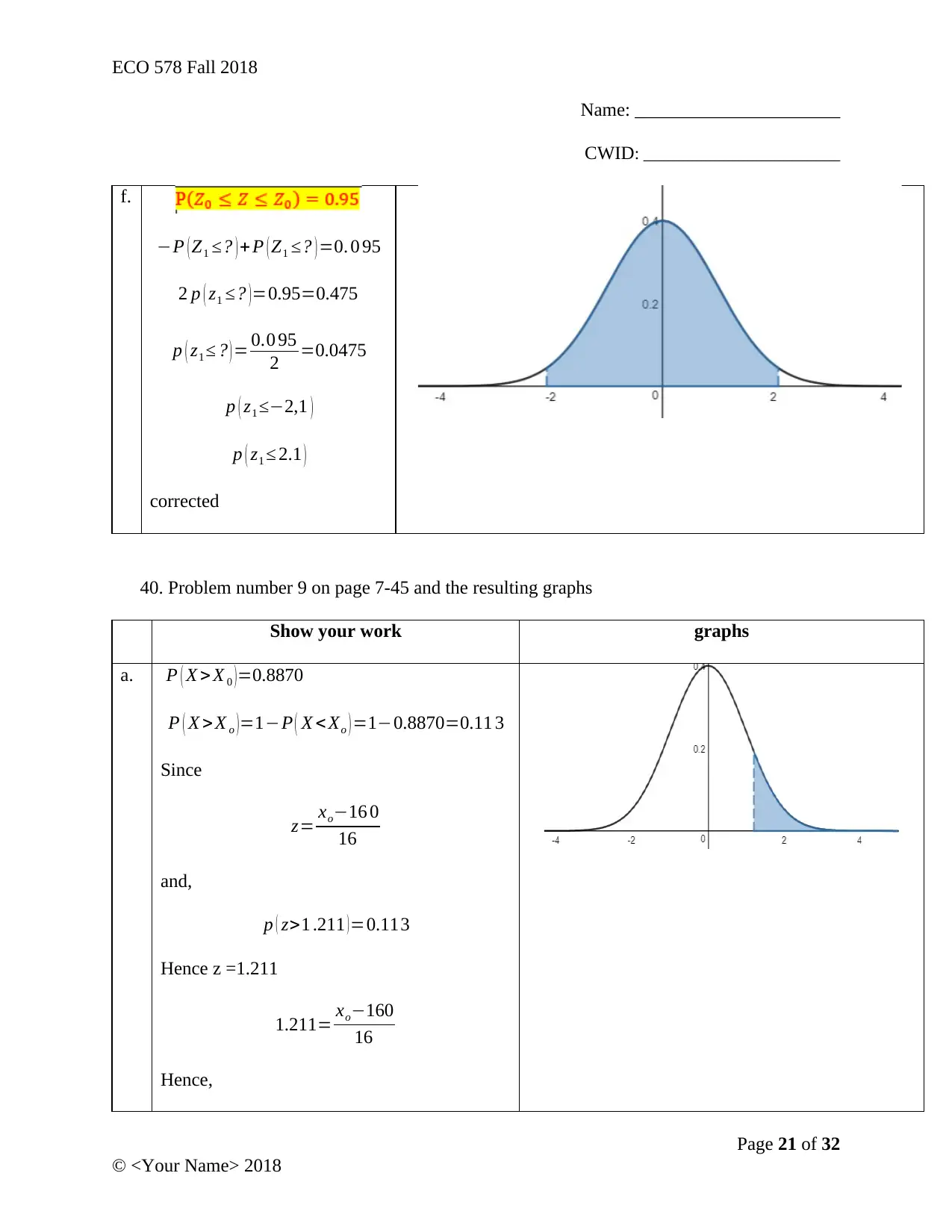
f.
−P ( Z1 ≤? ) + P ( Z1 ≤? ) =0. 0 95
2 p ( z1 ≤? )=0.95=0.475
p ( z1 ≤ ? ) = 0.0 95
2 =0.0475
p ( z1 ≤−2,1 )
p ( z1 ≤ 2.1 )
corrected
40. Problem number 9 on page 7-45 and the resulting graphs
Show your work graphs
a. P ( X > X 0 ) =0.8870
P ( X >X o )=1−P ( X < Xo ) =1−0.8870=0.11 3
Since
z= xo−16 0
16
and,
p ( z>1 .211 )=0.113
Hence z =1.211
1.211= xo−160
16
Hence,
Page 21 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
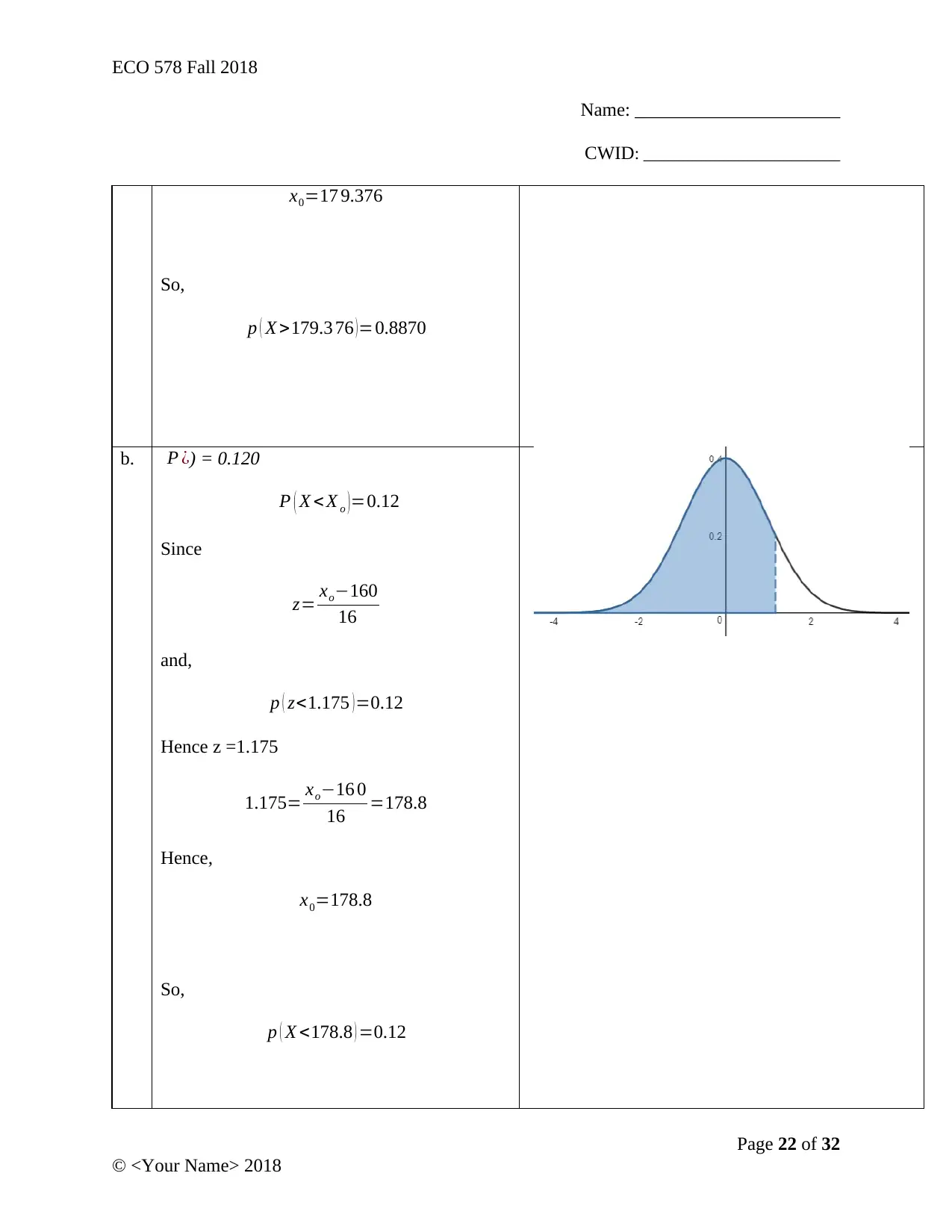
x0=17 9.376
So,
p ( X >179.3 76 )=0.8870
b. P ¿) = 0.120
P ( X < X o ) =0.12
Since
z= xo−160
16
and,
p ( z<1.175 )=0.12
Hence z =1.175
1.175= xo−16 0
16 =178.8
Hence,
x0=178.8
So,
p ( X <178.8 ) =0.12
Page 22 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
c. P ¿) = 0.97
P ( X <X o )=0.97
Since
z= xo−160
16
and,
p ( z<1.881 )=0.97
Hence z =1.881
1.881= xo−16 0
16 =190.09 6
Hence,
x0=190.096
So,
p ( X <190.096 ) =0.97
d. P ¿) = 0.4808
p 1= p(x <136)
p 2= p(x < xo)
p 2− p 1
zi= 136−160
16 =−1.5
p 1=0.0668
p= p 2− p 1
Page 23 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
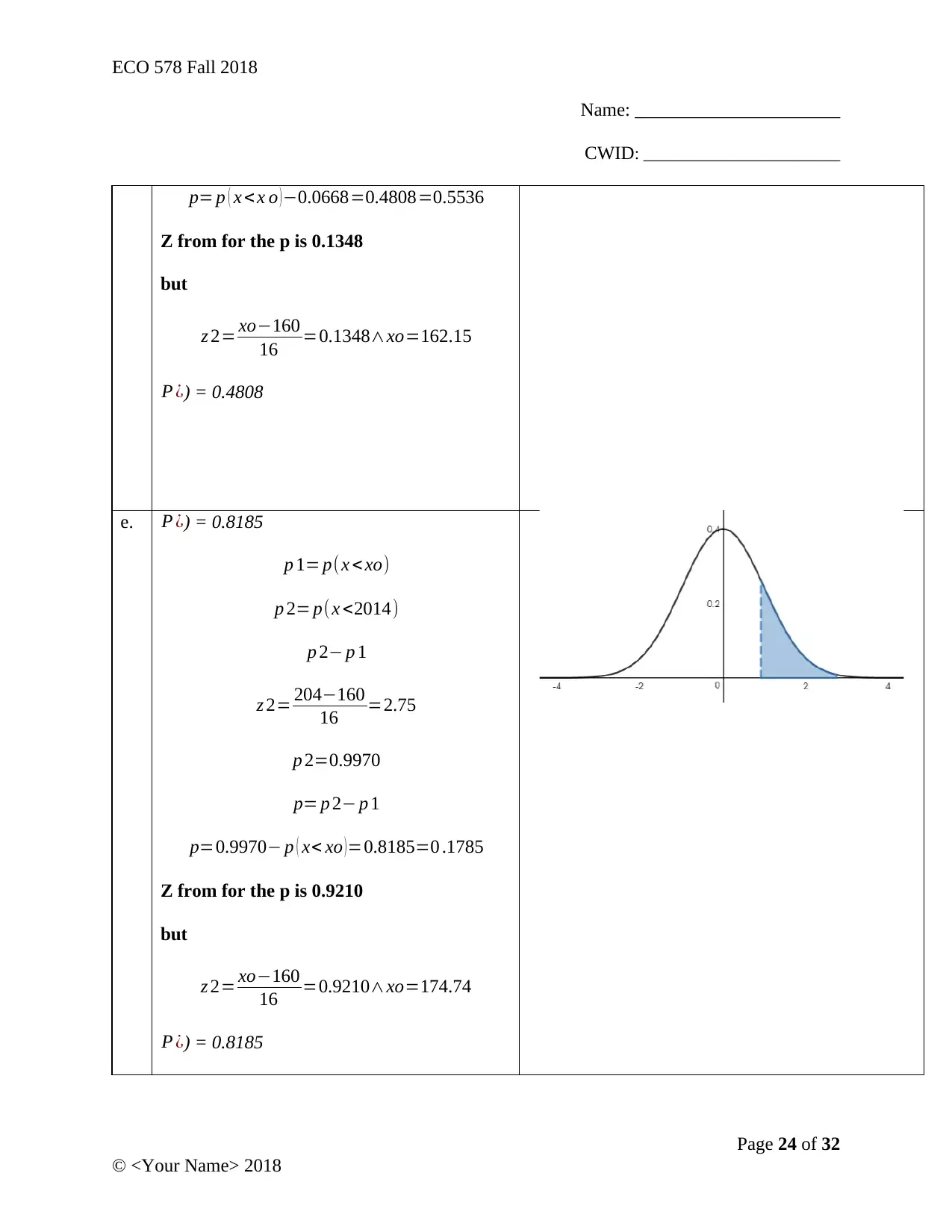
p= p ( x < x o )−0.0668=0.4808=0.5536
Z from for the p is 0.1348
but
z 2= xo−160
16 =0.1348∧xo=162.15
P ¿) = 0.4808
e. P ¿) = 0.8185
p 1= p(x < xo)
p 2= p(x <2014)
p 2− p 1
z 2= 204−160
16 =2.75
p 2=0.9970
p= p 2− p 1
p=0.9970− p ( x< xo )=0.8185=0 .1785
Z from for the p is 0.9210
but
z 2= xo−160
16 =0.9210∧xo=174.74
P ¿) = 0.8185
Page 24 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
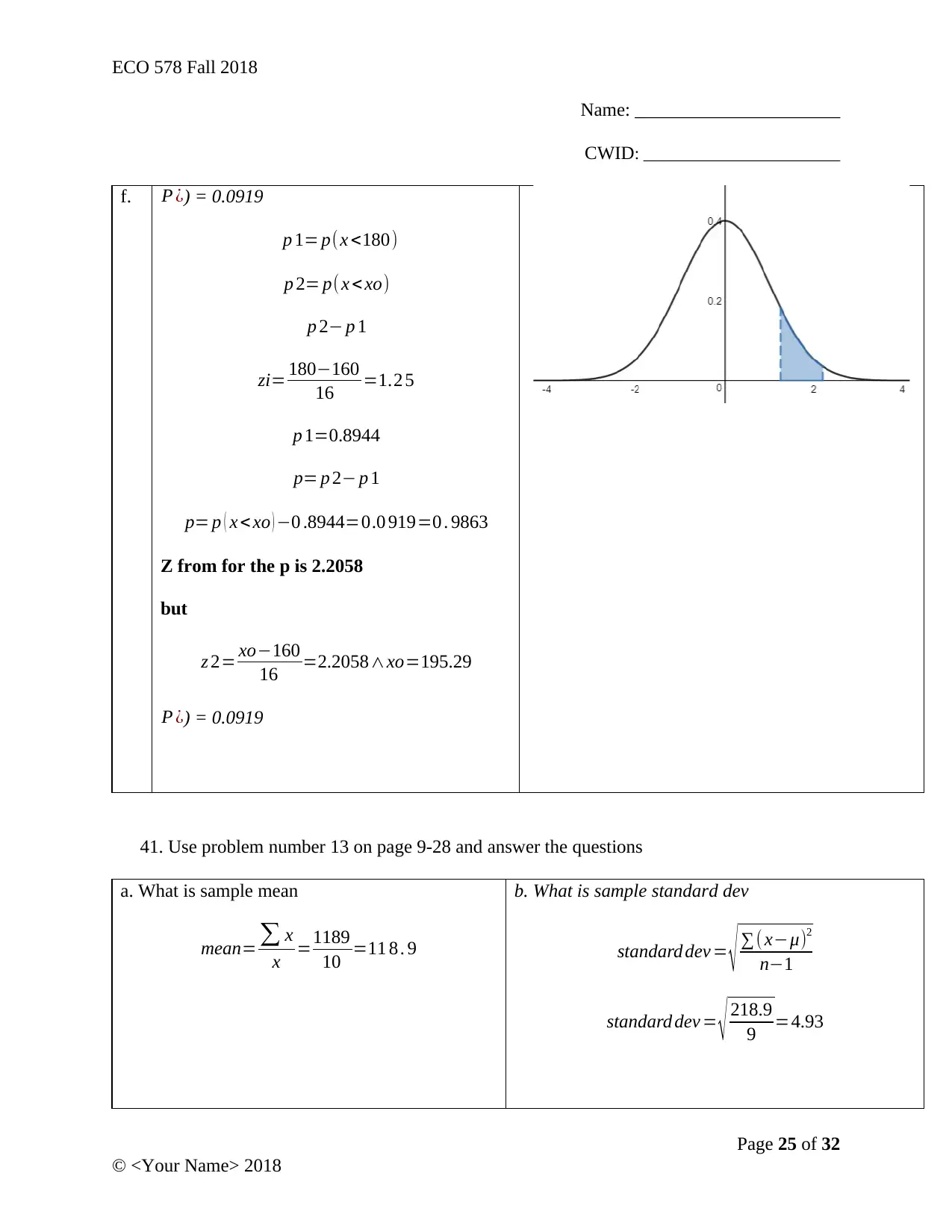
f. P ¿) = 0.0919
p 1= p(x <180)
p 2= p(x < xo)
p 2− p 1
zi= 180−160
16 =1.2 5
p 1=0.8944
p= p 2− p 1
p= p ( x < xo ) −0 .8944=0.0 919=0 . 9863
Z from for the p is 2.2058
but
z 2= xo−160
16 =2.2058∧xo=195.29
P ¿) = 0.0919
41. Use problem number 13 on page 9-28 and answer the questions
a. What is sample mean
mean= ∑ x
x =1189
10 =11 8 . 9
b. What is sample standard dev
standard dev = √ ∑( x−μ)2
n−1
standard dev = √ 218.9
9 =4.93
Page 25 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
a. c. Use z test or t test and why
We use Z test because the standard
deviation of the sample is known for the
sample size
d. What is your hypothesis
null hypothesis Ho=μ=120
Alternative hypothesis Hi=μ ≠ 120
f. Compute the value of the test statistic
Z= x −μ
σ
Z=120−118.9
4.83
z=0.228
42. Work on problem no 12 on page 8-11
Sol
X 4 8 12 11 14 6 12 8 9 5 ∑ x =¿ 89
X2 16 64 144 121 196 36 144 64 81 25 ∑ x2=891
x-
mean
-4.9 -0.9 3.1 2.1 5.1 -2.9 3.1 -0.9 0.1 -3.9
(x-
mean)
24.01 0.81 9.61 4.41 26.01 8.41 9.61 0.81 0.01 15.2
1
98.9
Page 26 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
sqrd
mean= ∑ x
x = 89
10 =8.9
standard dev = √ ∑ (x−μ)2
n
standard dev = √ 98.9
10 =3.144
Z-value for 90% confidence is 1.645
CI =μ ± zx σ
√n
CI =8.9 ±1.645 x 3.144
√10
CI =8.9 ±0.994
7.906 ≤CI <9.894
43. Work on problem no 9 on page 8-10 and interpret your result
Sol
n=27, X = 128.4, S = 20.6 what is the 98% confidence interval for μ
n = 27
The standard deviation of the sample =20.6
The mean of the sample =128.4
Confidence interval is given by,
CI =X ± tn−1 X s
√ n
tn−1 is the critical value of t ¿ t distribution
The degrees of freedom = n-1 = 27-1=26
Page 27 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
From the t distribution table, the critical for 98% confidence interval and 26 df is 2.479.
Substituting into the equation
CI =128.4 ± 2.479 X 20.6
√27
CI =128.4 ± 9.828
118.572≤ CI ≤ 138.228
44. Given a normally distributed population and a sampling distribution summarized by μ
=50,
n = 100, and σ 2 = 225. What is the probability that a simple random will reveal a sample
mean?
a. Above 63
b. between 53 and 63
c. between 40 and 63
d. below 30
e. between 30 and 45
a) P ¿)
Z=( M −μ)
√σ2
Z=(63−50)
√ 225
Z=1 3
1 5 =0 .87
P ( X >55 )=1- P ( Z<0.87 )=1-0.
Page 28 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
8078=0.1922
b) P(53 ≤ X ≥63)
Z 1= 53−50
15 =0.2
Z 2=1− 63−50
25 =0.13
From standard normal table
P1=0.5793
P2=0.5517
Hence The probability is
0.5793−0.5517=0.0276
c) P(40≤ X ≥ 63)
Z 1= 40−50
15 =−0. 67
Z 2=1− 63−50
25 =0.13
From standard normal table
P1=0.2514
P2=0.5517
Page 29 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
Hence The probability is
0.5793−0.5517=0.0276
d) P( X ≤ 30)
Z=( M −μ)
√σ2
Z=(30−50)
√ 225
Z=−20
15 =−1.33
P ( X ≤30 )= 0.4483
e) P(53 ≤ X ≥63)
Z 1= 53−50
15 =0.2
Z 2=1− 63−50
25 =0.13
From standard normal table
P1=0.5793
P2=0.5517
Hence The probability is
0.5793−0.5517=0.0276
Page 30 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
Page 31 of 32
© <Your Name> 2018

ECO 578 Fall 2018
Name: ______________________
CWID: _______________________
References
Bruce, P. (2015). Introductory statistics and analytics. New Jersey: Wiley.
Hinton, P. R. (2014). Statistics explained. 3rd ed. London: Routledge, Taylor & Francis Group.
Page 32 of 32
© <Your Name> 2018