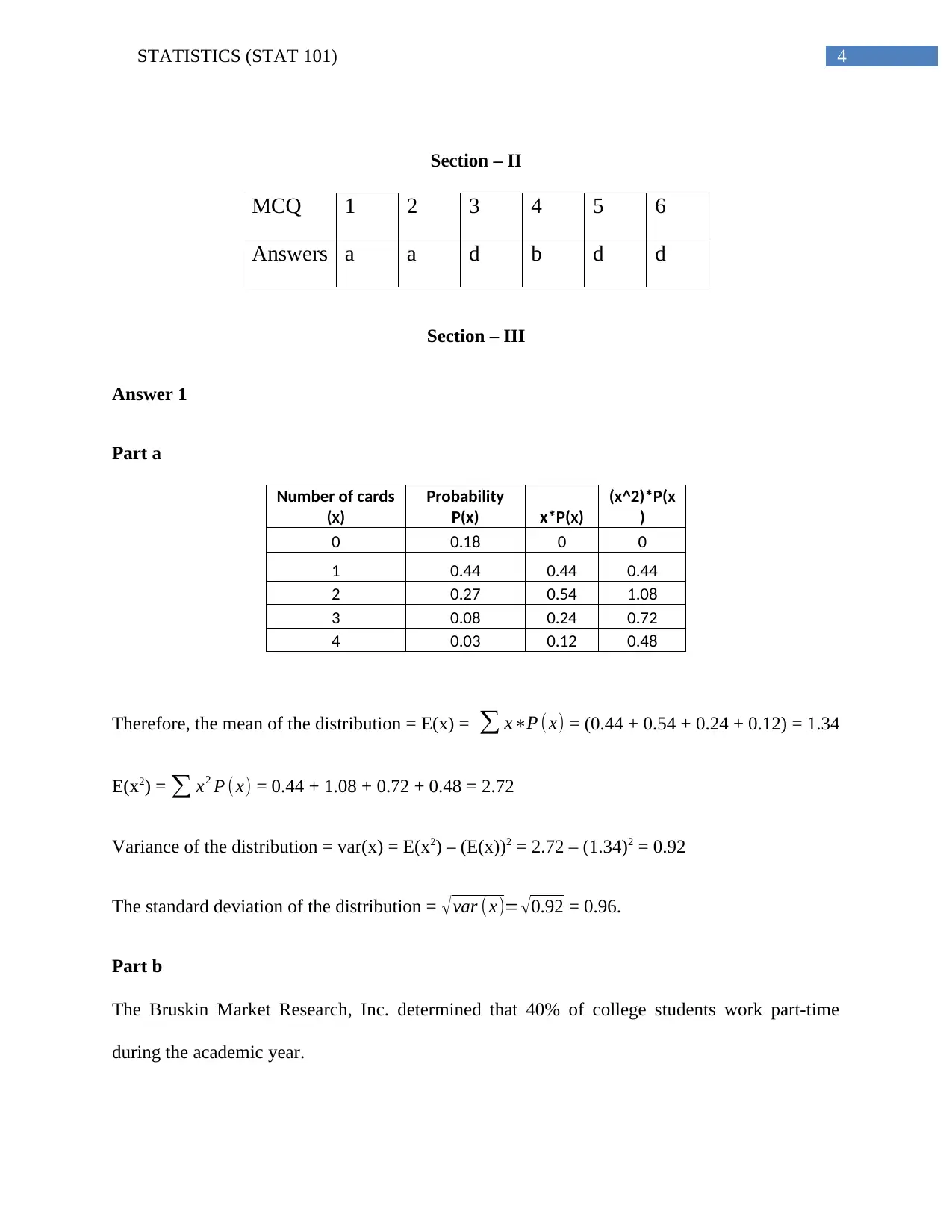
Statistics Assignment: Probability and Confidence Intervals

This statistics assignment focuses on fundamental concepts like probability distributions and confidence intervals. It includes problems related to calculating probabilities for various events, working with z-scores and standard normal distributions, constructing confidence intervals for population means, and interpreting results within a given context. The assignment requires applying statistical formulas and techniques to solve real-world scenarios.

Running Head: STATISTICS (STAT 101)
Statistics (STAT 101)
Name of the Student
Name of the University
Author Note

1STATISTICS (STAT 101)
Table of Contents
Section – I........................................................................................................................................3
Answer 1......................................................................................................................................3
Answer 2......................................................................................................................................3
Answer 3......................................................................................................................................3
Answer 4......................................................................................................................................3
Answer 5......................................................................................................................................3
Answer 6......................................................................................................................................3
Section – II.......................................................................................................................................4
Section – III.....................................................................................................................................4
Answer 1......................................................................................................................................4
Part a........................................................................................................................................4
Part b........................................................................................................................................4
Answer 2......................................................................................................................................6
Part a........................................................................................................................................6
Part b........................................................................................................................................6
Answer 3......................................................................................................................................7
Part a........................................................................................................................................7
Part b........................................................................................................................................8

2STATISTICS (STAT 101)

3STATISTICS (STAT 101)
Section – I
Answer 1
Let X be defined as an event defined as the occurrence of head.
Thus, X ~ Bin (6, 0.5), where the number of independent trials = 6 and the probability of success
is 0.5.
Thus, P (X = 3) = 6C3 * (0.5)3 * (1 – 0.5)3 = 20 * (1/2)6 = 10× ( 1
2 ¿ ¿5
≠ 10× ( 1
2 ¿ ¿4
.
False
Answer 2
True
Answer 3
True
Answer 4
True
Answer 5
True
Answer 6
False

4STATISTICS (STAT 101)
Section – II
MCQ 1 2 3 4 5 6
Answers a a d b d d
Section – III
Answer 1
Part a
Number of cards
(x)
Probability
P(x) x*P(x)
(x^2)*P(x
)
0 0.18 0 0
1 0.44 0.44 0.44
2 0.27 0.54 1.08
3 0.08 0.24 0.72
4 0.03 0.12 0.48
Therefore, the mean of the distribution = E(x) = ∑ x∗P (x) = (0.44 + 0.54 + 0.24 + 0.12) = 1.34
E(x2) = ∑ x2 P ( x) = 0.44 + 1.08 + 0.72 + 0.48 = 2.72
Variance of the distribution = var(x) = E(x2) – (E(x))2 = 2.72 – (1.34)2 = 0.92
The standard deviation of the distribution = √var (x)= √0.92 = 0.96.
Part b
The Bruskin Market Research, Inc. determined that 40% of college students work part-time
during the academic year.

5STATISTICS (STAT 101)
Therefore, the probability of the students working part time = 0.4
Let X be the number of students working part time.
Therefore, X ~ Bin (5, 0.4).
P (X = 0) = ( 5
0 ) (0.4)0 ¿ = 0.0778
P (X = 1) = (5
1 )(0.4 )1 ¿ = 0.2592
P (X = 2) = ( 5
2 ) (0.4 )2 ¿ = 0.3456
P (X = 3) = ( 5
3 ) (0.4 )3 ¿ = 0.2304
P (X = 4) = ( 5
4 ) (0.4)4 ¿ = 0.0768
P (X = 5) = (5
5 )(0.4 )5 ¿ = 0.01024
1. Therefore, the probability of at most 3 students working part time = P (X = 0) + P
(X = 1) + P (X = 2) + P (X = 3) = 0.0778 + 0.2592 + 0.3456 + 0.2304 = 0.91296.
2. Therefore, the probability of at least 2 students working part time = P (X = 2) + P
(X = 3) + P (X = 4) + P (X = 5) = 0.3456 + 0.2304 + 0.0768 + 0.01024 = 0.5853.
3. Therefore, the probability that less than 3 students work part time = P (X = 0) + P (X = 1)
+ P (X = 2) = 0.0778 + 0.2592 + 0.3456 = 0.6826.

6STATISTICS (STAT 101)
Answer 2
Part a
1. P(z < 2.0) = 0.9772
2. P(z > -1.88) = 1 – P(z < -1.88) = 1 – (1 – P(z < 1.88)) = P(z < 1.88) = 0.9699
3. P(-1.18 < z < 2.1) = P(z < 2.1) – P(z < -1.18) = P(z < 2.1) – (1 – P(z < 1.18)) = P(z < 2.1)
+ P(z < 1.18) – 1 = 0.9821 + 0.8810 – 1 = 0.8631.
Part b

7STATISTICS (STAT 101)
Answer 3
Part a

8STATISTICS (STAT 101)
Part b
Sample number (n) = 40
Average playing time (μ) = 51.3 minutes
Standard deviation of the playing time (σ) = 5.8 minutes
Therefore, the 95 percent confidence interval = (μ ± t * σ
√ n) = (51.3 ± 2.023 * 5.8
√40 ) = (49.44,
53.16).