Statistics Homework: Hypothesis Testing and Mean Earnings Comparison
VerifiedAdded on 2023/01/17
|5
|708
|36
Homework Assignment
AI Summary
This statistics homework assignment presents solutions to two problems involving hypothesis testing. The first problem focuses on comparing the variability of waiting times in two different banks using a Levene's test and then comparing the means using a pooled-variance t-test, concluding that there ...

Statistics
Statistics:
Student name:
Instructor:
1 | P a g e
Statistics:
Student name:
Instructor:
1 | P a g e
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
Statistics
PROBLEM ONE
Test for a difference in variability in waiting times in bank 1 and bank 2
The test employed independent samples t-test to establish whether the two variances were
different
Hypothesis
H0: Variability in waiting time in bank 1 and bank 2 are the same.
Versus
H1: There is a difference in variability in waiting time in bank 1 and bank 2.
The test was conducted at α = 0.05
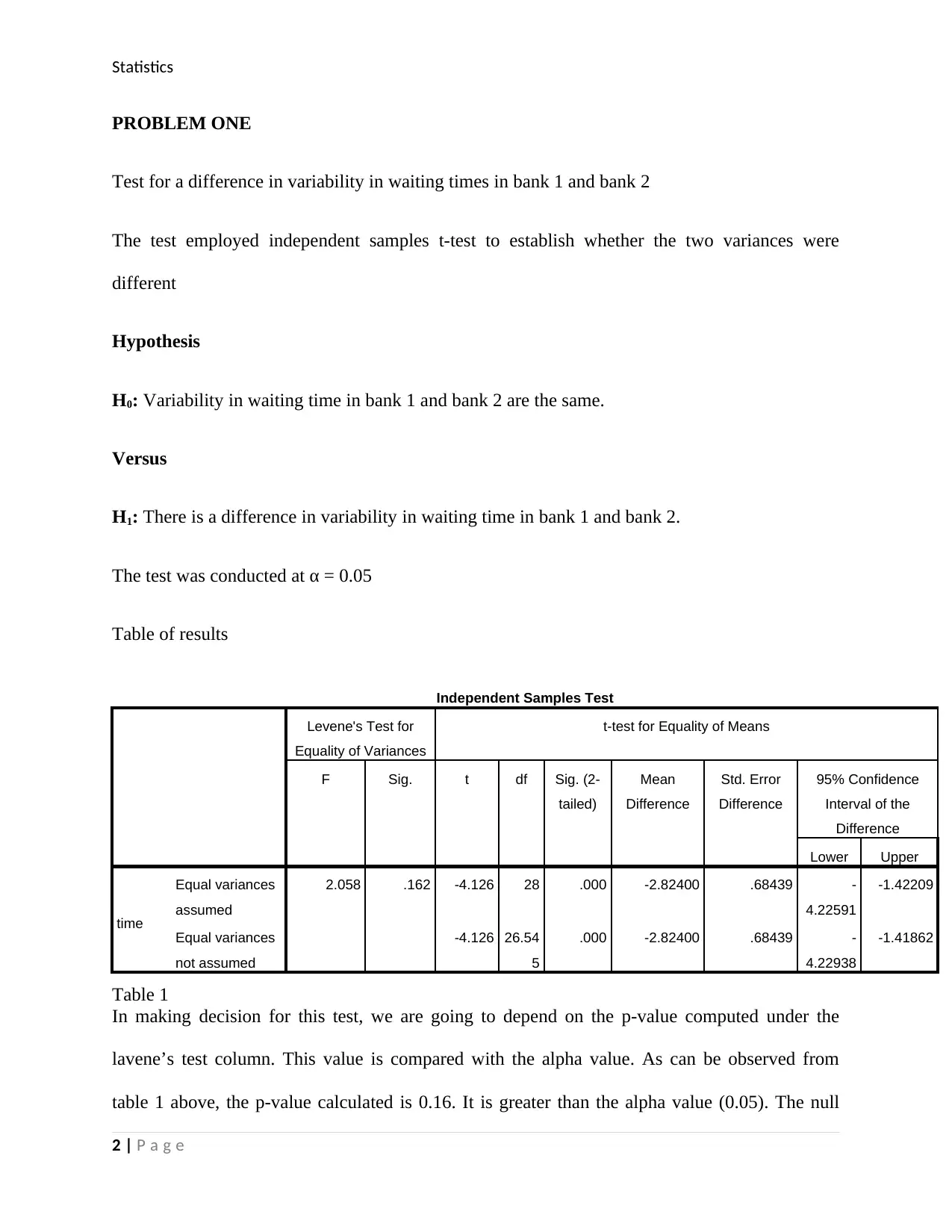
Table of results
Independent Samples Test
Levene's Test for
Equality of Variances
t-test for Equality of Means
F Sig. t df Sig. (2-
tailed)
Mean
Difference
Std. Error
Difference
95% Confidence
Interval of the
Difference
Lower Upper
time
Equal variances
assumed
2.058 .162 -4.126 28 .000 -2.82400 .68439 -
4.22591
-1.42209
Equal variances
not assumed
-4.126 26.54
5
.000 -2.82400 .68439 -
4.22938
-1.41862
Table 1
In making decision for this test, we are going to depend on the p-value computed under the
lavene’s test column. This value is compared with the alpha value. As can be observed from
table 1 above, the p-value calculated is 0.16. It is greater than the alpha value (0.05). The null
2 | P a g e
PROBLEM ONE
Test for a difference in variability in waiting times in bank 1 and bank 2
The test employed independent samples t-test to establish whether the two variances were
different
Hypothesis
H0: Variability in waiting time in bank 1 and bank 2 are the same.
Versus
H1: There is a difference in variability in waiting time in bank 1 and bank 2.
The test was conducted at α = 0.05
Table of results
Independent Samples Test
Levene's Test for
Equality of Variances
t-test for Equality of Means
F Sig. t df Sig. (2-
tailed)
Mean
Difference
Std. Error
Difference
95% Confidence
Interval of the
Difference
Lower Upper
time
Equal variances
assumed
2.058 .162 -4.126 28 .000 -2.82400 .68439 -
4.22591
-1.42209
Equal variances
not assumed
-4.126 26.54
5
.000 -2.82400 .68439 -
4.22938
-1.41862
Table 1
In making decision for this test, we are going to depend on the p-value computed under the
lavene’s test column. This value is compared with the alpha value. As can be observed from
table 1 above, the p-value calculated is 0.16. It is greater than the alpha value (0.05). The null
2 | P a g e

Statistics
hypothesis is therefore not rejected thus it is concluded that variability in waiting time in bank 1
and bank 2 are the same. There is equality of variance.
The assumption made in this test is that the data are normally distributed. However, this
assumption is not valid for these data since the two variables have data points less than 30.
Based on the results above (equality of variance), we are going to use pooled-variance t-test to
compare the means of the two variables.
Pooled-variance t-test to compare the means
Hypothesis
H0: Mean waiting time in bank 1 and bank 2 are the same.
Versus
H1: There is a difference in mean in waiting time in bank 1 and bank 2.
The test was conducted at α = 0.05
Table of results
t-Test: Two-Sample Assuming Equal Variances
BANK 1 BANK 2
Mean 4.290666667 7.114666667
Variance 2.690378095 4.335512381
Observations 15 15
Pooled Variance 3.512945238
Hypothesized Mean Difference 0
df 28
t Stat -4.126288924
P(T<=t) one-tail 0.000149618
t Critical one-tail 1.701130934
P(T<=t) two-tail 0.000299237
t Critical two-tail 2.048407142
3 | P a g e
hypothesis is therefore not rejected thus it is concluded that variability in waiting time in bank 1
and bank 2 are the same. There is equality of variance.
The assumption made in this test is that the data are normally distributed. However, this
assumption is not valid for these data since the two variables have data points less than 30.
Based on the results above (equality of variance), we are going to use pooled-variance t-test to
compare the means of the two variables.
Pooled-variance t-test to compare the means
Hypothesis
H0: Mean waiting time in bank 1 and bank 2 are the same.
Versus
H1: There is a difference in mean in waiting time in bank 1 and bank 2.
The test was conducted at α = 0.05
Table of results
t-Test: Two-Sample Assuming Equal Variances
BANK 1 BANK 2
Mean 4.290666667 7.114666667
Variance 2.690378095 4.335512381
Observations 15 15
Pooled Variance 3.512945238
Hypothesized Mean Difference 0
df 28
t Stat -4.126288924
P(T<=t) one-tail 0.000149618
t Critical one-tail 1.701130934
P(T<=t) two-tail 0.000299237
t Critical two-tail 2.048407142
3 | P a g e
You're viewing a preview
Unlock full access by subscribing today!

Statistics
Table 2
In making decision for this test, we are going to depend on the p-value computed under the two-
tailed test. This value is compared with the alpha value. As can be observed from table 2 above,
the p-value calculated is 0.00. It is less than the alpha value (0.05). The null hypothesis is
therefore rejected and the alternative hypothesis accepted thus it is concluded that there is a
difference in mean in waiting time in bank 1 and bank 2.
PROBLEM 2
Test for the differences in mean earnings between 1996 and 1997
Hypothesis
H0: Mean earning in 1996 and 1997 was the same.
Versus
H1: There is a difference in mean earning between 1996 and 1997.
The test was conducted at α = 0.05
4 | P a g e
Table 2
In making decision for this test, we are going to depend on the p-value computed under the two-
tailed test. This value is compared with the alpha value. As can be observed from table 2 above,
the p-value calculated is 0.00. It is less than the alpha value (0.05). The null hypothesis is
therefore rejected and the alternative hypothesis accepted thus it is concluded that there is a
difference in mean in waiting time in bank 1 and bank 2.
PROBLEM 2
Test for the differences in mean earnings between 1996 and 1997
Hypothesis
H0: Mean earning in 1996 and 1997 was the same.
Versus
H1: There is a difference in mean earning between 1996 and 1997.
The test was conducted at α = 0.05
4 | P a g e
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
Statistics
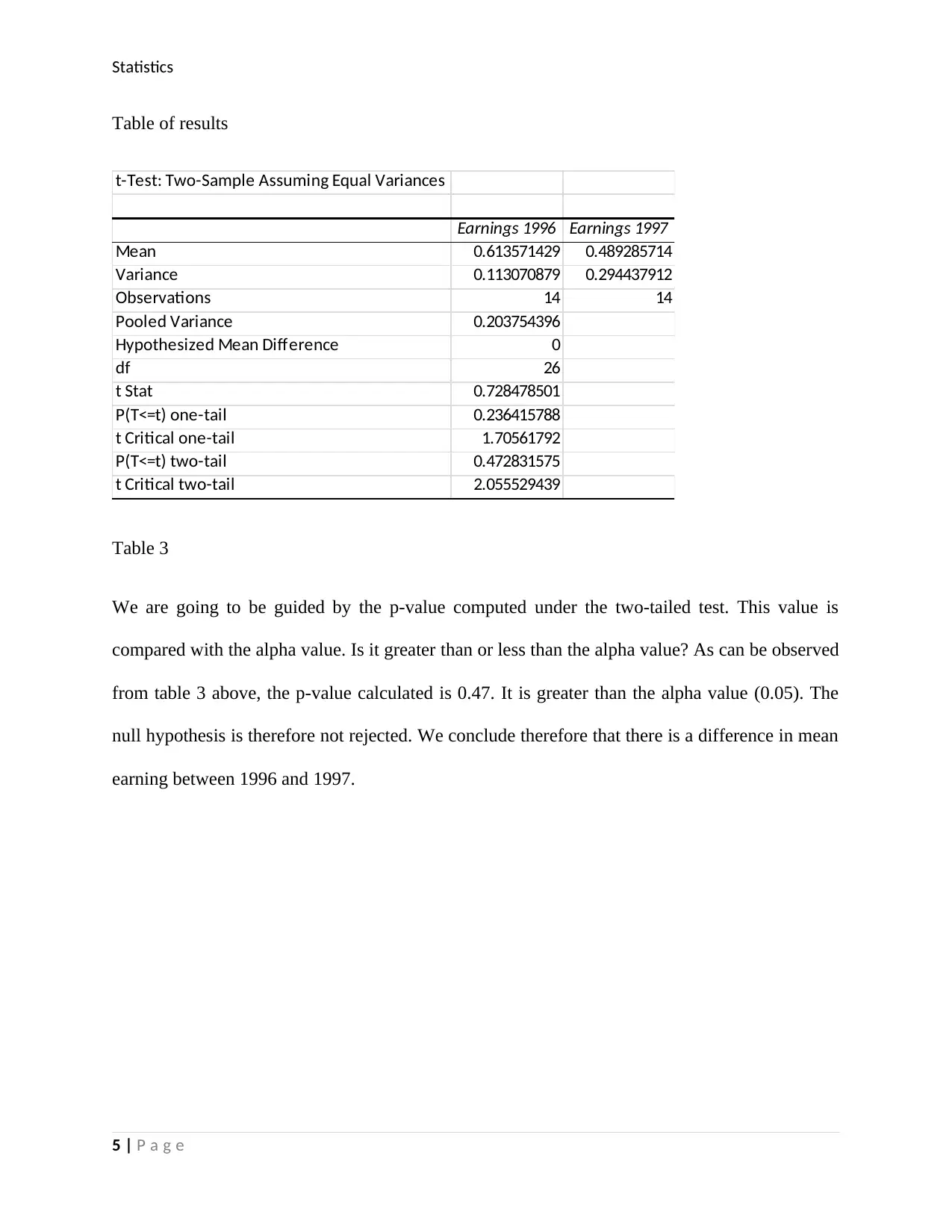
Table of results
t-Test: Two-Sample Assuming Equal Variances
Earnings 1996 Earnings 1997
Mean 0.613571429 0.489285714
Variance 0.113070879 0.294437912
Observations 14 14
Pooled Variance 0.203754396
Hypothesized Mean Difference 0
df 26
t Stat 0.728478501
P(T<=t) one-tail 0.236415788
t Critical one-tail 1.70561792
P(T<=t) two-tail 0.472831575
t Critical two-tail 2.055529439
Table 3
We are going to be guided by the p-value computed under the two-tailed test. This value is
compared with the alpha value. Is it greater than or less than the alpha value? As can be observed
from table 3 above, the p-value calculated is 0.47. It is greater than the alpha value (0.05). The
null hypothesis is therefore not rejected. We conclude therefore that there is a difference in mean
earning between 1996 and 1997.
5 | P a g e
Table of results
t-Test: Two-Sample Assuming Equal Variances
Earnings 1996 Earnings 1997
Mean 0.613571429 0.489285714
Variance 0.113070879 0.294437912
Observations 14 14
Pooled Variance 0.203754396
Hypothesized Mean Difference 0
df 26
t Stat 0.728478501
P(T<=t) one-tail 0.236415788
t Critical one-tail 1.70561792
P(T<=t) two-tail 0.472831575
t Critical two-tail 2.055529439
Table 3
We are going to be guided by the p-value computed under the two-tailed test. This value is
compared with the alpha value. Is it greater than or less than the alpha value? As can be observed
from table 3 above, the p-value calculated is 0.47. It is greater than the alpha value (0.05). The
null hypothesis is therefore not rejected. We conclude therefore that there is a difference in mean
earning between 1996 and 1997.
5 | P a g e
1 out of 5
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.





