Structural Analysis 1

This document contains solutions for Structural Analysis 1. It includes calculations for reactions at the support, node analysis, deflection at midspan and free ends, and shear force and bending moments for a trapezoidal load. It also includes solutions for a section from C to E.

Structural Analysis 1
STRUCURAL ANALYSIS
Name:
Class:
Professor:
School:
City:
Date:

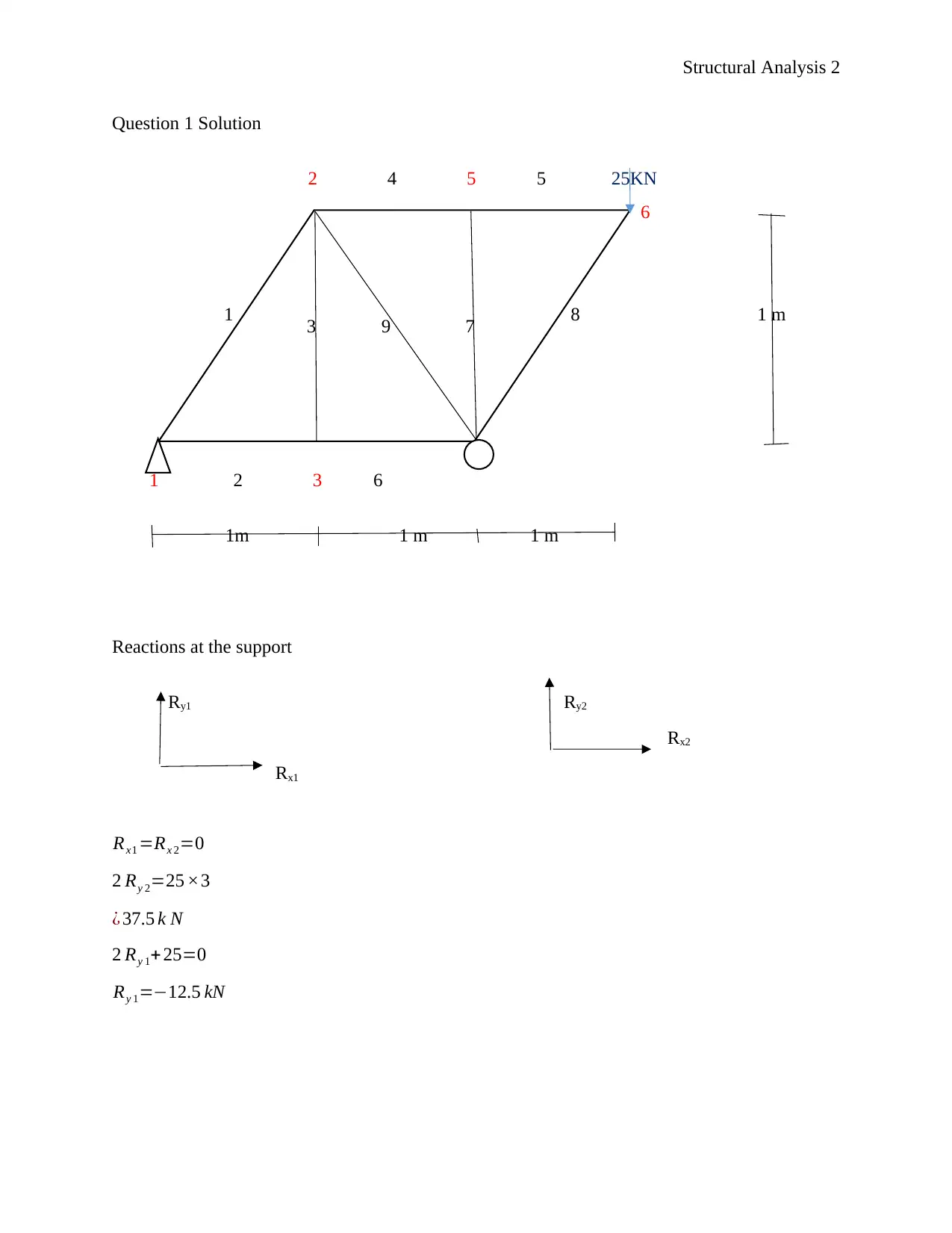
Structural Analysis 2
Question 1 Solution
2 4 5 5 25KN
6
1 8 1 m
1 2 3 6
1m 1 m 1 m
Reactions at the support
Ry1 Ry2
Rx2
Rx1
Rx1 =Rx 2=0
2 Ry 2=25 ×3
¿ 37.5 k N
2 Ry 1+ 25=0
Ry 1=−12.5 kN
3 9 7

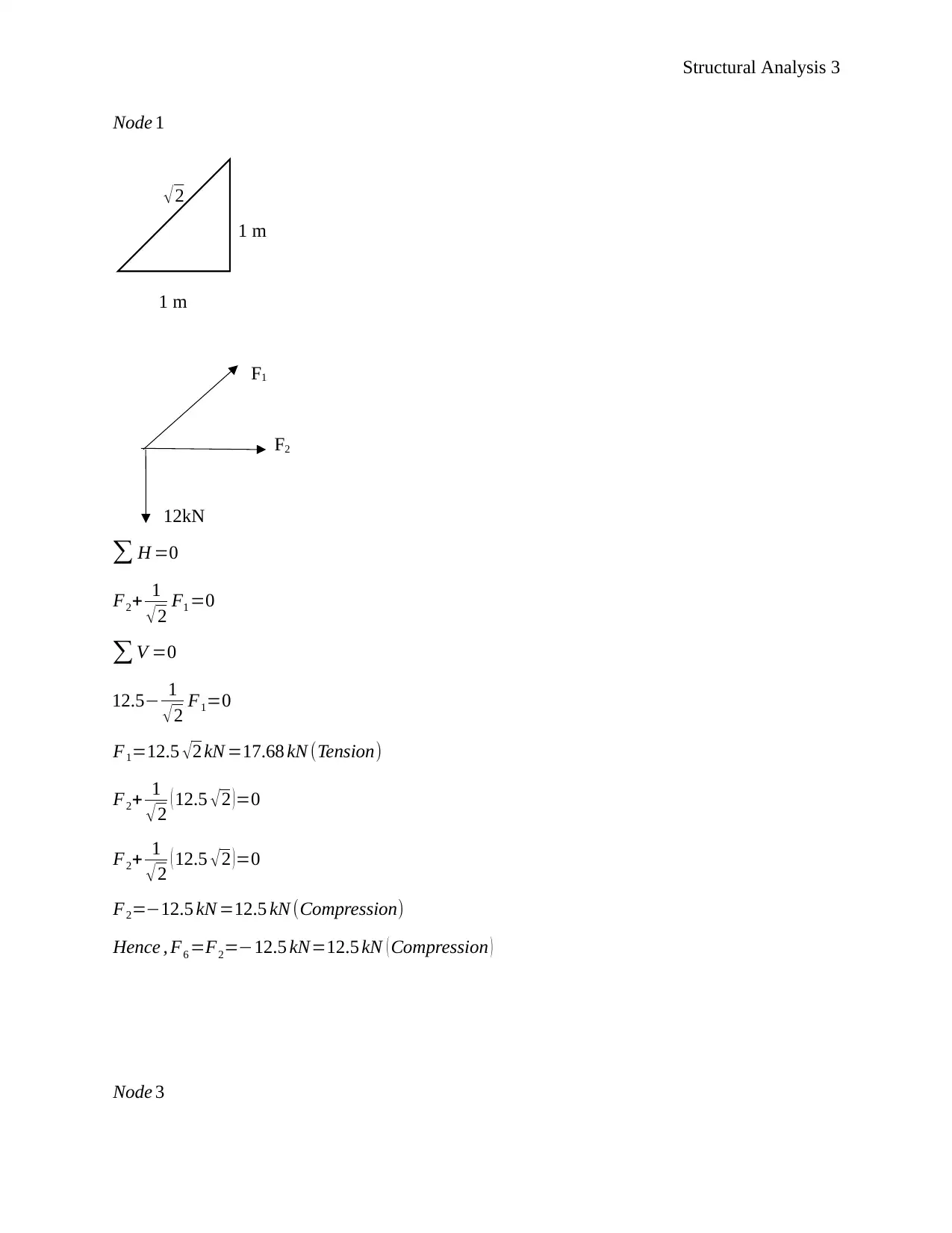
Structural Analysis 3
Node 1
√2
1 m
1 m
F1
F2
12kN
∑ H =0
F2+ 1
√ 2 F1 =0
∑ V =0
12.5− 1
√ 2 F1=0
F1=12.5 √2 kN =17.68 kN (Tension)
F2+ 1
√ 2 ( 12.5 √2 )=0
F2+ 1
√ 2 ( 12.5 √2 )=0
F2=−12.5 kN =12.5 kN (Compression)
Hence , F6 =F2=−12.5 kN=12.5 kN ( Compression )
Node 3

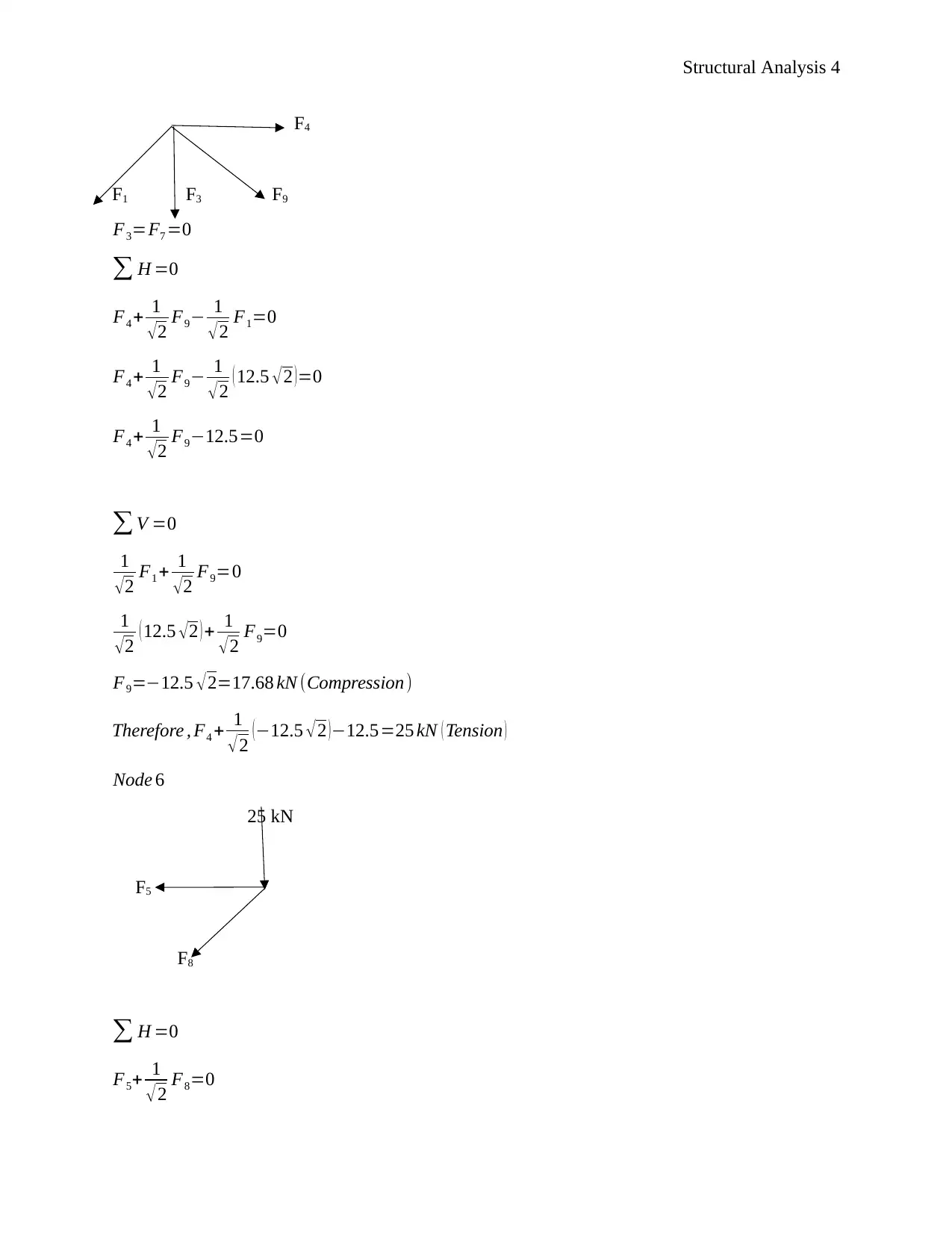
Structural Analysis 4
F4
F1 F3 F9
F3=F7 =0
∑ H =0
F4 + 1
√2 F9− 1
√2 F1=0
F4 + 1
√2 F9− 1
√2 ( 12.5 √2 )=0
F4 + 1
√2 F9−12.5=0
∑ V =0
1
√2 F1 + 1
√2 F9=0
1
√2 ( 12.5 √2 ) + 1
√2 F9=0
F9=−12.5 √ 2=17.68 kN (Compression)
Therefore , F4 + 1
√ 2 (−12.5 √2 )−12.5=25 kN ( Tension )
Node 6
25 kN
F5
F8
∑ H =0
F5+ 1
√ 2 F8=0

Structural Analysis 5
∑ V =0
25+ 1
√ 2 F8 =0
F8=−25 √ 2 kN=35.36 kN (Compression)
F5+ 1
√ 2 (−25 √2 )=0
F5=25 kN ( Tension )
F1=12.5 √ 2 kN =17.68 kN (Tension)
F2=12.5 kN (Compression)
F3=0
F4=25 kN (Tension )
F5=25 kN ( Tension )
F6=12.5 kN (Compression)
F7=0
F8=35.36 kN (Compression)
F9=17.68 kN (Compression)
Question 2 Solution
2kN UDL=5kN/m 2kN
2m 8m 2m
x
EI =43 ×1012 N ∙mm2=43 ×103 kN ∙ m2
E I y' ' =M
Moments about R1=0 , hence

Structural Analysis 6
8 R2= (2 ×10 )+ ( 5× 10× 5 )− (2 ×2 )− ( 2× 2× 1 )
R2=32 kN
R1= (12 ×5 )+ ( 4 )−32=32 kN
E I y' ' =2 x +5 x2
2 −32 ( x−2 ) +5 ( x−10 ) 2
2 −32 ( x−10 ) +5 ( x−10 ) 2
2
Integrate bothsides of the equation
E I ∫ ( y' ' ) =∫ ( 2 x +5 x2
2 −32 ( x−2 ) +5 ( x−2 )2
2 −32 ( x−10 ) +5 ( x −2 ) 2
2 )
E I y'=x2 + 5 x3
6 −16 ( x−2 ) 2 + 5 ( x−2 ) 3
6 −16 ( x−10 ) 2+ 5 ( x−10 ) 3
6 +C1
Integrate bothsides of the equation
E I ∫ ( y' )=∫ ( x2 + 5 x3
6 −16 ( x−2 ) 2+ 5 ( x−2 ) 3
6 −16 ( x−10 ) 2 + 5 ( x−10 ) 3
6 +C1 )
E I y = x3
3 + 5 x4
24 −16 ( x−2 ) 3
3 + 5 ( x −2 ) 4
24 − 16 ( x−10 ) 3
3 + 5 ( x−10 ) 4
24 +C1 x +C2
At x=2 , y =0 , hence
23
3 + 5(2)4
24 − 16 ( 2−2 ) 3
3 + 5 ( 2−2 ) 4
24 −16 ( 2−10 ) 3
3 + 5 ( 2−10 ) 4
24 +2 C1 +C2=0
8
3 + 10
3 + 8192
3 − 2560
3 +2C1 +C2 =0
5650
3 +2 C1+C2=0 (i)
At x=10 , y =0 , hence
103
3 + 5(10)4
24 − 16 ( 10−2 )3
3 + 5 ( 10−2 ) 4
24 − 16 ( 10−10 )3
3 + 5 ( 10−10 ) 4
24 +10 C1 +C2=0
1000
3 + 6250
3 − 8192
3 + 2560
3 +10 C1+C2=0
1618
3 +10 C1 +C2=0(ii)
Solving (i ) ∧ ( ii ) simultaneously ,

Structural Analysis 7
5650
3 +2 C1+C2=0
−¿
1618
3 +10 C1 +C2=0
4032
3 −8 C1=0
C1=504
3 =168
1618
3 + 504 0
3 +C2=0
C2=−6658
3
Therefore ,
EIy= x3
3 + 5 x4
24 −16 ( x−2 )3
3 + 5 ( x−2 )4
24 −16 ( x−10 )3
3 + 5 ( x −10 )4
24 + 504
3 x− 6658
3
Deflection at midspan , x=6
EIy= 63
3 + 5 ( 6 ) 4
24 − 16 ( 6−2 )3
3 + 5 ( 6−2 ) 4
24 − 16 ( 6−10 ) 3
3 + 5 ( 6−10 ) 4
24 + 504 ( 6 )
3 − 6658
3
EIy= 18
3 + 810
3 −1024
3 + 160
3 −1024
3 + 160
3 + 3024
3 − 6658
3
EIy=−4534
3
But EI=43 ×103 kN ∙ m2 , hence
y= −4534
3 ( 43 × 103 ) =−0.03515 m=35.15 mm
Deflectioat free ends , x=0∧x=12
EIy= 03
3 + 5 ( 0 ) 4
24 − 16 ( 0−2 ) 3
3 + 5 ( 0−2 ) 4
24 − 16 ( 0−10 ) 3
3 + 5 ( 0−10 ) 4
24 + 504 ( 0 )
3 −6658
3
EIy= 128
3 + 10
3 + 16000
3 + 6250
3 − 6658
3 =15730
3

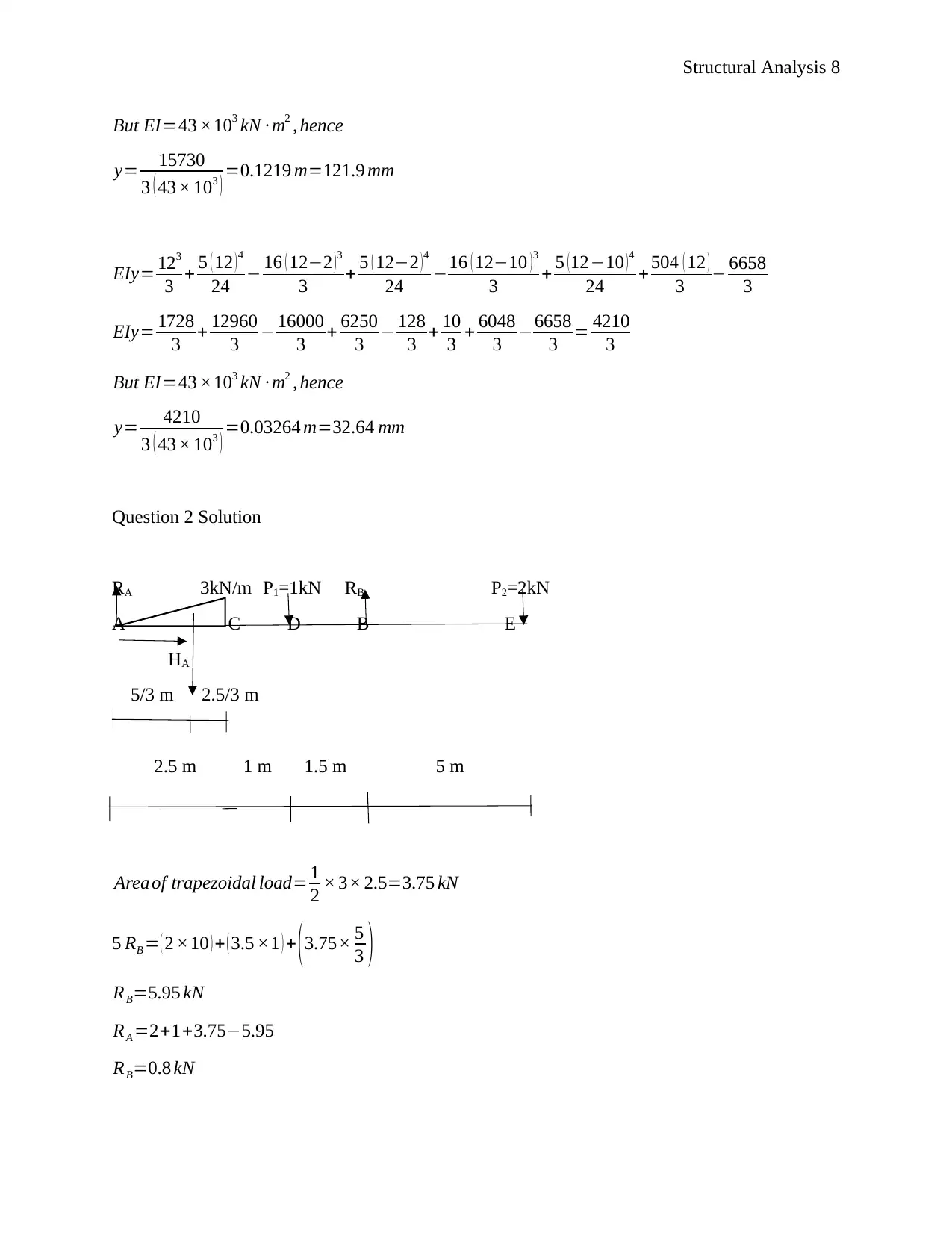
Structural Analysis 8
But EI=43 ×103 kN ∙ m2 , hence
y= 15730
3 ( 43 × 103 ) =0.1219 m=121.9 mm
EIy= 123
3 + 5 ( 12 ) 4
24 − 16 ( 12−2 ) 3
3 + 5 ( 12−2 ) 4
24 −16 ( 12−10 ) 3
3 + 5 ( 12−10 ) 4
24 + 504 ( 12 )
3 − 6658
3
EIy= 1728
3 + 12960
3 − 16000
3 + 6250
3 − 128
3 + 10
3 + 6048
3 −6658
3 = 4210
3
But EI=43 ×103 kN ∙ m2 , hence
y= 4210
3 ( 43 × 103 ) =0.03264 m=32.64 mm
Question 2 Solution
RA 3kN/m P1=1kN RB P2=2kN
A C D B E
HA
5/3 m 2.5/3 m
2.5 m 1 m 1.5 m 5 m
Area of trapezoidal load= 1
2 × 3× 2.5=3.75 kN
5 RB = ( 2 ×10 ) + ( 3.5 ×1 ) + (3.75× 5
3 )
RB=5.95 kN
RA =2+1+3.75−5.95
RB=0.8 kN

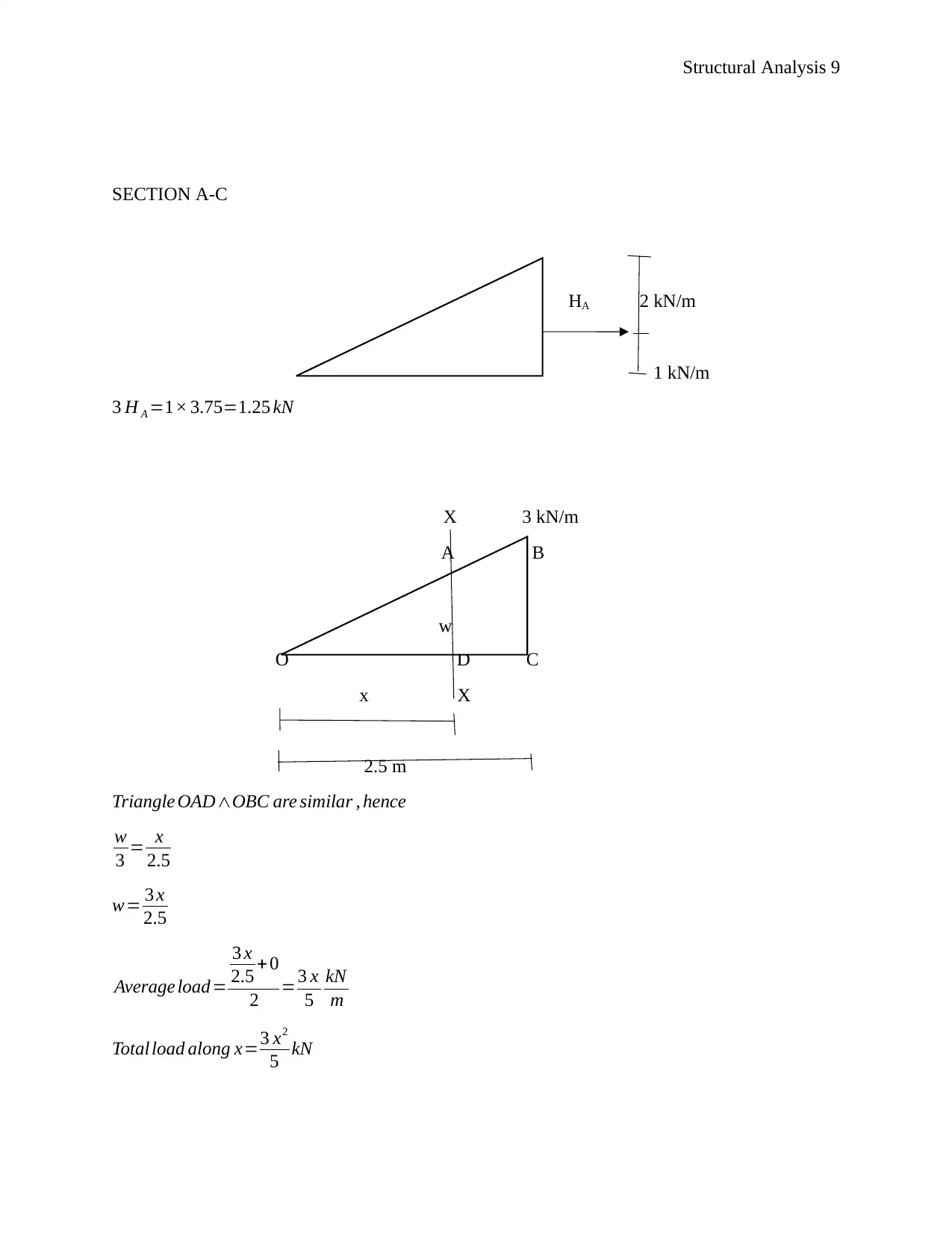
Structural Analysis 9
SECTION A-C
HA 2 kN/m
1 kN/m
3 H A =1× 3.75=1.25 kN
X 3 kN/m
A B
W
O D C
x X
2.5 m
Triangle OAD∧OBC are similar , hence
w
3 = x
2.5
w= 3 x
2.5
Average load=
3 x
2.5 + 0
2 =3 x
5
kN
m
Total load along x=3 x2
5 kN
w

Structural Analysis 10
Shear Force
Along the X−X line , SFxx=0.8−3 x2
5
At x=0
SF=0.8 kN
At x=2.5 m
SF=0.8− 3 ( 2.5 ) 2
5 =−2.95 kN
If SF =0
0.8−3 x2
5 =0 , x=1.15 m
Bending Moments
Along the X−X line , BM xx=0.8 x− 3 x2
5 ∙ x
3 =0.8 x− x3
5
At x=0
BM =0 kNm
At x=2.5 m
BM =0.8 ( 2.5 ) − ( 2.5 ) 3
5 =−1.125 kNm
Maximum BM occurs when SF=0. For our case , at x=1.15 m
BM =0.8 ( 1.15 )− ( 1.15 )3
5 =0.616 kNm
When BM =0 , then
0.8 x− x3
5 =0
0.8− x2
5 =0
x2
5 =0.8
x=2

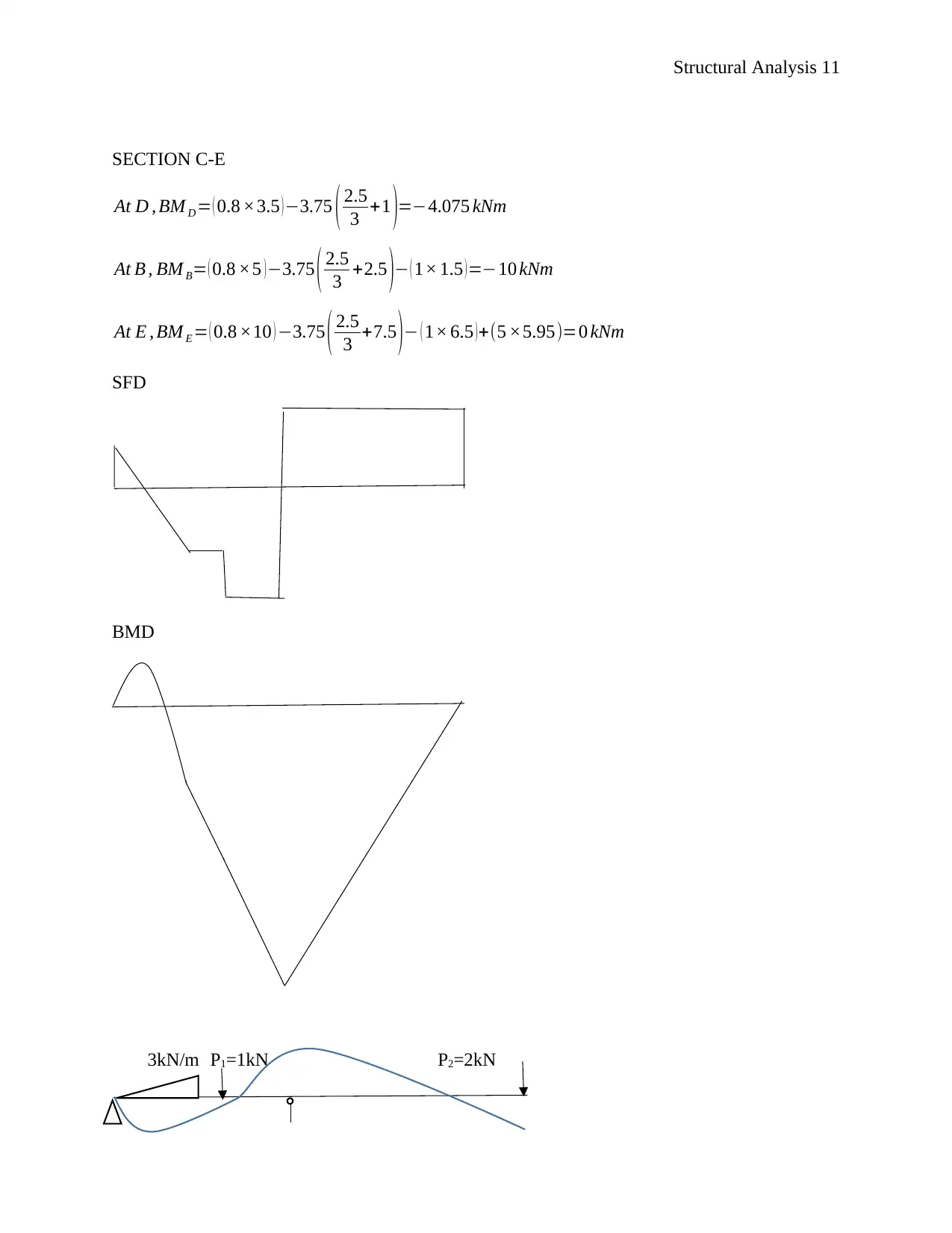
Structural Analysis 11
SECTION C-E
At D , BM D= ( 0.8 ×3.5 )−3.75 ( 2.5
3 +1 )=−4.075 kNm
At B , BM B= ( 0.8 ×5 )−3.75 ( 2.5
3 +2.5 )− ( 1× 1.5 )=−10 kNm
At E , BM E = ( 0.8 ×10 ) −3.75 ( 2.5
3 +7.5 )− ( 1× 6.5 )+(5 ×5.95)=0 kNm
SFD
BMD
3kN/m P1=1kN P2=2kN

Structural Analysis 12
References
Bansal, R. K., 2010. Srength of Materials. 4th ed. New Delhi: Laxmi Publications (P) Ltd..
Beer, F. P., Jonston Jr., E. R. & DeWolf, J. T., 2015. Mechanics of Materials. 7th ed. New York:
McGraw-Hill Education.
Gere, J. M. & Goodno, B. J., 2013. Mechanics of Materials. 8th ed. Stamford: Cengage
Learning.
Menon, D., 2008. Structural Analysis. 1st ed. Oxford: Alpha Science.