University Maths: Module 4 Linear Graphs Summary Assignment
VerifiedAdded on 2023/04/21
|5
|787
|83
Homework Assignment
AI Summary
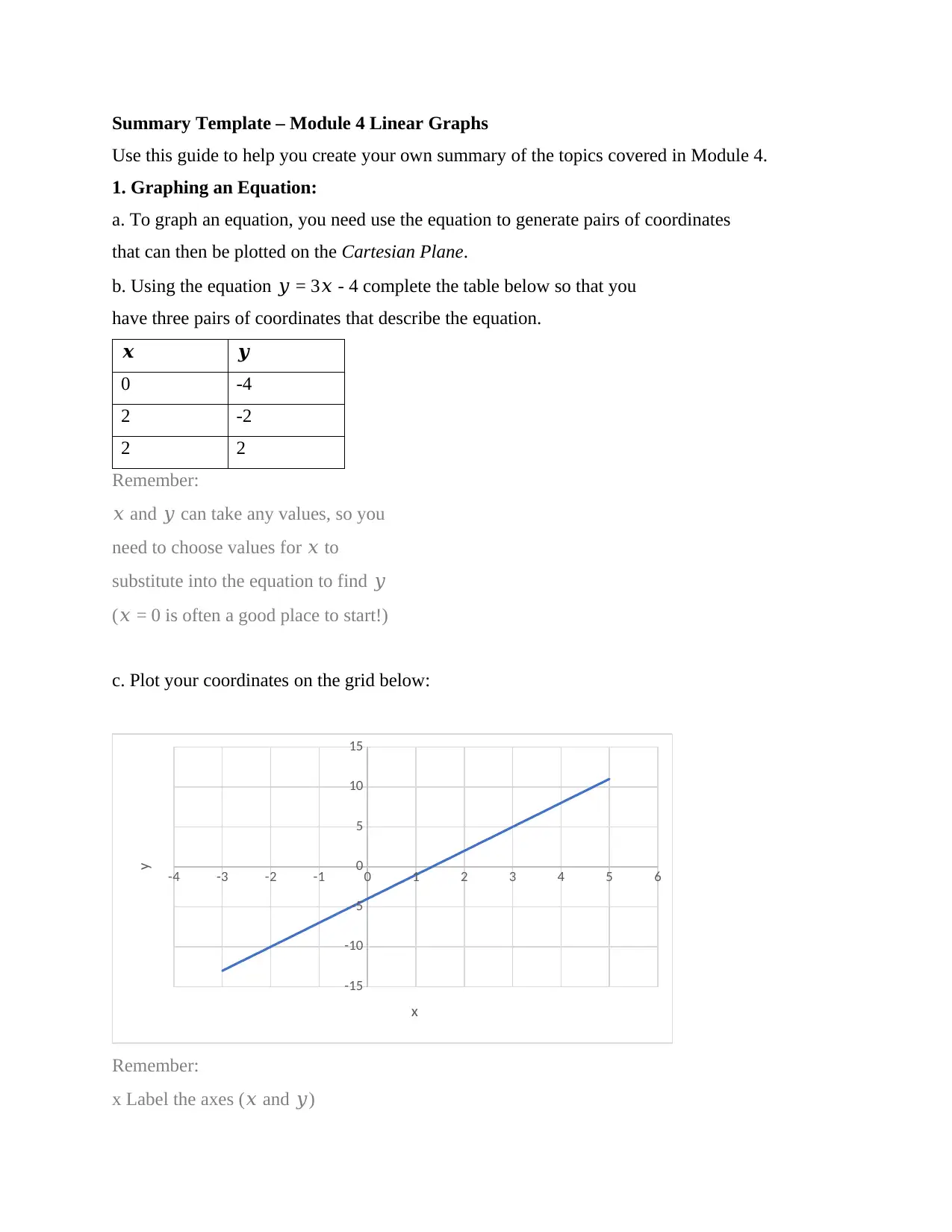
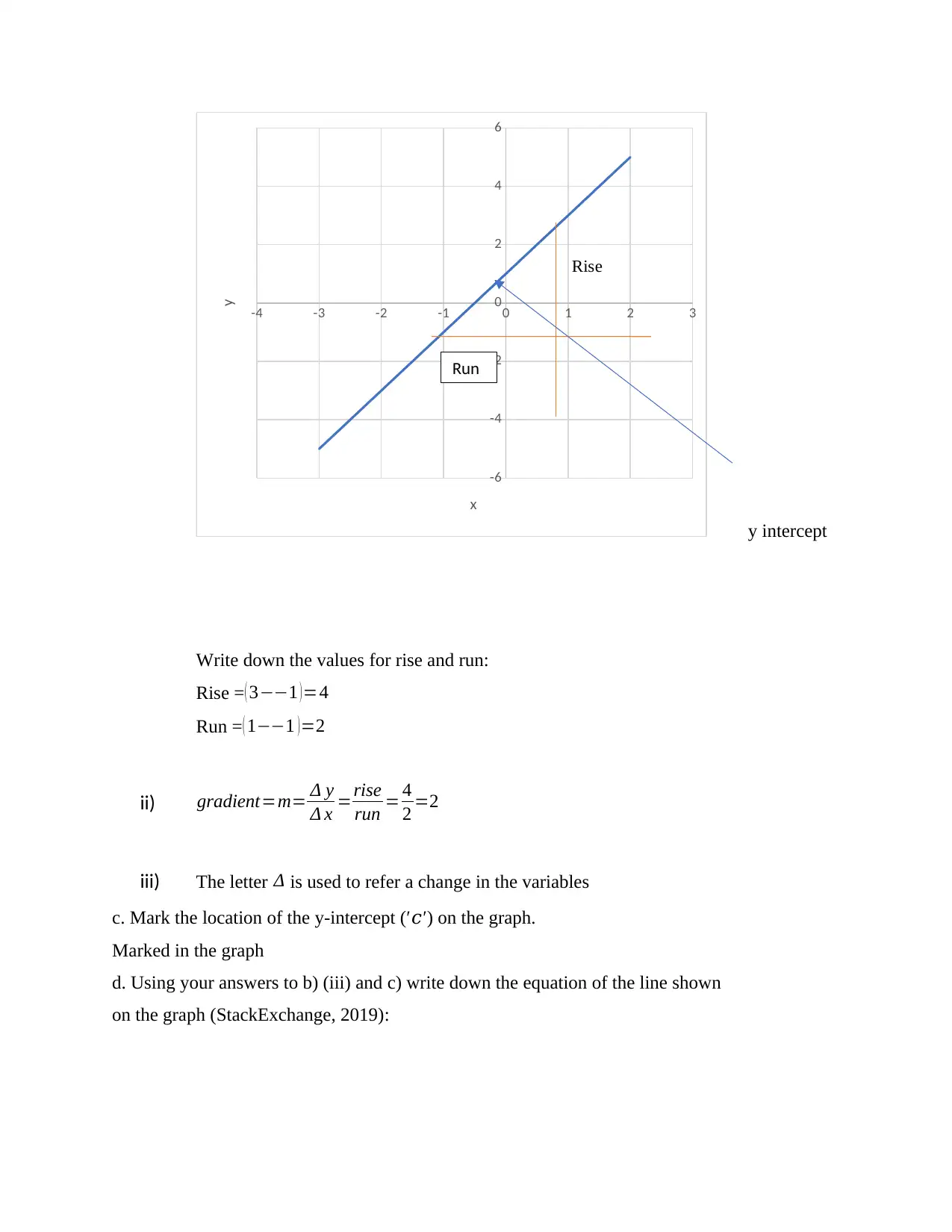
This assignment is a comprehensive summary of Module 4's linear graphs, covering key concepts such as graphing equations by generating coordinate pairs and plotting them on the Cartesian Plane. It explains the process of finding an equation from a graph using the slope-intercept form (y = mx + c) and the rise-over-run method to determine the gradient and y-intercept. The summary also delves into finding equations using coordinates, including the gradient formula and the two-point formula. Furthermore, it explores the properties of parallel and perpendicular lines, detailing their gradient relationships. The document provides step-by-step examples and clear explanations to reinforce understanding of these fundamental concepts in linear algebra, with references to external resources for further study.
1 out of 5