MATLAB EXERCISES. LINEAR ALGEBRA

LINEAR ALGEBRA clear; close all; clc; QUESTION 6 disp('---------------------------------------------------') disp('Question 6') %set #1 sa=[1,2,-1,0]; sb=[-3,-6,3,0]; sc=[-2,-2,1,-1]; disp('Question6: Set (a)') s1=[sa;sb;sc] %The first set % finding the subset that forms

TABLE OF CONTENTS
QUESTION 6.........................................................................................................................................1
QUESTION 7.........................................................................................................................................2
QUESTION 8.........................................................................................................................................3
QUESTION 9.........................................................................................................................................7
QUESTION 10.......................................................................................................................................7
PRELIMINARY SECTION
... MATLAB EXERCISES
... LINEAR ALGEBRA
clear;
close all;
clc;
QUESTION 6
disp('-------------------------------------------------------------------')
disp('Question 6')
%set #1
sa=[1,2,-1,0];
sb=[-3,-6,3,0];
sc=[-2,-2,1,-1];
disp('Question6: Set (a)')
s1=[sa;sb;sc] %The first set
% finding the subset that forms a basis for the span of the vectors
R1=rref(s1) %determine the linearly independent vectors
disp('The vector that forms the basis for the span of the vectors: ')
v_span=R1(1,:)
%set #
sd=[0,0,1,1,0];
se=[1,1,0,0,1];
sf=[1,1,1,1,1];
sg=[1,1,2,2,1];
sh=[0,0,3,3,1];
sk=[0,0,0,0,1];
disp('Question6: Set (b)')
s2=[sd;se;sf;sg;sh;sk]
% finding the subset that forms a basis for the span of the vectors
R2=rref(s2)
disp('The vector that forms the basis for the span of the vectors: ')
v_span2=R2(3,:)
-------------------------------------------------------------------
Question 6
Question6: Set (a)
s1 =
1

1 2 -1 0
-3 -6 3 0
-2 -2 1 -1
R1 =
1.0000 0 0 1.0000
0 1.0000 -0.5000 -0.5000
0 0 0 0
The vector that forms the basis for the span of the vectors:
v_span =
1 0 0 1
Question6: Set (b)
s2 =
0 0 1 1 0
1 1 0 0 1
1 1 1 1 1
1 1 2 2 1
0 0 3 3 1
0 0 0 0 1
R2 =
1 1 0 0 0
0 0 1 1 0
0 0 0 0 1
0 0 0 0 0
0 0 0 0 0
0 0 0 0 0
The vector that forms the basis for the span of the vectors:
v_span2 =
0 0 0 0 1
2

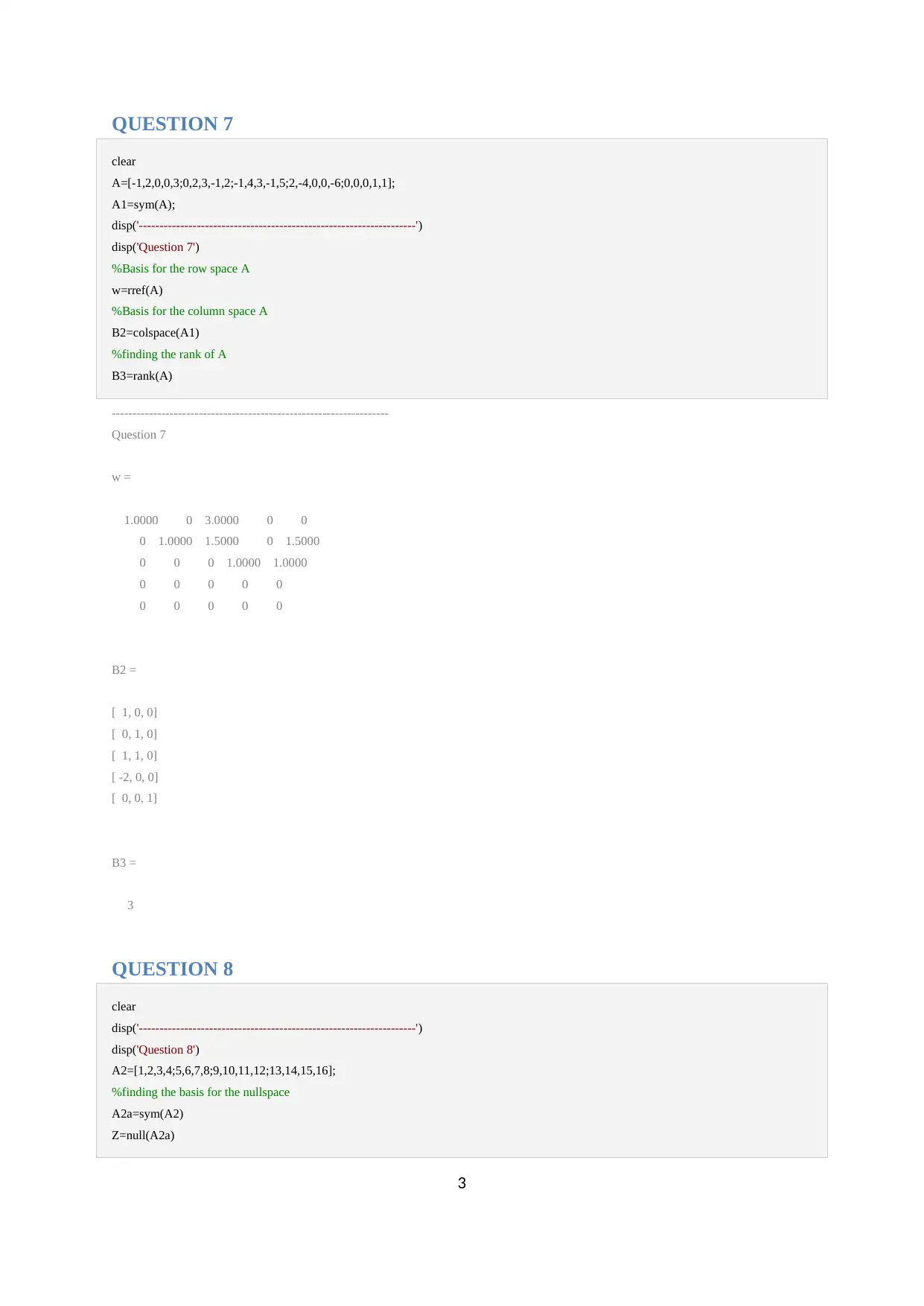
QUESTION 7
clear
A=[-1,2,0,0,3;0,2,3,-1,2;-1,4,3,-1,5;2,-4,0,0,-6;0,0,0,1,1];
A1=sym(A);
disp('-------------------------------------------------------------------')
disp('Question 7')
%Basis for the row space A
w=rref(A)
%Basis for the column space A
B2=colspace(A1)
%finding the rank of A
B3=rank(A)
-------------------------------------------------------------------
Question 7
w =
1.0000 0 3.0000 0 0
0 1.0000 1.5000 0 1.5000
0 0 0 1.0000 1.0000
0 0 0 0 0
0 0 0 0 0
B2 =
[ 1, 0, 0]
[ 0, 1, 0]
[ 1, 1, 0]
[ -2, 0, 0]
[ 0, 0, 1]
B3 =
3
QUESTION 8
clear
disp('-------------------------------------------------------------------')
disp('Question 8')
A2=[1,2,3,4;5,6,7,8;9,10,11,12;13,14,15,16];
%finding the basis for the nullspace
A2a=sym(A2)
Z=null(A2a)
3

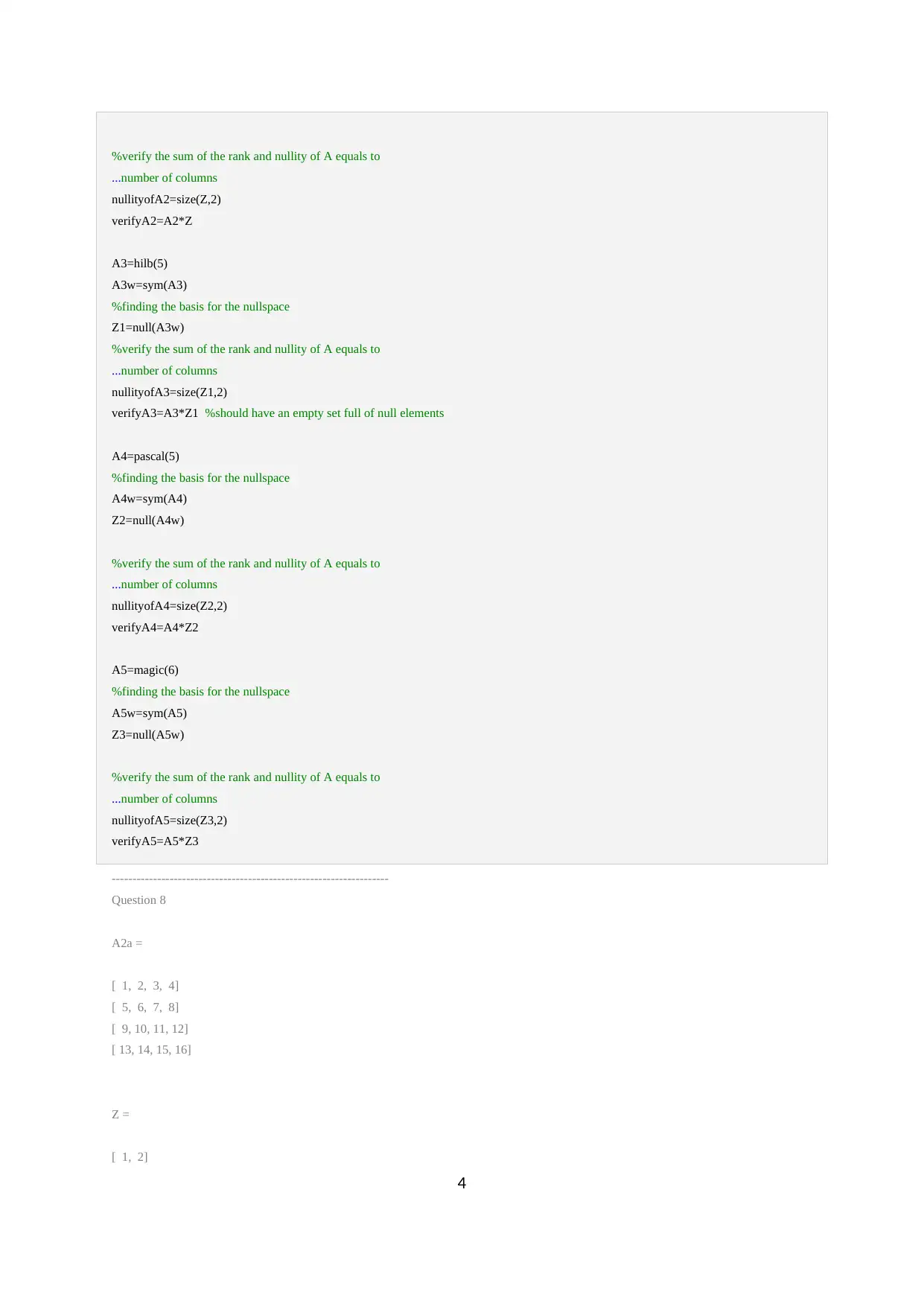
%verify the sum of the rank and nullity of A equals to
...number of columns
nullityofA2=size(Z,2)
verifyA2=A2*Z
A3=hilb(5)
A3w=sym(A3)
%finding the basis for the nullspace
Z1=null(A3w)
%verify the sum of the rank and nullity of A equals to
...number of columns
nullityofA3=size(Z1,2)
verifyA3=A3*Z1 %should have an empty set full of null elements
A4=pascal(5)
%finding the basis for the nullspace
A4w=sym(A4)
Z2=null(A4w)
%verify the sum of the rank and nullity of A equals to
...number of columns
nullityofA4=size(Z2,2)
verifyA4=A4*Z2
A5=magic(6)
%finding the basis for the nullspace
A5w=sym(A5)
Z3=null(A5w)
%verify the sum of the rank and nullity of A equals to
...number of columns
nullityofA5=size(Z3,2)
verifyA5=A5*Z3
-------------------------------------------------------------------
Question 8
A2a =
[ 1, 2, 3, 4]
[ 5, 6, 7, 8]
[ 9, 10, 11, 12]
[ 13, 14, 15, 16]
Z =
[ 1, 2]
4

[ -2, -3]
[ 1, 0]
[ 0, 1]
nullityofA2 =
2
verifyA2 =
[ 0, 0]
[ 0, 0]
[ 0, 0]
[ 0, 0]
A3 =
1.0000 0.5000 0.3333 0.2500 0.2000
0.5000 0.3333 0.2500 0.2000 0.1667
0.3333 0.2500 0.2000 0.1667 0.1429
0.2500 0.2000 0.1667 0.1429 0.1250
0.2000 0.1667 0.1429 0.1250 0.1111
A3w =
[ 1, 1/2, 1/3, 1/4, 1/5]
[ 1/2, 1/3, 1/4, 1/5, 1/6]
[ 1/3, 1/4, 1/5, 1/6, 1/7]
[ 1/4, 1/5, 1/6, 1/7, 1/8]
[ 1/5, 1/6, 1/7, 1/8, 1/9]
Z1 =
Empty sym: 1-by-0
nullityofA3 =
0
verifyA3 =
[ [], [], [], [], []]
5

[ [], [], [], [], []]
[ [], [], [], [], []]
[ [], [], [], [], []]
[ [], [], [], [], []]
A4 =
1 1 1 1 1
1 2 3 4 5
1 3 6 10 15
1 4 10 20 35
1 5 15 35 70
A4w =
[ 1, 1, 1, 1, 1]
[ 1, 2, 3, 4, 5]
[ 1, 3, 6, 10, 15]
[ 1, 4, 10, 20, 35]
[ 1, 5, 15, 35, 70]
Z2 =
Empty sym: 1-by-0
nullityofA4 =
0
verifyA4 =
[ [], [], [], [], []]
[ [], [], [], [], []]
[ [], [], [], [], []]
[ [], [], [], [], []]
[ [], [], [], [], []]
A5 =
35 1 6 26 19 24
3 32 7 21 23 25
31 9 2 22 27 20
8 28 33 17 10 15
6

30 5 34 12 14 16
4 36 29 13 18 11
A5w =
[ 35, 1, 6, 26, 19, 24]
[ 3, 32, 7, 21, 23, 25]
[ 31, 9, 2, 22, 27, 20]
[ 8, 28, 33, 17, 10, 15]
[ 30, 5, 34, 12, 14, 16]
[ 4, 36, 29, 13, 18, 11]
Z3 =
2
2
-1
-2
-2
1
nullityofA5 =
1
verifyA5 =
0
0
0
0
0
0
QUESTION 9
clear
disp('-------------------------------------------------------------------')
disp('Question 9')
q1=[1,0,1];
q2=[0,-1,2];
q3=[2,3,-5];
Q=[q1;q2;q3]; %non-standard basis for R^3
7

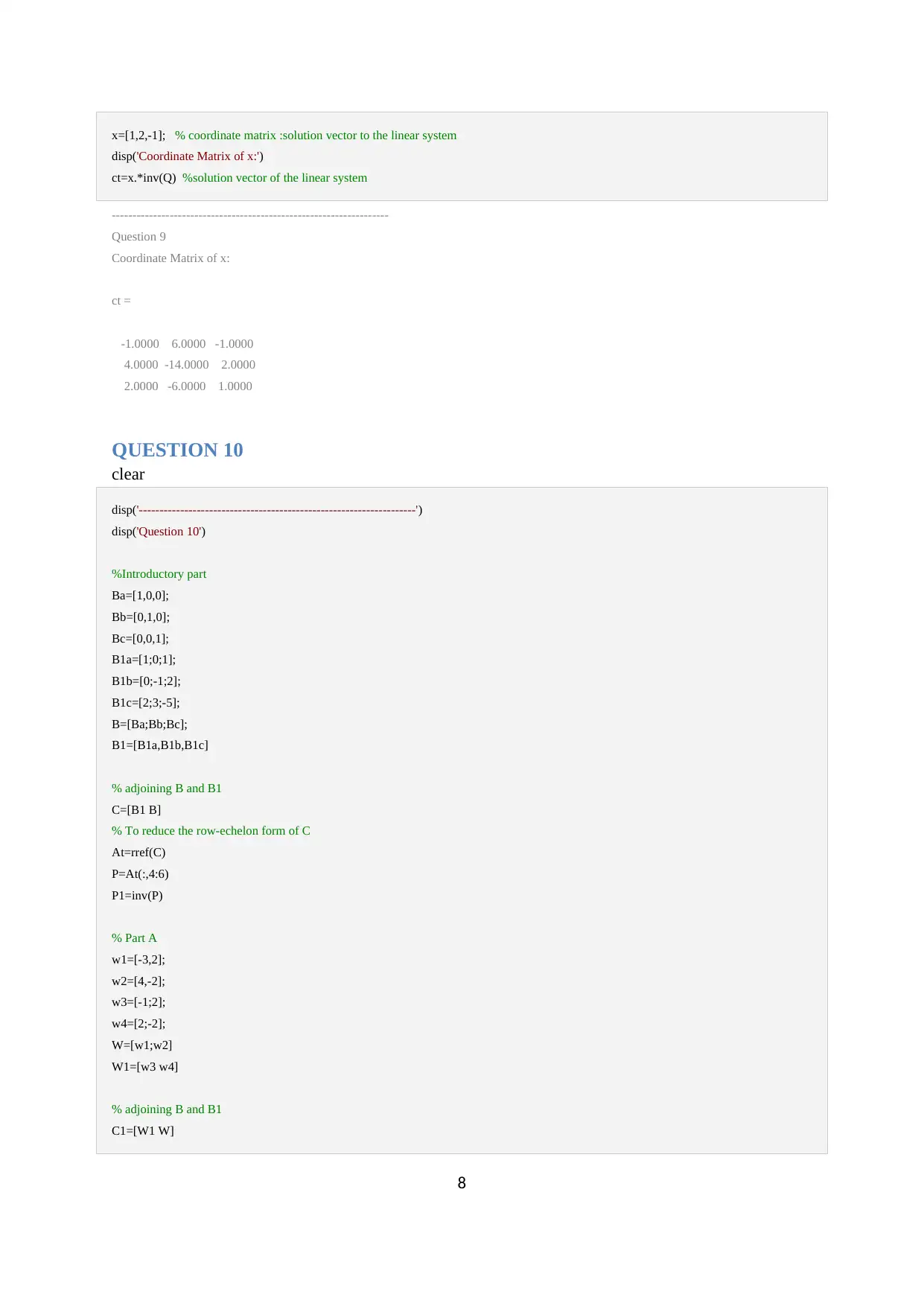
x=[1,2,-1]; % coordinate matrix :solution vector to the linear system
disp('Coordinate Matrix of x:')
ct=x.*inv(Q) %solution vector of the linear system
-------------------------------------------------------------------
Question 9
Coordinate Matrix of x:
ct =
-1.0000 6.0000 -1.0000
4.0000 -14.0000 2.0000
2.0000 -6.0000 1.0000
QUESTION 10
clear
disp('-------------------------------------------------------------------')
disp('Question 10')
%Introductory part
Ba=[1,0,0];
Bb=[0,1,0];
Bc=[0,0,1];
B1a=[1;0;1];
B1b=[0;-1;2];
B1c=[2;3;-5];
B=[Ba;Bb;Bc];
B1=[B1a,B1b,B1c]
% adjoining B and B1
C=[B1 B]
% To reduce the row-echelon form of C
At=rref(C)
P=At(:,4:6)
P1=inv(P)
% Part A
w1=[-3,2];
w2=[4,-2];
w3=[-1;2];
w4=[2;-2];
W=[w1;w2]
W1=[w3 w4]
% adjoining B and B1
C1=[W1 W]
8

% To reduce the row-echelon form of C
At1=rref(C1)
Pa=At1(:,3:4)
Pa1=inv(Pa) %inverting the Pa matrix using the inv function
% Part B
Bx=[[1,1,1,1];[0,1,1,1];[0,0,1,1];[0,0,0,1]]
Bx1=[[1;0;1;0],[1;0;-1;0],[0;1;0;1],[0;1;0;-1]]
% adjoining B and B1
C2=[Bx1 Bx]
% To reduce the row-echelon form of C
At2=rref(C2)
Pb=At2(:,5:8)
Pb1=inv(Pb)
-------------------------------------------------------------------
Question 10
B1 =
1 0 2
0 -1 3
1 2 -5
C =
1 0 2 1 0 0
0 -1 3 0 1 0
1 2 -5 0 0 1
At =
1 0 0 -1 4 2
0 1 0 3 -7 -3
0 0 1 1 -2 -1
P =
-1 4 2
3 -7 -3
1 -2 -1
P1 =
1.0000 0.0000 2.0000
9

0 -1.0000 3.0000
1.0000 2.0000 -5.0000
W =
-3 2
4 -2
W1 =
-1 2
2 -2
C1 =
-1 2 -3 2
2 -2 4 -2
At1 =
1 0 1 0
0 1 -1 1
Pa =
1 0
-1 1
Pa1 =
1 0
1 1
Bx =
1 1 1 1
0 1 1 1
0 0 1 1
0 0 0 1
Bx1 =
10

1 1 0 0
0 0 1 1
1 -1 0 0
0 0 1 -1
C2 =
1 1 0 0 1 1 1 1
0 0 1 1 0 1 1 1
1 -1 0 0 0 0 1 1
0 0 1 -1 0 0 0 1
At2 =
Columns 1 through 7
1.0000 0 0 0 0.5000 0.5000 1.0000
0 1.0000 0 0 0.5000 0.5000 0
0 0 1.0000 0 0 0.5000 0.5000
0 0 0 1.0000 0 0.5000 0.5000
Column 8
1.0000
0
1.0000
0
Pb =
0.5000 0.5000 1.0000 1.0000
0.5000 0.5000 0 0
0 0.5000 0.5000 1.0000
0 0.5000 0.5000 0
Pb1 =
1 1 -1 -1
-1 1 1 1
1 -1 -1 1
0 0 1 -1
11