Task 1. Part 1. Provided Data:. Radius of the wheel rim
VerifiedAdded on 2022/11/18
|7
|456
|90
AI Summary
Assignment to be done using microsoft Word. All diagrams/pictures to be clearly identified. Please ensure ALL criteria is attempted as the professor will not except the work unless all the parts are attempted. Please ensure this assignment passes first time - I have had many assignments fail from yourselves. Please do not ring me, message me instead.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.
1 out of 7


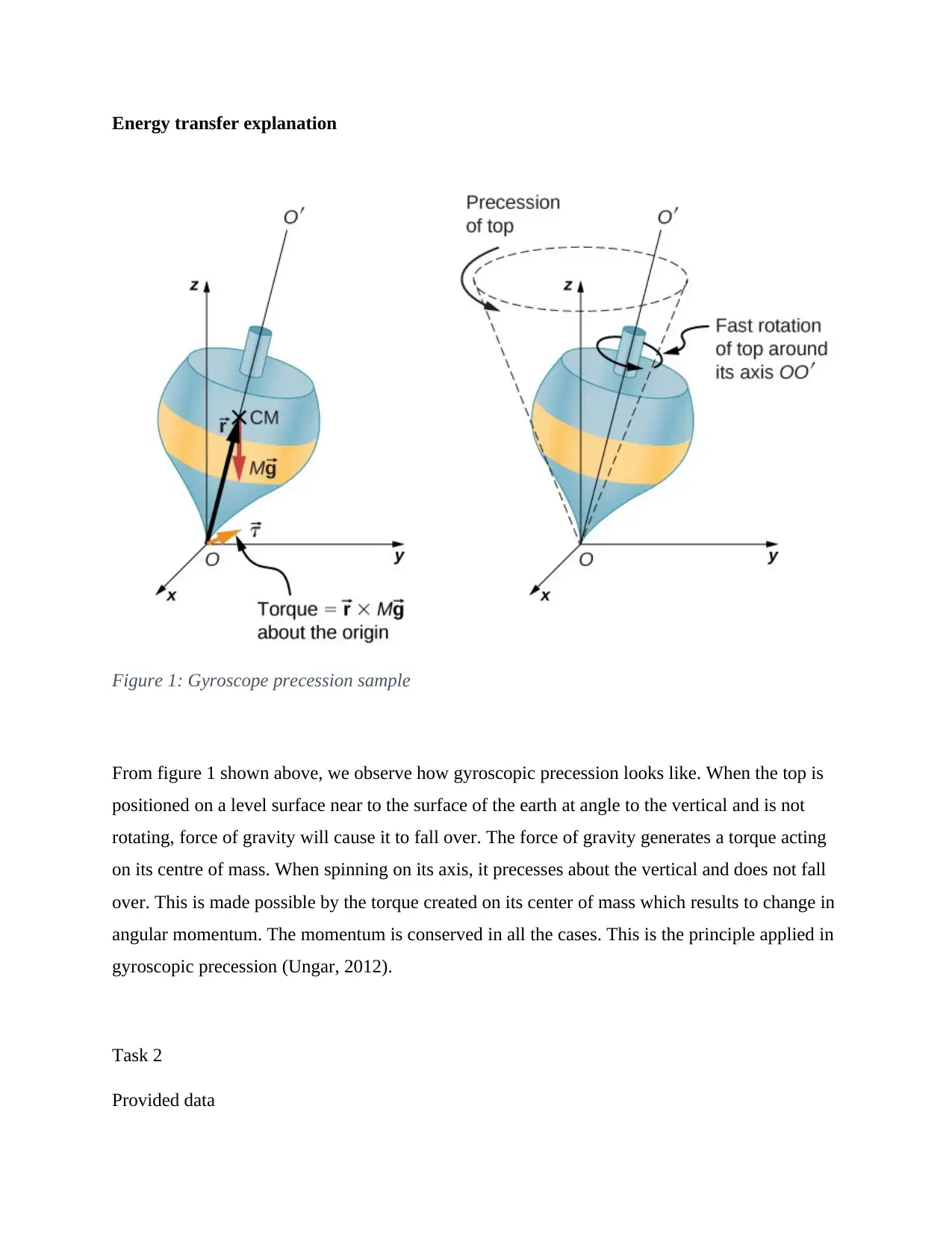
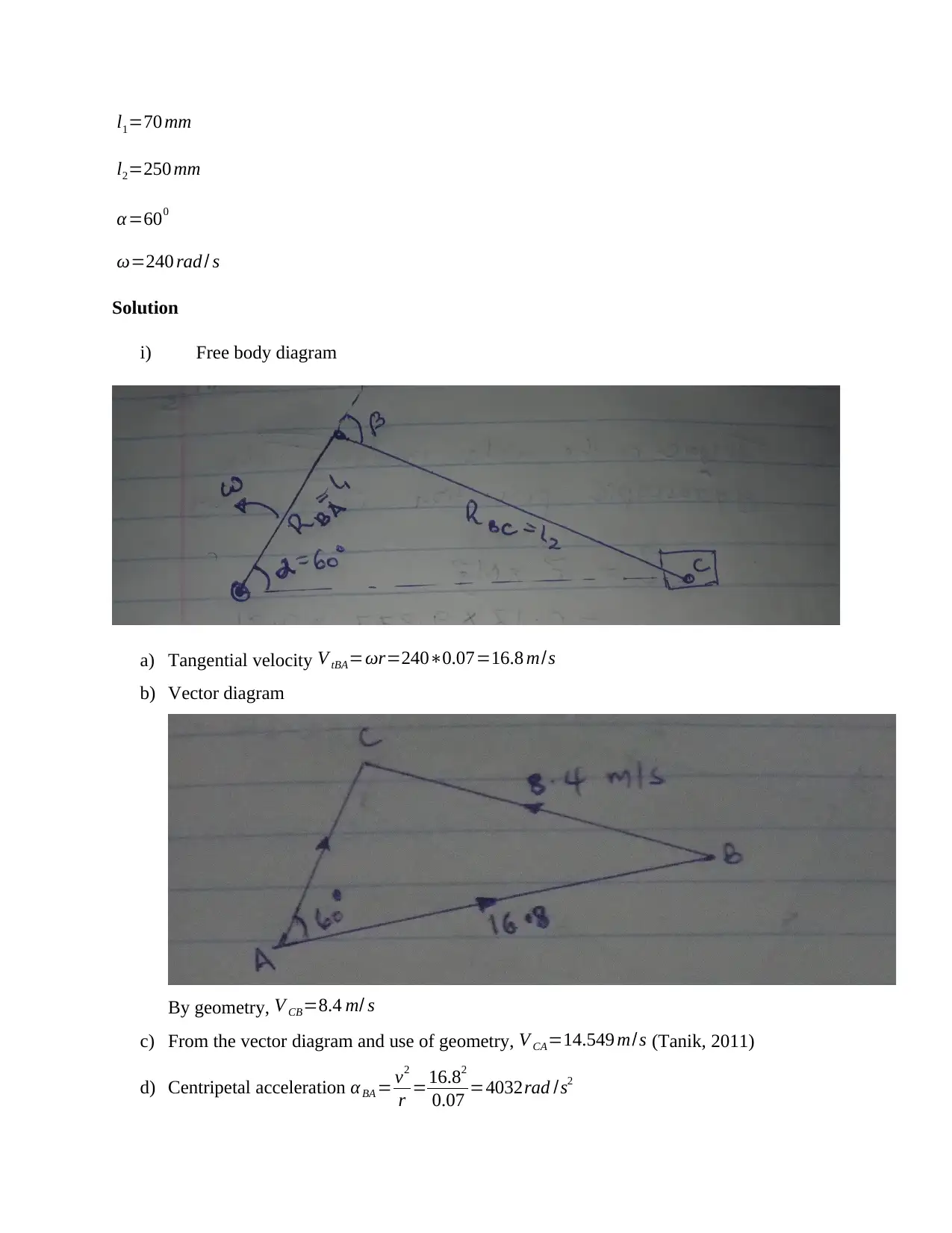
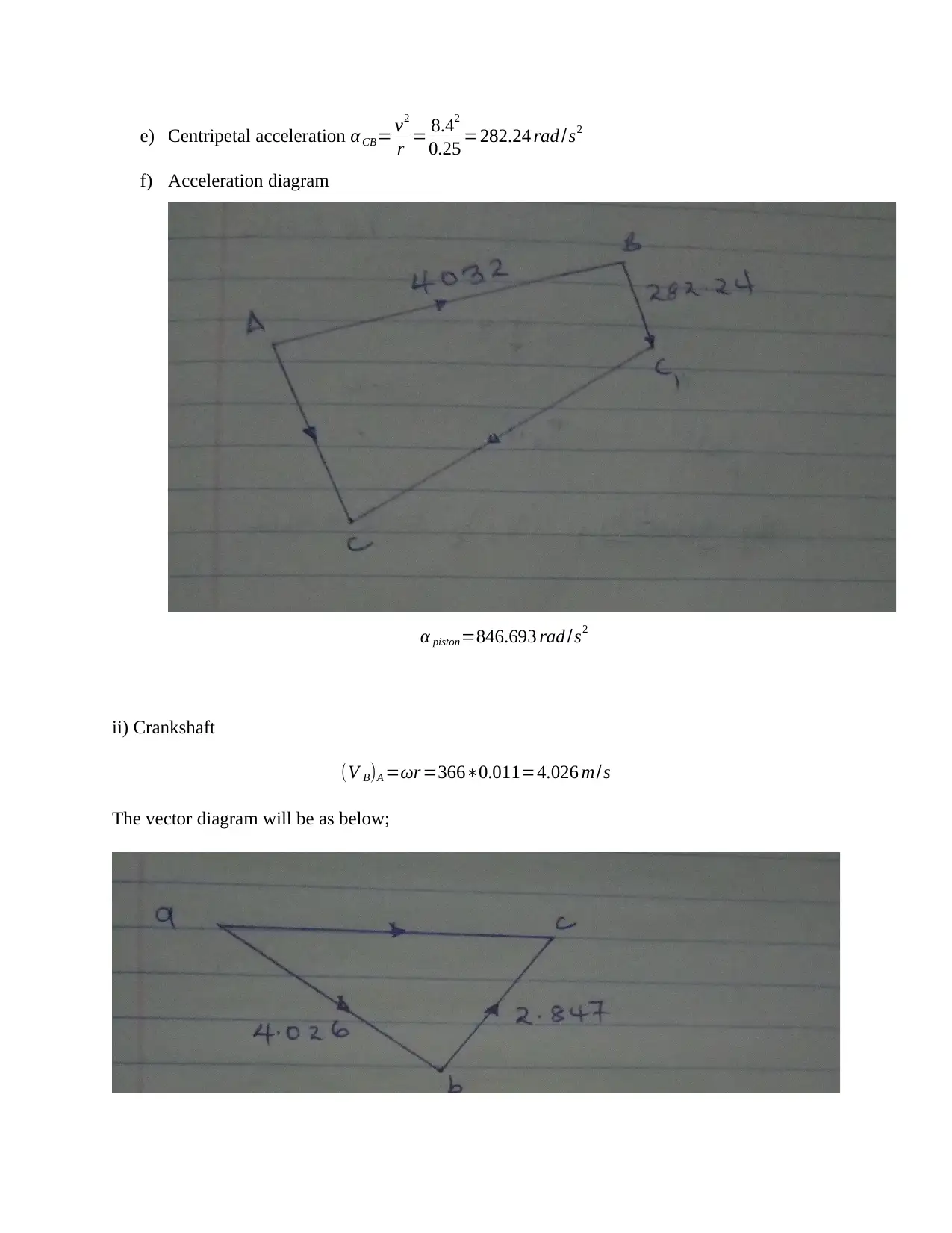



![[object Object]](/_next/static/media/star-bottom.7253800d.svg)