HNC Engineering Maths Assignment: Solving Engineering Problems
VerifiedAdded on 2022/10/31
|8
|1653
|2
Homework Assignment
AI Summary
This document provides a detailed solution to an HNC Engineering Maths assignment, focusing on the application of analytical and computational methods. The solution covers four tasks. Task 1 analyzes a sinusoidal wave, determining its amplitude, angular velocity, phase angle, and values at specific...
Task 1
V = 340 sin (50πt − 0.541)
Answer
Sine wave in form:
V = Vm sin (ωt − φ)
has an amplitude of Vm , angular velocity of ω and phase angle (lagging) of φ
radians.
a)
Comparing the given sine wave equation with the standard form, we have:
Amplitude = 340 V
Angular velocity : ω = 50π
frequency : f =
ω
2π = 50π
2π = 25 Hz
Time Period : T =1
f = 1
25 = 0.04 sec
Phase Angle : φ = 0.541 radians (lagging) or=⇒ 0.541 × 180
π = 31◦ (lagging)
b)
When t = 0:
V = 340 sin (50π · 0 − 0.541)
= 340 sin (−0.541) = −175.0978 V
c)
When t = 10 ms:
V = 340 sin (50π · 10−2 − 0.541)
= 340 sin (0.5π − 0.541) = 291.446 V
d)
1
V = 340 sin (50πt − 0.541)
Answer
Sine wave in form:
V = Vm sin (ωt − φ)
has an amplitude of Vm , angular velocity of ω and phase angle (lagging) of φ
radians.
a)
Comparing the given sine wave equation with the standard form, we have:
Amplitude = 340 V
Angular velocity : ω = 50π
frequency : f =
ω
2π = 50π
2π = 25 Hz
Time Period : T =1
f = 1
25 = 0.04 sec
Phase Angle : φ = 0.541 radians (lagging) or=⇒ 0.541 × 180
π = 31◦ (lagging)
b)
When t = 0:
V = 340 sin (50π · 0 − 0.541)
= 340 sin (−0.541) = −175.0978 V
c)
When t = 10 ms:
V = 340 sin (50π · 10−2 − 0.541)
= 340 sin (0.5π − 0.541) = 291.446 V
d)
1
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

When V = 200 V
200 = 340 sin (50πt − 0.541)
=⇒ sin (50πt − 0.541) =
200
340
50πt − 0.541 = sin−1 200
340
50πt − 0.541 = 0.6289
∴ t = 0.6289 + 0.541
50π = 0.007 sec
The sine wave first reaches 200 V at the time t = 0.007 sec.
e)
Maximum voltage occurs when the sine term of the sine wave is maximum, that
is sin (50πt − 0.541) = 1.Implies,
Vmax = 340 · max[sin (50πt − 0.541)]
| {z }
=1
=⇒ sin (50πt − 0.541) = 1
∴ 50πt − 0.541 = sin−1(1)
[sin−1(1) = π
2 + 2n, where, n = 0, 1, 2, . . . ]
∴ 50πt − 0.541 =
π
2 + 2n
=⇒ t = 1
50π
π
2 + 2n + 0.541
The sine wave first reaches it’s maximum value, when n = 0, that is:
t = 1
50π
π
2 + 0.541 = 0.0134 sec
f )
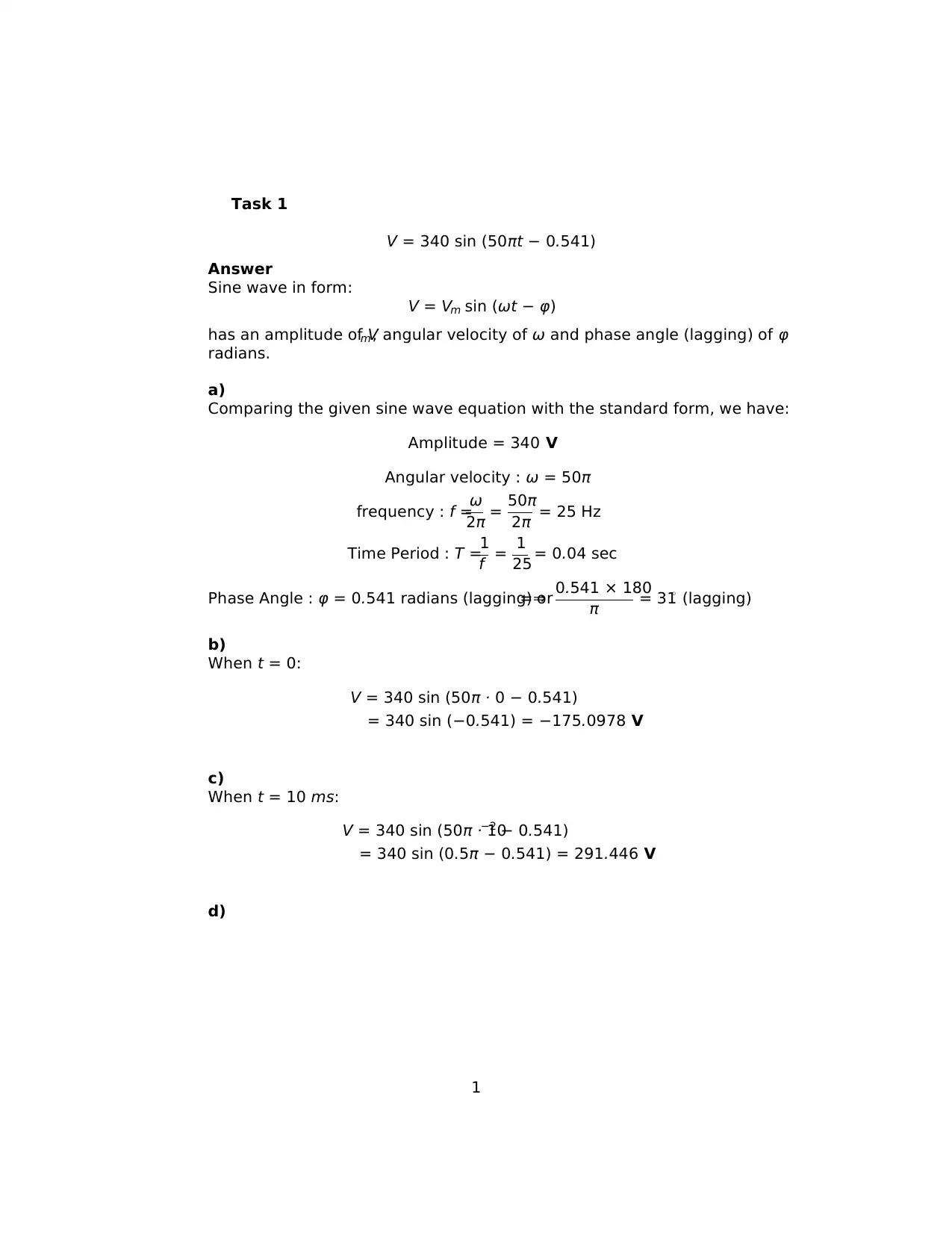
The given sine function is plotted using a graphing calculator (Desmos).The
y-axis is scaled by a factor of10000,so 1 unit which is seen to be 0.005 V
actually represents 50 V.
2
200 = 340 sin (50πt − 0.541)
=⇒ sin (50πt − 0.541) =
200
340
50πt − 0.541 = sin−1 200
340
50πt − 0.541 = 0.6289
∴ t = 0.6289 + 0.541
50π = 0.007 sec
The sine wave first reaches 200 V at the time t = 0.007 sec.
e)
Maximum voltage occurs when the sine term of the sine wave is maximum, that
is sin (50πt − 0.541) = 1.Implies,
Vmax = 340 · max[sin (50πt − 0.541)]
| {z }
=1
=⇒ sin (50πt − 0.541) = 1
∴ 50πt − 0.541 = sin−1(1)
[sin−1(1) = π
2 + 2n, where, n = 0, 1, 2, . . . ]
∴ 50πt − 0.541 =
π
2 + 2n
=⇒ t = 1
50π
π
2 + 2n + 0.541
The sine wave first reaches it’s maximum value, when n = 0, that is:
t = 1
50π
π
2 + 0.541 = 0.0134 sec
f )
The given sine function is plotted using a graphing calculator (Desmos).The
y-axis is scaled by a factor of10000,so 1 unit which is seen to be 0.005 V
actually represents 50 V.
2
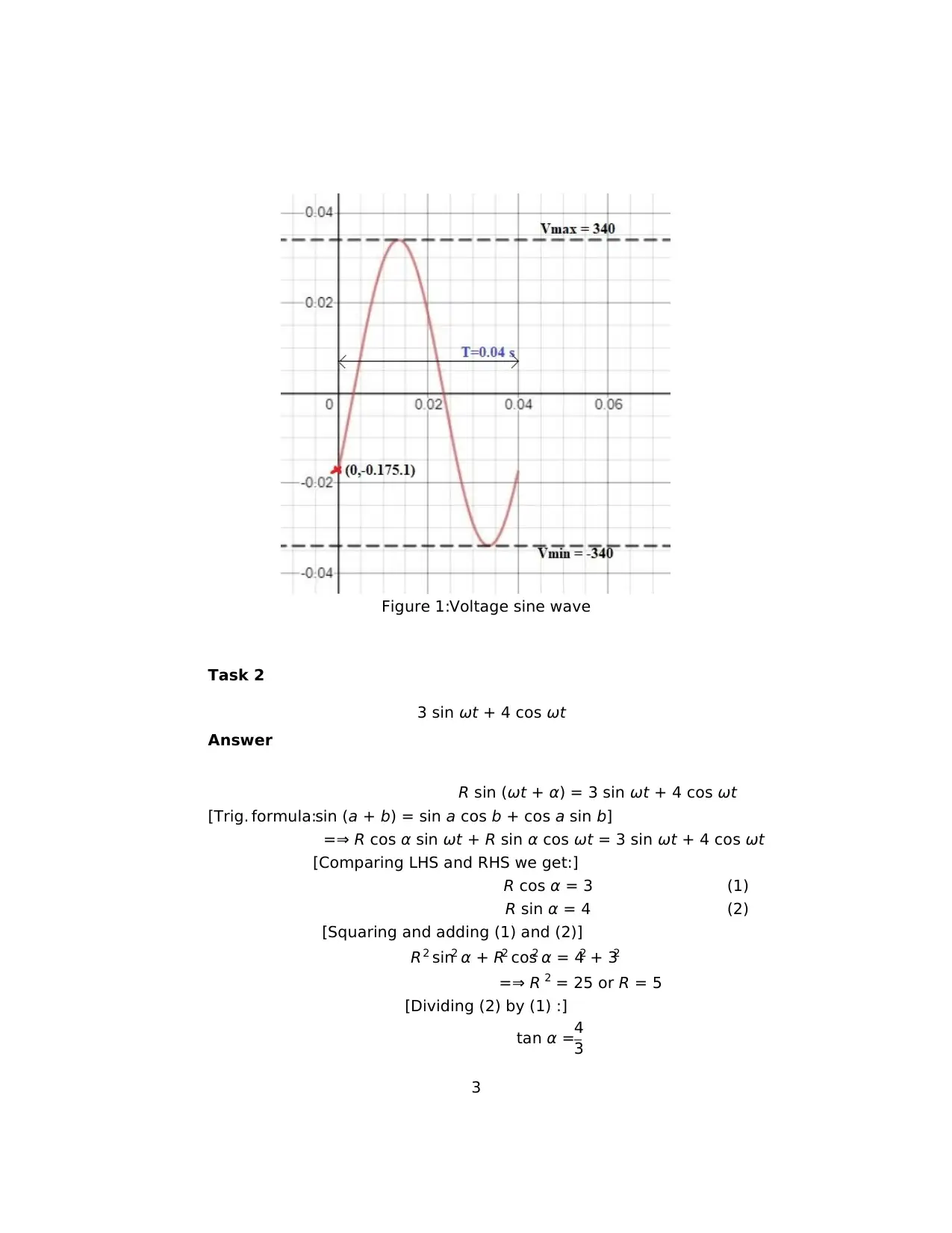
Figure 1:Voltage sine wave
Task 2
3 sin ωt + 4 cos ωt
Answer
R sin (ωt + α) = 3 sin ωt + 4 cos ωt
[Trig. formula:sin (a + b) = sin a cos b + cos a sin b]
=⇒ R cos α sin ωt + R sin α cos ωt = 3 sin ωt + 4 cos ωt
[Comparing LHS and RHS we get:]
R cos α = 3 (1)
R sin α = 4 (2)
[Squaring and adding (1) and (2)]
R2 sin2 α + R2 cos2 α = 42 + 32
=⇒ R 2 = 25 or R = 5
[Dividing (2) by (1) :]
tan α =4
3
3
Task 2
3 sin ωt + 4 cos ωt
Answer
R sin (ωt + α) = 3 sin ωt + 4 cos ωt
[Trig. formula:sin (a + b) = sin a cos b + cos a sin b]
=⇒ R cos α sin ωt + R sin α cos ωt = 3 sin ωt + 4 cos ωt
[Comparing LHS and RHS we get:]
R cos α = 3 (1)
R sin α = 4 (2)
[Squaring and adding (1) and (2)]
R2 sin2 α + R2 cos2 α = 42 + 32
=⇒ R 2 = 25 or R = 5
[Dividing (2) by (1) :]
tan α =4
3
3
You're viewing a preview
Unlock full access by subscribing today!
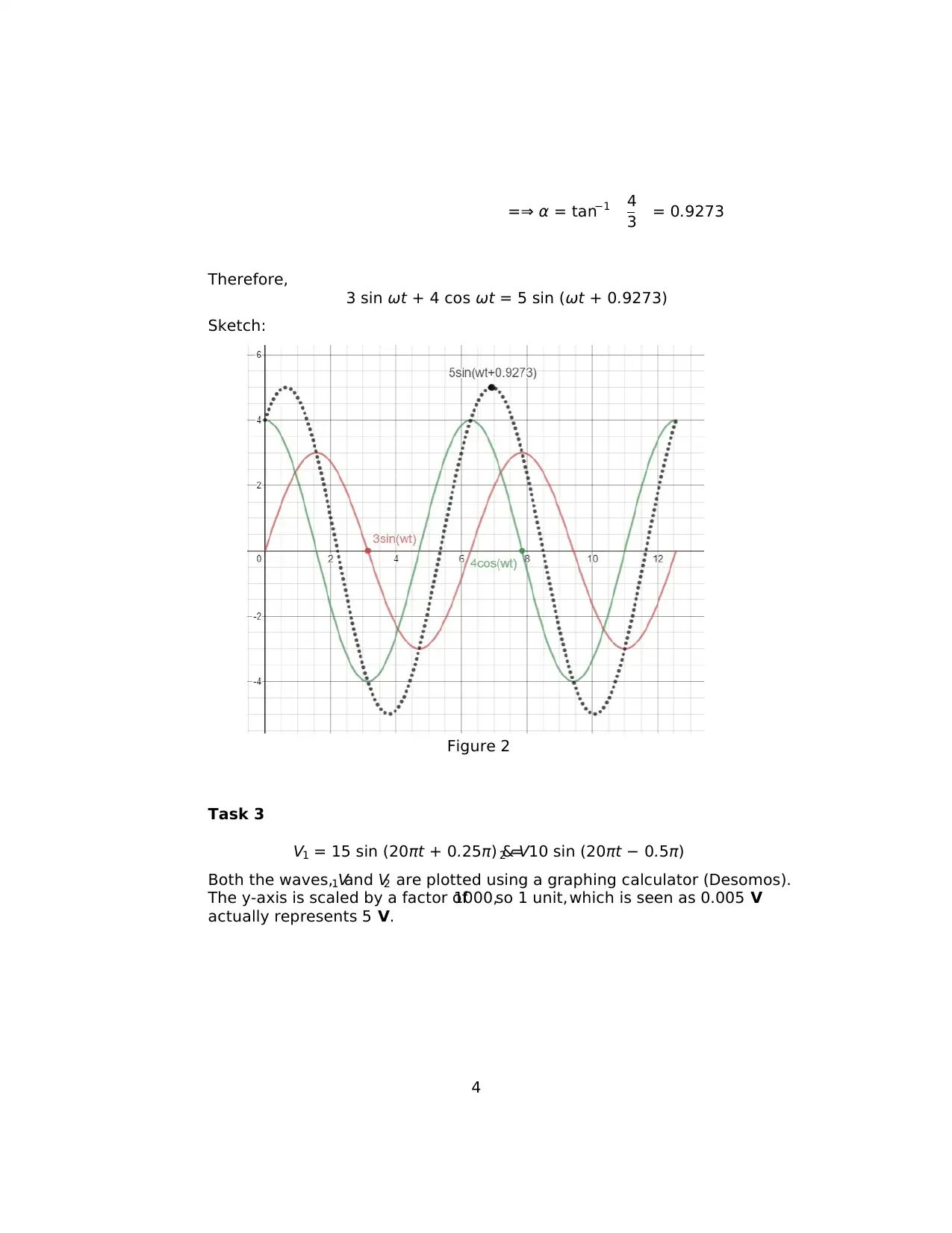
=⇒ α = tan−1 4
3 = 0.9273
Therefore,
3 sin ωt + 4 cos ωt = 5 sin (ωt + 0.9273)
Sketch:
Figure 2
Task 3
V1 = 15 sin (20πt + 0.25π) & V2 = 10 sin (20πt − 0.5π)
Both the waves, V1 and V2 are plotted using a graphing calculator (Desomos).
The y-axis is scaled by a factor of1000,so 1 unit,which is seen as 0.005 V
actually represents 5 V.
4
3 = 0.9273
Therefore,
3 sin ωt + 4 cos ωt = 5 sin (ωt + 0.9273)
Sketch:
Figure 2
Task 3
V1 = 15 sin (20πt + 0.25π) & V2 = 10 sin (20πt − 0.5π)
Both the waves, V1 and V2 are plotted using a graphing calculator (Desomos).
The y-axis is scaled by a factor of1000,so 1 unit,which is seen as 0.005 V
actually represents 5 V.
4
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
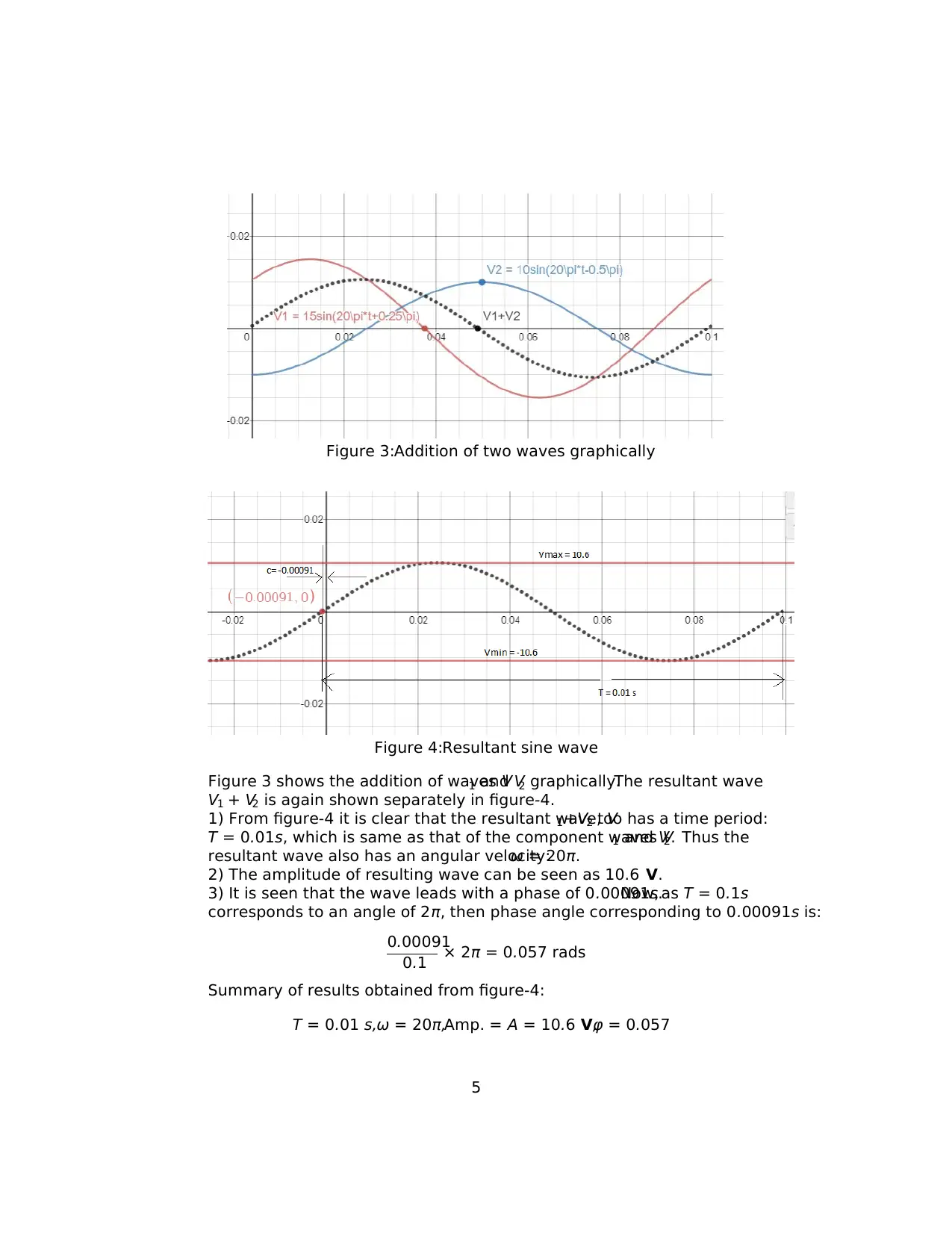
Figure 3:Addition of two waves graphically
Figure 4:Resultant sine wave
Figure 3 shows the addition of waves V1 and V2 graphically.The resultant wave
V1 + V2 is again shown separately in figure-4.
1) From figure-4 it is clear that the resultant wave, V1 +V2 too has a time period:
T = 0.01s, which is same as that of the component waves V1 and V2. Thus the
resultant wave also has an angular velocity:ω = 20π.
2) The amplitude of resulting wave can be seen as 10.6 V.
3) It is seen that the wave leads with a phase of 0.00091s.Now, as T = 0.1s
corresponds to an angle of 2π, then phase angle corresponding to 0.00091s is:
0.00091
0.1 × 2π = 0.057 rads
Summary of results obtained from figure-4:
T = 0.01 s,ω = 20π,Amp. = A = 10.6 V,φ = 0.057
5
Figure 4:Resultant sine wave
Figure 3 shows the addition of waves V1 and V2 graphically.The resultant wave
V1 + V2 is again shown separately in figure-4.
1) From figure-4 it is clear that the resultant wave, V1 +V2 too has a time period:
T = 0.01s, which is same as that of the component waves V1 and V2. Thus the
resultant wave also has an angular velocity:ω = 20π.
2) The amplitude of resulting wave can be seen as 10.6 V.
3) It is seen that the wave leads with a phase of 0.00091s.Now, as T = 0.1s
corresponds to an angle of 2π, then phase angle corresponding to 0.00091s is:
0.00091
0.1 × 2π = 0.057 rads
Summary of results obtained from figure-4:
T = 0.01 s,ω = 20π,Amp. = A = 10.6 V,φ = 0.057
5

When these values are plugged into the standard equation for sine waves:
V = A sin (ωt + φ)
then the equation for resulting wave is:
V1 + V2 = 10.6 sin (20πt + 0.057)
Obtaining the resultant wave equation using trigonometric manipulation:
R sin (ωt + α) = 15 sin (ωt + 0.25π) + 10 sin (ωt − 0.5π)
[Trig. formula:sin (a ± b) = sin a cos b ± cos a sin b]
∴ R cos α sin ωt + R sin α cos ωt = 15 cos (0.25π) sin ωt + 15 sin (0.25π) cos ω
+ 10 cos (0.5π) sin ωt − 10 sin (0.5π) cos ωt
= 10.6 sin ωt + 10.6 cos ωt − 10 cos ωt
[Comparing LHS and RHS]
R cos α = 10.6 (1)
R sin α = 0.6 (2)
[Squaring and adding (1) and (2)]
R2 sin2 α + R2 cos2 α = 10.62 + 0.62
=⇒ R 2 = 112.72 or R = 10.62 ≈ 10.6
[Dividing (2) by (1) ]
tan α = 0.6
10.6
=⇒ α = tan−1 0.6
10.6 = 0.057 rads
Substituting R = 10.6, ω = 20π and α = 0.057 in the resultant wave equation,
we get:
R sin (ωt + α) = 10.6 sin (20πt + 0.057)
This is same as the result obtained from graphical analysis.
Task 4
F1 = h2, −1, 1ir1 = h3, 1, 5i
F2 = h8, −6, 6ir2 = h5, −2, −1i
F3 = h4, 0, 3ir3 = h1, −6, 7i
F4 = h1, −5, 0ir4 = h1, 0, 6i
6
V = A sin (ωt + φ)
then the equation for resulting wave is:
V1 + V2 = 10.6 sin (20πt + 0.057)
Obtaining the resultant wave equation using trigonometric manipulation:
R sin (ωt + α) = 15 sin (ωt + 0.25π) + 10 sin (ωt − 0.5π)
[Trig. formula:sin (a ± b) = sin a cos b ± cos a sin b]
∴ R cos α sin ωt + R sin α cos ωt = 15 cos (0.25π) sin ωt + 15 sin (0.25π) cos ω
+ 10 cos (0.5π) sin ωt − 10 sin (0.5π) cos ωt
= 10.6 sin ωt + 10.6 cos ωt − 10 cos ωt
[Comparing LHS and RHS]
R cos α = 10.6 (1)
R sin α = 0.6 (2)
[Squaring and adding (1) and (2)]
R2 sin2 α + R2 cos2 α = 10.62 + 0.62
=⇒ R 2 = 112.72 or R = 10.62 ≈ 10.6
[Dividing (2) by (1) ]
tan α = 0.6
10.6
=⇒ α = tan−1 0.6
10.6 = 0.057 rads
Substituting R = 10.6, ω = 20π and α = 0.057 in the resultant wave equation,
we get:
R sin (ωt + α) = 10.6 sin (20πt + 0.057)
This is same as the result obtained from graphical analysis.
Task 4
F1 = h2, −1, 1ir1 = h3, 1, 5i
F2 = h8, −6, 6ir2 = h5, −2, −1i
F3 = h4, 0, 3ir3 = h1, −6, 7i
F4 = h1, −5, 0ir4 = h1, 0, 6i
6
You're viewing a preview
Unlock full access by subscribing today!

Answer
The equilibrium of a body is determined by the condition:
(r1 × F1) + (r2 × F2) + (r3 × F3) + (r4 × F4) = 0
Substituting the given force and position vectors in the LHS of the above equa-
tion:
(h3, 1, 5i×h2, −1, 1i)+(h5, −2, −1i×h8, −6, 6i)+(h1, −6, 7i×h4, 0, 3i)+(h1, 0, 6i×h1, −5, 0i)
First carry out the cross products individually and then add the results:
h3, 1, 5i × h2, −1, 1i =
ˆi ˆj ˆk
3 1 5
2 −1 1
= (1 + 5)ˆi − (3 − 10)ˆj + (−3 − 2)ˆk
= h6, 7, −5i
h5, −2, −1i × h8, −6, 6i =
ˆi ˆj ˆk
5 −2 −1
8 −6 6
= (−12 − 6)ˆi − (30 + 8)ˆj + (−30 + 16)ˆk
= h−18, −38, −14i
h1, −6, 7i × h4, 0, 3i =
ˆi ˆj ˆk
1 −6 7
4 0 3
= (−18 − 0)ˆi − (3 − 28)ˆj + (0 + 24)ˆk
= h−18, 25, 24i
h1, 0, 6i × h1, −5, 0i =
ˆi ˆj ˆk
1 0 6
1 −5 0
= (0 + 30)ˆi − (0 − 6)ˆj + (−5 + 0)ˆk
= h30, 6, −5i
Adding all the results together:
(h3, 1, 5i × h2, −1, 1i) + (h5, −2, −1i × h8, −6, 6i)+
(h1, −6, 7i × h4, 0, 3i) + (h1, 0, 6i × h1, −5, 0i) = h6, 7, −5i + h−18, −38, −14i+
h−18, 25, 24i + h30, 6, −5i
= h6 − 18 − 18 + 30, 7 − 38 + 25 + 6, −5 − 14 + 2
= h0, 0, 0i
7
The equilibrium of a body is determined by the condition:
(r1 × F1) + (r2 × F2) + (r3 × F3) + (r4 × F4) = 0
Substituting the given force and position vectors in the LHS of the above equa-
tion:
(h3, 1, 5i×h2, −1, 1i)+(h5, −2, −1i×h8, −6, 6i)+(h1, −6, 7i×h4, 0, 3i)+(h1, 0, 6i×h1, −5, 0i)
First carry out the cross products individually and then add the results:
h3, 1, 5i × h2, −1, 1i =
ˆi ˆj ˆk
3 1 5
2 −1 1
= (1 + 5)ˆi − (3 − 10)ˆj + (−3 − 2)ˆk
= h6, 7, −5i
h5, −2, −1i × h8, −6, 6i =
ˆi ˆj ˆk
5 −2 −1
8 −6 6
= (−12 − 6)ˆi − (30 + 8)ˆj + (−30 + 16)ˆk
= h−18, −38, −14i
h1, −6, 7i × h4, 0, 3i =
ˆi ˆj ˆk
1 −6 7
4 0 3
= (−18 − 0)ˆi − (3 − 28)ˆj + (0 + 24)ˆk
= h−18, 25, 24i
h1, 0, 6i × h1, −5, 0i =
ˆi ˆj ˆk
1 0 6
1 −5 0
= (0 + 30)ˆi − (0 − 6)ˆj + (−5 + 0)ˆk
= h30, 6, −5i
Adding all the results together:
(h3, 1, 5i × h2, −1, 1i) + (h5, −2, −1i × h8, −6, 6i)+
(h1, −6, 7i × h4, 0, 3i) + (h1, 0, 6i × h1, −5, 0i) = h6, 7, −5i + h−18, −38, −14i+
h−18, 25, 24i + h30, 6, −5i
= h6 − 18 − 18 + 30, 7 − 38 + 25 + 6, −5 − 14 + 2
= h0, 0, 0i
7
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Thus, the equilibrium condition is satisfied and the body seems to maintain
equilibrium under the given condition.
References:
Ayres, F. and Mendelson, E. (2013).Calculus.New York:McGraw-Hill.
Haykin,S. (2014).Digitalcommunication systems.Hoboken,NJ: J. Wiley &
Sons.
8
equilibrium under the given condition.
References:
Ayres, F. and Mendelson, E. (2013).Calculus.New York:McGraw-Hill.
Haykin,S. (2014).Digitalcommunication systems.Hoboken,NJ: J. Wiley &
Sons.
8
1 out of 8
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.





