Trigonometric Methods for HNC/HND Electrical and Electronic Engineering

This article discusses the application of sine and cosine rules in solving engineering problems, the characteristics of sinusoidal waveform, and the use of trigonometric identities to expand and simplify formulae. It also includes basic operations of multiplication and division to complex numbers and expressing them in both rectangular and polar form.

Running head: HNC/HND ELECTRICAL AND ELECTRONIC ENGINEERING 1
Trigonometric Methods
Student Name
University

HNC/HND ELECTRICAL AND ELECTRONIC ENGINEERING 2
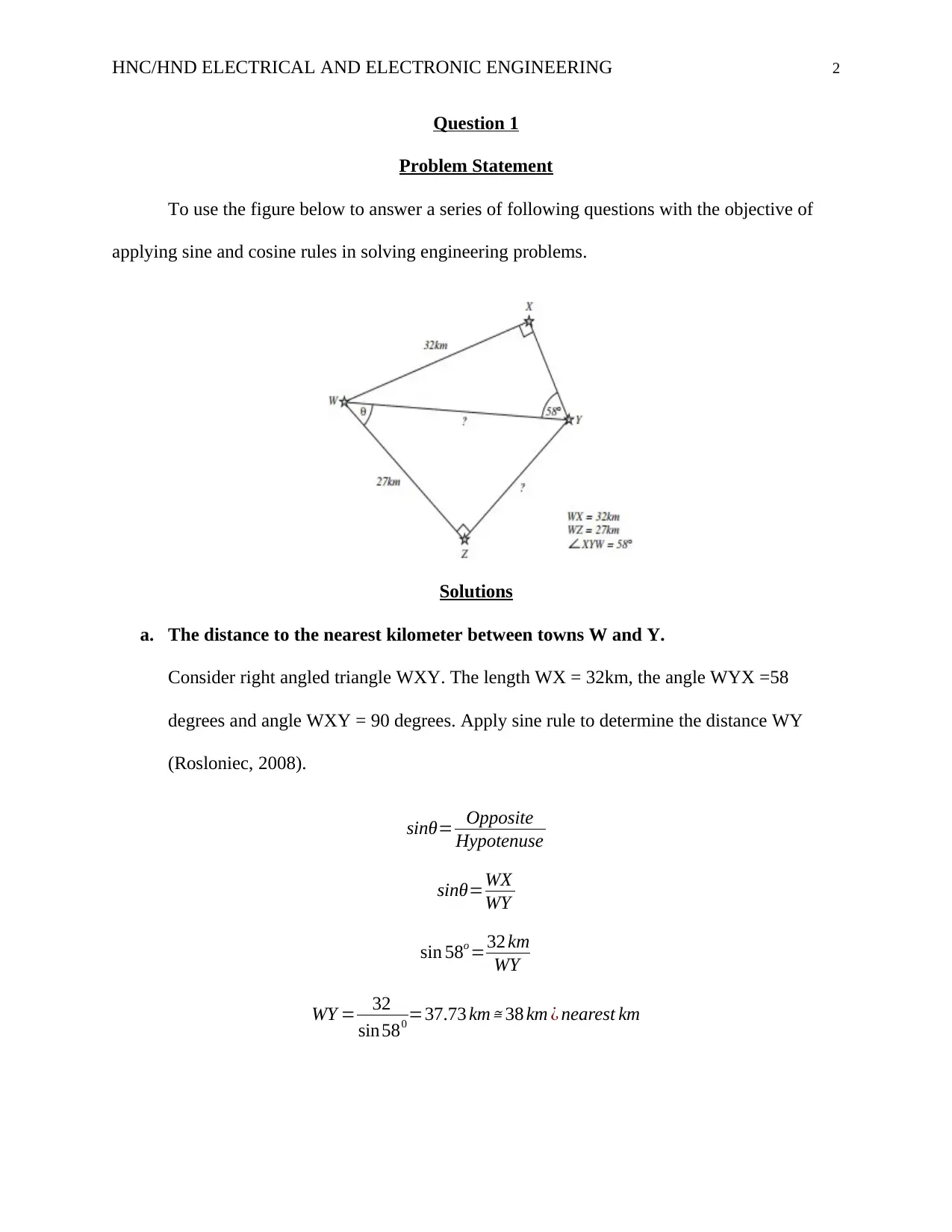
Question 1
Problem Statement
To use the figure below to answer a series of following questions with the objective of
applying sine and cosine rules in solving engineering problems.
Solutions
a. The distance to the nearest kilometer between towns W and Y.
Consider right angled triangle WXY. The length WX = 32km, the angle WYX =58
degrees and angle WXY = 90 degrees. Apply sine rule to determine the distance WY
(Rosloniec, 2008).
sinθ= Opposite
Hypotenuse
sinθ=WX
WY
sin 58o =32 km
WY
WY = 32
sin580 =37.73 km≅ 38 km ¿ nearest km

HNC/HND ELECTRICAL AND ELECTRONIC ENGINEERING 3
Therefore, the distance between towns W and Y to the nearest kilometer is equal to
38km.
b. The size of the angle marked θ to the nearest degree.
Consider the right angle triangle WZY, the length WZ = 27km, the length WY found
previously to the nearest kilometer is equal to 38km. Apply the cosine rule (Rosloniec,
2008).
cosθ= Adjacent
Hypotenuse
cosθ= WZ
WY
cosθ= 27 km
38 km
cosθ=0.7105
θ=cos−1 0.7105=44.72 ≅ 45o
Hence the angle YWZ to the nearest degree is equivalent to 45o
c. The distance between towns Y and Z to the nearest kilometer.
Consider right angled triangle WZY. The length WZ = 27km, the angle ZWY= 45
degrees. The angle ZYW will be given by:
ZYW =180−(YZW + ZWY )
ZYW =180− ( 90+45 )
ZYW =45o
Apply cosine rule using angle ZYW and length WY:
cosθ= Adjacent
Hypotenuse
cosθ= ZY
WY

HNC/HND ELECTRICAL AND ELECTRONIC ENGINEERING 4
cos 45= xkm
38 km
ZY =38 cos 45=26.87 Km ≅ 27 km
Alternatively, the sine rule can be applied directly as follows using angle ZWY and the
distance WY.
sinθ= Opposite
Hypotenuse
sin 45= ZY
WY
sin 45o= xkm
38
ZY =38 sin 45=26.87 ≅ 27 km
Hence the distance ZY to the nearest km is equal to 27km
Question 2
Problem Statement
To use the value of instantaneous current, I Amperes, at T seconds to find the value of
amplitude, the period T, the frequency and use the same equation of current to solve engineering
numerical problems with the aim of demonstrating the understanding of sinusoidal waveform
characteristics and apply them in solving engineering problems.
The value of instantaneous current given is given by:
i=15 sin (100 πt+ 0.6)
Solutions
a. The value of amplitude:

HNC/HND ELECTRICAL AND ELECTRONIC ENGINEERING 5
The amplitude denoted by A is the intensity or the magnitude of the sinusoidal current in
Amperes. Alternatively, it is the maximum displacement from the mean position
(Subramanyam, 2008). In the sinusoidal waveform, it is the coefficient of the sin
function. Hence:
Amplitude A=15 A
b. The value of the period of the wave:
Period is the time it takes the sinusoidal waveform to complete a single oscillation
(Subramanyam, 2008). It is given by:
T = 1
f
But,
f = ω
2 π = 100 π
2 π =50 Hz
Therefore,
T = 1
50 =0.02 s
The period or the time taken by the waveform to complete a single oscillation is therefore
0.02s
c. The value of frequency of the wave:
Frequency refers to the number of oscillations completed within a unit time
(Subramanyam, 2008). It is given by:
F= 1
T = 1
0.02 =50 Hz
Therefore, the frequency of the waveform is equal to 50Hz.
d. The initial phase angle (when t=0), expressed in both radians and degrees.

HNC/HND ELECTRICAL AND ELECTRONIC ENGINEERING 6
The current waveform is:
i=15 sin (100 πt+ 0.6)
Substituting the value of the time t=0 to the equation of the waveform:
i=15 sin (100 π ( 0)+ 0.6)
i=15 sin (0.6)
Hence the initial phase angle = 0.6 radians
To convert the radians to degrees:
1 radian=57.3 degrees
Therefore 0.6 radians will be:
0.6
1 x 57.3=34.38 degrees
Alternatively, to convert radians to degrees we can use the following expression,
180
π x 0.6=34.38 degrees
The initial phase angle in degrees is equal to 34.38o.
e. The value of I when t = 2.5s.
The current waveform is:
i=15 sin (100 πt+ 0.6)
Substituting the value of the time t=0 to the equation of the waveform:
i=15 sin (100 π (2.5)+0.6)
i=15 sin (785.40+0.6)
i=15 sin (786)
i=15 sin ( 786 ) =13.70 A
The value of current at t = 2.5s is equal to 13.07A.

HNC/HND ELECTRICAL AND ELECTRONIC ENGINEERING 7
f. The time (in milliseconds) when the current first reaches its maximum value.
The current reaches the maximum value when the sin function is maximum (equal to 1).
Therefore, we equate the sin function to 1.
sin ( 100 πt +0.6 )=1
100 πt +0.6=sin−1 1
100 πt +0.6 rads=90∈degrees
100 πt +34.38 degress=90 degrees
t= 55.62
100 π =0.17704 seconds
To covert the seconds to milliseconds, multiply by 1000.
T ( ms ) =0.177 x 1000=177.04 ms
The current therefore takes 177.04ms to reach maximum value.
Question 3
Problem Statements
To use trigonometric identities to expand and simplify formulae. The objective is to
demonstrate an understanding of applying compound angle formulae to prove the identity and
solve engineering problems.
Solutions
Part A
i. Cos (270o – θ)
The above equation can be re-written as:

HNC/HND ELECTRICAL AND ELECTRONIC ENGINEERING 8
cos (180o ¿+900−θ) ¿
cos [(180o )¿+(900−θ)]¿
But,
cos (180o ¿+θ)=−cos θ ¿
Therefore
cos [(180o )¿+( 900−θ)]=−cos (900 −θ)¿
However,
cos (90¿¿ 0−θ)=sinθ ¿
Therefore,
−cos (90¿ ¿ 0−θ)=−sin (θ) ¿
ii. Sin (270o – θ)
The above equation can be re-written as:
sin(180o ¿+900 −θ)¿
sin [(180o )¿+(900−θ)]¿
But,
sin(180o ¿+θ)=−sin θ ¿
Therefore,
sin [(180o )¿+(900−θ)]=−sin (900−θ) ¿
However,
sin( 90¿¿ 0−θ)=cosθ ¿
Therefore,
−sin (90¿¿ 0−θ)=−cos (θ) ¿
iii. Cos (270o + θ)

HNC/HND ELECTRICAL AND ELECTRONIC ENGINEERING 9
cos (180o ¿+900+ θ)¿
cos [(180o )¿+( 900 +θ)]¿
But,
cos (180o ¿+θ)=−cos θ ¿
Therefore,
cos [(180o )¿+(900 +θ)]=−cos (900 +θ)¿
However,
cos (90¿¿ 0+θ)=−sinθ¿
Therefore,
−cos (90¿ ¿ 0+θ)=−(−sin ( θ))=sinθ ¿
Part B
Given value of voltage V1 and V2 as V 1=3 sin (ωt ) and V 2=2 cos (ωt ). The value of V3 is the
sum of V1 and V2 such that V 3=V 1 +V 2. We find the expression of V3 in sine waveform such that
V 3=R sin (ωt +α ) and verify that the resultant voltage V3 is in the same frequency as V1 and V2.
Solution
V 3=V 1 +V 2
V 3=3 sin ( ωt ) +2 cos (ωt )
The equation above can be re-written in the form
3 sin ( ωt ) +2 cos ( ωt ) =R ( cosa ) ¿
But,
Rcosa=3∧Rsina=2
However,
( Rcosθ )2 + ( Rsinθ )2=R2 since cos2 θ+sin2 θ=1

HNC/HND ELECTRICAL AND ELECTRONIC ENGINEERING 10
Therefore,
32 +22=R2=13
R= √13=3.606
Consequently,
Rsina
Rcosa =tan a
Therefore,
2
3 =tan a
a=tan−1 2
3 =33.69
Thus,
V 3=3.606 sin ( wt +33.69 ) V
To verify that V1, V2, and V3 belong in the same frequency.
The frequency of V1:
Fv 1= ω
2 π
The frequency of V2:
Fv 2= ω
2 π
The frequency of V3:
Fv 3= ω
2 π
We see that
The frequency of V1 is equal to frequency of V2 and is equal to V3, hence they belong to the same
frequency
Fv 1=Fv2 =F v3

HNC/HND ELECTRICAL AND ELECTRONIC ENGINEERING 11
Question 4
Problem Statement
To perform basic operations of multiplication and division to complex numbers and express the
complex numbers in both rectangular and polar form.
Part A
Z= Z1 Z2
Z1+ Z2
Z1 =4+ j 10∧Z2=12− j3
Z1 Z2=(4+ j10)(12− j3)
Z1 Z2=4 (12− j3)+ j 10(12− j3)
Z1 Z2=48− j12+ j120−30( j2)
Z1 Z2=48+ j108+30
Z1 Z2=78+ j 108
Consequently,
Z1 +Z2= ( 4+ j 10 )+(12− j 3)
Z1 + Z2=16+ j 7
Therefore,
Z1 Z2
Z1 +Z2
= 78+ j 108
16 + j 7
Multiply both sides by the conjugate of the denominator:
78+ j 108
16 + j 7 x 16− j 7
16− j 7
78 (16− j7 )+ j108 (16− j7)
305
1248− j546+ j1728+756
305

HNC/HND ELECTRICAL AND ELECTRONIC ENGINEERING 12
2004+ j1182
305
Therefore, in rectangular form,
Z1 Z2
Z1 +Z2
=6.57+ j3.88
In polar form,
R<θ
R= √ ( real part )2 + ( imaginary part )2
R= √ ( 6.57 )2+ ( 3.88 )2=7.63
θ=tan−1 ( imaginary part
Real part )
θ=tan−1
( 3.88
6.57 )=30.56
Hence in polar form:
Z1 Z2
Z1 +Z2
=7.63<30.56o
Part B
Admittance is given by:
Y = 1
Z1
+ 1
Z2
+ 1
Z3
And Z1 =2+ J 2, Z2 =1+ J 5, Z3 =J 6
Now,
1
Z1
= 1
2+ j 2 =0.25− j 0.25
1
Z2
= 1
1+ j5 =0.0385− j0.192
1
Z3
= 1
j 6 =− j 0.167

HNC/HND ELECTRICAL AND ELECTRONIC ENGINEERING 13
Therefore, admittance in rectangular form,
Y =[ ( 0.25− j 0.25 )+ ( 0.0385− j0.192 ) + (− j 0.167 ) ]
Y =0.2885− j 0.609
The admittance in polar form is:
R<θ
R= √ ( real part )2 + ( imaginary part )2
R= √ ( 0.2885 ) 2+ ( 0.609 ) 2=0.674
θ=tan−1 ( imaginary part
Real part )
θ=tan−1
( 0.609
0.2885 )=64.65o
Hence in polar form, the admittance is:
0.674< 64.65o
Question 5
Problem Statement
To apply appropriate mathematical techniques to solve engineering problems and be able to
produce appropriate phasor diagrams. The given potential difference across a circuit is given by:
V =40+ j35 Volts
The current in the circuit is given by:
I =6+ j3 Amps
Solutions
a. To sketch the appropriate phasors on an Argand diagram

HNC/HND ELECTRICAL AND ELECTRONIC ENGINEERING 14
The real part is plotted on the x-axis whereas the imaginary part is plotted on the Y-axis
as shown below.
b. The phase difference (i.e. the angle ∅ ) between the phasors for voltage V and the
current I.
Phase angle for voltage:
Tan−1 35
40 =41.19o
The phase angle for current:
Ta n−1 3
6 =26.57o
The difference in phase angle of voltage and current:
41.19−26.57=14.62o
c. The power given that

HNC/HND ELECTRICAL AND ELECTRONIC ENGINEERING 15
power=|V |x|I |x cos(∅ )
|V |= √ ¿ ¿
|I |= √ ¿ ¿
cos ( ∅ ) =cos ( 14.62 )=0.97o
Therefore, power will be:
P=53.15 x 6.71 x 0.97=345.94 Watts
P=345.94 watts

HNC/HND ELECTRICAL AND ELECTRONIC ENGINEERING 16
References
Rosloniec, S. (2008). Fundamental numerical methods for electrical engineering (2nd ed.).
Berlin, Heidelberg: Springer-Verlag Berlin Heidelberg.
Subramanyam, P. S. (2008). Basic concepts of electrical engineering (3rd ed.). Hyderabad: BS
Publications.